Two thin wood screens A and B are separated by 200m. A bullet travelling horizontally at a speed of 600ms−1 hits the screen A, penetrates through it and finally emerges out from B making holes in A and B. If the resistance of air and wood arc negligible, the difference of heights of the holes in A and B is
5 m
4990 m
7√90 m
zero
The correct Answer is:B
To solve the problem step by step, we need to analyze the motion of the bullet as it travels horizontally and the effect of gravity on its vertical position.
Step 1: Identify the given data
- Distance between screens A and B, D=200m
- Speed of the bullet, v=600m/s
- Acceleration due to gravity, g=9.8m/s2
Step 2: Calculate the time taken for the bullet to travel from A to B
Using the formula for time:
t=Dv
Substituting the values:
t=200m600m/s=13s
Step 3: Analyze the vertical motion of the bullet
Since the bullet is moving horizontally, its initial vertical velocity u=0. The vertical displacement h after time t can be calculated using the second equation of motion:
s=ut+12at2
Here, a=g (acceleration due to gravity), so:
h=0⋅t+12gt2
Substituting t=13s:
h=12⋅9.8⋅(13)2
Calculating (13)2=19:
h=12⋅9.8⋅19=9.818=4990m
Step 4: Conclusion
The difference in heights of the holes in screens A and B is:
h=4990m
- Distance between screens A and B, D=200m
- Speed of the bullet, v=600m/s
- Acceleration due to gravity, g=9.8m/s2
Using the formula for time:
t=Dv
Substituting the values:
t=200m600m/s=13s
Since the bullet is moving horizontally, its initial vertical velocity u=0. The vertical displacement h after time t can be calculated using the second equation of motion:
s=ut+12at2
Here, a=g (acceleration due to gravity), so:
h=0⋅t+12gt2
Substituting t=13s:
h=12⋅9.8⋅(13)2
Calculating (13)2=19:
h=12⋅9.8⋅19=9.818=4990m
The difference in heights of the holes in screens A and B is:
h=4990m
Similar Questions
Two paper screens A and B are separated by 150m. A bullet pierces A and B. The hole in B in 15 cm below the hole in A. If the bullet is travelling horizontally at the time of hitting A, then the velocity of the bullet at A is: (g=10ms−2)
View SolutionTwo paper screens A and B are separated by a distance of 100m. A bullet pierces A and B. The hole in B is 10cm below the hole in A. If the bullet is travelling horizontally at the time of hitting the screen A, calculate the velocity of the bullet when it hits the screen A. Neglect resistance of paper and air.
View SolutionIf two objects A and B of same volume and same density are dropped from a height of 200 m, then (neglect air resistance)
View SolutionTwo particles A and B have the same mass m. A is moving along X-axis with a speed of 5ms−1 and B is at rest. After undergoing a perfectly elastic collision with B, particle A gets scattered through an angle of 60∘. What is the direction of B, and the speeds of A and B, after the collision ?
View SolutionA horizontal thin tube of length 2L completely filled with a liquid of density δ rotates about a vertical axis passing through one of its end and along the diameter of its side face with an constant angular velocity ω. Two vertical thin long tubes are fitted with the horizontal tube to measure the pressure difference between points A and B which are at same horizontal level as shown. Neglect viscous forces and surfaces tension of liquid.

The difference in the heights (h) of the liquid column in the two vertical thin tubes is :
View SolutionA travelling wave has speeds 50m/sand200m/s in two different media AandB . Such a wave travelling through A , gets incident normally on a plane boundary , separating AandB. Find the ratio of amplitudes of the reflected anf transmitted waves.
View SolutionTwo cars A and B are moving away from each other in opposite directions. Both the cars are moving with a speed of 20ms−1 with respect to the ground. If an observer in car A detects a frequency 2000 Hz of the sound coming from car B, what is the natural frequency of the sound source in car B? (Speed of sound in air =340ms−1)
View SolutionA bullet of mass 2g travelling at a speed of 500m/s is fired into a ballistic pendulum of mass 1.0kg suspended from a cord 1.0m long. The bullet penetrates the pendulum and emerges with a velocity of 100m/s. Through what vertical height will the pendulum rise?
View SolutionA motor cyclist is trying to jump across a path as shown by driving horizontally off a cliff A at a speed of 5ms−1. Ignore air resistance and take g=10ms−2. The speed with which he touches the peak B is:
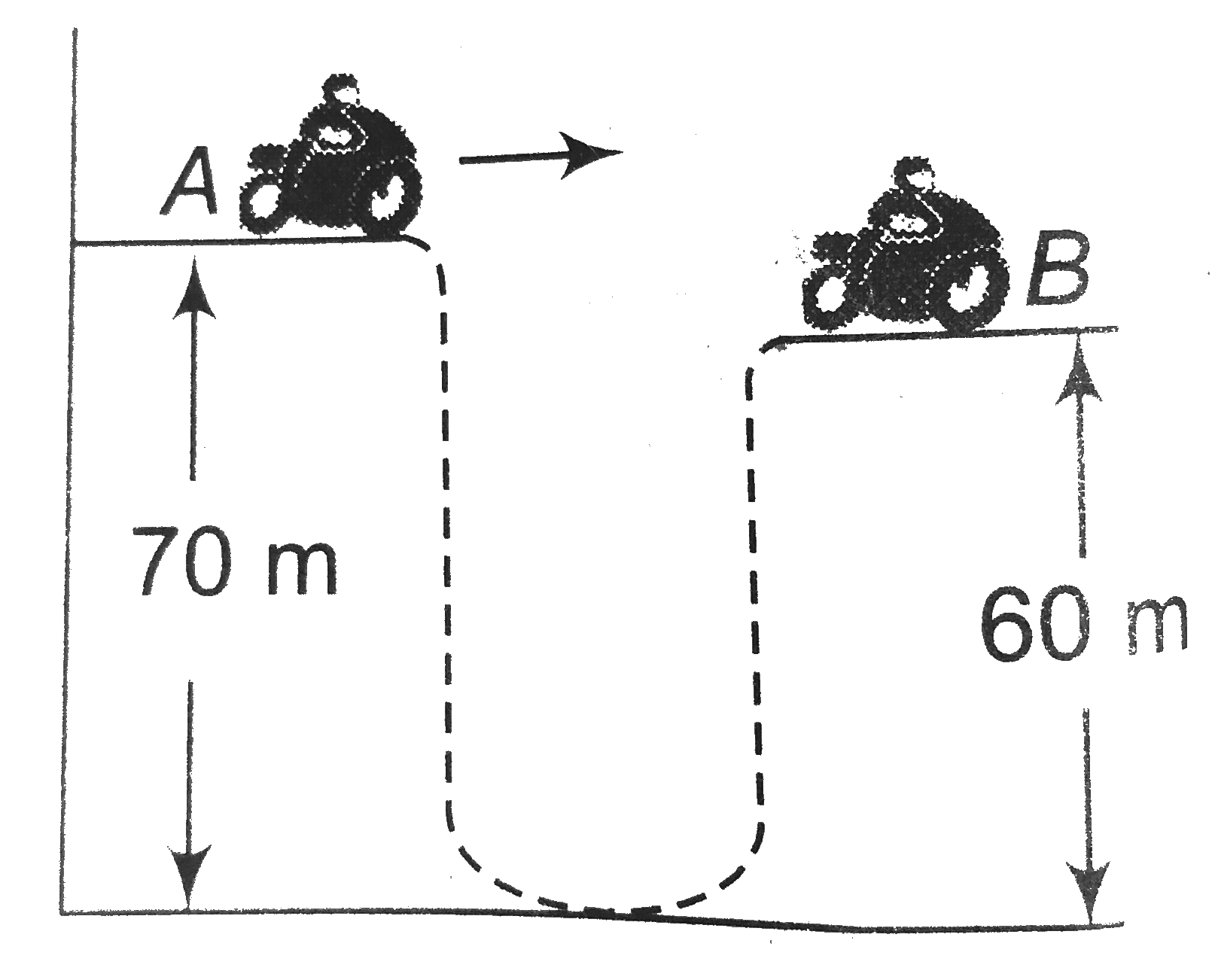
View SolutionA particle is projected from a point A vertically upwards with a speed of 50ms−1 and another is dropped simultaneously from B which is 200 m vertically above A. They cross each other after [Given: g=10ms−2].
View Solution
