What is γ ? Obtain the value of γ for (i) monatomic (ii) diatomic and (iii) triatomic gas.
To solve the question regarding the value of γ (gamma) for different types of gases, we will follow these steps:
Step 1: Understand the Definition of γ
γ (gamma) is defined as the ratio of the heat capacity at constant pressure (Cₚ) to the heat capacity at constant volume (Cᵥ). Mathematically, it can be expressed as:
γ=CpCv
Step 2: Relate Heat Capacities to Degrees of Freedom
For an ideal gas, the heat capacities can be expressed in terms of the degrees of freedom (F) of the gas:
- The formula for heat capacity at constant pressure is:
Cp=F+22R
- The formula for heat capacity at constant volume is:
Cv=F2R
Step 3: Derive the Expression for γ
Substituting the expressions for Cₚ and Cᵥ into the formula for γ gives:
γ=CpCv=F+22RF2R
This simplifies to:
γ=F+2F
Step 4: Calculate γ for Different Types of Gases
Now, we will calculate γ for monatomic, diatomic, and triatomic gases based on their degrees of freedom.
(i) For Monatomic Gases
- Monatomic gases have 3 translational degrees of freedom, so:
F=3
Substituting into the expression for γ:
γ=3+23=53≈1.67
(ii) For Diatomic Gases
- Diatomic gases have 5 degrees of freedom (3 translational + 2 rotational), so:
F=5
Substituting into the expression for γ:
γ=5+25=75≈1.4
(iii) For Triatomic Gases
Triatomic gases can be linear or non-linear.
- Linear Triatomic Gases: They have 5 degrees of freedom (3 translational + 2 rotational), so:
F=5
Substituting into the expression for γ:
γ=5+25=75≈1.4
- Non-linear Triatomic Gases: They have 6 degrees of freedom (3 translational + 3 rotational), so:
F=6
Substituting into the expression for γ:
γ=6+26=86=43≈1.33
Final Values of γ
- For Monatomic Gases: γ=53≈1.67
- For Diatomic Gases: γ=75≈1.4
- For Linear Triatomic Gases: γ=75≈1.4
- For Non-linear Triatomic Gases: γ=43≈1.33
γ (gamma) is defined as the ratio of the heat capacity at constant pressure (Cₚ) to the heat capacity at constant volume (Cᵥ). Mathematically, it can be expressed as:
γ=CpCv
For an ideal gas, the heat capacities can be expressed in terms of the degrees of freedom (F) of the gas:
- The formula for heat capacity at constant pressure is:
Cp=F+22R
- The formula for heat capacity at constant volume is:
Cv=F2R
Substituting the expressions for Cₚ and Cᵥ into the formula for γ gives:
γ=CpCv=F+22RF2R
This simplifies to:
γ=F+2F
Now, we will calculate γ for monatomic, diatomic, and triatomic gases based on their degrees of freedom.
- Monatomic gases have 3 translational degrees of freedom, so:
F=3
Substituting into the expression for γ:
γ=3+23=53≈1.67
- Diatomic gases have 5 degrees of freedom (3 translational + 2 rotational), so:
F=5
Substituting into the expression for γ:
γ=5+25=75≈1.4
Triatomic gases can be linear or non-linear.
F=5
Substituting into the expression for γ:
γ=5+25=75≈1.4
F=6
Substituting into the expression for γ:
γ=6+26=86=43≈1.33
- For Monatomic Gases: γ=53≈1.67
- For Diatomic Gases: γ=75≈1.4
- For Linear Triatomic Gases: γ=75≈1.4
- For Non-linear Triatomic Gases: γ=43≈1.33
Topper's Solved these Questions
- INTERNAL ENERGY BOOK - ICSECHAPTER - INTERNAL ENERGY EXERCISE - VERY SHORT ANSWER QUESTIONS
17 Videos
- INTERNAL ENERGY BOOK - ICSECHAPTER - INTERNAL ENERGY EXERCISE - SELECTED PROBLEMS (FROM FIRST LAW OF THERMODYNAMICS )
6 Videos
- INTERNAL ENERGY BOOK - ICSECHAPTER - INTERNAL ENERGY EXERCISE - LONG ANSWER QUESTIONS
8 Videos
- GRAVITATIONBOOK - ICSECHAPTER - GRAVITATIONEXERCISE - FROM THE HUBBLE TELESCOP
2 Videos
- MOTION IN FLUIDS BOOK - ICSECHAPTER - MOTION IN FLUIDS EXERCISE - SELECTED PROBLEMS (FROM POISEUILLE.S FORMULA)
19 Videos
Similar Questions
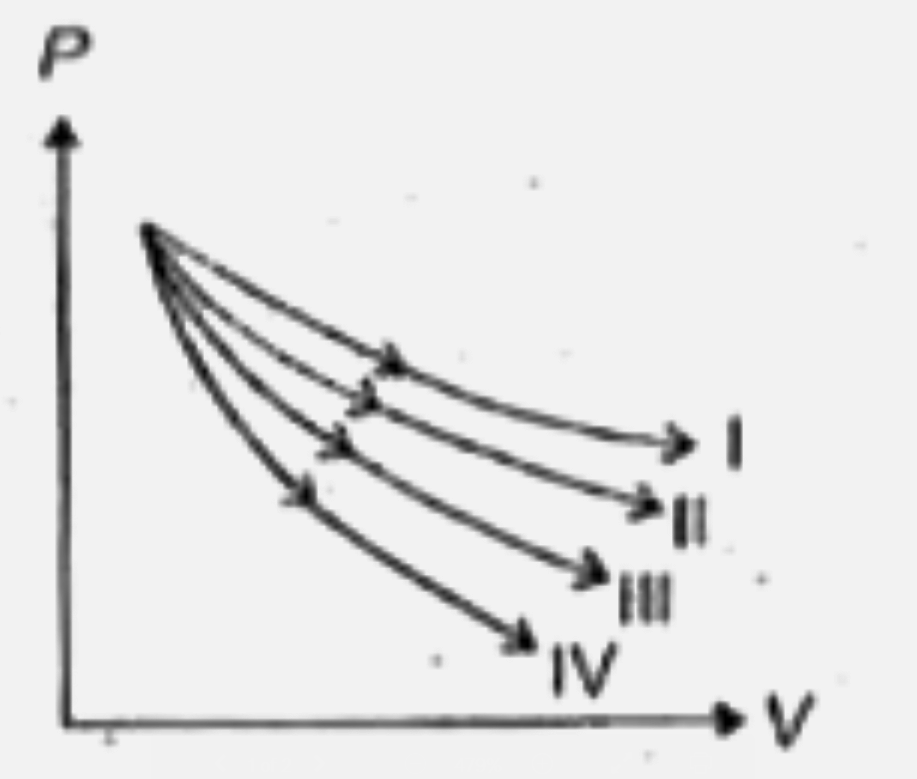
In the following plots match I,II, III,Iv with (a) isothermal process and Adiabatic process for (b) monatomic, (c ) diatomic and (d) triatomic gases respectively.
View SolutionWhat are (i) audible frequency, (ii) infra-sonics and (iii) ultra-sonics ?
View SolutionValue of Cp for monatomic gas is 52R. Find CV.
View SolutionFind the values of the following :
(i) i73
(ii) i−6
(iii) 1i
View SolutionThe value of CV for monatomic gas is 32R, then CP will be
View SolutionThe straight lines in the figure depict the variations in temperature ΔT as a function of the amount of heat supplied Q is different process involving the change of state of a monoatomic and a diatomic ideal gas . The initial states, (P,V,T) of the two gases are the same . Match the processes as described, with the straight lines in the graph as numbered.
_E01_155_Q01.png)
Column-IColumn-II(A)Isobaric process of monoatomic gas.(p)1(B)Isobaric process of diatomic gas(q)2(C)Isochoric process of monoatomic gas(r)Minimum at section B(D)Isochoric process of diatomic gas(s)x-axis (i.e.'Q' axis)
View SolutionThe value of γ of linear, arragement of triatomic gas molecules is
View SolutionQ cal of heat is required to raise the temperature of 1 mole of a monatomic gas from 20∘C to 30∘C at constant pressure. The amount of heat required to raise the temperature of 1 mole of diatomic gas form 20∘C to 25∘C
View SolutionThe heat capacity of a diatomic gas is higher than that of a monatomic gas.
View SolutionCompressibility factor, Z of a gas is given as Z=pVnRT
(i) What is the value of Z for an ideal gas ?
(ii) For real gas what will be the effect on value of Z above boyle's temperature ?
View Solution
