A non-conducting thin disc of radius R rotates about its axis with an angular velocity ω. The surface charge density on the disc varies with the distance r from the centre as σ(r)=σ0[1+(rR)β], where σ0 and β are constants. If the magnetic induction at the center is B=(910)μ0σ0ωR, the value of β isSolution in Telugu
14
4
12
2
The correct Answer is:C
Step by step video, text & image solution for A non-conducting thin disc of radius R rotates about its axis with an angular velocity omega. The surface charge density on the disc varies with the distance r from the centre as sigma(r)=sigma_(0)[1+((r)/(R))^(beta)], where sigma_(0) and beta are constants. If the magnetic induction at the center is B=((9)/(10))mu_(0)sigma_(0)omegaR, the value of beta is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- EAMCET - 2018 (TS) SHIFT - 1BOOK - SIA PUBLICATIONCHAPTER - EAMCET - 2018 (TS) SHIFT - 1EXERCISE - EXERCISE - Chemistry
8 Videos
- EAMCET - 2018 (TS) SHIFT - 1BOOK - SIA PUBLICATIONCHAPTER - EAMCET - 2018 (TS) SHIFT - 1EXERCISE - EXERCISE - Chemistry
8 Videos
- DIFFERENTIATION BOOK - SIA PUBLICATIONCHAPTER - DIFFERENTIATION EXERCISE - Problems
50 Videos
- EAMCET - 2018 (TS) SHIFT - 2BOOK - SIA PUBLICATIONCHAPTER - EAMCET - 2018 (TS) SHIFT - 2EXERCISE - Chemistry
18 Videos
Similar Questions
Match the reactants in List - I with the products in List - II
List - I List - II(a)H2O+H2S(i)(H3O+,HS−)(b)H2O+N3−(ii)(NH3,OH−)(c)H2O+SiCI4(iii)(OH−,H3S+)(d)H2O+F2(iv)(SiO2,HCI)(v)(SiO4−4,HCI)(vi)(O2,F−)(vii)(HF,OH−)(viii)(OH−,HN3)
The correct answer is
View SolutionMatch the reactants in List - I with the products in List - II
List - I List - II(a)H2O+H2S(i)(H3O+,HS−)(b)H2O+N3−(ii)(NH3,OH−)(c)H2O+SiCI4(iii)(OH−,H3S+)(d)H2O+F2(iv)(SiO2,HCI)(v)(SiO4−4,HCI)(vi)(O2,F−)(vii)(HF,OH−)(viii)(OH−,HN3)
The correct answer is
View SolutionIf x=1−√y1+√9, then (x+1)d2ydx2+(3√y+1√y)dydx=
View SolutionStatement (a) : Na2O<MgO<ZnO<P4O16−Acidic property
Statement (b) : F>CI>Br− Electron gain enthalpy
Statement (c ) : M2−>M−>M+>M2+- ionic size
Statement (d) : The second ionisation enthalpy of Cu is more than second ionisation enthalpy of K.
Which of the following is the correct representation of True (T) / Fale(F) for the given statements
View SolutionA non-conducting thin disc of radius R charged uniformly over one side with surface density s rotates about its axis with an angular velocity ω. Find
(a) the magnetic induction at the centre of the disc,
(b) the magnetic moment of the disc.
View SolutionA non-conducting thin disc of radius R charged uniformly over one side with surface density σ, rotates about its axis with an angular velocity ω. Find (a) the magnetic field induction at the centre of the disc (b) the magnetic moment of the disc.
View SolutionA non-conducting thin disc of radius R and mass m having charge uniformly over one side with surface density sigma rotates about its axis with an angular velocity omega . Find : (a) the magnetic induction at the centre of the disc, (b) the magnetic moment of the disc. ( c) the ratio of magnetic moment and angular momentum of disc.
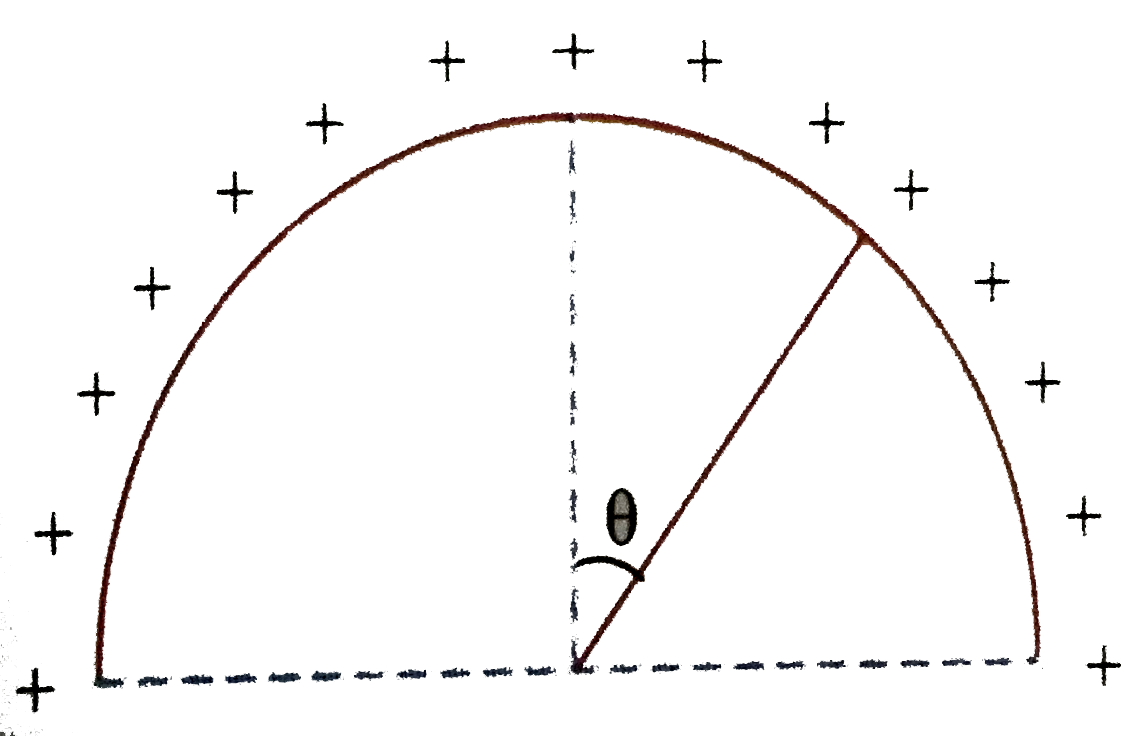
View SolutionFor the hemisphere of radius R shown in the figure the surface charge density σ varies with θ (shown in figure) as σ=σ0cosθ the total charge on the sphere is
View SolutionA flat disc of radius R charged uniformly on its surface at a surface charge density sigma . About its central axis of rotation it rotates at an angular speed omega . Find the magnetic moment of disc due to rotation of charges.
View SolutionA disc of radius R rotates with constant angular velocity ω about its own axis. Surface charge density of this disc varies as σ=αr2, where r is the distance from the centre of disc. Determine the magnetic field intensity at the centre of disc.
View SolutionIf Σnr=1cos−1xr=0,thenΣnr=1xr equals
View SolutionA non-conducting thin disc of radius R rotates about its axis with an angular velocity omega . The surface charge density on the disc varies with the distance r from the centre as sigma(r)=sigma_(0)[1+((r)/(R))^(beta)] , where sigma_(0) and beta are constants. If the magnetic induction at the center is B=((9)/(10))mu_(0)sigma_(0)omegaR , the value of beta is
View SolutionA non0conducting disk of radius R has surface charge density which varies with distance from the centre as sigma (r ) = sigma _(0)[1+ sqrt((r )/(R ))], where sigma _(0) is a constant. The disc rotates about its axis with angular velocity omega. If B is the magnitude of magnetic induction at the centre, then (B)/(mu_(0) sigma _(o)omega R) will be
View SolutionA circular disc of radius carries surface charge density =sigma_(0)(1-(r )/(R )) , where sigma_(0) is a constant and is the distance from the center of the disc Electric flux through a lange spherical surface that endcloses the charged disc completely is phi_(0) . electric flux through another spherical surface of radius (R ) /(4) and consentri with the disc is phi . Then the ratio (phi_(0))/(phi) is __________.
View SolutionThe surface charge density of a thin charged disc of radius R is σ. The value of the electric field at the centre of the disc σ2∈0. With respect to the field at the centre, the electric field along the axis at a distance R from the centre of the disc is
View Solution
