A particle moves on a line according to the law s=at2+bt+c. If the displacement after 1 sec is 16 cm, the velocity after 2 sec is 24 cm/sec and acceleration is 8cm/sec2, then
a=4, b=8, c=4
a=4, b=4, c=8
a=8, b=4, c=4
none of these
The correct Answer is:A
To solve the problem, we have the displacement of a particle given by the equation:
s=at2+bt+c
where s is the displacement, t is the time, and a, b, and c are constants we need to determine.
Step 1: Find the acceleration
The acceleration a is given as 8cm/s2. The acceleration can be derived from the displacement function by differentiating twice:
1. First, we find the velocity v by differentiating s:
v=dsdt=2at+b
2. Then, we find the acceleration a by differentiating the velocity:
a=dvdt=2a
Given that the acceleration is 8cm/s2, we can set up the equation:
2a=8
From this, we can solve for a:
a=4
Step 2: Find the velocity at t=2 seconds
We know the velocity at t=2 seconds is 24cm/s. Using the velocity equation we derived:
v=2at+b
Substituting a=4 and t=2:
v=2(4)(2)+b=16+b
Setting this equal to the given velocity:
16+b=24
Solving for b:
b=24−16=8
Step 3: Find the displacement at t=1 second
We are given that the displacement at t=1 second is 16cm. Using the displacement equation:
s=at2+bt+c
Substituting t=1:
s=4(12)+8(1)+c=4+8+c=12+c
Setting this equal to the given displacement:
12+c=16
Solving for c:
c=16−12=4
Final Values
We have found:
- a=4
- b=8
- c=4
Thus, the values of a, b, and c are 4,8,4 respectively.
Summary
The values of a,b,c are:
- a=4
- b=8
- c=4
The acceleration a is given as 8cm/s2. The acceleration can be derived from the displacement function by differentiating twice:
v=dsdt=2at+b
a=dvdt=2a
2a=8
From this, we can solve for a:
a=4
We know the velocity at t=2 seconds is 24cm/s. Using the velocity equation we derived:
v=2at+b
Substituting a=4 and t=2:
v=2(4)(2)+b=16+b
Setting this equal to the given velocity:
16+b=24
Solving for b:
b=24−16=8
We are given that the displacement at t=1 second is 16cm. Using the displacement equation:
s=at2+bt+c
Substituting t=1:
s=4(12)+8(1)+c=4+8+c=12+c
Setting this equal to the given displacement:
12+c=16
Solving for c:
c=16−12=4
We have found:
- a=4
- b=8
- c=4
The values of a,b,c are:
- a=4
- b=8
- c=4
Topper's Solved these Questions
- DERIVATIVE AS A RATE MEASURER BOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DERIVATIVE AS A RATE MEASURER EXERCISE - Section I - Solved Mcqs
27 Videos
- DEFINITE INTEGRALSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DEFINITE INTEGRALSEXERCISE - Chapter Test 2
56 Videos
- DIFFERENTIAL EQUATIONSBOOK - OBJECTIVE RD SHARMA ENGLISHCHAPTER - DIFFERENTIAL EQUATIONSEXERCISE - Chapter Test
30 Videos
Similar Questions
A particle moves in a straight line such that its position in 't' time is s(t)=t2+3t−1 cm. find its velocity at t=4 sec.
View SolutionA particle moves in a straight line such that its distance in 't' seconds from a fixed point is (6t−t2) cm. find its velocity at the end of t=2 sec.
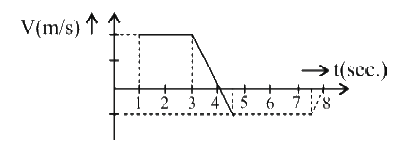
View SolutionThe velocity-time graph of a linear motion is shown in figure. The displacement from the origin after 8 sec is :
View SolutionA wheel starts rotating at 10 rad/sec and attains the angular velocity of 100 rad/sec in 15 seconds. What is the angular acceleration in rad/sec2?
View SolutionThe speed of a particle moving in a circle slows down at a rate of 3m/sec2. At some instant the magnitude of the total acceleration is 5msec2 and the particle's speed is 12 m/sec. The radius of circle will be :
View SolutionA particles starts from rest and has an acceleration of 2m/s2 for 10 sec. After that , it travels for 30 sec with constant speed and then undergoes a retardation of 4m/s2 and comes back to rest. The total distance covered by the particle is
View SolutionA particle is moving in a straight line with initial velocity u and uniform acceleration f. If the sum of the distances travelled in tthand(t+1)th seconds is 100cm, then its velocity after t seconds, in cm/s, is.
View SolutionIf a man has a velocity varying with time given as v=3t2,v is in m/s and t in sec then :

Find out the velocity of the man after 3sec.
View SolutionA particle is executing SHM with amplitude 4 cm and has a maximum velocity of 10 cm/sec
What is its velocity at displacement 4 cm/sec ?
View SolutionA particle is executing SHM with amplitude 4 cm and has a maximum velocity of 10 cm/sec
What is its velocity at displacement 2 cm?
View Solution