A vertical tower PQ subtends th same angle at each of two place A and B, 60 m apart on the ground, AB subtends an angle at the foot of the tower. If h is the height of the tower, then is equal to Solution in Punjabi
3121
2136
3600
None of these
The correct Answer is:A, C
Step by step video & image solution for A vertical tower PQ subtends th same angle 30^(@) at each of two place A and B, 60 m apart on the ground, AB subtends an angle 120^(@) at the foot of the tower. If h is the height of the tower, then 9h^(2)+h+1 is equal to by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - SOLVED EXAMPLES
1 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 1
20 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
52 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHSCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
Similar Questions
Match the statements of Column I with values of Column II.

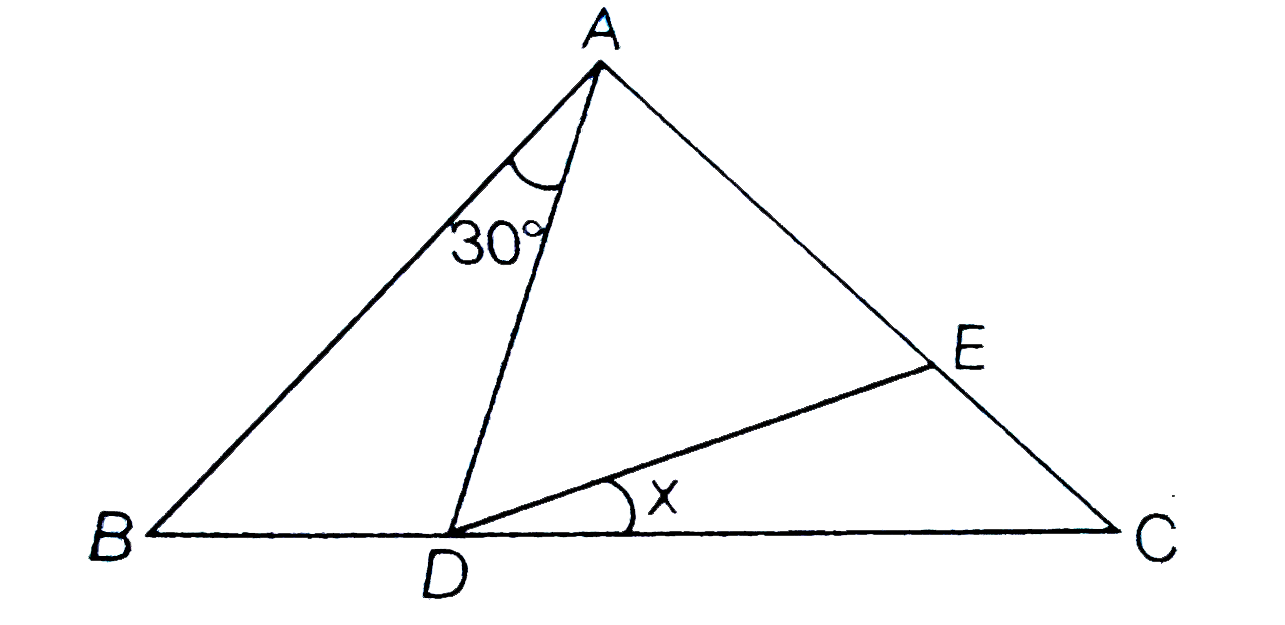
View SolutionIn the given figure,if AB=AC,∠BAD=30∘andAE=AD, then x is equal to
View SolutionLet O be the origin and let PQR be an arbitrary triangle. The point S is such that ¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OQ+¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OR⋅¯¯¯¯¯¯OP+¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OS=¯¯¯¯¯¯OQ⋅¯¯¯¯¯¯OR+¯¯¯¯¯¯OP⋅¯¯¯¯¯¯OS Then the triangle PQR has S as its
View SolutionLet a,b,c be in A.P. and |a|<1,|b|<1|c|<1.ifx=1+a+a2+....to ∞,y=1+b+b2+....to ∞and,z=1+c+c2+...to ∞, then x,y,z are in
View SolutionA vertical tower PQ subtends the same anlgle of 30∘ at each of two points A and B ,60 m apart on the ground .If AB subtends an angle of 120∘ at p the foot of the tower ,then find the height of the tower .
View SolutionA tower of height b subtends an angle at a point 0 on the ground level through the foot of the tower and at a distance a from the foot of the tower. A pole mounted on the top of the tower also subtends an equal angle at 0. The height of the pole is
View SolutionA tower stands at the centre of a circular park . A and B are two points on the boundary of the park such that AB(=a) subtends an angle of 60^@ at the foot of the tower , and the angle of elevation of the top of the tower from A or B is 30^@ . The height of the tower is
View SolutionA vertical tower subtends an angle of 60∘ at a point on the same level as the foot of the tower. On moving 100 m further in line with the tower, it subtends an angle of 30∘ at the point. Then, the height of the tower, it subtends an angle of 30∘ at the point. Then, the height of the tower is
View SolutionAt a distance d from the foot of tower AB of height h metres, a flagstaff BC (surmounted on the tower) and the tower subtend equal angles. Then the height of the flagstaff is :
View SolutionA tower stands at the centre of a circular park .A and B are two points on the boundary of the park such that AB = (=a) subtends an angle of 60^@ at the foot of the tower ,and the angle of elevation of the top of the tower from A or B is 30^@ the height of hte tower is
View SolutionA vertical tower PQ subtends the same anlgle of 30∘ at each of two points A and B ,60 m apart on the ground .If AB subtends an angle of 120∘ at p the foot of the tower ,then find the height of the tower .
View SolutionA tower of height b subtends an angle at a point 0 on the ground level through the foot of the tower and at a distance a from the foot of the tower. A pole mounted on the top of the tower also subtends an equal angle at 0. The height of the pole is
View SolutionA Tower stands at the centre of a circular park. A and B are two points on the boundary of the park such that AB (a) subtends an angle of 60^(@) at the foot of the tower, and the angle of elevation of the top of the tower from A or B is 30°. The height of the tower is
View SolutionA tower subtends an angle α at a point A on the same level as the foot of the tower B is a point vertically above A and AB = h metres. The angle of depression of the foot of the tower from B is β. The height of the tower is
View SolutionA tower stands at the centre of a circular park . A and B are two points on the boundary of the park such that AB(=a) subtends an angle of 60^@ at the foot of the tower , and the angle of elevation of the top of the tower from A or B is 30^@ . The height of the tower is
View SolutionA vertical tower PQ subtends the same anlgle of 30∘ at each of two points A and B ,60 m apart on the ground .If AB subtends an angle of 120∘ at p the foot of the tower ,then find the height of the tower .
View Solution
