AB and CD are two chords of a circle intersecting at a point P outside the circle. If
PC=30cm, CD=14cm and PA=24cm, determine AB.
The correct Answer is:4cm
To solve the problem, we will use the property of intersecting chords in a circle. The property states that if two chords AB and CD intersect at a point P outside the circle, then the following relationship holds:
PA×PB=PC×PD
Where:
- PA = distance from point P to point A
- PB = distance from point P to point B
- PC = distance from point P to point C
- PD = distance from point P to point D
Given:
- PC=30cm
- CD=14cm
- PA=24cm
We need to find the length of chord AB.
1. Identify the lengths:
- We know PA=24cm and PC=30cm.
- The length of chord CD is given as CD=14cm.
2. Calculate PD:
- Since CD=14cm, we can find PD using the relationship:
PD=CD−PC=30−14=16cm
3. Set up the equation:
- Let PB=x. Then, we can express PB in terms of AB:
PB=AP−AB=PA−AB=24−AB
4. Apply the intersecting chords property:
- According to the property:
PA×PB=PC×PD
Substituting the known values:
24×(24−AB)=30×16
5. Calculate the right-hand side:
- Calculate 30×16:
30×16=480
6. Expand and simplify the equation:
24×(24−AB)=480
Expanding the left side:
576−24AB=480
7. Rearranging the equation:
576−480=24AB
96=24AB
8. Solve for AB:
AB=9624=4cm
Thus, the length of chord AB is 4cm.
- PA = distance from point P to point A
- PB = distance from point P to point B
- PC = distance from point P to point C
- PD = distance from point P to point D
- PC=30cm
- CD=14cm
- PA=24cm
- We know PA=24cm and PC=30cm.
- The length of chord CD is given as CD=14cm.
- Since CD=14cm, we can find PD using the relationship:
PD=CD−PC=30−14=16cm
- Let PB=x. Then, we can express PB in terms of AB:
PB=AP−AB=PA−AB=24−AB
- According to the property:
PA×PB=PC×PD
Substituting the known values:
24×(24−AB)=30×16
- Calculate 30×16:
30×16=480
24×(24−AB)=480
Expanding the left side:
576−24AB=480
576−480=24AB
96=24AB
AB=9624=4cm
Topper's Solved these Questions
- BOOK - ICSECHAPTER - CHAPTERWISE REVISION EXERCISEEXERCISE - CHAPTERWISE REVISION EXERCISE (CONSTRUCTION)3videos
CHAPTERWISE REVISION EXERCISE
- BOOK - ICSECHAPTER - CHAPTERWISE REVISION EXERCISEEXERCISE - CHAPTERWISE REVISION EXERCISE (MENSURATION)8videos
CHAPTERWISE REVISION EXERCISE
- BOOK - ICSECHAPTER - CHAPTERWISE REVISION EXERCISEEXERCISE - CHAPTERWISE REVISION EXERCISE (CIRCLES)9videos
CHAPTERWISE REVISION EXERCISE
- BOOK - ICSECHAPTER - BANKING (RECURRING DEPOSIT ACCOUNTS)EXERCISE - QUESTIONS7videos
BANKING (RECURRING DEPOSIT ACCOUNTS)
- BOOK - ICSECHAPTER - CIRCLESEXERCISE - EXERCISE 17( C )28videos
CIRCLES
Similar Questions
AB and CD are two chords of a circle intersecting at a point P outside the circle. If
PA= 8cm, PC= 5cm and PD= 4cm, determine AB.View SolutionAB and CD are two chords of a circle intersecting at a point P inside the circle. If
AB= 24cm, AP= 4cm and PD = 8cm, determine CPView SolutionAB and CD are two chords of a circle intersecting at a point P inside the circle. If
AP=3cm, PB= 2.5cm and CD= 6.5 cm, determine CP.View SolutionAB and CD are two chords of a circle intersecting at P. Prove that AP×PB=CP×PD
 View Solution
View SolutionAB and CD are two parallel chords of a circle which are on opposite sides of the centre such that AB=10cm, CD=24cm and the distance between AB and CD is 17cm. Find the radius of the circle.
View SolutionIn a circle, chords AB and CD intersect at a point R inside the circle. If AR : RB = 1:4 and CR: RD = 4:9, then the ratio AB: CD is
View SolutionIf chords AB and CD of a circle intersect each other at a point inside the circle, prove that : PA×PB=PC×PD .
View SolutionIn Figure two chords AB and CD of a circle intersect each other at the point P (when produced) outside the circle. Prove that
(i) ΔPAC~ΔPDB
(ii) PA⋅PB = PC⋅PD
 View Solution
View SolutionAB and CD are two parallel chords of a circle on opposite sides of a diameter such that AB = 24 cm and CD = 10 cm. If the radius of the circle is 13 cm, find the distance between the two chords.
View SolutionIn the figure given below, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD =7.8 cm, PD = 5 cm, PB = 4 cm. Find
(i) AB.
(ii) The length of tangent PT.
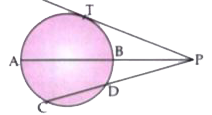 View Solution
View Solution
