If bisectors of opposite angles of a cyclic quadrilateral ABCD intersect the circle, circumscribing it at the points P and Q, prove that PQ is a diameter of the circle.
Given, ABCD is a cyclic quadrilateral.
DP and QB are the bisectors of ∠Dand∠B, respectively.
To prove PQ is the diameter of a circle.
Construction Join QD and QC.
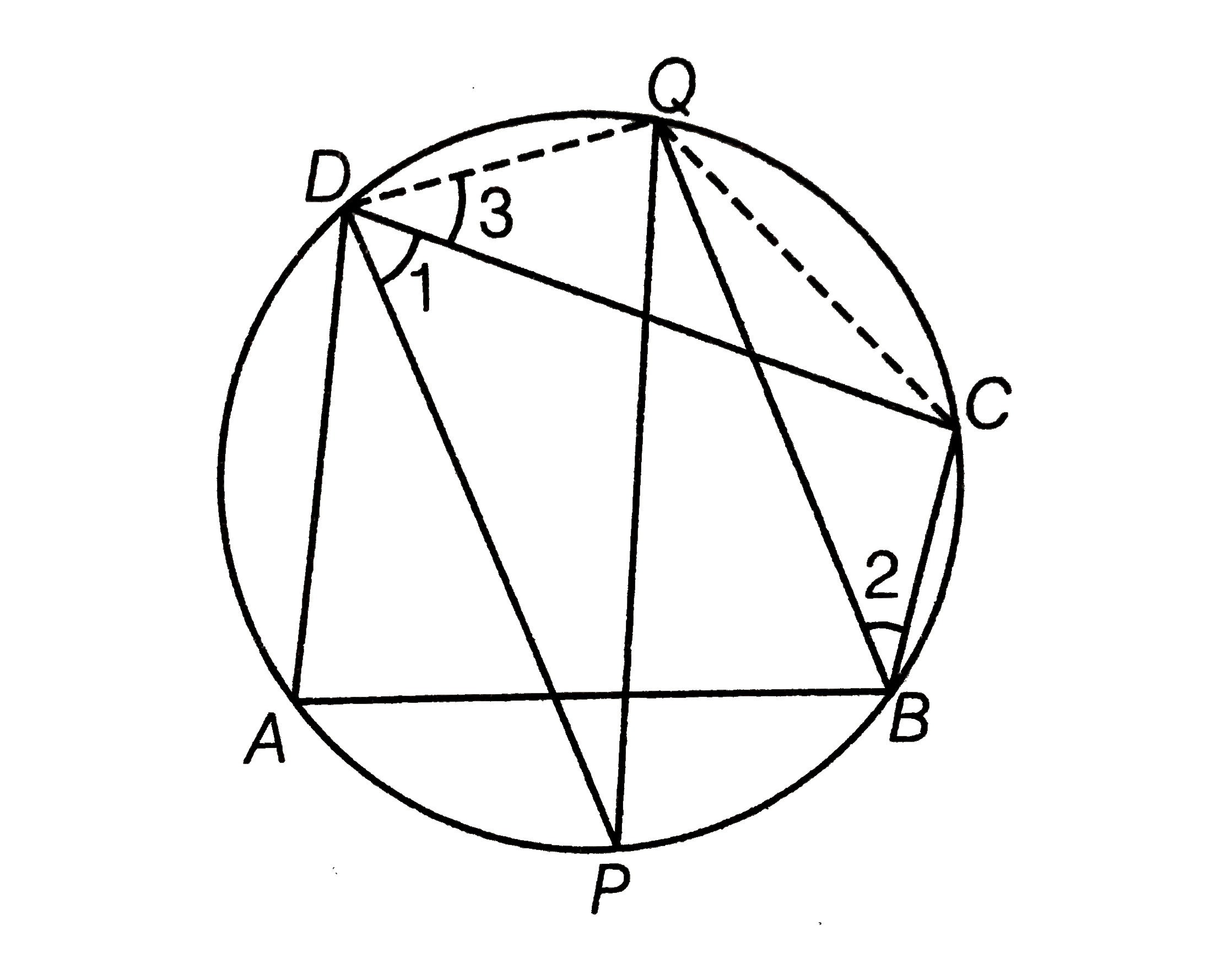
Proof Since, ABCD is a cyclic quadrilateral.
∴∠CDA+∠CBA=180∘
[sum of opposite angles of cyclic quadrilateral is 180∘]
On dividing both sides by 2, we get
12∠CDA+12∠CBA=12×180∘=90∘
⇒∠1+∠2=90∘...(i)
[∠1=12∠CDAand∠2=12∠CBA]
But ∠2=∠3 [ angles in the same segment QC are equal] ...(ii)
∠1+∠3=90∘
From Eqs. (i) and (ii), ∠PDQ=90∘
Hence, PQ is a diameter of a circle, because diameter of the circle.
Subtends a right angle at the circumference.
Topper's Solved these Questions
- CIRCLESBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CIRCLESEXERCISE - Exercise 10.3
20 Videos
- Areas of Parallelograms and TrianglesBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - Areas of Parallelograms and TrianglesEXERCISE - Exercise-9.4 Long Answer Type Questions
10 Videos
- CONSTRUCTIONSBOOK - NCERT EXEMPLAR ENGLISHCHAPTER - CONSTRUCTIONSEXERCISE - Long Answer Type Questions
5 Videos
Similar Questions
If the bisectors of the opposite angles ∠Aand∠B of a cyclic quadrilateral ABCD intersect the corresponding cicle at PandQ respectively, then PQ is a diameter of the circle.
View SolutionProve that the sum of opposite pair of angles of a cyclic quadrilateral is 180∘
View SolutionIf diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral, prove that it is a rectangle
View SolutionIf diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral, prove that it is a rectangle.
View SolutionProve that the rectangle circumscribing a circle is a square
View SolutionIn the given figure, a diameter PQ of a circle bisects the chord RS at the point O. If PS is parallel to RQ, prove that RS is also a diameter of the circle.
View SolutionABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and ∠ADC=140∘, than ∠BAC is equal to
View SolutionThe bisectors of ∠Band∠C of a quadrilateral ABCD intersect each other at point P. Show that P is equidistant from the opposite sides AB and CD.
View SolutionThe quadrilateral formed by angle bisectors of a cyclic quadrilateral is also cyclic.
View SolutionIf the sides of a quadrilateral ABCD touch a circle prove that
AB+CD=BC+AD.
View Solution
