In a ΔABC, ∠B is an acute-angle and AD⊥BC. Prove that:
AB2+CD2=AC2+BD2
To prove that in triangle ABC where ∠B is an acute angle and AD is perpendicular to BC, the following equation holds:
AB2+CD2=AC2+BD2
we will use the Pythagorean theorem in the right triangles ADB and ADC.
Step 1: Identify the Right Triangles
Since AD is perpendicular to BC, we have two right triangles: △ADB and △ADC.
Step 2: Apply the Pythagorean Theorem in △ADC
Using the Pythagorean theorem in triangle ADC:
AC2=AD2+CD2(1)
Step 3: Apply the Pythagorean Theorem in △ADB
Now, applying the Pythagorean theorem in triangle ADB:
AB2=AD2+BD2(2)
Step 4: Rearranging the Equations
From equation (1), we can express AD2:
AD2=AC2−CD2(3)
From equation (2), we can also express AD2:
AD2=AB2−BD2(4)
Step 5: Set Equations (3) and (4) Equal to Each Other
Since both equations (3) and (4) equal AD2, we can set them equal to each other:
AC2−CD2=AB2−BD2
Step 6: Rearranging the Equation
Rearranging the above equation gives us:
AC2−AB2=CD2−BD2
Step 7: Final Rearrangement
Now, adding BD2 to both sides:
AC2+BD2=AB2+CD2
Conclusion
Thus, we have proved that:
AB2+CD2=AC2+BD2
Since AD is perpendicular to BC, we have two right triangles: △ADB and △ADC.
Using the Pythagorean theorem in triangle ADC:
Now, applying the Pythagorean theorem in triangle ADB:
From equation (1), we can express AD2:
Since both equations (3) and (4) equal AD2, we can set them equal to each other:
Rearranging the above equation gives us:
Now, adding BD2 to both sides:
Thus, we have proved that:
Topper's Solved these Questions
- SAMPLE PAPER 4BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 4EXERCISE - PART-B(SECTION-IV)
10 Videos
- SAMPLE PAPER 3 [CBSE 2019 (TOPPER SOLVED)]BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 3 [CBSE 2019 (TOPPER SOLVED)]EXERCISE - SECTION - D
12 Videos
- SAMPLE PAPER 5BOOK - EDUCART PUBLICATIONCHAPTER - SAMPLE PAPER 5EXERCISE - PART - B (SECTION - V)
4 Videos
Similar Questions
In a ΔABC,∠B is an acute-angle and AD⊥BC
Prove that :
(i) AC2=AB2+BC2−2BC×BD
(ii) AB2+CD2=AC2+BD2
View SolutionIn fig. ∠B of △ABC is an acute angle and AD⊥BC ; prove that AC2=AB2+BC2−2BC×BD
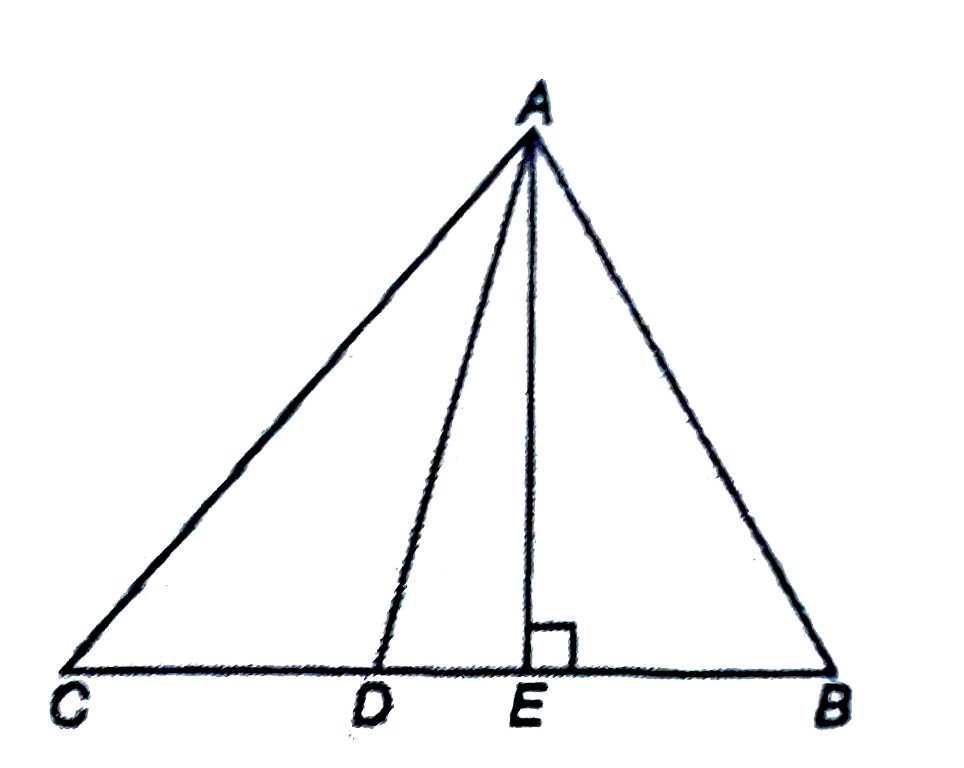
View SolutionThe following figure shows a triangle ABC in which AD is a median and AE⊥BC. Prove that 2AB2+2AC2=4AD2+BC2 .
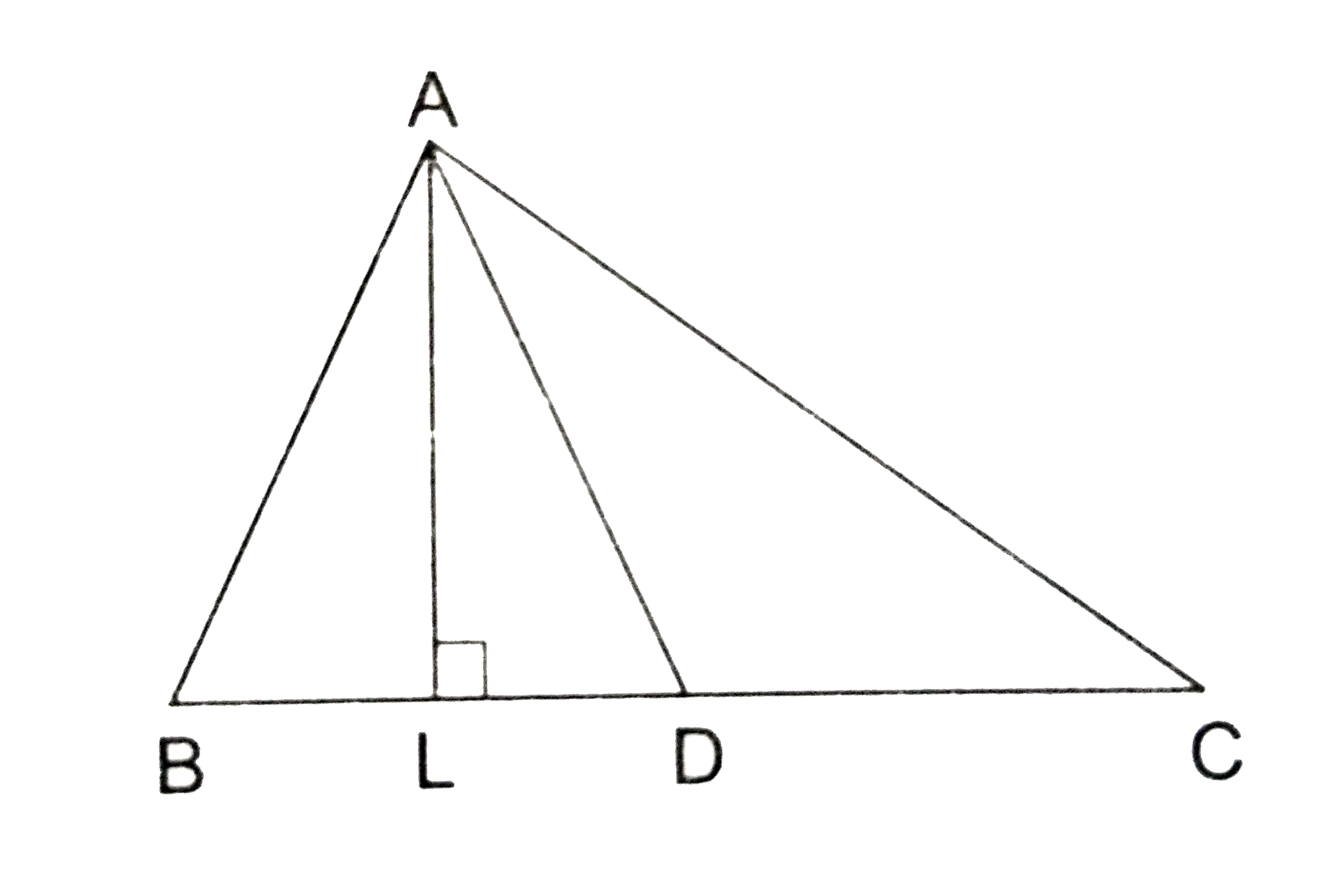
View SolutionIn aΔABC,AD is a median and AL⊥BC.
Prove that
(a) AC2=AD2+BC⋅DL+(BC2)2
(b) AB2=AD2−BC⋅DL+(BC2)2
(c) AC2+AB2=2AD2=2AD2+12BC2
View SolutionIn a right-angled triangle, the square of hypotenuse is equal to the sum of the squares of the two sides. Given that ∠B of △ABC is an acute angle and AD⊥BC . Prove that AC2=AB2+BC2−2BC.BD.
View Solution(Result on acute triangle) In Fig. 4.184, ∠B of ABC is an acute angle and AD⊥BC , prove that AC2=AB2+BC2−2 BC×BD
View SolutionIn the given figure if AD ⊥ BC prove that AB2+CD2=BD2+AC2
View SolutionIn ΔABC,∠B=90∘ and d is the mid - point of BC. Prove that AC2=AD2+3CD2
View SolutionΔABD is a right triangle, right-angled at A and AC⊥BD. Prove that AB2=BC×BD.
View SolutionIn Figure 2,AD⊥BC. Prove that AB2+CD2=BD2+AC2.
View Solution
