In a square ABCD, diagonals meet at O. P is a point on BC, such that OB= BP. Show that:
∠BOP=3∠COP
To solve the problem, we need to prove that in square ABCD, with diagonals meeting at point O and point P on side BC such that OB = BP, the angle BOP is three times the angle COP.
1. Identify the Properties of the Square:
- In square ABCD, the diagonals AC and BD intersect at point O.
- The diagonals of a square bisect each other at right angles, so ∠AOB = ∠COD = 90°.
2. Establish the Given Condition:
- We are given that OB = BP. Let OB = BP = x.
3. Analyze Triangle BOP:
- Since OB = BP, triangle BOP is isosceles with OB = BP.
- Let ∠BOP = θ. By the properties of isosceles triangles, ∠OBP = θ as well.
4. Calculate the Angles in Triangle BOP:
- The sum of angles in triangle BOP is 180°:
∠BOP+∠OBP+∠PBO=180°
- Substituting the known angles:
θ+θ+∠PBO=180°
2θ+∠PBO=180°
- Therefore, we can express ∠PBO as:
∠PBO=180°−2θ
5. Find the Angle COP:
- Since diagonals bisect at right angles, ∠AOB = 90°.
- Therefore, ∠COP = 90° - ∠PBO:
∠COP=90°−(180°−2θ)
∠COP=90°−180°+2θ
∠COP=2θ−90°
6. Relate the Angles:
- We need to show that ∠BOP = 3 * ∠COP:
θ=3(2θ−90°)
- Expanding the right side:
θ=6θ−270°
- Rearranging gives:
270°=5θ
- Thus:
θ=54°
7. Final Calculation:
- Now substituting back:
∠COP=2θ−90°=2(54°)−90°=108°−90°=18°
- Therefore:
∠BOP=θ=54°=3∗18°=3∗∠COP
Conclusion:
We have shown that ∠BOP = 3 * ∠COP, thus proving the statement.
- In square ABCD, the diagonals AC and BD intersect at point O.
- The diagonals of a square bisect each other at right angles, so ∠AOB = ∠COD = 90°.
- We are given that OB = BP. Let OB = BP = x.
- Since OB = BP, triangle BOP is isosceles with OB = BP.
- Let ∠BOP = θ. By the properties of isosceles triangles, ∠OBP = θ as well.
- The sum of angles in triangle BOP is 180°:
∠BOP+∠OBP+∠PBO=180°
- Substituting the known angles:
θ+θ+∠PBO=180°
2θ+∠PBO=180°
- Therefore, we can express ∠PBO as:
∠PBO=180°−2θ
- Since diagonals bisect at right angles, ∠AOB = 90°.
- Therefore, ∠COP = 90° - ∠PBO:
∠COP=90°−(180°−2θ)
∠COP=90°−180°+2θ
∠COP=2θ−90°
- We need to show that ∠BOP = 3 * ∠COP:
θ=3(2θ−90°)
- Expanding the right side:
θ=6θ−270°
- Rearranging gives:
270°=5θ
- Thus:
θ=54°
- Now substituting back:
∠COP=2θ−90°=2(54°)−90°=108°−90°=18°
- Therefore:
∠BOP=θ=54°=3∗18°=3∗∠COP
We have shown that ∠BOP = 3 * ∠COP, thus proving the statement.
Topper's Solved these Questions
- RECTILINEAR FIGURESBOOK - ICSECHAPTER - RECTILINEAR FIGURESEXERCISE - Exercise 14(C )
28 Videos
- RECTILINEAR FIGURESBOOK - ICSECHAPTER - RECTILINEAR FIGURESEXERCISE - POLYGONS - 3 MARKS QUESTIONS
5 Videos
- RECTILINEAR FIGURESBOOK - ICSECHAPTER - RECTILINEAR FIGURESEXERCISE - Exercise 14(B) (true/false)
10 Videos
- RATIONAL AND IRRATIONAL NUMBERS BOOK - ICSECHAPTER - RATIONAL AND IRRATIONAL NUMBERS EXERCISE - EXERCISE 1 (D)
21 Videos
- SIMULTANEOUS EQUATIONSBOOK - ICSECHAPTER - SIMULTANEOUS EQUATIONSEXERCISE - EXERCISE 6 (G)
13 Videos
Similar Questions
In a square ABCD, diagonals meet at O. P is a point on BC, such that OB= BP. Show that:
∠BDC=2∠POC
View SolutionIn a square ABCD, diagonals meet at O. P is a point on BC, such that OB= BP. Show that:
∠POC=(2212)∘
View SolutionIn a square ABCD, diagonals meet at O. P is point on BC such that OB = BP.Show that (i) ∠POC=(2212)∘
(ii) ∠BDC=2∠POC
(iii) ∠BOP=3∠COP
View SolutionIn quadrilateral ABCD, diagonals AC and BD intersect at point E such that
AE:EC=BE:ED.
Show that : ABCD is a trapezium.
View SolutionIn a parallelogram ABCD, P is a point on side AD such that 3AP = AD and Q is a point on BC such that 3CQ = BC. Prove that : AQCP is a parallelogram.
View SolutionIn the figure, given below, ABCD is a parallelogram. P is a point on BC such that BP : PC = 1: 2. DP produced meets AB produced at Q. Given the area of triangle CPQ=20cm2
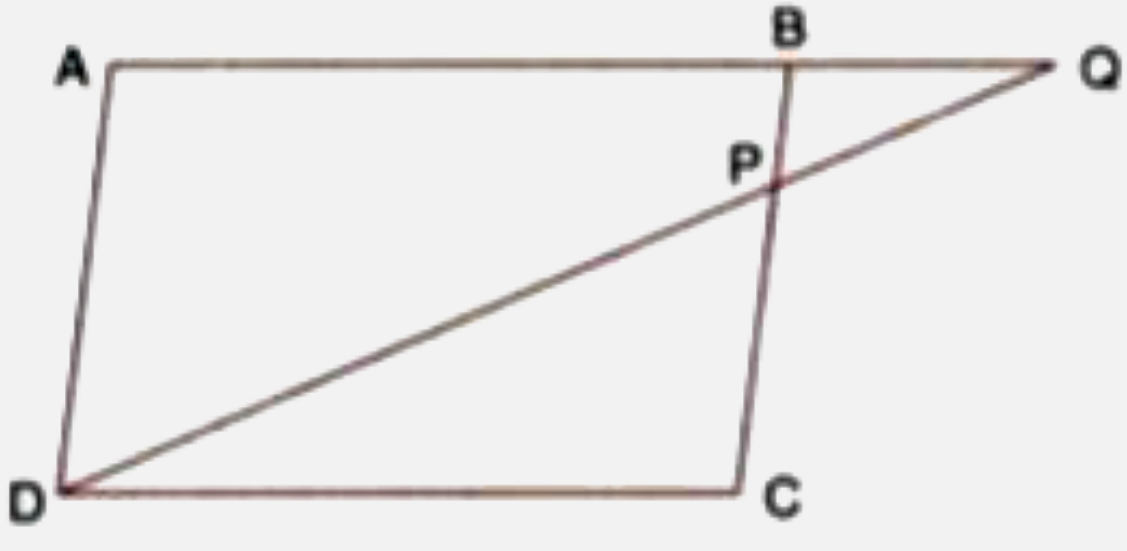
Calculate :
area of parallelogram ABCD.
View SolutionABCD is a square with each side 12 cm. P is a point on BC such that area of ΔABP : area of trapezium APCD = 1:5. Find the length of CP.
View SolutionIn the figure, given below, ABCD is a parallelogram. P is a point on BC such that BP : PC = 1: 2. DP produced meets AB produced at Q. Given the area of triangle CPQ=20cm2

Calculate :
area of triangle CDP.
View SolutionThe diagonals of a quadrilateral ABCD intersect each other at the point O such that AOBO=CODO. Show that ABCD is a trapezium.
View SolutionABCD is a square. P, Q and Rare the points on AB, BC and CD respectively, such that AP = BQ = CR. Prove that:
PB = QC
View Solution
