In cyclic quadrilateral ABCD, Diagonals AC and BD intersect at P. If ∠DBC=70∘ and ∠BAC=30∘ then find ∠BCD
The correct Answer is:80∘
To solve the problem, we need to find the angle ∠BCD in the cyclic quadrilateral ABCD where the diagonals AC and BD intersect at point P. Given that ∠DBC=70∘ and ∠BAC=30∘, we can follow these steps:
Step 1: Identify the angles in triangles
We know that in cyclic quadrilaterals, the angles subtended by the same chord are equal. Here, we have two triangles: △ABC and △DBC.
Step 2: Use the property of angles in triangles
From the cyclic nature of the quadrilateral, we can state that:
∠BAC=∠BDC
Thus, we have:
∠BDC=30∘
Step 3: Sum of angles in triangle BCD
Now, we can apply the triangle angle sum property in triangle BCD:
∠BDC+∠DBC+∠BCD=180∘
Substituting the known values:
30∘+70∘+∠BCD=180∘
Step 4: Solve for ∠BCD
Now, we can simplify the equation:
100∘+∠BCD=180∘
Subtracting 100∘ from both sides gives us:
∠BCD=180∘−100∘=80∘
Final Answer
Thus, the measure of angle ∠BCD is:
∠BCD=80∘
---
We know that in cyclic quadrilaterals, the angles subtended by the same chord are equal. Here, we have two triangles: △ABC and △DBC.
From the cyclic nature of the quadrilateral, we can state that:
∠BAC=∠BDC
Thus, we have:
∠BDC=30∘
Now, we can apply the triangle angle sum property in triangle BCD:
∠BDC+∠DBC+∠BCD=180∘
Substituting the known values:
30∘+70∘+∠BCD=180∘
Now, we can simplify the equation:
100∘+∠BCD=180∘
Subtracting 100∘ from both sides gives us:
∠BCD=180∘−100∘=80∘
Thus, the measure of angle ∠BCD is:
∠BCD=80∘
Topper's Solved these Questions
- CIRCLES BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLES EXERCISE - MULTIPLE CHOICE QUESTIONS (MCQ.s)
14 Videos
- CIRCLES BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLES EXERCISE - SUM TO ENRICH REMAMBER
6 Videos
- CIRCLES BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLES EXERCISE - EXERCISE 10.6
10 Videos
- INTRODUCTION TO EUCLID.S GEOMETRYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - INTRODUCTION TO EUCLID.S GEOMETRYEXERCISE - MULTIPLE CHOICE QUESTIONS
3 Videos
Similar Questions
Knowledge Check
ABCD is a cyclic quadrilateral whose diagonals intersect at P. If AB=BC,∠DBC=70∘and∠BAC=30∘, then the measure of ∠PCD is :
A35∘B50∘C55∘D30∘In the given figure, ABCD is a cyclic quadrilateral and diagonals D bisect each other at P. If ∠DBC=60∘and∠BAC=30∘, then ∠BCD is:
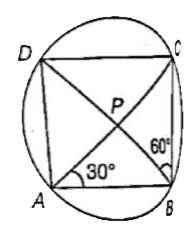 A90∘B60∘C80∘Dnone of the above
A90∘B60∘C80∘Dnone of the aboveABCD is a cyclic quadrilateral. Diagonals AC and BD meets at P. If ∠APB=110∘ and ∠CBD=30∘, then ∠ADB measures
A55∘B30∘C70∘D80∘
In the given figure if ∠DBC=70∘ and ∠BAC=40∘ then find the value of ∠BCD
View SolutionIn the given figure, ABCD is a cyclic quadrilateral in which AE is drawn paralllel to CD and BA is produced to F. If ∠ABC=70∘ and ∠FAE=30∘, Find ∠BCD.
View SolutionABCD is a cyclic quadrilateral whose diagonals intersect at P. If AB=BC, ∠DBC=70∘ and ∠BAC=30∘, then the measure of ∠PCD is :
ABCD एक चक्रीय चतुर्भुज है जिसके विकर्ण एक-दूसरे को P पर काटते हैं | यदि AB=BC, ∠DBC=70∘ तथा ∠BAC=30∘ है, तो कोण PCD का माप क्या है ?
View SolutionABCD is a cyclic quadrilateral whose diagonals intersect each other at point O. If ∠CAB=25∘ and ∠BCD=85∘ then find ∠CBD.
View SolutionABCD is a cyclic quadrilateral whose diagonals intersect at P. If AB=BC,∠DBC=70∘and∠BAC=30∘, then the measure of ∠PCD is:
View Solution

