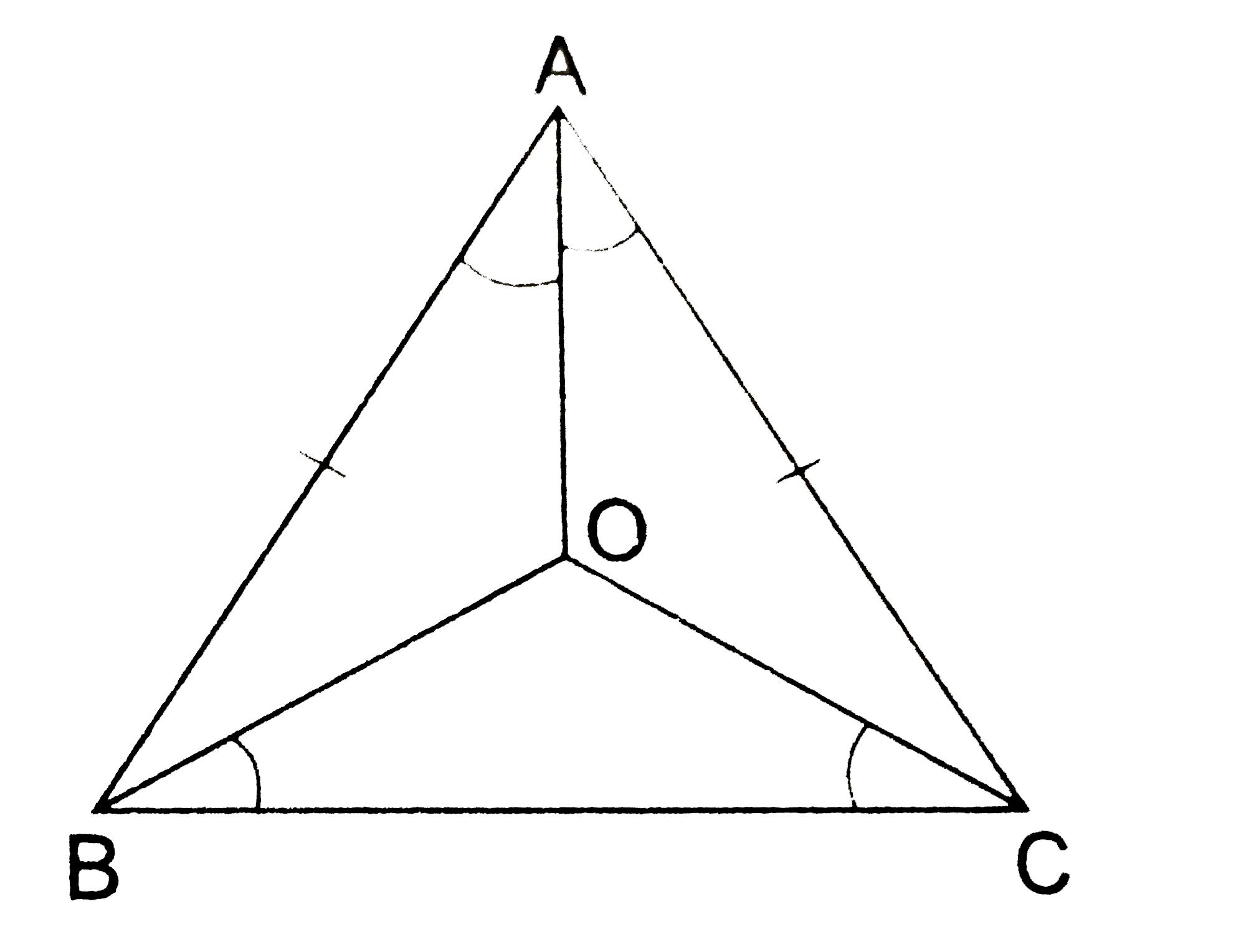
In ΔABC,AB=AC and the bisectors of ∠B and ∠C meet AC and AB at point D and E respectively. Prove that BD=CE.
To prove that BD=CE in triangle ΔABC where AB=AC and the angle bisectors of ∠B and ∠C meet AC and AB at points D and E respectively, we can follow these steps:
1. Identify the Triangle and Given Information:
We have triangle ΔABC with AB=AC. This means that triangle ABC is isosceles with ∠B=∠C.
Hint: Remember that in an isosceles triangle, the angles opposite the equal sides are also equal.
2. Draw the Angle Bisectors:
Draw the angle bisectors of ∠B and ∠C. Let the angle bisector of ∠B intersect AC at point D and the angle bisector of ∠C intersect AB at point E.
Hint: The angle bisector divides the angle into two equal parts.
3. Label the Angles:
Let ∠ABD=∠DBC=12∠B and ∠ACE=∠ECA=12∠C.
Hint: Use the property of angle bisectors to express the angles in terms of ∠B and ∠C.
4. Use the Isosceles Triangle Property:
Since AB=AC, we have ∠B=∠C. Therefore, 12∠B=12∠C.
Hint: This equality allows us to establish relationships between the segments created by the angle bisectors.
5. Consider Triangles ΔOBE and ΔOCD:
Let O be the intersection of BD and CE. In triangles ΔOBE and ΔOCD, we have:
- ∠OBE=12∠B
- ∠OCD=12∠C
Since ∠B=∠C, we can say ∠OBE=∠OCD.
Hint: Use the property of equal angles to establish similarity between the triangles.
6. Establish Similarity:
Since ∠OBE=∠OCD and ∠OEB=∠ODC (both are vertical angles), triangles ΔOBE and ΔOCD are similar by AA (Angle-Angle) similarity criterion.
Hint: Similar triangles have proportional sides.
7. Set Up Proportions:
From the similarity of triangles OBE and OCD, we have:
OEOB=ODOC
Hint: Write down the ratios of corresponding sides from the similar triangles.
8. Express BD and CE:
Since BD=OB+OD and CE=OC+OE, we can express these segments in terms of the segments we have established.
Hint: Use the relationships from the previous steps to express BD and CE.
9. Conclude that BD=CE:
By substituting the expressions from the similarity into BD and CE, we find that BD=CE.
Hint: Combine the results to show that both segments are indeed equal.
Final Statement:
Thus, we have proved that BD=CE.
We have triangle ΔABC with AB=AC. This means that triangle ABC is isosceles with ∠B=∠C.
Draw the angle bisectors of ∠B and ∠C. Let the angle bisector of ∠B intersect AC at point D and the angle bisector of ∠C intersect AB at point E.
Let ∠ABD=∠DBC=12∠B and ∠ACE=∠ECA=12∠C.
Since AB=AC, we have ∠B=∠C. Therefore, 12∠B=12∠C.
Let O be the intersection of BD and CE. In triangles ΔOBE and ΔOCD, we have:
- ∠OBE=12∠B
- ∠OCD=12∠C
Since ∠OBE=∠OCD and ∠OEB=∠ODC (both are vertical angles), triangles ΔOBE and ΔOCD are similar by AA (Angle-Angle) similarity criterion.
From the similarity of triangles OBE and OCD, we have:
OEOB=ODOC
Since BD=OB+OD and CE=OC+OE, we can express these segments in terms of the segments we have established.
By substituting the expressions from the similarity into BD and CE, we find that BD=CE.
Thus, we have proved that BD=CE.
Topper's Solved these Questions
- BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLESEXERCISE - Exercise 7b15videos
TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLESEXERCISE - Revision Exercise (very Short Answer Questions)10videos
TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - TRIANGLESEXERCISE - Problems From NCERT/exemplar5videos
TRIANGLES
- BOOK - NAGEEN PRAKASHANCHAPTER - SURFACE AREA AND VOLUMEEXERCISE - Revision Exercise (long Answer Questions)10videos
SURFACE AREA AND VOLUME
Similar Questions
Knowledge Check
- Question 1 - Select One
In a ΔABC, AD is a median . The bisectors of ∠ADBand∠ADC meet AB and AC at E and F respectively . If the ratio of AE : BE =2:3 , then find the ratio of EF : BC.
A2:5B4:3C7:3D3:7 - Question 2 - Select One
In ΔABC,∠C=70∘ . The bisectors of ∠Aand∠B meet BC and AC in points D and E . Respectively .AD and BE intersect each other at P. What is the measure of ∠DPB?
A55∘B65∘C35∘D45∘
AD is a median of ΔABC . The bisector of ∠ADB and ∠ADC meet AB and AC in E and F respectively. Prove that EF||BC
View SolutionIn ΔABC, the bisector of ∠B meets AC at D. A line PQ∣∣AC meets AB,BC and BD at P,Q and R respectively. Show that BP×QR=BQ×PR.
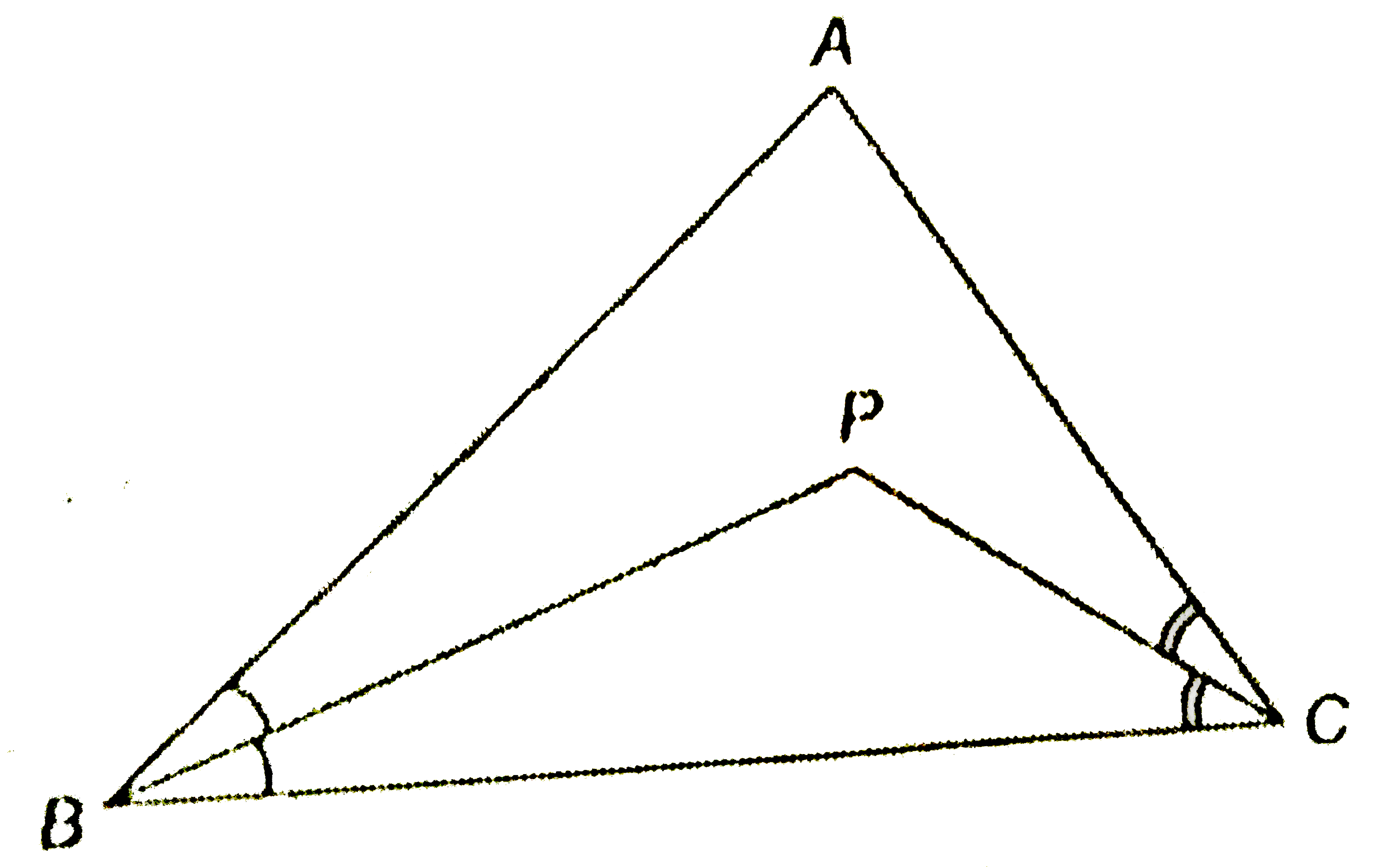
View SolutionIn the adjoining figure, AB>AC and the angle bisectors of ∠B and ∠C meet at point P. Prove that
PB>PC.
 View Solution
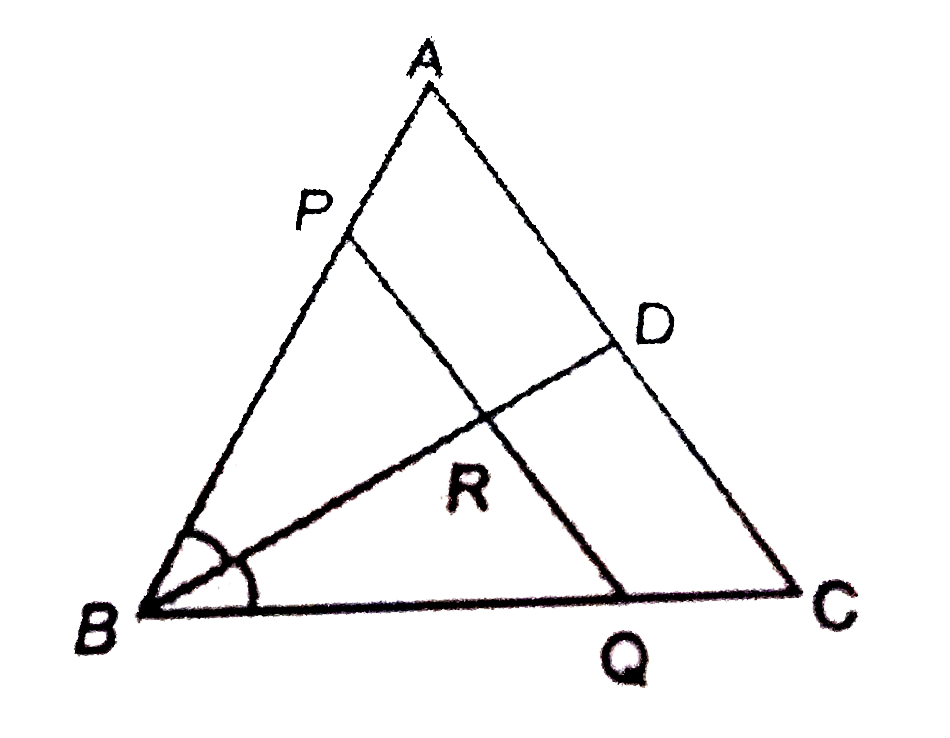
View SolutionIn △ABCthe bisector of ∠B meets AC at D. A line PQ||AC meets AB,BC and BD at P, Q and R respectively.
show that PR×BQ=QR×BP.
 View Solution
View SolutionIn ΔABC,∠C is obtuse. The exteral bisector of ∠Aand∠B meet the extended line BC and AC at point D and E. If AB = AD = BE then find ∠ACD?
View SolutionThe incircle of an isosceles triangle ABC, in which AB = AC, touches the sides BC, CA and AB at D, E and F respectively . Prove that BD = DC.
 View Solution
View Solution