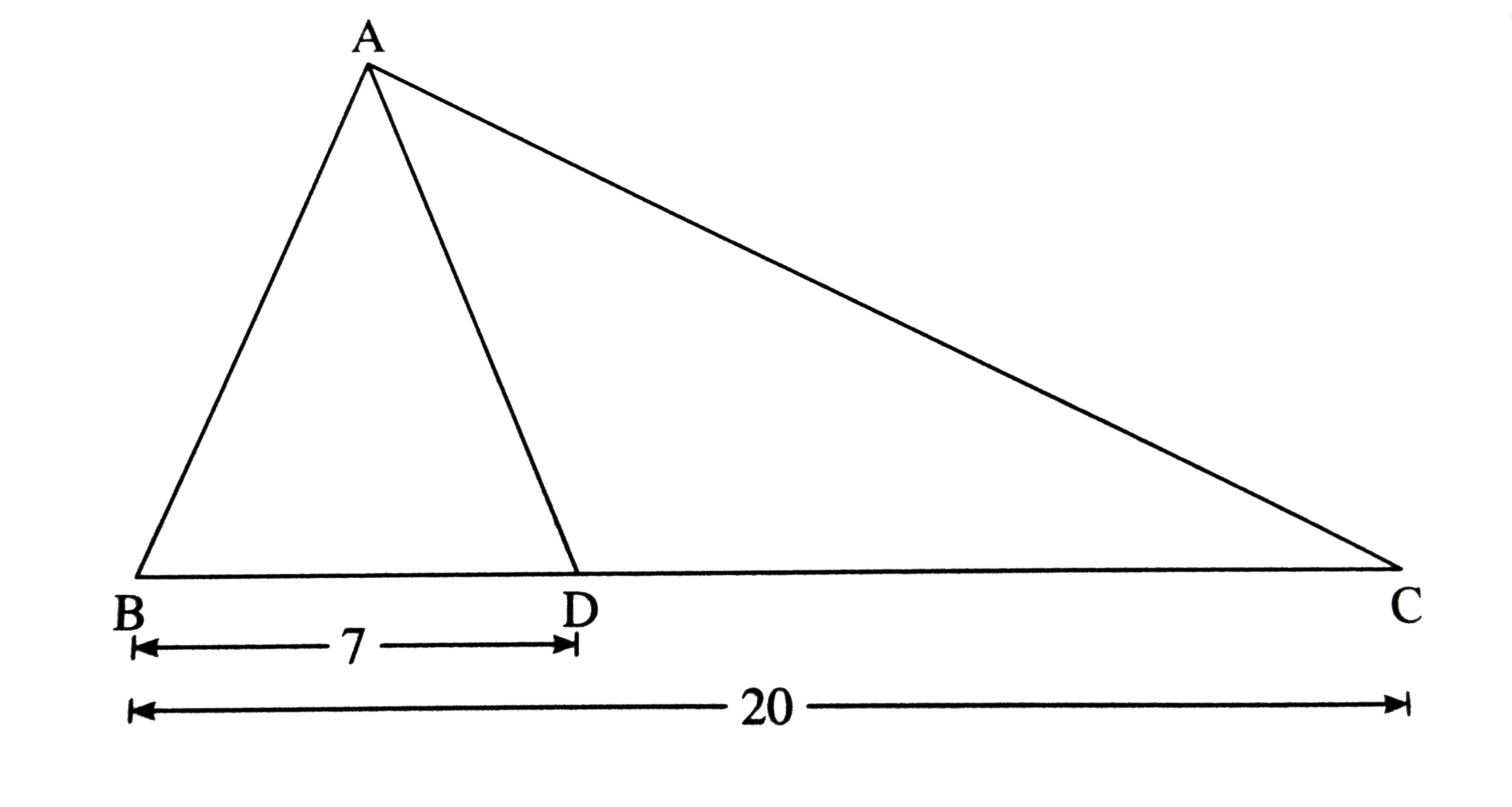
In ΔABC,B−D−C and BD=7,BC=20, then find followig ratios:
A(ΔABD)A(ΔADC)
A(ΔABD)A(ΔABC)
A(ΔADC)A(ΔABC)
BD=7,BC=20
BD+DC=BC..(B−D−C)
∴7+DC=20
∴DC=20−7
∴DC=13
ΔABD,ΔADC and ΔABC have a comon vertex A and their bases BD,DC and BC lie on the same line BC.
∴ they have equal heights.
Area of triangles with equal heights are proportional to their corresponding bases.
A(ΔABD)A(ΔADC)=BDDC
∴A(ΔABD)A(ΔADC)=713
A(De<ABD)A(ΔABC)=BDBC
∴A(ΔABD)A(ΔABC)=720
A(ΔADC)A(ΔABC)=DCBC
∴A(ΔADC)A(ΔABC)=1320
A(ΔABD)A(ΔADC)=713,A(ΔABD)A(ΔABC)=720,A(ΔADC)A(ΔABC)=1320
Topper's Solved these Questions
- SIMILARITYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - CHALLENGING QUESTIONS
8 Videos
- SIMILARITYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - MCQ TYPE
15 Videos
- SIMILARITYBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - SIMILARITYEXERCISE - 2.5 (4 mark each)
3 Videos
- QUADRATIC EQUATIONS BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - QUADRATIC EQUATIONS EXERCISE - ASSIGNEMENT 2.4
8 Videos
- STATISTICSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - STATISTICSEXERCISE - EXAMPLES FOR PRACTICE (MCQs)
35 Videos
Similar Questions
Knowledge Check
In the ΔABC,AB=a,AC=candBC=b, then
Aa+b+c=0Ba+b-c=0Ca-b+c=0D−a+b+c=0
In ΔABC,B - D - C and BD = 7, BC = 20, then find the ratio
A(ΔABD)A(ΔABC)
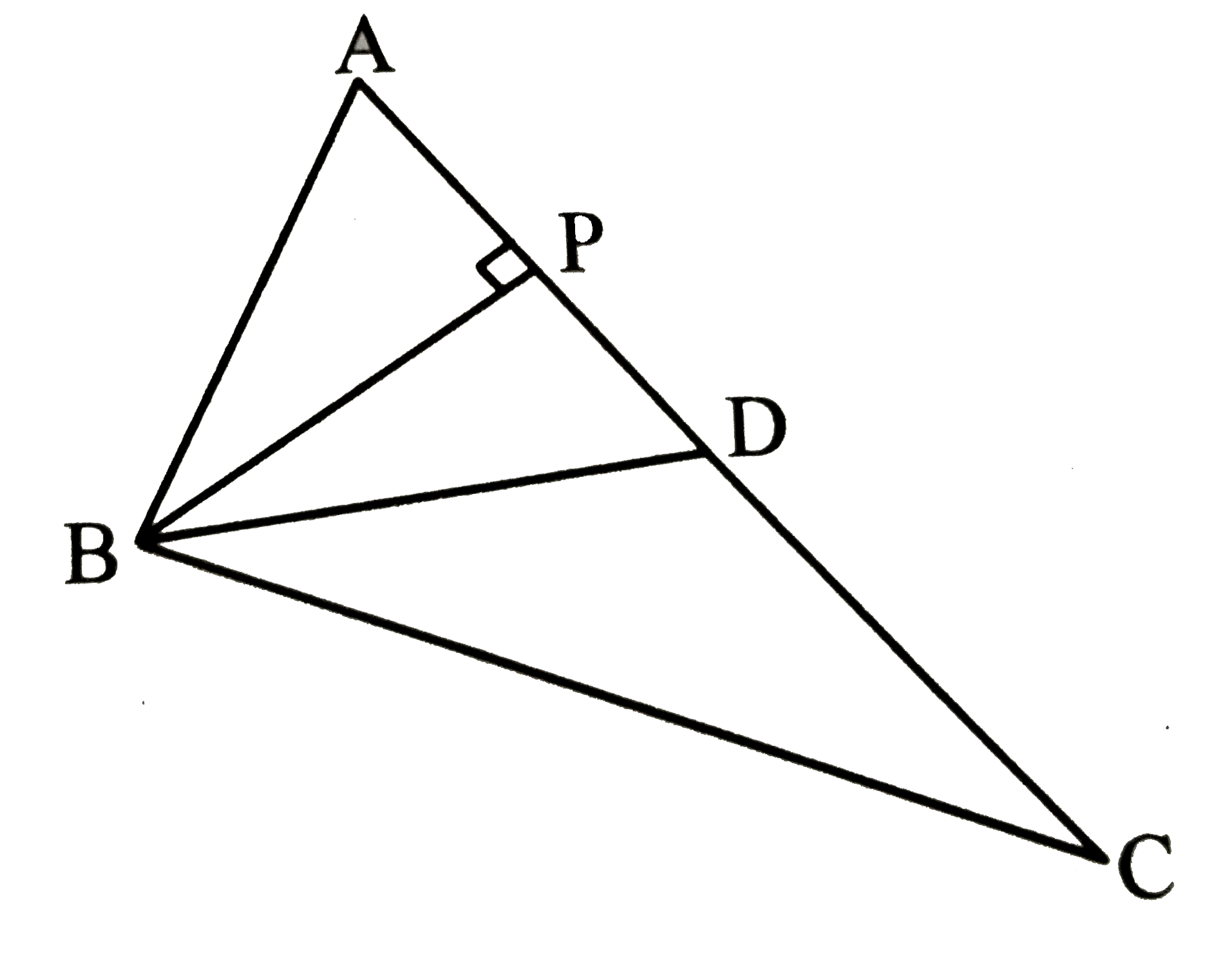
View SolutionIn the given figure, in ΔABC, point D is on side AC. If AC = 16, DC = 9 and BP⊥ AC then, find the following rations.
i. A(ΔABD)A(ΔABC)
ii.A(ΔBDC)A(ΔABC)
iii. A(ΔABD)A(ΔBDC)
View SolutionIn the adjoining figure PQ⊥BC,AD⊥BC, then find following ratios:
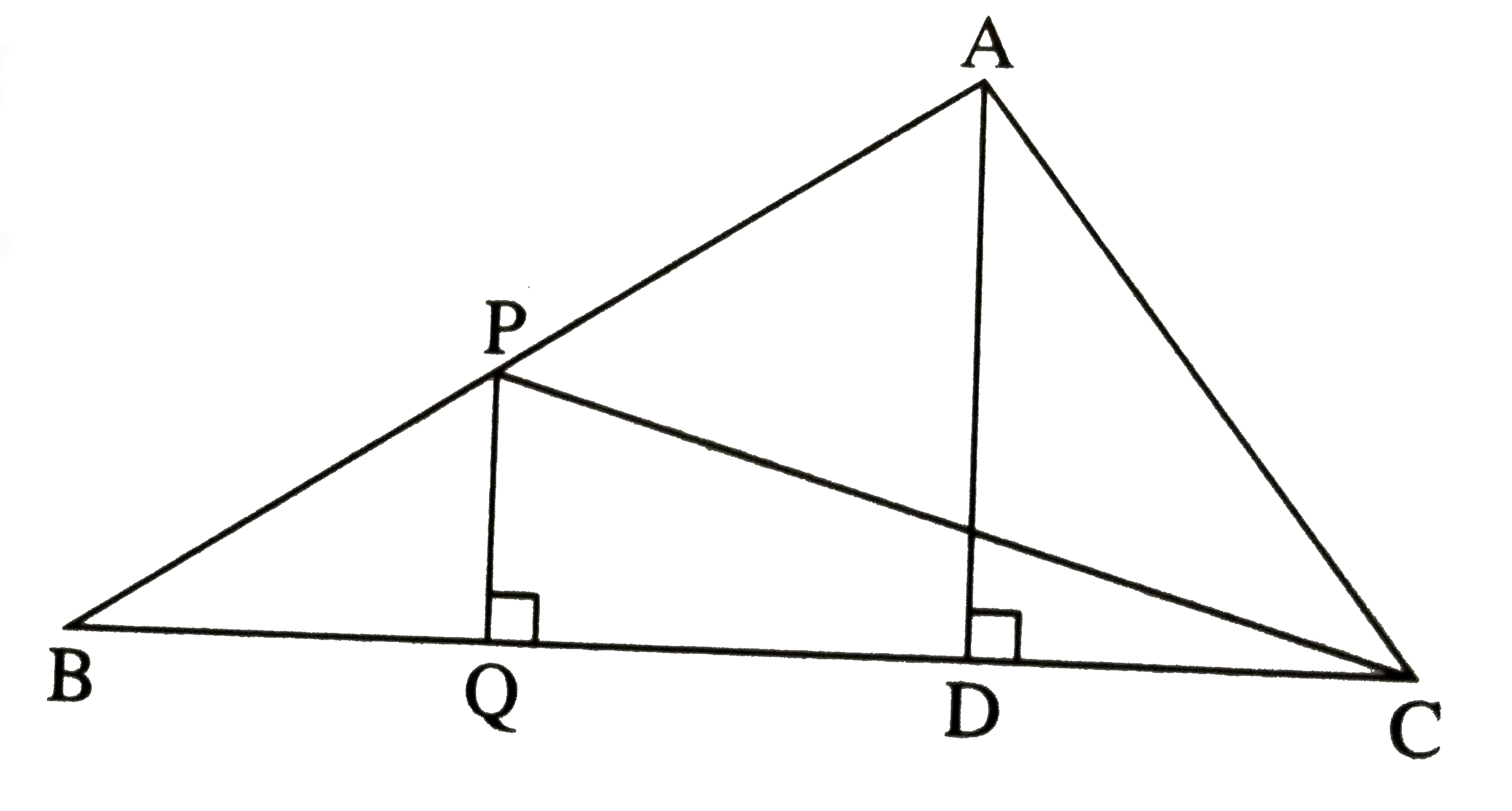
(i( A(ΔPQB)A(ΔPBC) (ii) A(ΔPBC)A(ΔABC)
(iii) A(ΔABC)A(ΔADC)(iv) A(ΔADC)A(ΔPQC)
View SolutionIn (ΔABC) , MN∣∣BC and AM:AB=13. Then find the ratio of ar(ΔAMN)ar(ΔABC)
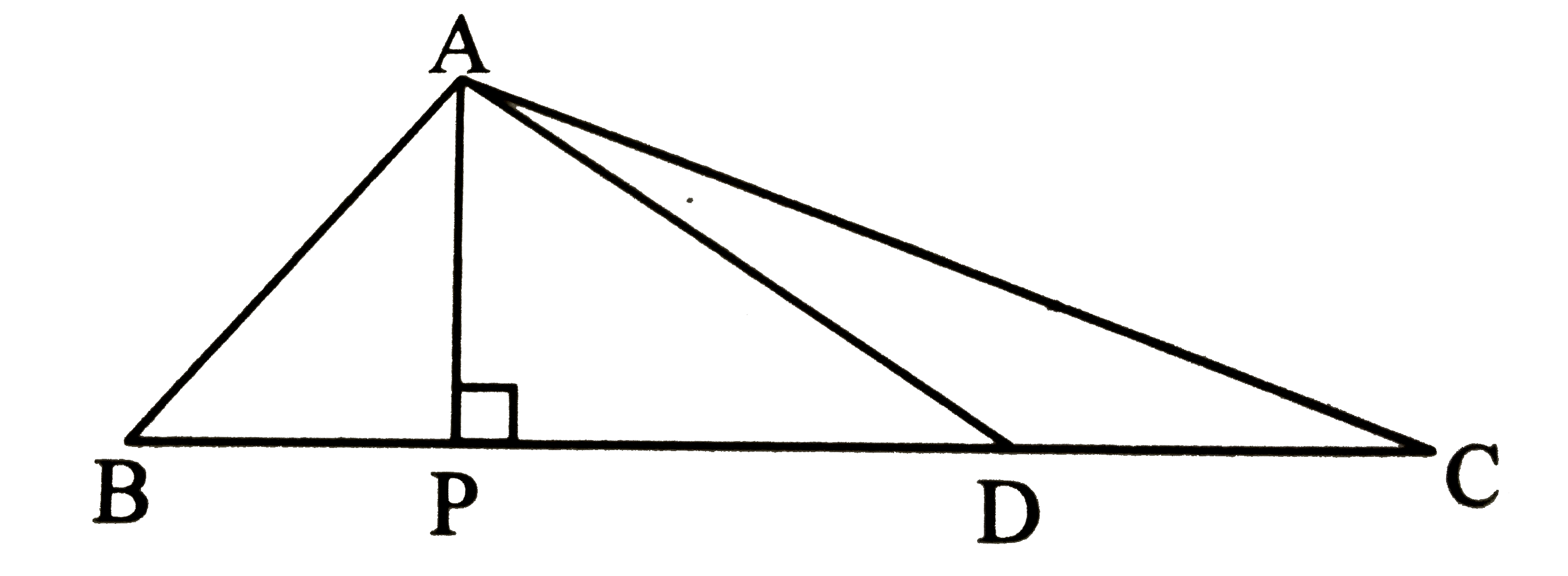
View SolutionIn ΔABC,point D is on side BC such that DC = 6, BC = 15. find
(i)A(ΔABD):A(ΔABC) and
(ii) A(ΔABD):A(ΔADC).
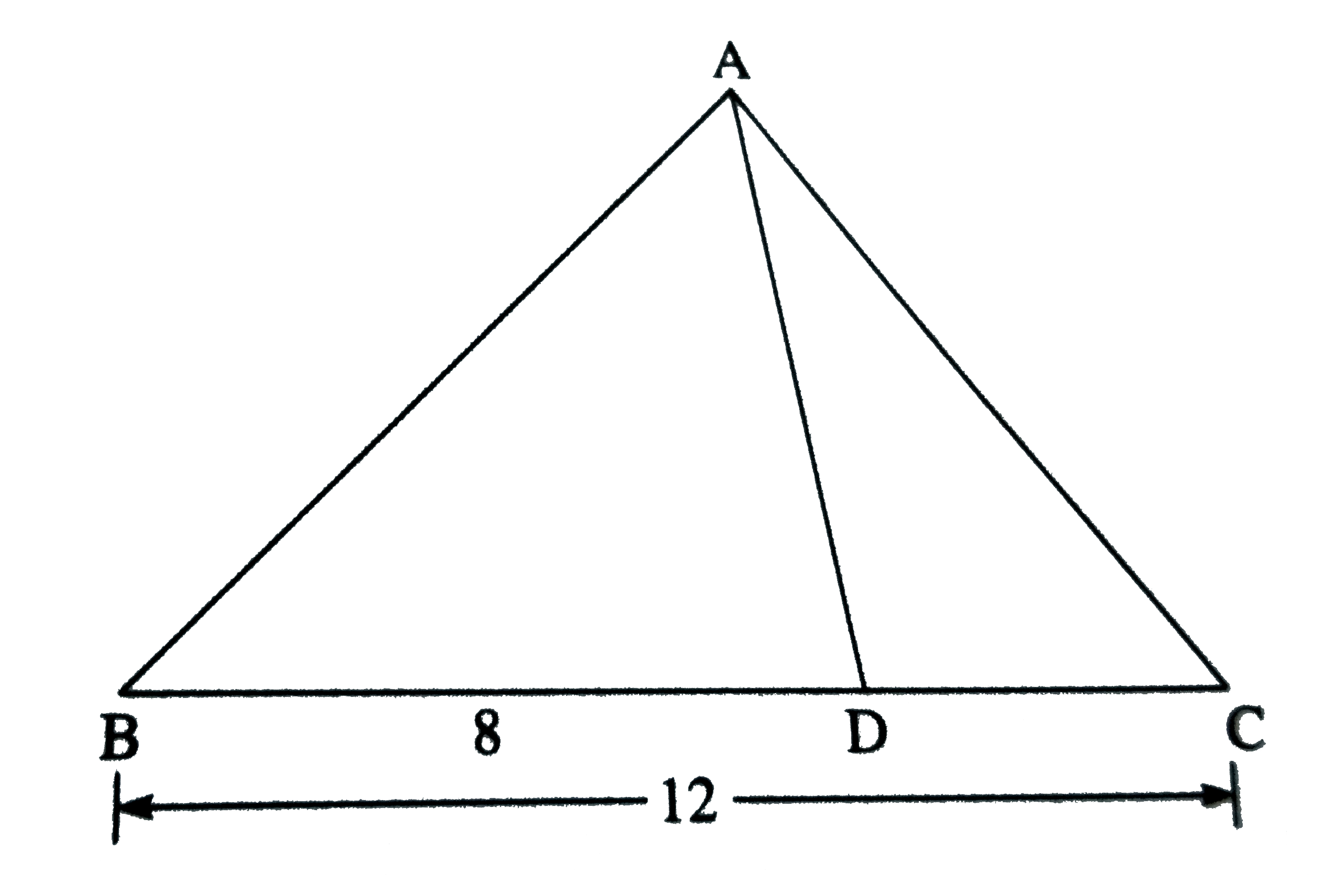
View SolutionIn the figure BD=8,BC=12 and B−D−C then A(ΔABD)A(ΔADC)=………….
View SolutionIn the adjoining figure, PQ⊥BC,AD⊥BC, then find ratio
A(ΔABC)A(ΔADC).
View Solution