In Fig. 10.20, the sides AB, BC and CA of triangle ABC touch a circle with centre O and radius r at P, Q and R respectively. (FIGURE) Prove that: 1) AB + CQ = AC + BQ 2) Area (ABC) = (r/2)*(Perimeter of ABC)
Step by step video & image solution for In Fig. 10.20, the sides A B ,\ B C and C A of triangle A B C touch a circle with centre O and radius r at P ,\ Q and R respectively. (FIGURE) Prove that: 1) AB + CQ = AC + BQ 2) Area (ABC) = (r/2)*(Perimeter of ABC) by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Topper's Solved these Questions
Similar Questions
The sides AB,BC and AC of a triangle ABC touch a circle with centre O and radius r at P, Q, and R respectively.
View SolutionIn the given figure the sides AB, BE and CA of triangle ABC touch a circle with centre O and radius r at P,Q and R respectively.
Prove that : (i)AB+CQ=AC+BQ
(ii) Area (△ABC)=12(Perimeter of△ABC)×r
 View Solution
View Solution25. In figure, the sides AB, BC and CA of triangle ABC touch a circle with centre and radius r at P, Q and R respectively. Prove that (i ) AB+CQ=AC+BQ (ii) Area ΔABC=12 perimeter of ΔABC).r.
View SolutionIn an isosceles triangle ABC with AB=AC, a circle passing through B and C intersects the sides ABandAC atDandE respectively. Prove that DECB.
View SolutionIn an isosceles triangle ABC with AB=AC, a circle passing through B and C intersects the sides AB and AC at D and E respectively. Prove that DEBC
View SolutionA circle is touching the side BC of a △ABC at point P and touching AB and AC produced at Q and R respectively. Prove that AQ=12(perimeter of △ABC).
View SolutionA circle is touching the side BC of ΔABC at P and touching AB and AC produced at Q and R respectively Prove that AQ=12(Perimeter of ΔABC)
View SolutionIn the figure, all three sides of a triangle ABC touch the circle at points P, Q and R. Find the value of x.
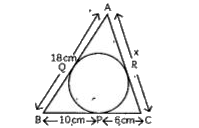 View Solution
View SolutionA circle is touching the side BC of ABC at P and touching AB and AC produced at Q and R respectively. Prove that AQ=12(Perimeter of ABC) .
View SolutionA circle with centre P is inscribed in the ΔABC Side AB, side BC and side A C touch the circle at points L,M and N respectively . Prove that :
A(ΔABC)=12(AB+BC+AC)×r
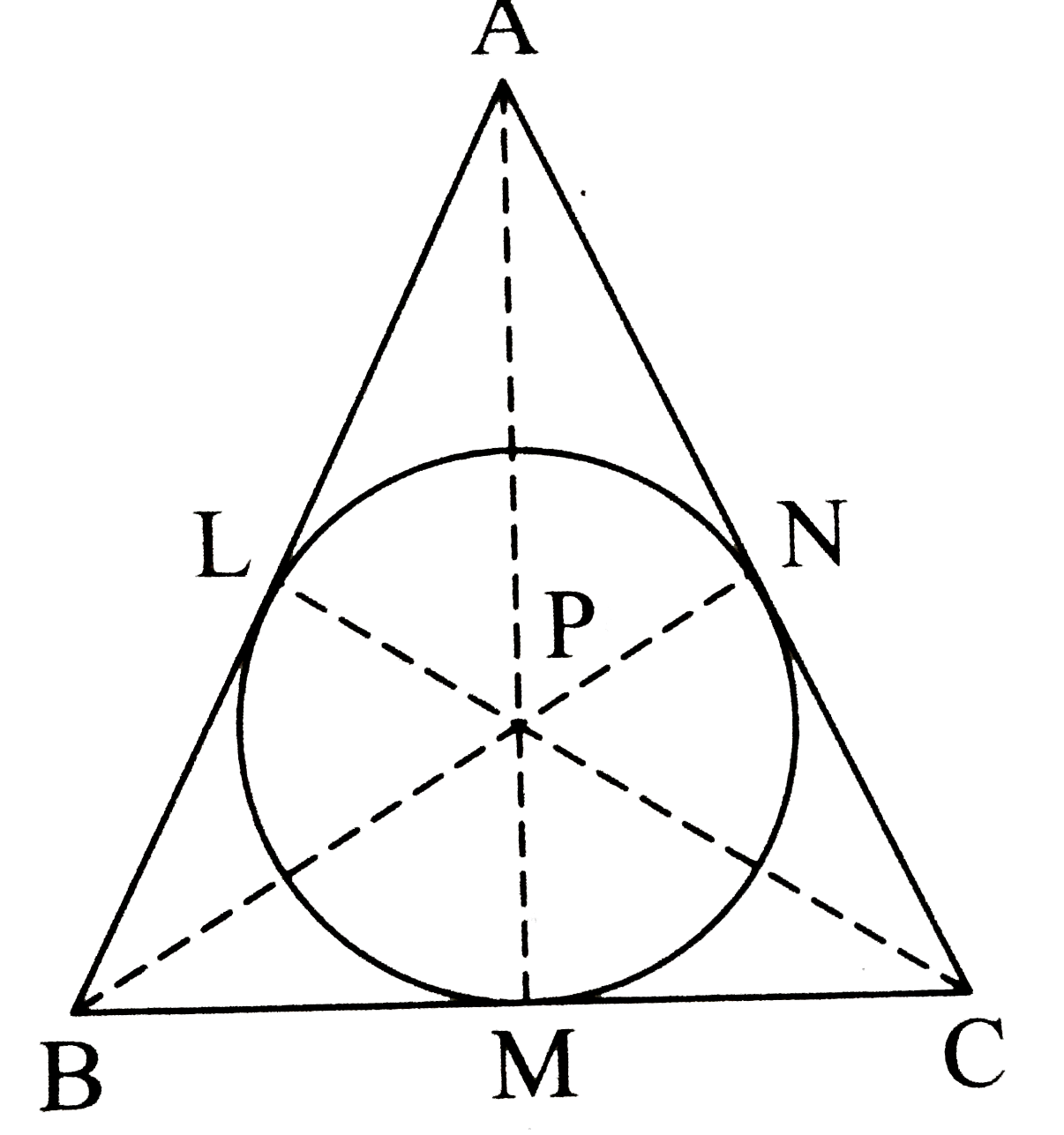 View Solution
View Solution

