In Figure, a crescent is formed by two circles which touch at A. C is the centre of the larger circle. The width of the crescent at BD is 9cm and at EF it is 5cm. Find (i) the radii of two circles (ii) the area of the shaded region.
To solve the problem, we need to find the radii of the two circles that form the crescent shape and the area of the shaded region. Let's break down the solution step by step.
Step 1: Define Variables
Let:
- R = radius of the larger circle
- r = radius of the smaller circle
Step 2: Relate the Widths of the Crescent
The width of the crescent at points BD is given as 9 cm, and at points EF is given as 5 cm. The width of the crescent can be expressed in terms of the radii:
- The width at BD (9 cm) can be expressed as R−r=4.5 cm (since the width is the difference of the two radii).
- The width at EF (5 cm) can be expressed as R−r=2.5 cm.
Step 3: Set Up the Equations
From the above relationships, we can establish the following equations:
1. R−r=4.5 (from BD)
2. R−r=2.5 (from EF)
Step 4: Solve the Equations
Now we can solve these equations. We know that:
- R−r=4.5
- R−r=2.5
However, we need to clarify that the widths are not directly equal but rather represent the distances from the center of the larger circle to the line segments BD and EF.
Step 5: Calculate the Radii
From the information given, we can conclude that:
- The total distance from the center of the larger circle to the line BD is R.
- The total distance from the center of the smaller circle to the line EF is r.
Thus, we can express:
- R=r+4.5
- R=r+2.5
Setting these equal gives us:
- r+4.5=r+2.5
This leads to:
- 4.5−2.5=0
- 2=0 (which is incorrect)
Step 6: Correct the Approach
Instead, we can use the difference in widths:
- The difference in the widths (9 cm - 5 cm) gives us the difference in the radii:
- R−r=4.5
Step 7: Solve for R and r
Using the equation R−r=4.5:
1. R=r+4.5
Now substituting R in terms of r into the area equations:
- The area of the shaded region can be calculated as the area of the larger circle minus the area of the smaller circle.
Step 8: Calculate Area of Shaded Region
The area A of a circle is given by the formula πR2.
Thus, the area of the shaded region is:
A=πR2−πr2=π(R2−r2)
Using the difference of squares:
A=π(R−r)(R+r)
Substituting R−r=4.5:
A=π(4.5)(R+r)
Step 9: Final Calculation
To find R+r, we can use:
R+r=9.5 (from the total width)
Thus:
A=π(4.5)(9.5)
Final Values
1. R=25 cm
2. r=20.5 cm
3. Area of shaded region A=π(4.5)(9.5)
Let:
- R = radius of the larger circle
- r = radius of the smaller circle
The width of the crescent at points BD is given as 9 cm, and at points EF is given as 5 cm. The width of the crescent can be expressed in terms of the radii:
- The width at BD (9 cm) can be expressed as R−r=4.5 cm (since the width is the difference of the two radii).
- The width at EF (5 cm) can be expressed as R−r=2.5 cm.
From the above relationships, we can establish the following equations:
1. R−r=4.5 (from BD)
2. R−r=2.5 (from EF)
Now we can solve these equations. We know that:
- R−r=4.5
- R−r=2.5
From the information given, we can conclude that:
- The total distance from the center of the larger circle to the line BD is R.
- The total distance from the center of the smaller circle to the line EF is r.
- R=r+4.5
- R=r+2.5
- r+4.5=r+2.5
- 4.5−2.5=0
- 2=0 (which is incorrect)
Instead, we can use the difference in widths:
- The difference in the widths (9 cm - 5 cm) gives us the difference in the radii:
- R−r=4.5
Using the equation R−r=4.5:
1. R=r+4.5
- The area of the shaded region can be calculated as the area of the larger circle minus the area of the smaller circle.
The area A of a circle is given by the formula πR2.
Thus, the area of the shaded region is:
A=πR2−πr2=π(R2−r2)
A=π(R−r)(R+r)
A=π(4.5)(R+r)
To find R+r, we can use:
R+r=9.5 (from the total width)
A=π(4.5)(9.5)
1. R=25 cm
2. r=20.5 cm
3. Area of shaded region A=π(4.5)(9.5)
Topper's Solved these Questions
Similar Questions
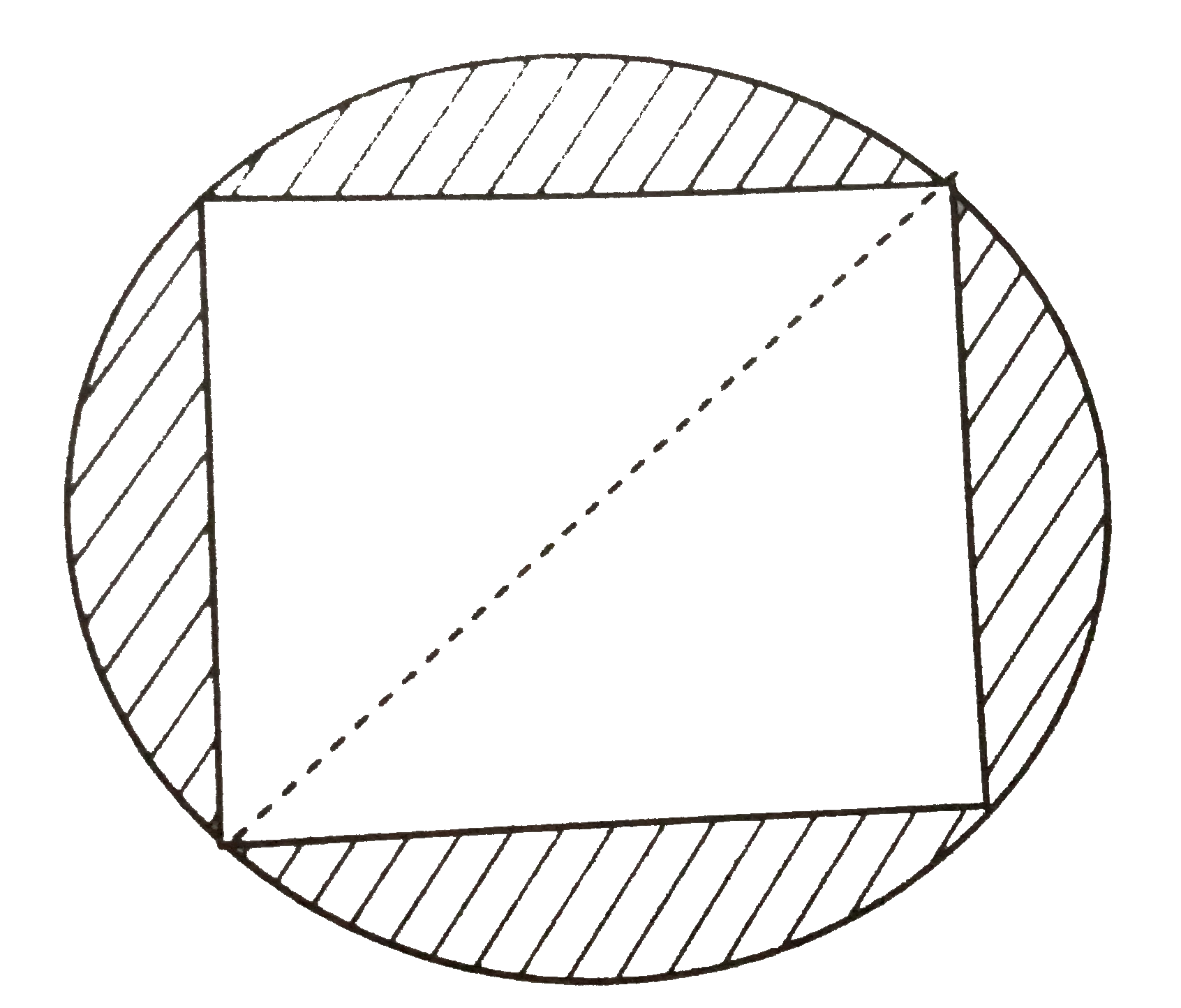
In figure, a square of diagonal 8 cm is inscribed in a circle. Find the area of the shaded region.
 View Solution
View SolutionThe adjoining figure shows two circles with the same centre. The radius of the larger circle is 10 cm and the radius of the smaller circle is 4 cm.Find:
(a) the area of the larger circle
(b) the area of the smaller circle
(c) the shaded area between two circles ( π=3.14)View SolutionTwo circles touch each other externally. The sum of their areas is 58πcm2 and the distance between their centres is 10 cm. Find the radii of the two circles.
View SolutionIn the given figure, PQ = 24 cm PR = 7 cm and O is the centre of the circle. Find the area of the shaded region. (Use π=3.14)
 View Solution
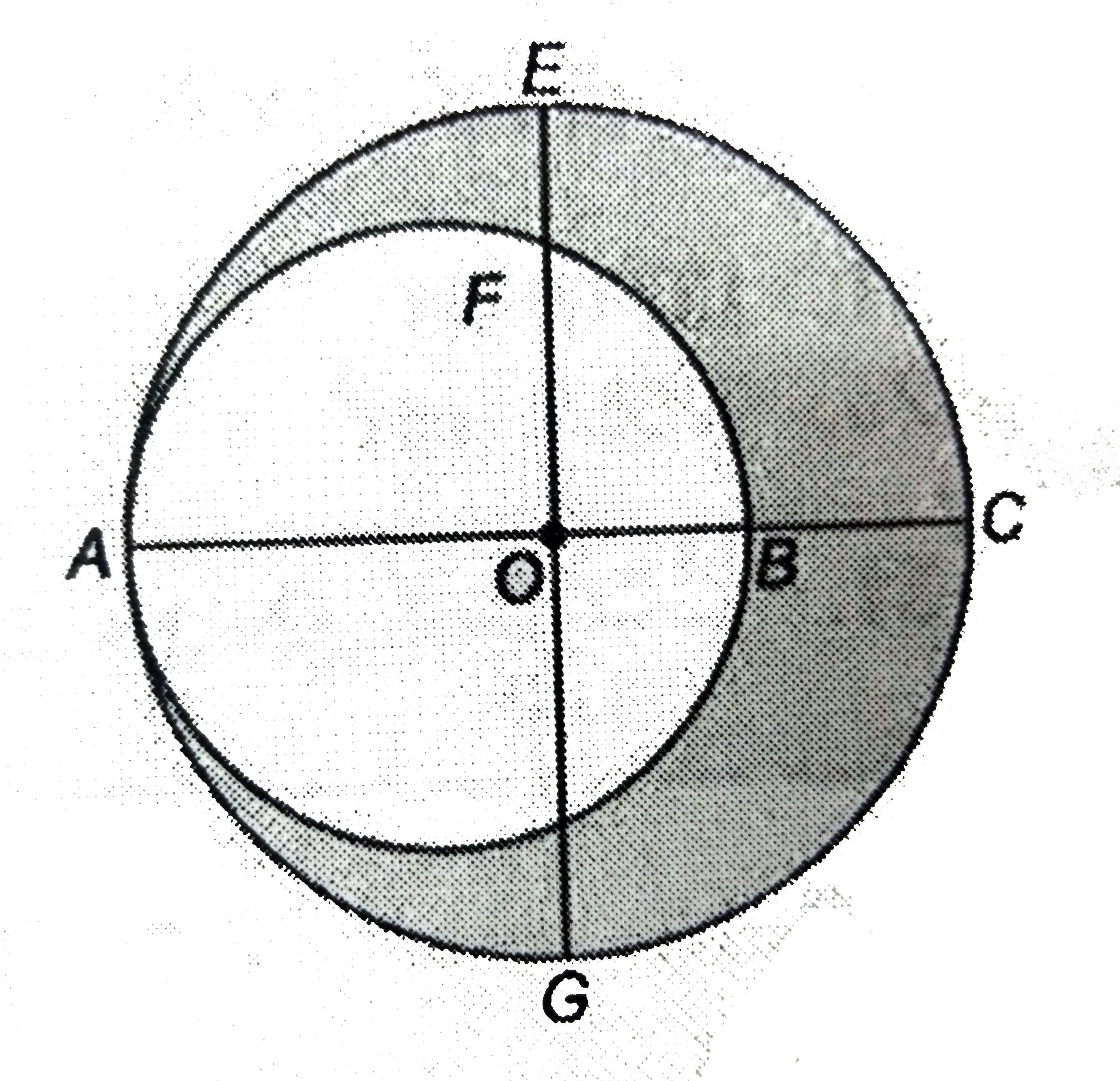
View SolutionIn the given figure, O is the centre of the bigger circle and AC is its diameter. Another circle with AB as diameter is drawn. If AC = 54 cm and BC = 10 cm. Find the area of the shaded region
 View Solution
View SolutionThe area of a ring is 528cm2 and the radius of the outer circle is 17 cm . Find :
(i) the radius of the smaller circle .
(ii) the width of the ring.View SolutionTwo circles touch internally. The sum of their areas is 116πcm2 and distance between their centres is 6 cm. Find the radii of the circles
View SolutionThe diameter of two circles are 32 cm and 24 cm. Find the radius of the circle having its area equal to sum of the areas of the two given circle.
View SolutionTwo circles touch externally. The sum of their areas is 130π sq. cm and the distance between their centres is 14 cm. Find the radii of the two circles.
View SolutionTwo circles touch internally. The sum of their areas is 116π cm2 and distance between their centres is 6 cm. Find the radii of the circles.
View Solution

