Question

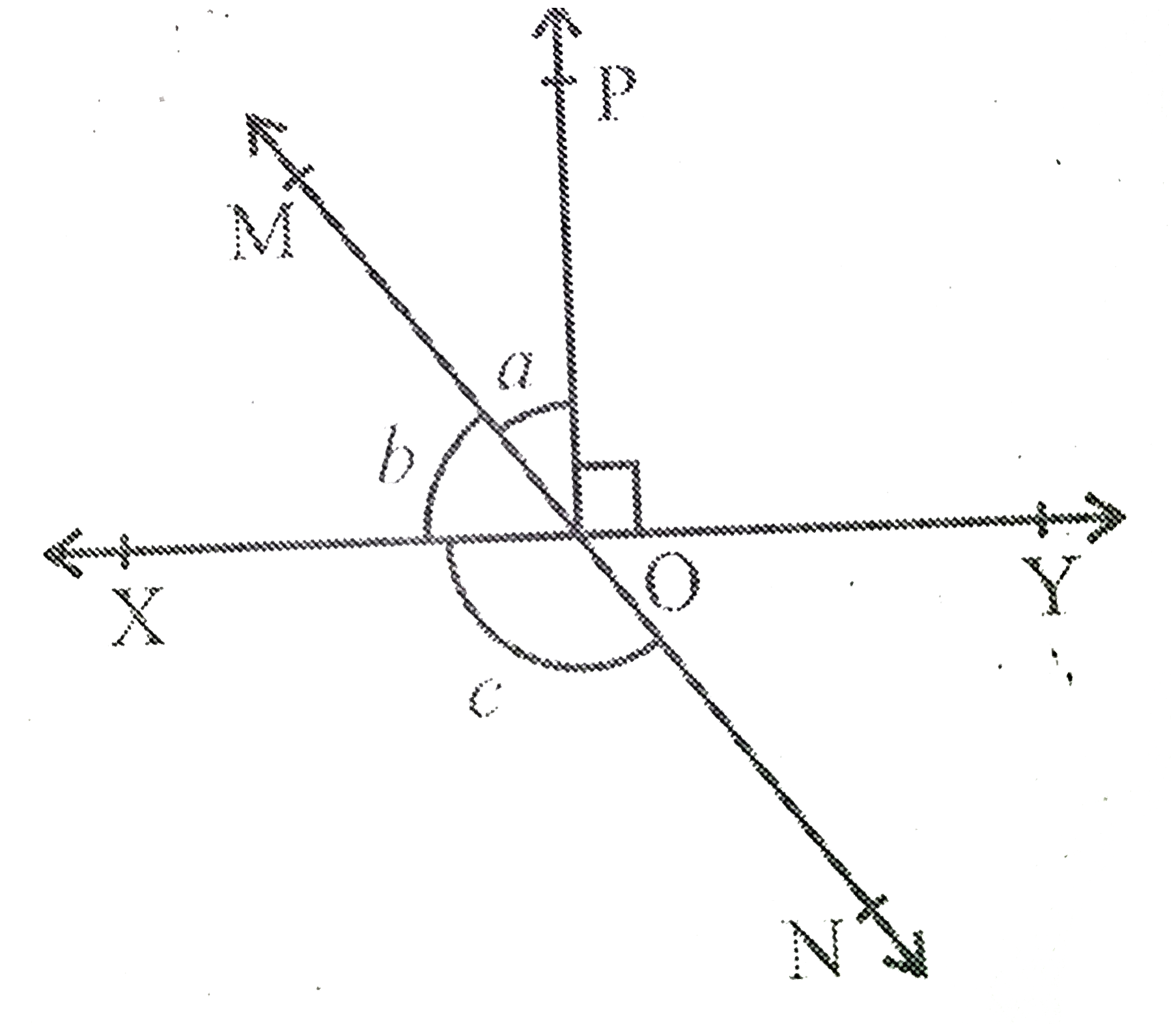
In Figure, lines $XYandMN$
intersect at $O$. If $\angle POY={90}^{0}anda:b=2:3,$
find $c$

Explanation

To solve the problem step by step, we will analyze the given information and apply the properties of angles formed by intersecting lines.

1. Identify the Given Information:
- We have two intersecting lines \( XY \) and \( MN \) that intersect at point \( O \).
- We know that \( \angle POY = 90^\circ \).
- The ratio of angles \( a \) to \( b \) is given as \( 2:3 \).

2. Use the Linear Pair Property:
- Since \( \angle POY \) and \( \angle POX \) form a linear pair, we can say:
\( \angle POY + \angle POX = 180^\circ \)
- Substituting the value of \( \angle POY \):
\( 90^\circ + \angle POX = 180^\circ \)
- Therefore, we can find \( \angle POX \):
\( \angle POX = 180^\circ - 90^\circ = 90^\circ \)

3. Express \( \angle POX \) in terms of \( a \) and \( b \):
- The angle \( \angle POX \) can also be expressed as the sum of angles \( a \) and \( b \):
\( \angle POX = a + b \)
- Since we have \( a + b = 90^\circ \), we can now express \( a \) and \( b \) in terms of a variable \( x \):
- Let \( a = 2x \) and \( b = 3x \).

4. Set up the equation:
- From the previous step, we have:
\( 2x + 3x = 90^\circ \)
- This simplifies to:
\( 5x = 90^\circ \)

5. Solve for \( x \):
- Dividing both sides by 5 gives:
\( x = \frac{90^\circ}{5} = 18^\circ \)

6. Find the values of angles \( a \) and \( b \):
- Now we can find \( a \) and \( b \):
\( a = 2x = 2 \times 18^\circ = 36^\circ \)
\( b = 3x = 3 \times 18^\circ = 54^\circ \)

7. Use the Linear Pair Property Again:
- Now, we need to find angle \( c \). The angles \( b \) and \( c \) also form a linear pair with \( \angle MOX \):
\( b + c = 180^\circ \)
- Substituting the value of \( b \):
\( 54^\circ + c = 180^\circ \)

8. Solve for \( c \):
- Rearranging gives:
\( c = 180^\circ - 54^\circ = 126^\circ \)

Final Answer:
Thus, the value of angle \( c \) is \( 126^\circ \).