In Figure, . Determine
To determine the angle ∠PQR given that OP∥RS, we can follow these steps:
Step 1: Identify the Given Angles
From the problem, we know:
- ∠OPQ=110∘
- ∠SRQ=130∘
Step 2: Find ∠RWS
Since OP∥RS, we can use the property of supplementary angles. The angles on the same side of the transversal (which is line QR in this case) add up to 180∘. Thus,
∠RWS=180∘−∠SRQ=180∘−130∘=50∘
Step 3: Find ∠PWQ
By the property of alternate interior angles, we have:
∠PWQ=∠RWS=50∘
Step 4: Find ∠WPQ
Using the supplementary angle property again on line OP:
∠OPQ+∠WPQ=180∘
Substituting the known value:
110∘+∠WPQ=180∘
Thus,
∠WPQ=180∘−110∘=70∘
Step 5: Use the Triangle Sum Property
In triangle PWQ, the sum of the angles is 180∘:
∠PQR+∠PWQ+∠WPQ=180∘
Substituting the known values:
∠PQR+50∘+70∘=180∘
This simplifies to:
∠PQR+120∘=180∘
Thus,
∠PQR=180∘−120∘=60∘
Final Answer
Therefore, the value of ∠PQR is 60∘.
---
From the problem, we know:
- ∠OPQ=110∘
- ∠SRQ=130∘
Since OP∥RS, we can use the property of supplementary angles. The angles on the same side of the transversal (which is line QR in this case) add up to 180∘. Thus,
∠RWS=180∘−∠SRQ=180∘−130∘=50∘
By the property of alternate interior angles, we have:
∠PWQ=∠RWS=50∘
Using the supplementary angle property again on line OP:
∠OPQ+∠WPQ=180∘
Substituting the known value:
110∘+∠WPQ=180∘
Thus,
∠WPQ=180∘−110∘=70∘
In triangle PWQ, the sum of the angles is 180∘:
∠PQR+∠PWQ+∠WPQ=180∘
Substituting the known values:
∠PQR+50∘+70∘=180∘
This simplifies to:
∠PQR+120∘=180∘
Thus,
∠PQR=180∘−120∘=60∘
Therefore, the value of ∠PQR is 60∘.
Topper's Solved these Questions
Similar Questions
In Figure, PQRS is a square. Determine ∠SRP.
View SolutionIn Figure, determine ∠P+∠Q+∠R+∠S+∠T.
View SolutionIn Figure, PS=PR, ∠TPS= ∠QPR. Prove that PT=PQ
View SolutionIn △PQR , right-angled at Q, PQ=3cm and PR=6cm . Determine ∠P and ∠R .
View SolutionIn PQR , right-angled at Q, PQ=3cm and PR=6cm . Determine ∠P and ∠R .
View SolutionIn Figure, PS=QR and ∠SPQ=∠RQP. Prove that PQS ≅ QPR, PR=QS and ∠QPR= ∠PQS
View SolutionIn Figure , find out ∠PXR, if PQ ∣∣ RS.
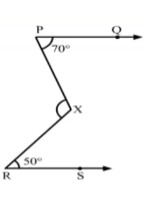 View Solution
View SolutionIn figure, if PQ⊥PS,PQ∣∣SR,∠SQR=280 and ∠QRT=650, then find the values of x and y. Figure
View SolutionIn Figure, If PQ=PT and ∠TPS=∠QPR, prove that PRS is isosceles
View SolutionIn Figure, PQR is a triangle and S is any point in its interior, show that SQ+SR<PQ+PR. Given : S is any point in the interior of PQR To Prove : SQ+SR<PQ+PR Construction: Produce QS to meet PR in T
View Solution

