Question

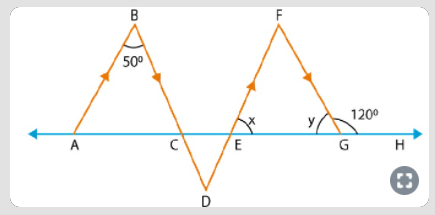
In Figure, show that $AB\mid \mid EF$

Explanation

To prove that lines $$AB$$ and $$EF$$ are parallel, we will use the properties of angles formed by a transversal intersecting two lines. Here’s the step-by-step solution:

Step 1: Identify Given Angles
We are given the following angles:
- \( \angle CAB = 57^\circ \)
- \( \angle ECD = 35^\circ \)
- \( \angle ACI = 22^\circ \)
- \( \angle CEF = 145^\circ \)

Step 2: Find \( \angle ACD \)
We know that:
\( \angle ACD = \angle ACE + \angle ECD \)
Substituting the values:
\( \angle ACD = 22^\circ + 35^\circ = 57^\circ \)

Step 3: Establish Relationship Between Angles
Since \( \angle BAC = \angle ACD \) (both equal \( 57^\circ \)), we have:
\( \angle BAC = \angle ACD \)
This indicates that \( AB \) and \( CD \) are cut by the transversal \( BC \).

Step 4: Apply the Corresponding Angles Postulate
According to the Corresponding Angles Postulate, if two lines are cut by a transversal and the corresponding angles are equal, then the lines are parallel. Here, since:
\( \angle ABC = \angle BCD = 57^\circ \)
we can conclude that:
\( AB \parallel CD \)

Step 5: Find \( \angle DCE \)
Now, we will check the angles on the other side of the transversal \( CE \):
\( \angle DCE + \angle CEF = 180^\circ \)
Substituting the values:
\( 35^\circ + 145^\circ = 180^\circ \)
This shows that the angles are supplementary.

Step 6: Conclude \( CD \parallel EF \)
Since the sum of the interior angles on the same side of the transversal \( CE \) is \( 180^\circ \), we conclude that:
\( CD \parallel EF \)

Step 7: Combine Results
From Steps 4 and 6, we have:
- \( AB \parallel CD \)
- \( CD \parallel EF \)

By the transitive property of parallel lines, we conclude:
\( AB \parallel EF \)

Final Conclusion
Thus, we have shown that \( AB \) is parallel to \( EF \).

---