In Figure, there are two concentric circles with centre O . PR and PQS are tangents to the inner circle from point plying on the outer circle. If PR=7.5cm , then PS is equal to (a) 10cm (b) 12cm (c) 15cm (d) 18cm
To solve the problem step by step, we will analyze the given information about the concentric circles and the tangents.
Step 1: Understand the Configuration
We have two concentric circles with center O. The tangents PR and PS are drawn from point P on the outer circle to the inner circle.
Step 2: Use the Property of Tangents
From the property of tangents, we know that the lengths of tangents drawn from a common external point to a circle are equal. Therefore, we can state:
PR=PS
Step 3: Identify the Given Length
We are given that:
PR=7.5cm
Step 4: Apply the Tangent Property
Since PR=PS, we can substitute the value of PR into the equation:
PS=PR=7.5cm
Step 5: Find the Length of PS
Now, we need to find the total length of PS. The tangents from point P to the inner circle are equal, so we can express PS in terms of PR:
PS=PQ+QS
Where PQ is equal to PR and QS is also equal to PR.
Step 6: Calculate PS
Since both PQ and QS are equal to PR:
PS=PR+PR=7.5cm+7.5cm=15cm
Conclusion
Thus, the length of PS is:
PS=15cm
The correct option is (c) 15 cm.
---
We have two concentric circles with center O. The tangents PR and PS are drawn from point P on the outer circle to the inner circle.
From the property of tangents, we know that the lengths of tangents drawn from a common external point to a circle are equal. Therefore, we can state:
PR=PS
We are given that:
PR=7.5cm
Since PR=PS, we can substitute the value of PR into the equation:
PS=PR=7.5cm
Now, we need to find the total length of PS. The tangents from point P to the inner circle are equal, so we can express PS in terms of PR:
PS=PQ+QS
Where PQ is equal to PR and QS is also equal to PR.
Since both PQ and QS are equal to PR:
PS=PR+PR=7.5cm+7.5cm=15cm
Thus, the length of PS is:
PS=15cm
Topper's Solved these Questions
Similar Questions
In Figure, PR= (a) 20cm (b) 26cm (c) 24cm (d) 28cm
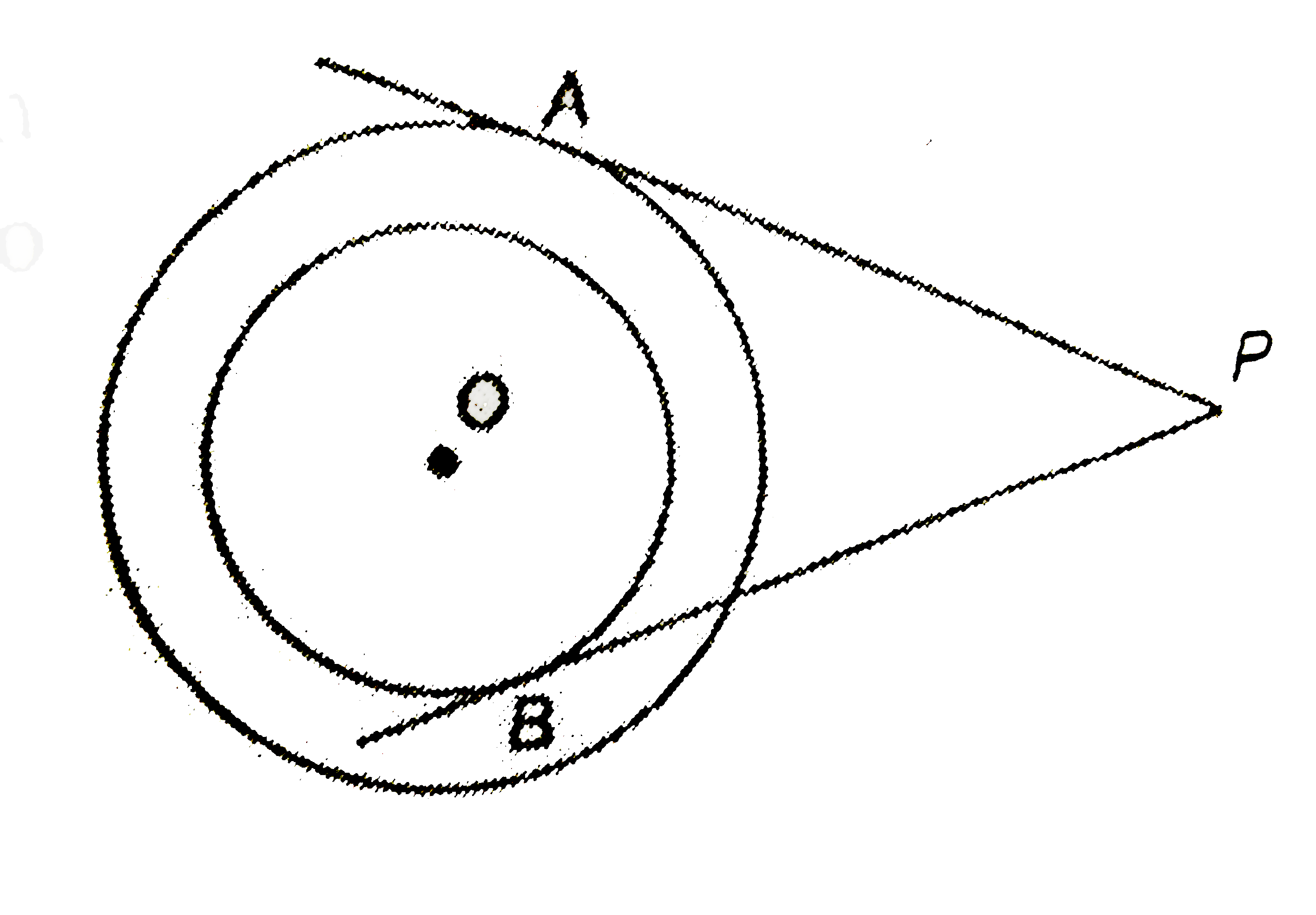
View SolutionIn Figure, there are two concentric circles with centre O of radii 5cmand3cm . From an external point P, tangents PAandPB are drawn to these circles. If AP=12cm, find the length of BP.
View SolutionIn Figure, two equal circles touch each other at T , if QP=4.5cm , then QR= (a) 9cm (b) 18cm (c) 15cm (d) 13.5cm
View SolutionIn the given figure two concentric circles with centre O are of radii 5 cm and 3 cm. From an external point P, tangents PA and PB are drawn to these circles. If AP =12 cm find BP.
 View Solution
View SolutionAP and AQ are tangents drawn from a point A to a circle with centre O and radius 9cm. If OA=15cm, then AP+AQ= (a) 12cm (b) 18cm (c) 24cm (d) 36cm
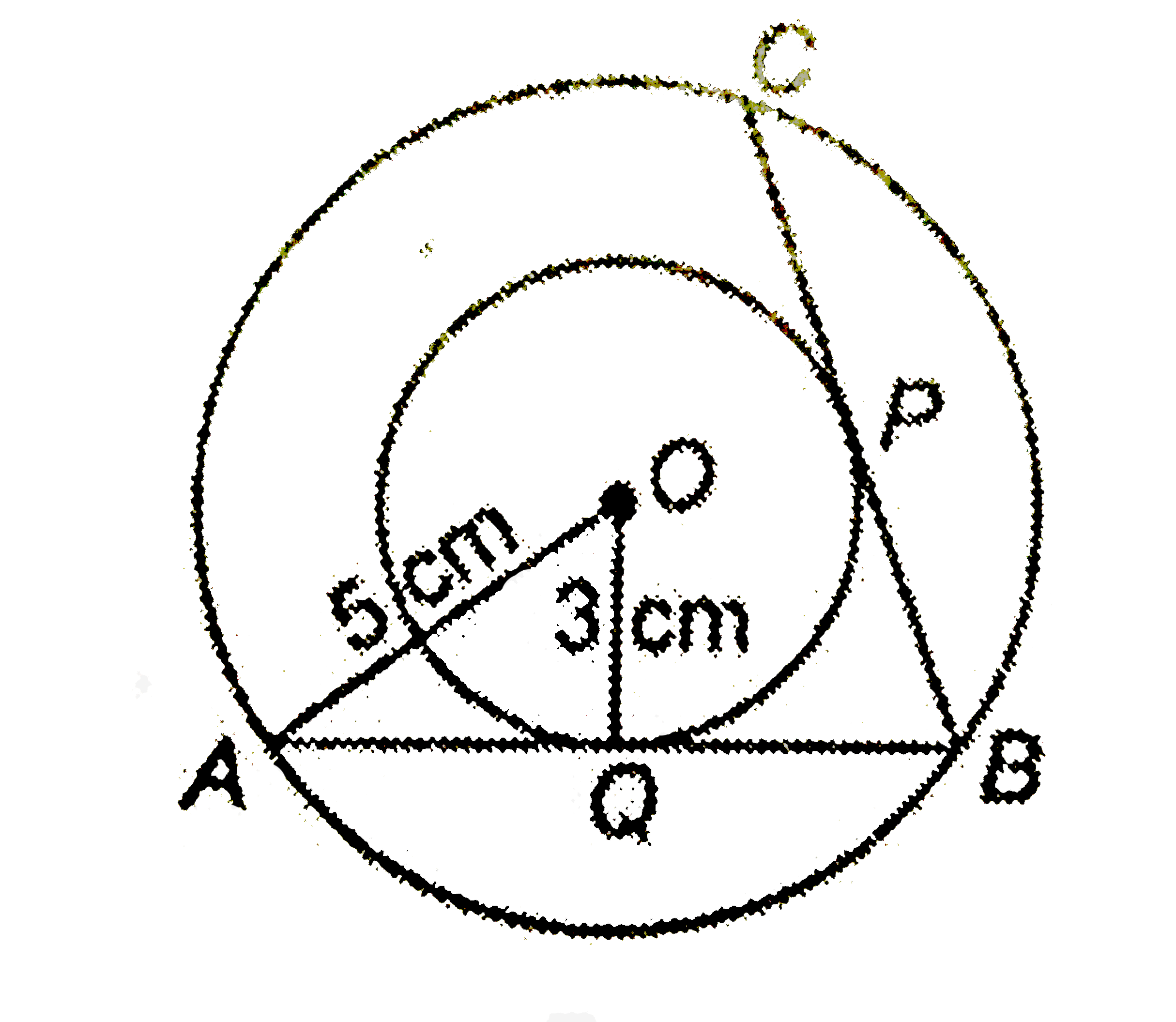
View SolutionTwo concentric circle of radii 3cm and 5cm are given. Find the chord BC which touches the inner circle at P.
 View Solution
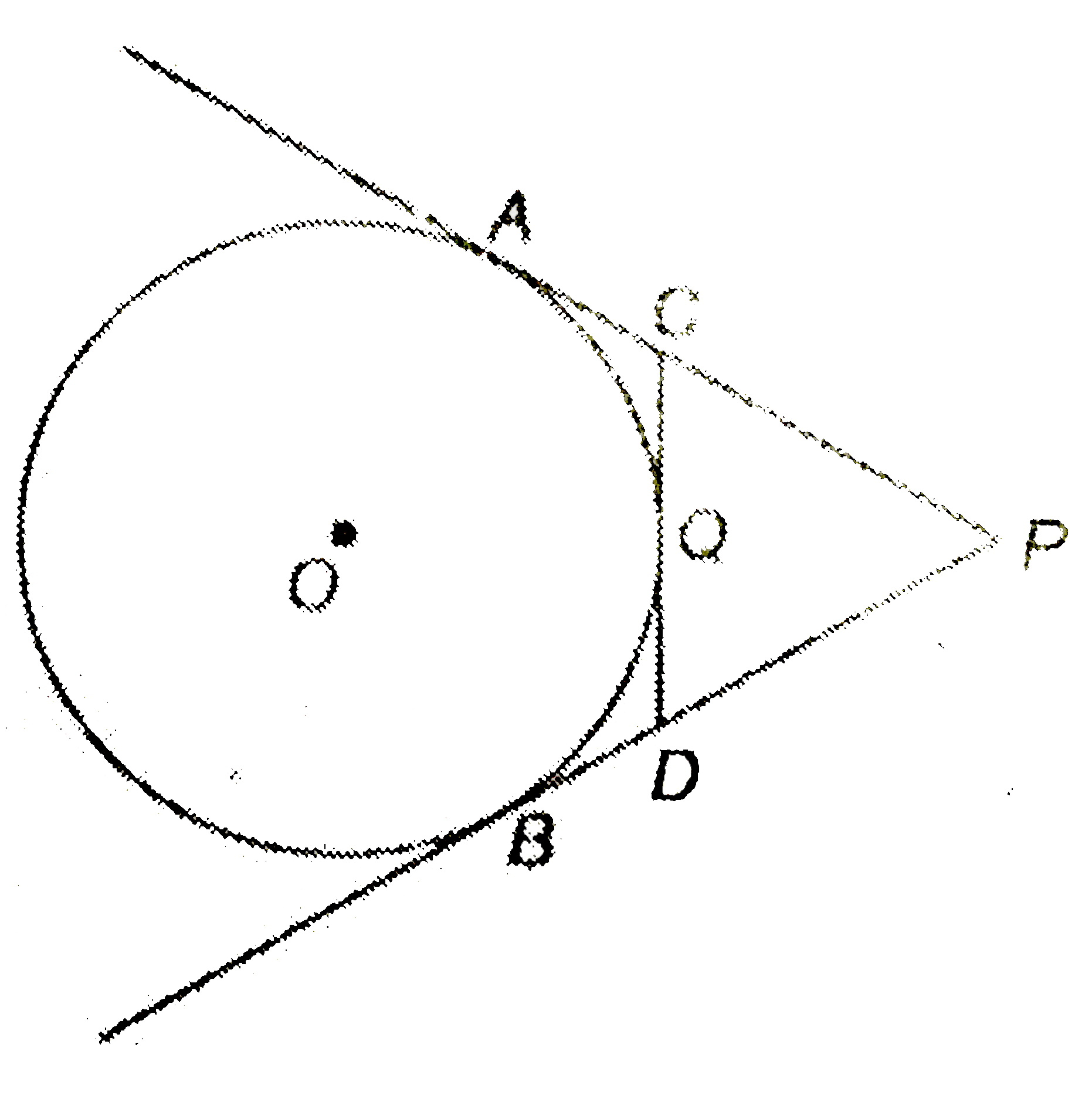
View SolutionIn the given figure, PA and PB are tangents to the circle from an external point P. CD is another tangent touching the circle at Q. If PA =12 cm, QC=3cm, then find PC+PD.
 View Solution
View SolutionIn Figure, RQ is a tangent to the circle with centre O . If SQ=6cm and QR=4cm , then OR= (a) 8cm (b) 3cm (c) 2.5cm (d) 5cm
View SolutionOut of the two concentric circle the radius of the outer circle is 5 cm and the chord AC of the length 8 cm is a tangent to the inner circle find the radius of the inner circle.
View SolutionPQ is a tangent to a circle with centre O at the point P . If △OPQ is an isosceles triangle, then ∠OQP is equal to :
View Solution

