In Figure, two circles intersect at A and B . The centre of the smaller circle is O and it lies on the circumference of the larger circle. If ∠APB=700, find ∠ACB
arcAB subtends ∠AOBat the centre and ∠APBat the remaining part of the circle.
∠AOB=2∠APB=2×700
Now in cyclic quadrilateral AOBC
∠AOB+∠ACB=1800(sum of opposite angle)
⇒1400+∠ACB=1800
∠ACB=1800−1400
∠ACB=400
Topper's Solved these Questions
Similar Questions
In Figure, two circles intersect at A and B . The centre of the smaller circle is O and it lies on the circumference of the larger circle. If ∠APB=700, find ∠ACB
View SolutionThe figure shows two circles which intersect at A and B. The centre of the smaller circle is O and lies on the circumference of the larger circle. Given ∠APB=a∘, Calculate in terms of a∘, the value of
View Solution
Knowledge Check
- Question 1 - Select One
There are two circles intersecting each other . Another smaller circle with centre O , is lying between the common region of two larger circles. Centres of the circle ( i.e., A,O and B) are lying on a straight line. AB =16 cm and the radii of the larger circles are 10 cm each . What is the area of the smaller circle ?
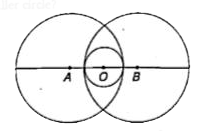 Aa. 4πcm2Bb. 2πcm2Ccc. 4πcm2Dd. π4cm2
Aa. 4πcm2Bb. 2πcm2Ccc. 4πcm2Dd. π4cm2 - Question 2 - Select One
A circle (with centre at O) is touching two intersecting lines AX and BY. The two points of contact A and B subtend an angle of 65∘ at any point C on the circumference of the circle. If P is the point of intersection for the two lines, then the measure of ∠APO is :
A25∘B65∘C90∘D40∘ - Question 3 - Select One
A circle (with centre at O) is touching two intersecting lines AX and BY. The two points of contact A and B subtend an angle of 65∘ at any point C on the circumference of the circle. If P is the point of intersection for the two lines, then the measure of ∠APO is :
A25∘B65∘C90∘D40∘
In the figure, two circles intersect each other at points A and B . C is a point on the smaller circle. Secant CA and secant CB of the smaller circle intersect the bigger circle at points D and E respectively then prove : seg DE ∣∣ line CF
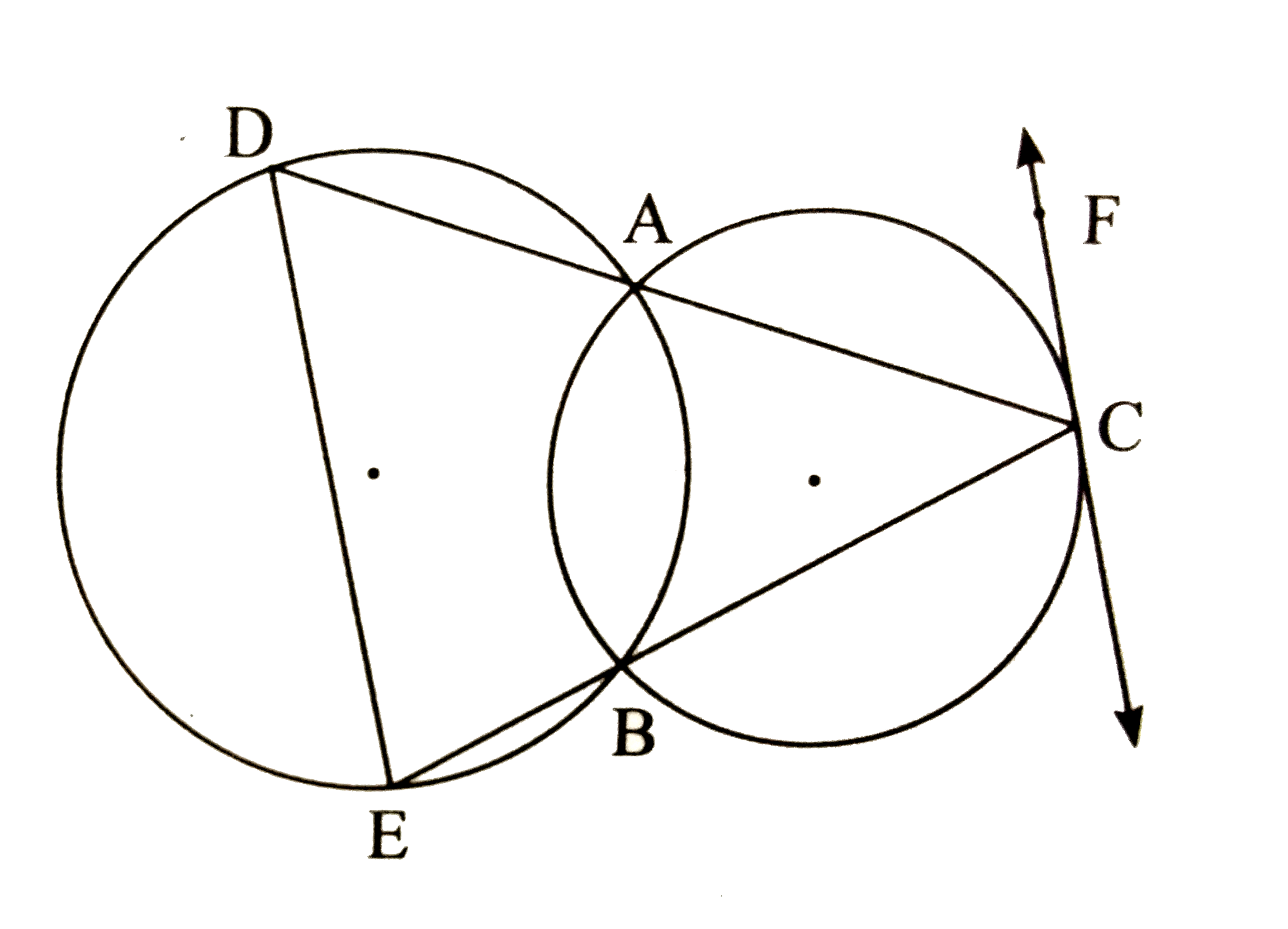 View Solution
View SolutionIn Figure, O and O' are centres of two circles intersecting at B and C.ACD is a straight line, find x
View SolutionThe adjoining figure shows two circles with the same centre. The radius of the larger circle is 10 cm and the radius of the smaller circle is 4 cm.
View SolutionA smaller circle touches internally to a larger circle at A and passes through the centre of the larger circle. O is the centre of the larger circle and BA, OA are of the diameters of the larger and smaller circles respectively. Chord AC intersects the smaller circle at a point D. If AC=12cm, then AD is :
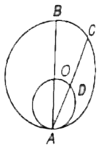 View Solution
View SolutionA circle (with centre at O) is touching two intersecting lines AX and BY. The two points of contact A and B subtend an angle of 65∘ at any point C on the circumference of the circle. If P is the point of intersection for the two lines, then the measure of ∠APO is :
View Solution

