In Figure, A is the centre of the circle. ABCD is a parallelogram and CDE is a straight line. Find ∠BCD : ∠ABE.
ABCD∣∣gm
∠A=∠C
∠C=∠ADE(corresponding angles)
∠BCD=∠ADE
Also ∠ABE=BED(alternate angles)
arcBD subtends ∠BAD at the centre BDE at the remaining part of the circle.
∠BED=12∠A=12∠C=12∠ADE,
∠BCD/ABE=∠BAD/BED=∠ADE∠ADE/2=21
∠BCD:∠ABE=2:1
Topper's Solved these Questions
Similar Questions
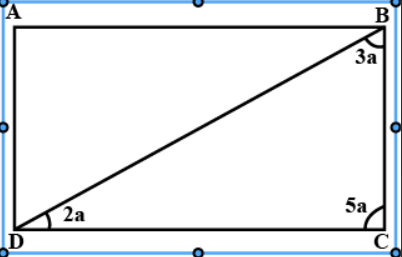
In Figure, find the four angles A, B, C and D in the parallelogram ABCD
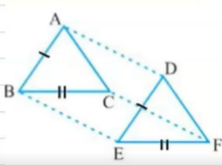
View SolutionIf ABC and DEF are two triangles such that AB, BC are respectively equal and parallel to DE, EF, then show that Quadrilateral ABED is a parallelogram Quadrilateral BCFE is a parallelogram AC=DF (iv) ABC ≅ DEF
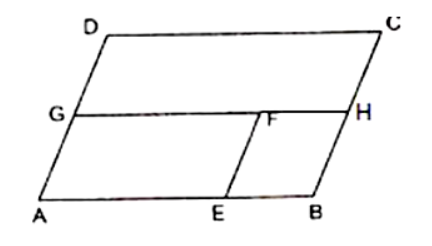
View SolutionIn Figure, ABCD and AEFG are two parallelograms. If ∠C=580, find ∠F
View SolutionIn Figure ABCDandAEFD are two parallelograms. Prove that: PE=FQ
View SolutionIn a parallelogram ABCD, if ∠D=115∘, then write the measure of ∠A
View SolutionABCD is a parallelogram in which ∠A=70∘. Compute ∠B, ∠C and ∠D.
View SolutionABCD is a parallelogram in which ∠A=70∘ Compute ∠B, ∠C and ∠D
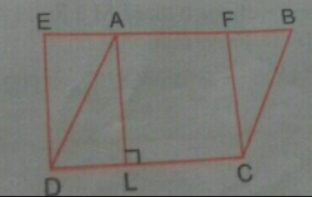
View SolutionIn the following figure, ABCD is a parallelogram and EFCD is a rectangle. Also, AL⊥DC. Prove that
(i) ar(ABCD)=ar(EFCD)
(ii) ar(ABCD)=DC×AL.
View SolutionIn Figure, ABCD is a rectangle in which CD=6cm,AD=8cm Find the area of parallelogram CDEF
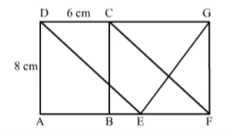View SolutionIn Figure, ABCD is a parallelogram and EFCD is a rectangle. Also AL⊥DC. Prove that : ar(ABCD)=ar(EFCD) ar(ABCD)=DC×AL
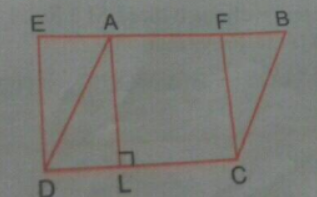View Solution