In parallelogram ABCD, E is the mid-point of AD and F is the mid-point of BC. Prove that BFDE is a parallelogram.
To prove that quadrilateral BFDE is a parallelogram, we will follow these steps:
Step 1: Identify the midpoints
Let E be the midpoint of side AD and F be the midpoint of side BC in parallelogram ABCD.
Step 2: Use the properties of a parallelogram
In a parallelogram, opposite sides are equal and parallel. Therefore, we have:
- AD = BC (opposite sides of parallelogram)
- AB = CD (opposite sides of parallelogram)
Step 3: Show that ED is parallel to BF
Since E is the midpoint of AD, we can express the length of segment ED as:
ED=12AD
Similarly, since F is the midpoint of BC, we can express the length of segment BF as:
BF=12BC
Since AD is equal to BC (as established in Step 2), we have:
ED=12AD=12BC=BF
Step 4: Conclude that BFDE is a parallelogram
Now we have shown that:
1. ED is equal to BF (ED = BF)
2. Since E and F are midpoints, we can also show that ED is parallel to BF.
According to the properties of parallelograms, if one pair of opposite sides is both equal and parallel, then the quadrilateral formed by those sides is a parallelogram. Therefore, BFDE is a parallelogram.
Final Statement
Thus, we have proved that quadrilateral BFDE is a parallelogram.
---
Let E be the midpoint of side AD and F be the midpoint of side BC in parallelogram ABCD.
In a parallelogram, opposite sides are equal and parallel. Therefore, we have:
- AD = BC (opposite sides of parallelogram)
- AB = CD (opposite sides of parallelogram)
Since E is the midpoint of AD, we can express the length of segment ED as:
ED=12AD
Similarly, since F is the midpoint of BC, we can express the length of segment BF as:
BF=12BC
ED=12AD=12BC=BF
Now we have shown that:
1. ED is equal to BF (ED = BF)
2. Since E and F are midpoints, we can also show that ED is parallel to BF.
Thus, we have proved that quadrilateral BFDE is a parallelogram.
Topper's Solved these Questions
Similar Questions
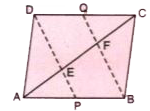
The adjoining figure shows a parallelogram ABCD in which P is mid-point of AB and Q is mid-point of CD. Prove that AE=EF=FC.
View SolutionABCD is a square, X is the mid-point of AB and Y the mid-point of BC. Prove that
DX is perpendicular to AY.
View SolutionD is the mid-point of side AB of the triangle ABC.E is the mid-point of CD and F is the mid-point of AE. Prove that 8× area of (ΔAFD)= area of ΔABC
View SolutionABCD is a parallelogram. E is the mid-point of AB and F is the mid-point of CD. GH is any line that intersects AD, EF and BC at G, P and H respectively. Prove that : GP=PH
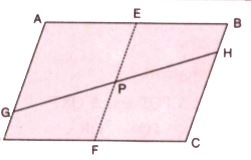
View SolutionIn trapezium ABCD, sides AB and DC are parallel to each other. E is mid-point of AD and F is mid-point of BC.
Prove that : AB + DC = 2EF.
View SolutionABCD is a square, X is the mid-point of AB and Y the mid-point of BC. Prove that
∠DXA=∠AYB
View SolutionABCD is a square, X is the mid-point of AB and Y the mid-point of BC. Prove that
the triangles ADX and BAY are congruent.
View SolutionIn parallelogram ABCD, E is the mid-point of side AB and CE bisect angle BCD. Prove that :
(i) AE=AD
(ii) DE bisect ∠ADC and
(iii) Angle DEC is a right angle.
View SolutionE is the mid-point of side AB and F is the mid point of side DC of parallelogram ABCD . Prove that AEFD is a parallelogram.
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
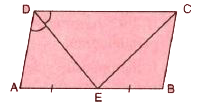
BC= BE
View Solution
