ABCD is a square, X is the mid-point of AB and Y the mid-point of BC. Prove that
To prove that ∠DXA=∠AYB in the square ABCD where X and Y are midpoints of sides AB and BC respectively, we can follow these steps:
Step 1: Construct the Square
Construct square ABCD with vertices A, B, C, and D. Mark the midpoints X and Y on sides AB and BC respectively.
Step 2: Identify the Midpoints
Since X is the midpoint of AB, we have:
AX=XB=AB2
Since Y is the midpoint of BC, we have:
BY=YC=BC2
Given that ABCD is a square, we know that AB=BC.
Step 3: Establish Equal Lengths
From the properties of the square, we can conclude:
AX=BY=AB2=BC2
This means AX=BY.
Step 4: Analyze the Triangles
Now, consider triangles △ADX and △BAY:
- AD=BA (sides of the square)
- AX=BY (from the previous step)
- ∠A=∠B=90∘ (angles of the square)
Step 5: Apply the Congruence Criterion
Since we have:
1. AD=BA
2. AX=BY
3. ∠A=∠B=90∘
By the Side-Angle-Side (SAS) congruence criterion, we can conclude:
△ADX≅△BAY
Step 6: Conclude the Angle Equality
From the congruence of triangles, we know that corresponding parts of congruent triangles are equal. Therefore:
∠DXA=∠AYB
Final Statement
Thus, we have proved that ∠DXA=∠AYB.
---
Construct square ABCD with vertices A, B, C, and D. Mark the midpoints X and Y on sides AB and BC respectively.
Since X is the midpoint of AB, we have:
AX=XB=AB2
Since Y is the midpoint of BC, we have:
BY=YC=BC2
Given that ABCD is a square, we know that AB=BC.
From the properties of the square, we can conclude:
AX=BY=AB2=BC2
This means AX=BY.
Now, consider triangles △ADX and △BAY:
- AD=BA (sides of the square)
- AX=BY (from the previous step)
- ∠A=∠B=90∘ (angles of the square)
Since we have:
1. AD=BA
2. AX=BY
3. ∠A=∠B=90∘
△ADX≅△BAY
From the congruence of triangles, we know that corresponding parts of congruent triangles are equal. Therefore:
∠DXA=∠AYB
Thus, we have proved that ∠DXA=∠AYB.
Topper's Solved these Questions
- CHAPTERWISE REVISION (STAGE 3) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 3) EXERCISE - ISOSCELES TRIANGLES
2 Videos
- CHAPTERWISE REVISION (STAGE 3) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 3) EXERCISE - INEQUALITIES
7 Videos
- CHAPTERWISE REVISION (STAGE 3) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 3) EXERCISE - LOGARITHM
9 Videos
- CHAPTERWISE REVISION (STAGE 1) BOOK - ICSECHAPTER - CHAPTERWISE REVISION (STAGE 1) EXERCISE - Graphical solution
10 Videos
- CIRCLEBOOK - ICSECHAPTER - CIRCLEEXERCISE - EXERCISE 17(D)
12 Videos
Similar Questions
ABCD is a square, X is the mid-point of AB and Y the mid-point of BC. Prove that
the triangles ADX and BAY are congruent.
View SolutionABCD is a square, X is the mid-point of AB and Y the mid-point of BC. Prove that
DX is perpendicular to AY.
View SolutionIn parallelogram ABCD, E is the mid-point of AD and F is the mid-point of BC. Prove that BFDE is a parallelogram.
View SolutionD is the mid-point of side AB of the triangle ABC.E is the mid-point of CD and F is the mid-point of AE. Prove that 8× area of (ΔAFD)= area of ΔABC
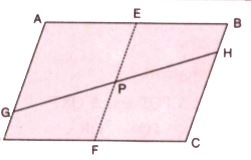
View SolutionABCD is a parallelogram. E is the mid-point of AB and F is the mid-point of CD. GH is any line that intersects AD, EF and BC at G, P and H respectively. Prove that : GP=PH
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
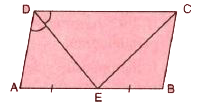
BC= BE
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
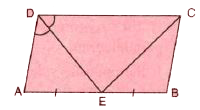
∠DEC=90∘
View SolutionIn trapezium ABCD, sides AB and DC are parallel to each other. E is mid-point of AD and F is mid-point of BC.
Prove that : AB + DC = 2EF.
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
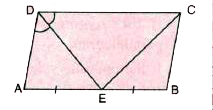
CE bisects angle C
View SolutionABCD is a square EF is parallel to BD. R is the mid-point of EF. Prove that :
(i) BE=DF
(ii) AR bisects angle BAD
(iii) If AR produced it will pass through C.
View Solution
