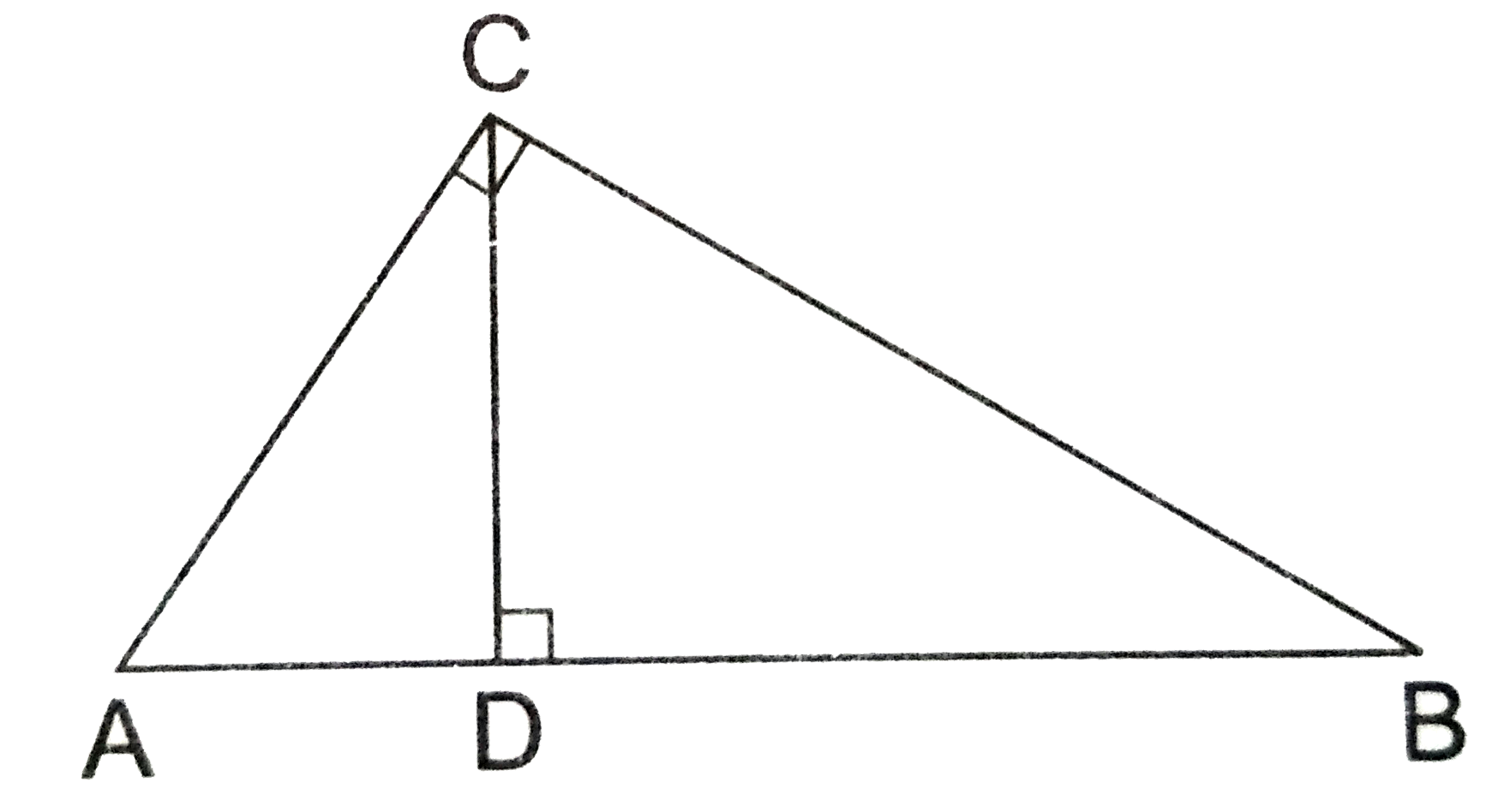
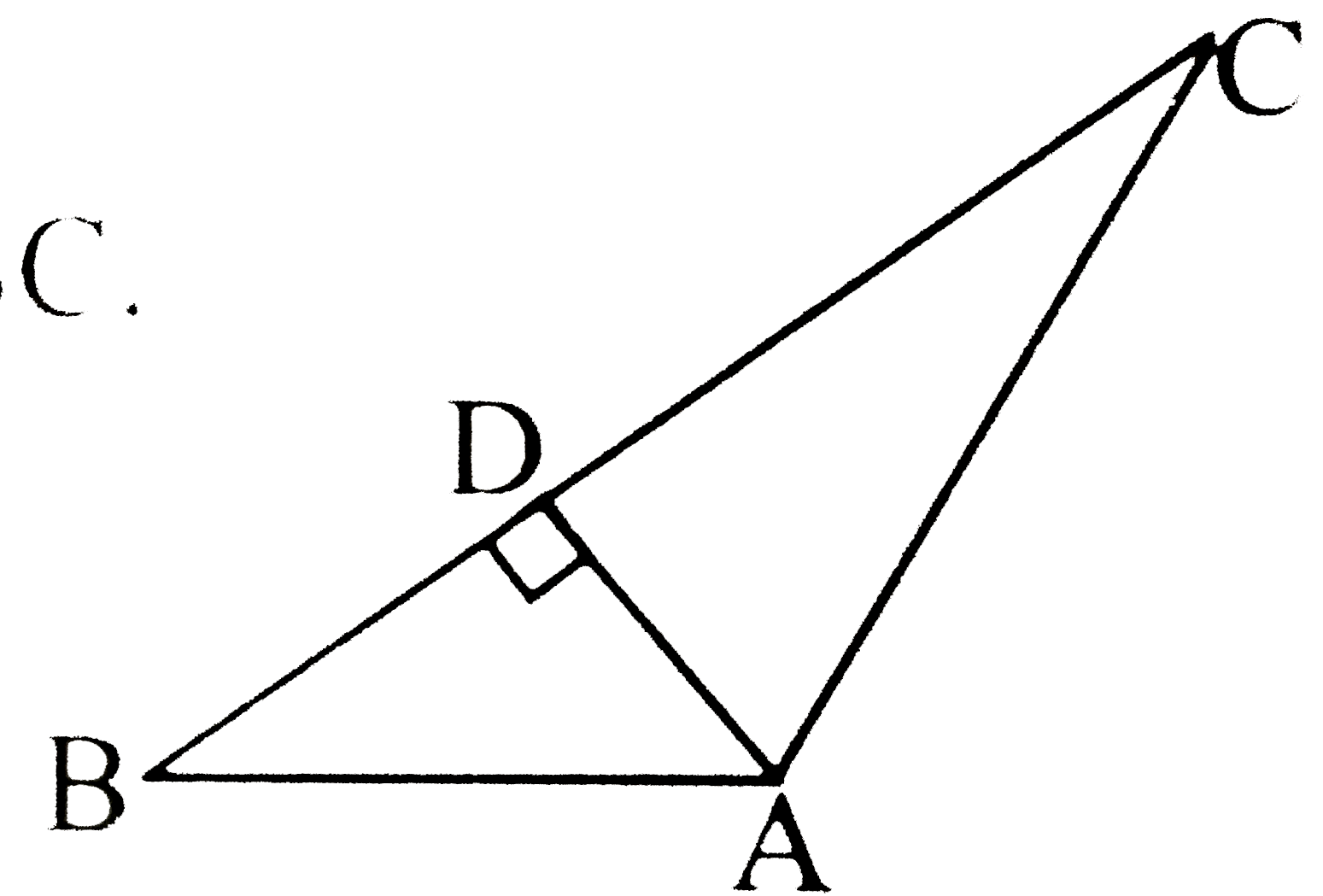
In the given figure ∠ABC=90∘andCD⊥AB. Prove that BC2AC2=BDAD
Video Solution
Text Solution
ΔABC~ΔCBD
⇒BCBD=ABCB⇒BC2=AB⋅BD ....(ii)
ΔABC~ΔACD
⇒ACAD=ABAC⇒AC2=AB⋅BD. ....(iii)
Divinding, (i)by (ii), we get BC2AC2=BDAD
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7E30videos
TRIANGLES
- BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Multiple Choice Questions (Mcq)53videos
TRIANGLES
- BOOK - RS AGGARWALCHAPTER - TRIANGLESEXERCISE - Exercise 7C13videos
TRIANGLES
- BOOK - RS AGGARWALCHAPTER - T-RATIOS OF SOME PARTICULAR ANGLESEXERCISE - Exercise 1127videos
T-RATIOS OF SOME PARTICULAR ANGLES
- BOOK - RS AGGARWALCHAPTER - TRIGONOMETRIC IDENTITIESEXERCISE - Test Yourself20videos
TRIGONOMETRIC IDENTITIES
Similar Questions
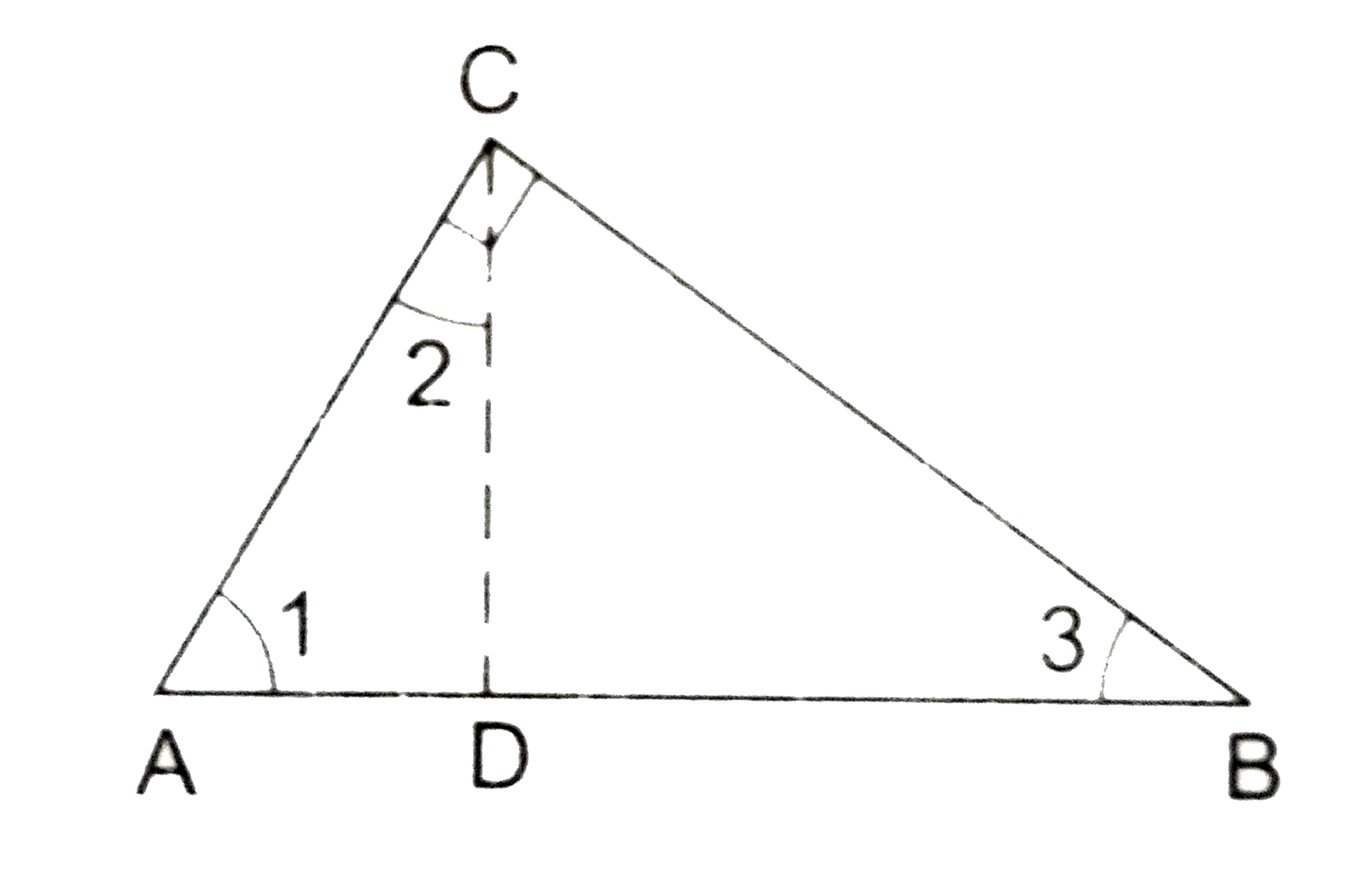
In the given figure, ∠ACB=90∘andCD⊥AB. Prove that CD2=BD⋅AD
 View Solution
View SolutionIn figure∠ACB=90∘and CD⊥AB. Prove that BC2AC2=BDAD.
View SolutionIn Fig. 4.134, ∠ACB=90o and CD⊥AB . Prove that CB2CA2=BDAD (FIGURE)
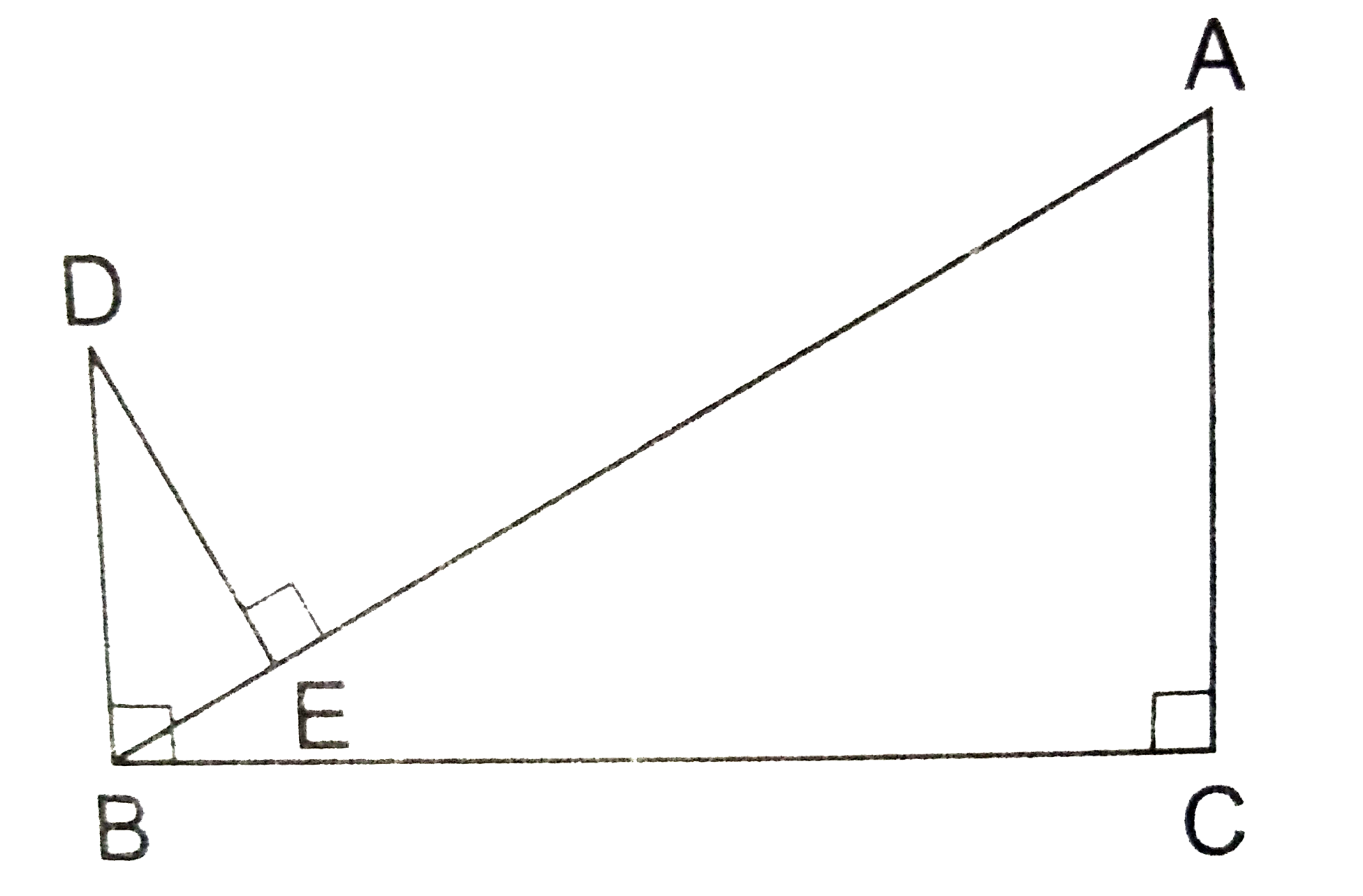
View SolutionIn the given figure, DB⊥BC,DE⊥ABandAC⊥BC.
Prove that BEDE=ACBC
 View Solution
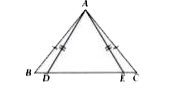
View SolutionIn the given figure, AB = AC and AD = AE. Prove that:
BD = CE
 View Solution
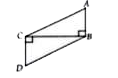
View SolutionIn the given figure, Ab = CD and ∠ABC=∠DCB=90∘. Prove that AC = DB.
 View Solution
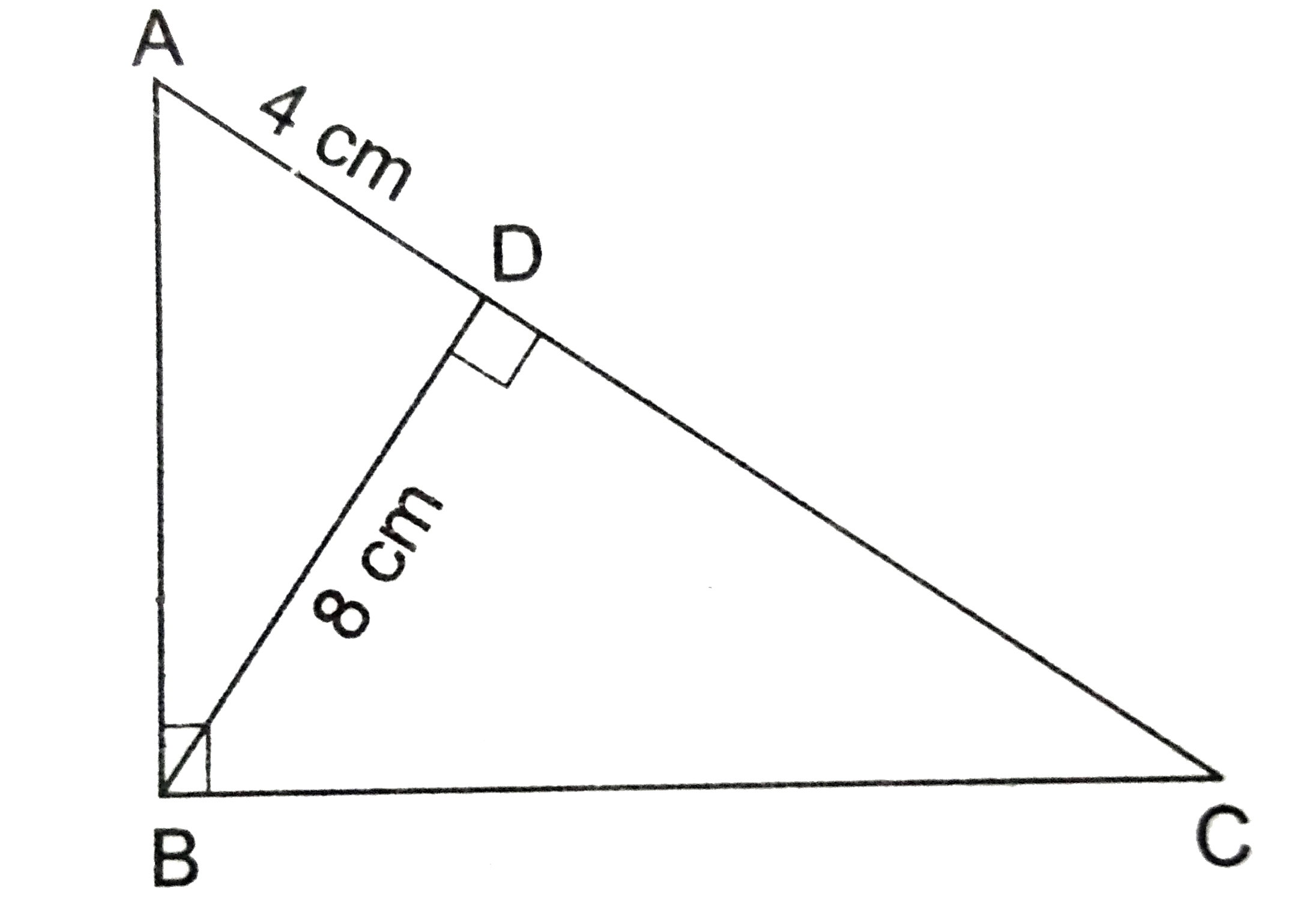
View SolutionIn the given figure, ∠ABC=90∘andBD⊥AC. If BD=8cm,AD=4cm find CD.
 View Solution
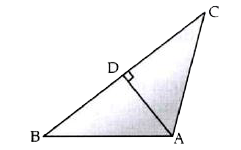
View SolutionIn the given figure if AD ⊥ BC prove that AB2+CD2=BD2+AC2
 View Solution
View SolutionIn figure, ∠B<90∘andAD⊥BC. Prove that AC2=AB2+BC2−2BC.BD
View SolutionSee the given figure. In ΔABC, seg AD⊥ seg BC. Prove that: AB2+CD2=BD2+AC2
 View Solution
View Solution

