Question

In the given figure, D is the midpoint of side BC and $AE\perp BC$. If $BC=a,AC=b,AB=c,ED=xAD=p\text{and}AE=h$ prove that
$\left(i\right)b^2=p^2+ax+\frac{a^2}{4}$
(ii) $c^2=p^2-ax+\frac{a^2}{4}$
(iii) $(b^2+c^2)=2p^2+\frac{1}{2}{a}^2$
$\left(iv\right)(b^2-c^2)=2ax$.

Explanation

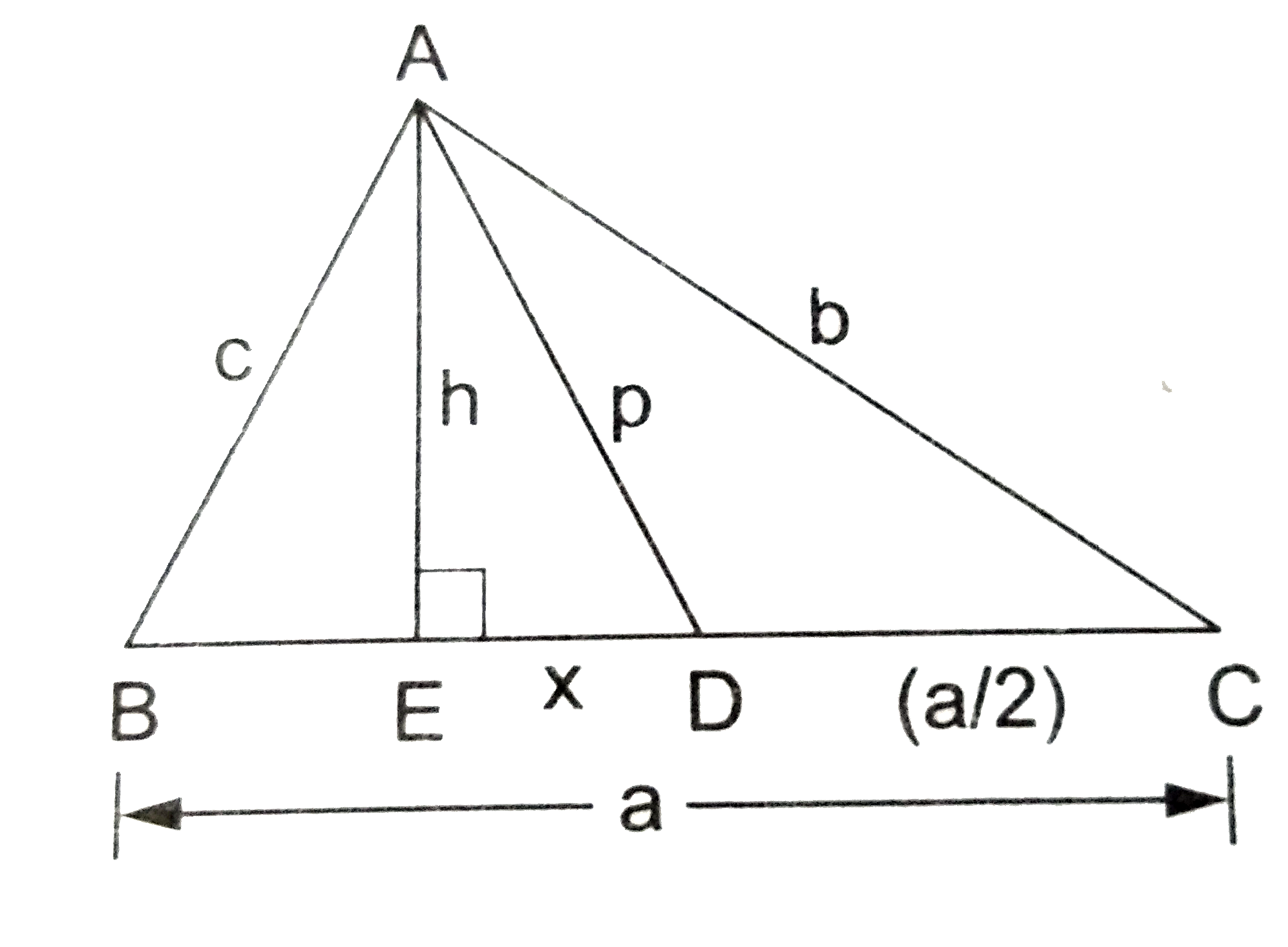
`(i) AC^(2)=AE^(2)+EC^(2)`
`rArr b^(2)=h^(2)+(x(a)/(3))^(2)=(h^(2)+x^(2))+ax+(a^(2))/(4)`
` =p^(2)-ax+(a^(2))/(4)`

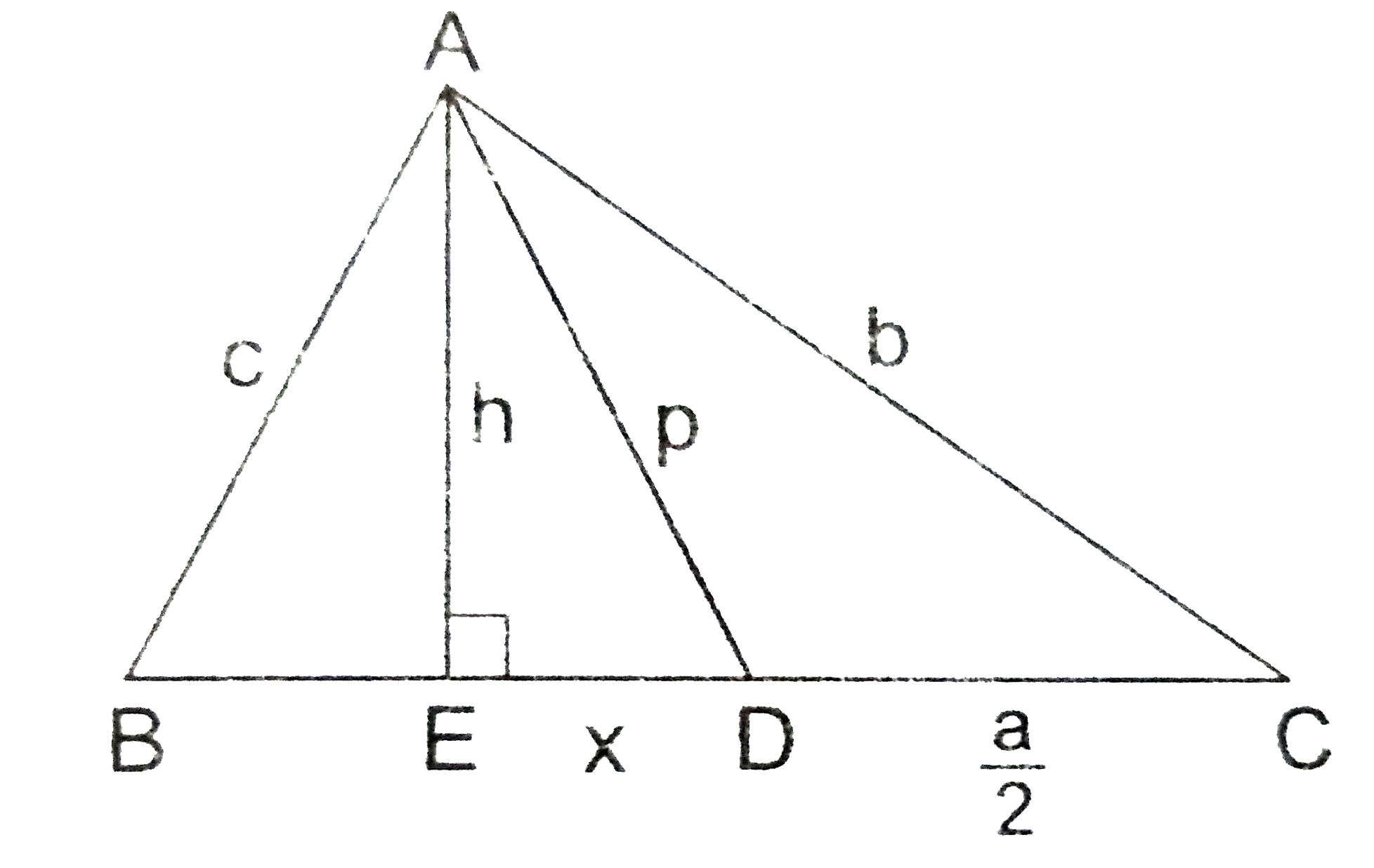
`(ii) AB^(2)=AE^(2)+BE^(2)`
`rArr c^(2)=h^(2)+((a)/(2)-x)^(2)=(h^(2)+x^(2))-ax+(a^(2))/(4)`
`=p^(2)-ax+(a^(2))/(4)`
(iii) and (i) and (ii).
(iv) Subtract (ii) from (ii).