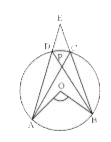
In the given figure, O is the centre of a circle and chords AC and BD intersect at E. If ∠AEB=110∘then find angle ∠ADB

A
70∘
B
60∘
C
80∘
D
90∘
Video Solution
Text Solution
The correct Answer is:C
∠AEB+∠CEB=180∘
⇒110∘+∠CEB=180∘⇒∠CEB=70∘
In ΔCEB,∠CEB+∠EBC+∠ECB=180∘
⇒∠70∘+30∘+∠ECB=180∘⇒∠ECB=80∘
∴∠ADB=∠ACB=∠ECB=80∘(∠S in the same segment).
|
Topper's Solved these Questions
- CIRCLES BOOK - RS AGGARWALCHAPTER - CIRCLES EXERCISE - Multiple Choice Questions (Mcq)
5 Videos
- BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGONBOOK - RS AGGARWALCHAPTER - BAR GRAPH, HISTOGRAM AND FREQUENCY POLYGONEXERCISE - EXERCISE 17B
16 Videos
- CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEBOOK - RS AGGARWALCHAPTER - CONGRUENCE OF TRIANGLES AND INEQUALITIES IN A TRIANGLEEXERCISE - Multiple Choice Questions (Mcq)
20 Videos
Similar Questions
Knowledge Check
In the given figure, O is the centre of the circle. The measure of ∠ ADB is
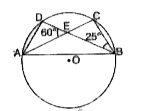 A90∘B85∘C95∘D120∘
A90∘B85∘C95∘D120∘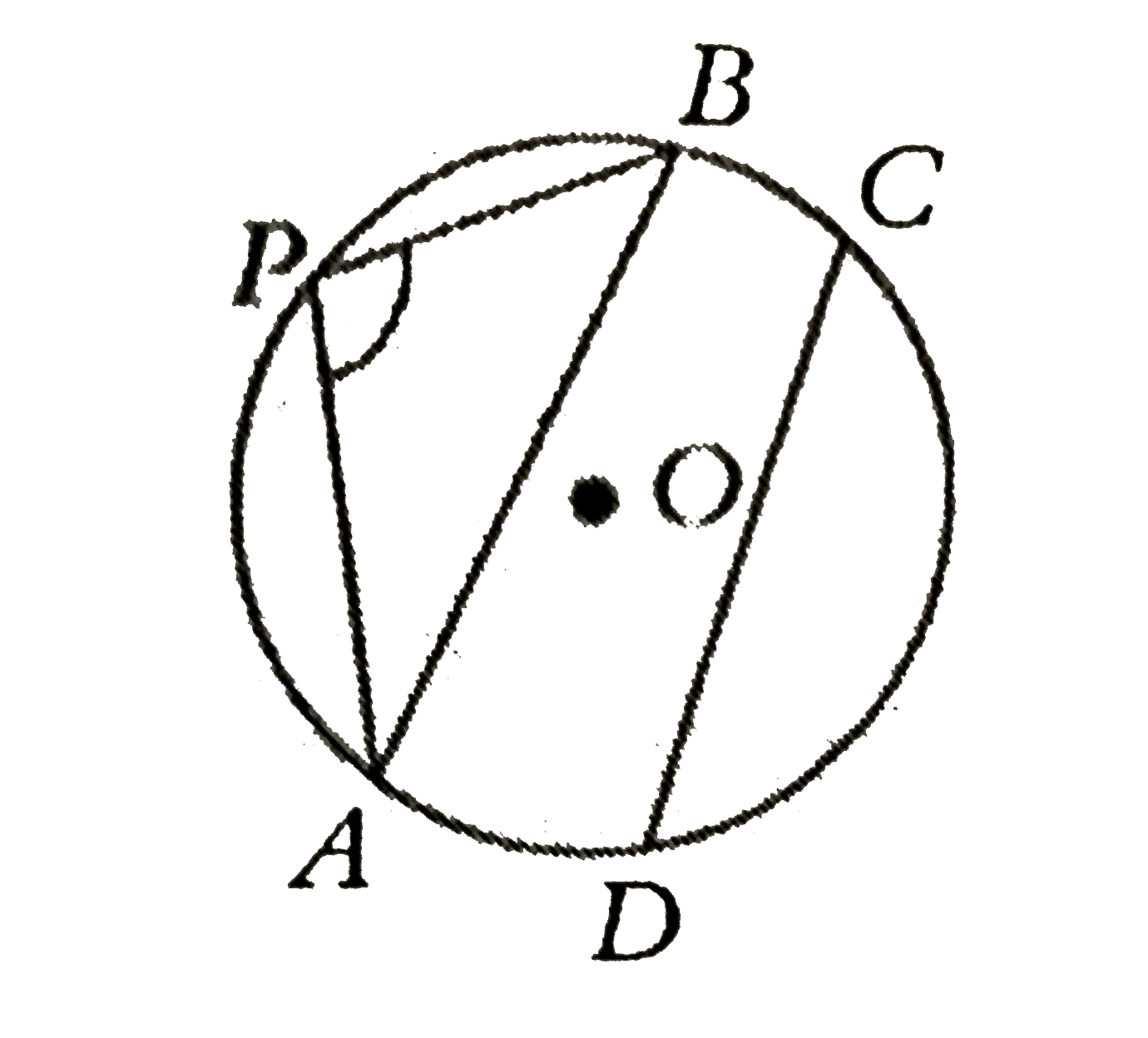
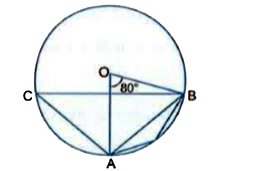
In the above figure, O is the centre of the circle and AB=CD. If ∠APB=110∘, then find the angle made by the chord CD at the centre.A220∘B110∘C120∘D140∘
‘O’ is the centre of the circle. Find ∠(ADB)
View SolutionIn the given figure, O is the centre of the circle. Its two chords AB and CD intersect each other at the point p within the circle. If AB=15cm,PB=9cm, then find the length of PD.

View SolutionIn the given figure, O is the centre of the circle, PA and PB are tangents to the circle then find ∠AQB.
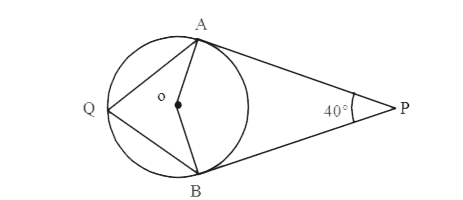
View Solution
In the above figure, O is the centre of the circle and AB=CD. If ∠APB=110∘, then find the angle made by the chord CD at the centre.
View SolutionIn given figure, O is the centre of the circle.
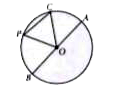
A chord, which is not the diameter of the circle is
View SolutionIn the given figure, O is the centre of the circle.

Name a chord, which is not the diameter of the circle.
View Solution