M is a point on the side BC of a parallelogram ABCD. DM when produced meets AB produced at N. Prove that
$(i) \frac{D M}{M N} = \frac{D C}{B N} (i i) \frac{D N}{D M} = \frac{A N}{D C}$

Video Solution

Text Solution

Verified by Experts

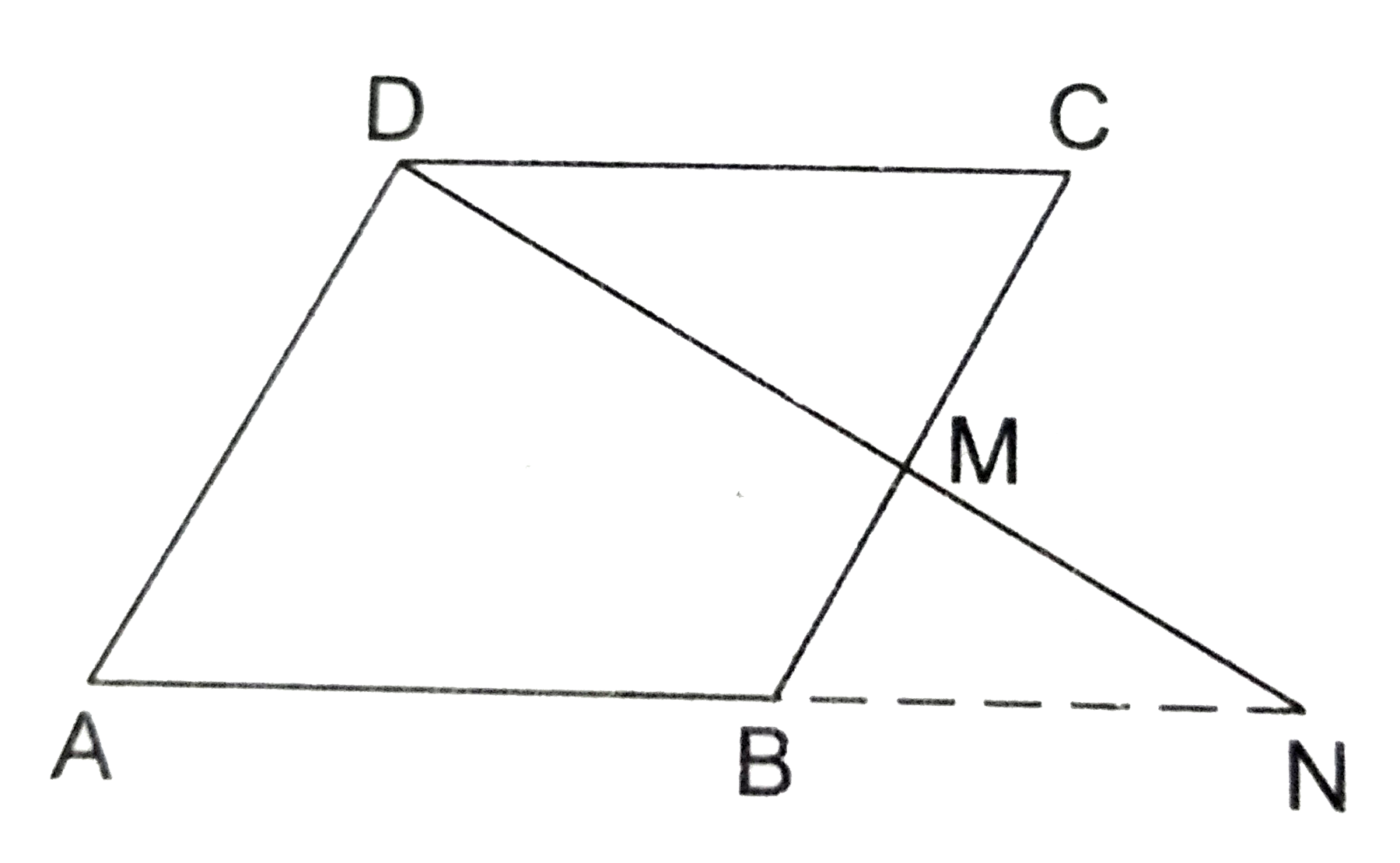
In $Δ N D A, M B ∣ ∣ D A$ ,
$\Rightarrow \frac{N M}{D M} = \frac{N B}{B A} \Rightarrow \frac{D M}{M N} = \frac{A B}{B N} \Rightarrow \frac{D M}{M N} = \frac{D C}{B N} [∴ A B = D C]$
(ii) $\Rightarrow \frac{N M}{D M} = \frac{N B}{B A} \Rightarrow \frac{N M}{M D} = + 1 = \frac{N B}{B A} + 1 \Rightarrow \frac{N M + M D}{M D} = \frac{N B + B A}{B A}$
$\frac{D N}{D M} = \frac{A N}{A B} \Rightarrow \frac{D N}{D M} = \frac{A N}{D C} [∴ A B = D C]$

|

Updated on:21/07/2023

Class 10 MATHS TRIANGLES

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
X and Y are the mid-points of the opposite sides AB and DC of a parallelogram ABCD. Then $□ A X C Y$ is a ?
ATrapezium
BKite
CRhombus
DParallelogram

RS AGGARWAL-TRIANGLES-Exercise 7A

Home
Ask Doubt
Profile