One diagonal of a square is along the line and one of its vertex is (1, 2). Then the equations of the sides of the square passing through this vertex are (d)none of theseSolution in Bengali
7x-8y+9=0, 8x+7y-22=0
9x-8y+7=0.8x+9y-26=0
23x-7y-9=0,7x+23y-53=0
none of these
The correct Answer is:C
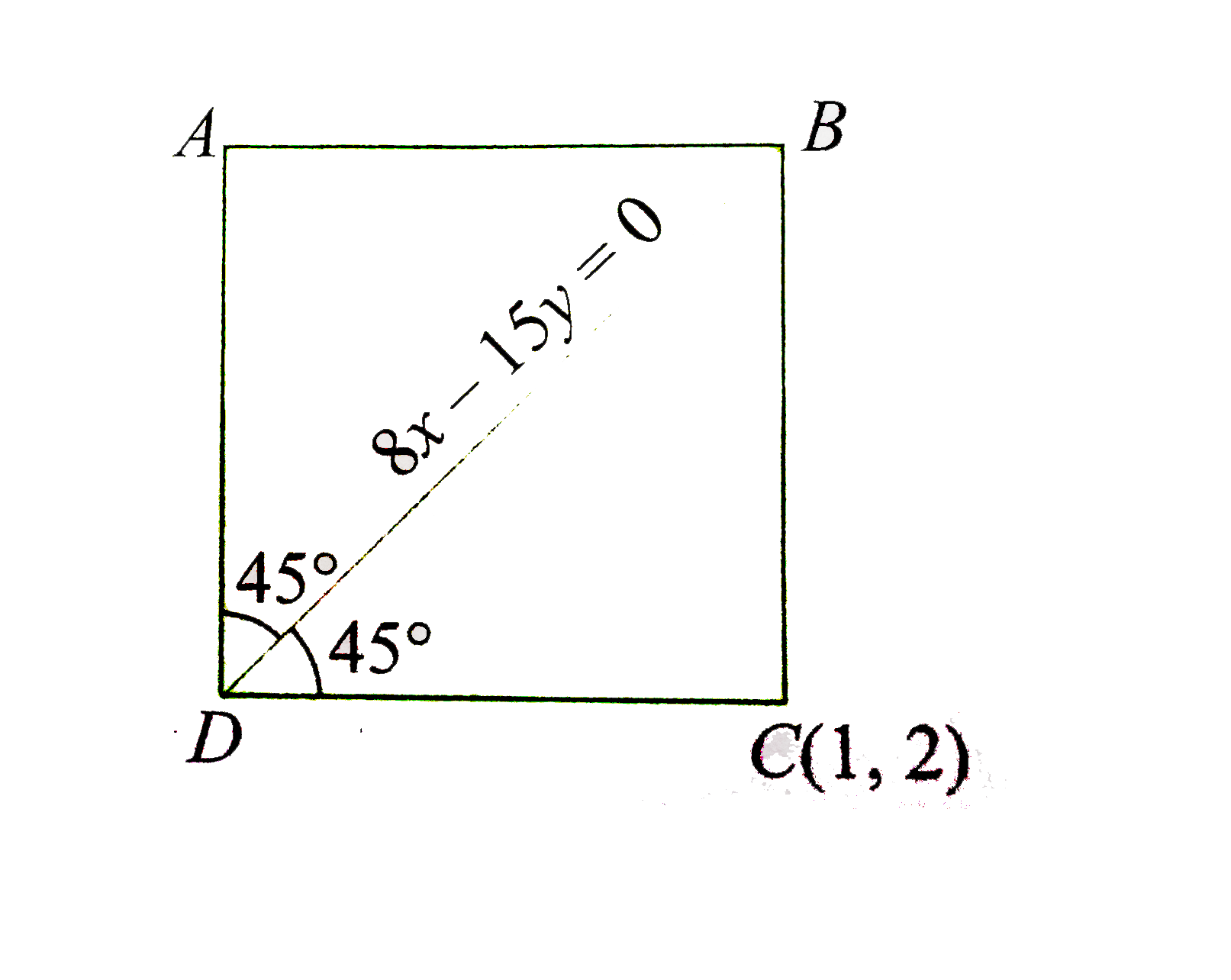
The slope of BD is 8/15 and the angle made by BD with DC and BC is . So, let the slope of DC be m. Then,
Hence, the equations of DC and BC are
or 23x-7y-9=0
or 7x+23y-53=0
Topper's Solved these Questions
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MULTIPLE CORRECT ANSWERS TYPE)30videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (LINKED COMPREHENSION TYPE)27videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINESEXERCISE - CONCEPT APPLICATION EXERCISE 2.65videos
STRAIGHT LINES
- BOOK - CENGAGE PUBLICATIONCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGE PUBLICATIONCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )1videos
THEORY OF EQUATIONS
Similar Questions
If P is a point (x,y) on the line y=−3x such that P and the point (3, 4) are on the opposite sides of the line 3x−4y=8, then x>815 (b) x>85 y<−85 (d) y<−815
02:15View SolutionLet l be the line belonging to the family of straight lines (a+2b)x+(a−3b)y+a−8b=0,a,b∈R, which is farthest from the point (2,2), then area enclosed by the line L and the coordinate axes is
03:51View SolutionIf aandb are two arbitrary constants, then prove that the straight line (a−2b)x+(a+3b)y+3a+4b=0 will pass through a fixed point. Find that point.
01:53View SolutionThe point P(α,α+1) will lie inside the triangle whose vertices are A(0,3),B(−2,0) and C(6,1) if
02:35View SolutionThe number of distinct real roots of x4−4x3+12x2+x−1=0is_____.
01:48View SolutionSolve the system of equation :11x+15y+23=0,7x-2y-20=0
03:26View SolutionOne diagonal of a square is along the line 8x−15y=0 and one of its vertex is (1, 2). Then the equations of the sides of the square passing through this vertex are 23x+7y=9,7x+23y=53 23x−7y+9=0,7x+23y+53=0 23x−7y−9=0,7x+23y−53=0 none of these
02:59View Solutionif (23^(9)-23^(8))/(22)=23^(x) then x=?
03:56View SolutionThe point (−4,5) is vertex of a square and one of its diagonal is 7x−y+8=0. The equation of other diagonal is 7x−y+23=0 7y+x+=30 7y+x=31 x−7y=30 x+7y+31=0
10:48View SolutionThe remainder when 23^(23) is divided by 53 is
03:08View SolutionSolve the given pair of equations:
23x−29y=98 ,
29x−23y=11005:52View Solutionसिद्ध कीजिए कि -
√3cos23∘−sin23∘=2cos53∘08:20View Solution23 23 29 53 113 ?
04:08View SolutionThe ratio in which the line x+y -1 =0 divides the line segment joining the origin and the point of intersection of the lines represented by
2x2−13xy−7y2+x+23y−6=0 is01:16View SolutionIf the lines 2x+3y+1=0 and 3x−y−4=0 lie along diameters of a circle of circumference 10π ,then the equation of the circle is (A) x2+y2−2x+2y−23=0 (B) x2+y2+2x−2y−23=0 (C) x2+y2+2x+2y−23=0 (D) x2+y2−2x−2y−23=0
01:34View SolutionOne diagonal of a square is along the line 8x−15y=0 and one of its vertex is (1, 2). Then the equations of the sides of the square passing through this vertex are (a)23x+7y=9,7x+23y=53 (b)23x−7y+9=0,7x+23y+53=0 (c)23x−7y−9=0,7x+23y−53=0 (d)none of these
05:43View Solution

