Prove that the perpendicular bisector of a chord of a circle always passes through the centre.
To prove: The perpendicular bisector of a chord of a circle always passes through the centre.
Proof:
Let us support does not pass through .
Since is perpendicular bisector of
................(1)
is the mid-point of .
passes through center from this, we can say that
i.e., ................(2)
Comparing 1 & 2, we can say
, this can be possible only when , and are on the same line.
It is a contradiction passes through .
Hence Proved.
Topper's Solved these Questions
Similar Questions
Consider the following statements :
1.The perpendicular bisector of chord of a circle does not pass through the centre of the circle.
2. The angle in a semicircle is a right angle.
Which of the statements given above is/are correct ?View SolutionTheorem :-2 The perpendicular from centre of a circle to the chord bisects the chord and Perpendicular bisectors of two chords of a circle intersects at the centre.
View Solution
Knowledge Check
- Question 1 - Select One
Consider the following statements
I. The perpendicular bisector of a chord of a circle does not pass through the centre of the circle.
II. The angle in a semicircle is a right angle.
Which of the statements given above is/are correct?AOnly IBOnly IICBoth I and IIDNeither I nor II - Question 2 - Select One
The chord of a circle which passes through the centre is___the radius
AThriceBDoubleCHalfDEqual to
Prove that the perpendicular at the point of contact to the tangent to a circle passes through the centre.
View SolutionProve that the right bisector of a chord of a circle, bisects the corresponding arc or the circle.
View SolutionProve that the right bisector of a chord of a circle, bisects the corresponding arc or the circle.
View SolutionProve that the line joining the mid-points of two parallel chords of a circle passes through the centre.
View SolutionIf the perpendicular bisector of a chord AB of a circle PXAQBY intersects the circle at P and Q, prove that arc PXA≅arc PYB.
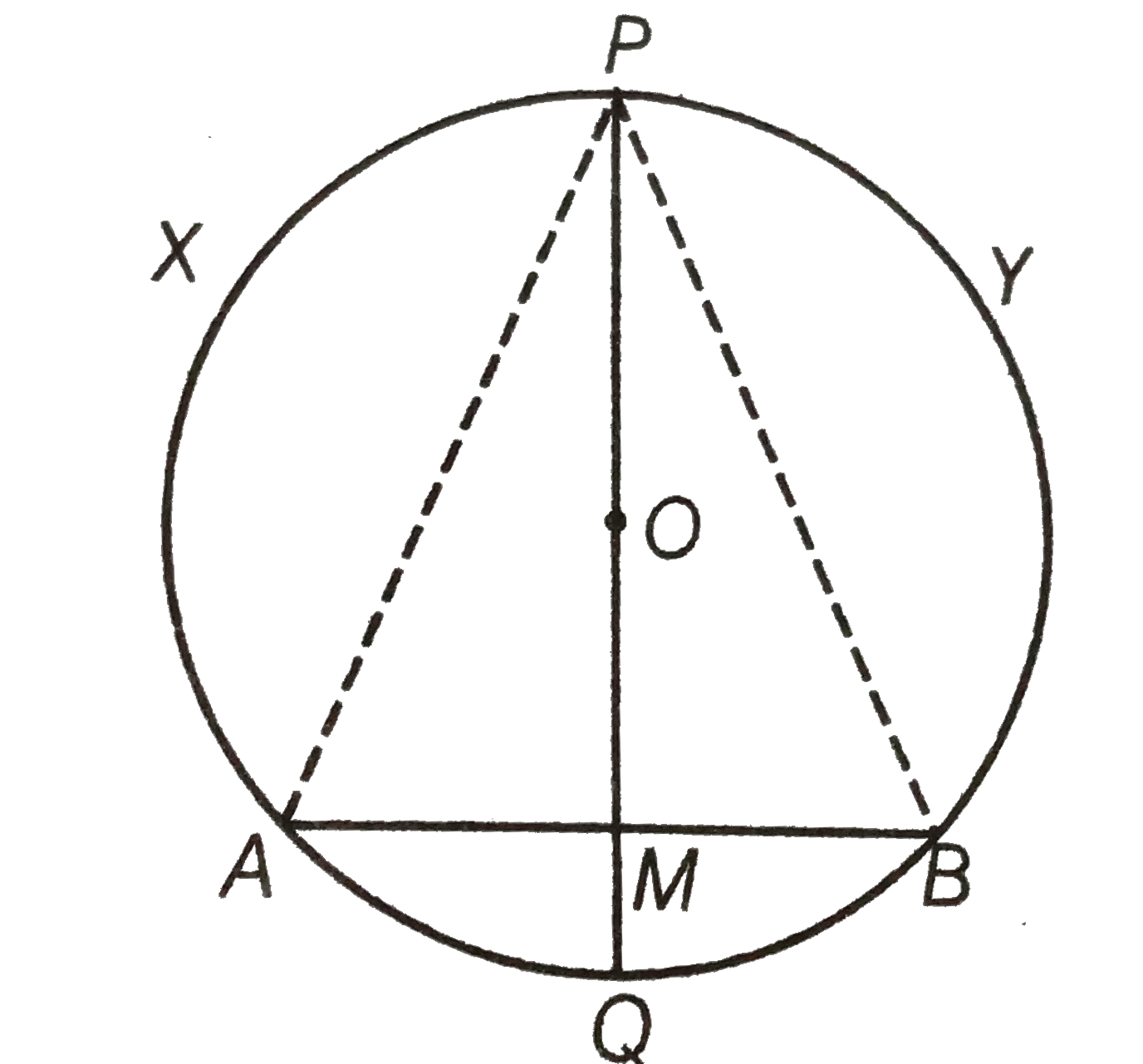 View Solution
View SolutionDraw a circle with centre C and radis 3.4 cm . Draw any chord ¯¯¯¯¯¯AB. Construct the perpendicular bisector of ¯¯¯¯¯¯AB. and examine if it passes through C.
View Solution

