Prove that the perpendicular bisectors of the sides of a cyclic quadrilateral are concurrent.
To prove that the perpendicular bisectors of the sides of a cyclic quadrilateral are concurrent, we can follow these steps:
Step 1: Define the Cyclic Quadrilateral
Let ABCD be a cyclic quadrilateral, which means that all its vertices lie on a circle.
Step 2: Identify the Perpendicular Bisectors
Draw the perpendicular bisectors of the sides AB, BC, CD, and DA. Let the perpendicular bisectors be denoted as follows:
- OL for side AB
- OM for side BC
- ON for side CD
- OP for side DA
Step 3: Use the Property of Perpendicular Bisectors
Recall the property of the perpendicular bisector of a chord in a circle: the perpendicular bisector of any chord passes through the center of the circle.
Step 4: Apply the Property to Each Side
Since ABCD is a cyclic quadrilateral, the following holds true:
- The perpendicular bisector OL of side AB passes through the center O of the circle.
- The perpendicular bisector OM of side BC also passes through the center O.
- The perpendicular bisector ON of side CD passes through the center O.
- The perpendicular bisector OP of side DA passes through the center O.
Step 5: Conclude that the Perpendicular Bisectors are Concurrent
Since all the perpendicular bisectors OL,OM,ON,OP pass through the same point O (the center of the circle), we conclude that the perpendicular bisectors of the sides of the cyclic quadrilateral are concurrent.
Final Statement
Thus, we have proved that the perpendicular bisectors of the sides of a cyclic quadrilateral are concurrent at the center of the circle circumscribing the quadrilateral.
---
Let ABCD be a cyclic quadrilateral, which means that all its vertices lie on a circle.
Draw the perpendicular bisectors of the sides AB, BC, CD, and DA. Let the perpendicular bisectors be denoted as follows:
- OL for side AB
- OM for side BC
- ON for side CD
- OP for side DA
Recall the property of the perpendicular bisector of a chord in a circle: the perpendicular bisector of any chord passes through the center of the circle.
Since ABCD is a cyclic quadrilateral, the following holds true:
- The perpendicular bisector OL of side AB passes through the center O of the circle.
- The perpendicular bisector OM of side BC also passes through the center O.
- The perpendicular bisector ON of side CD passes through the center O.
- The perpendicular bisector OP of side DA passes through the center O.
Since all the perpendicular bisectors OL,OM,ON,OP pass through the same point O (the center of the circle), we conclude that the perpendicular bisectors of the sides of the cyclic quadrilateral are concurrent.
Thus, we have proved that the perpendicular bisectors of the sides of a cyclic quadrilateral are concurrent at the center of the circle circumscribing the quadrilateral.
Topper's Solved these Questions
Similar Questions
Show that the perpendicular bisectors of the sides of a triangle are concurrent.
View SolutionDefinition of Cyclic quadrilateral
View SolutionProve that the perpendicular bisector of a chord of a circle always passes through the centre.
View SolutionProve that the internal bisectors of the angles of a triangle are concurrent
View SolutionThe opposite angles of a cyclic quadrilaterals are supplimentary
View SolutionThe number of triangles that can be formed by the perpendiculars bisectors of the sides of a triangle is
View SolutionProve that the internal bisectors of the angles of a triangle are concurrent
View SolutionProve that the sum of opposite pair of angles of a cyclic quadrilateral is 180∘
View SolutionA, B and C are three points on a circle. Prove that the perpendicular bisectors of AB, BC and CA are concurrent.
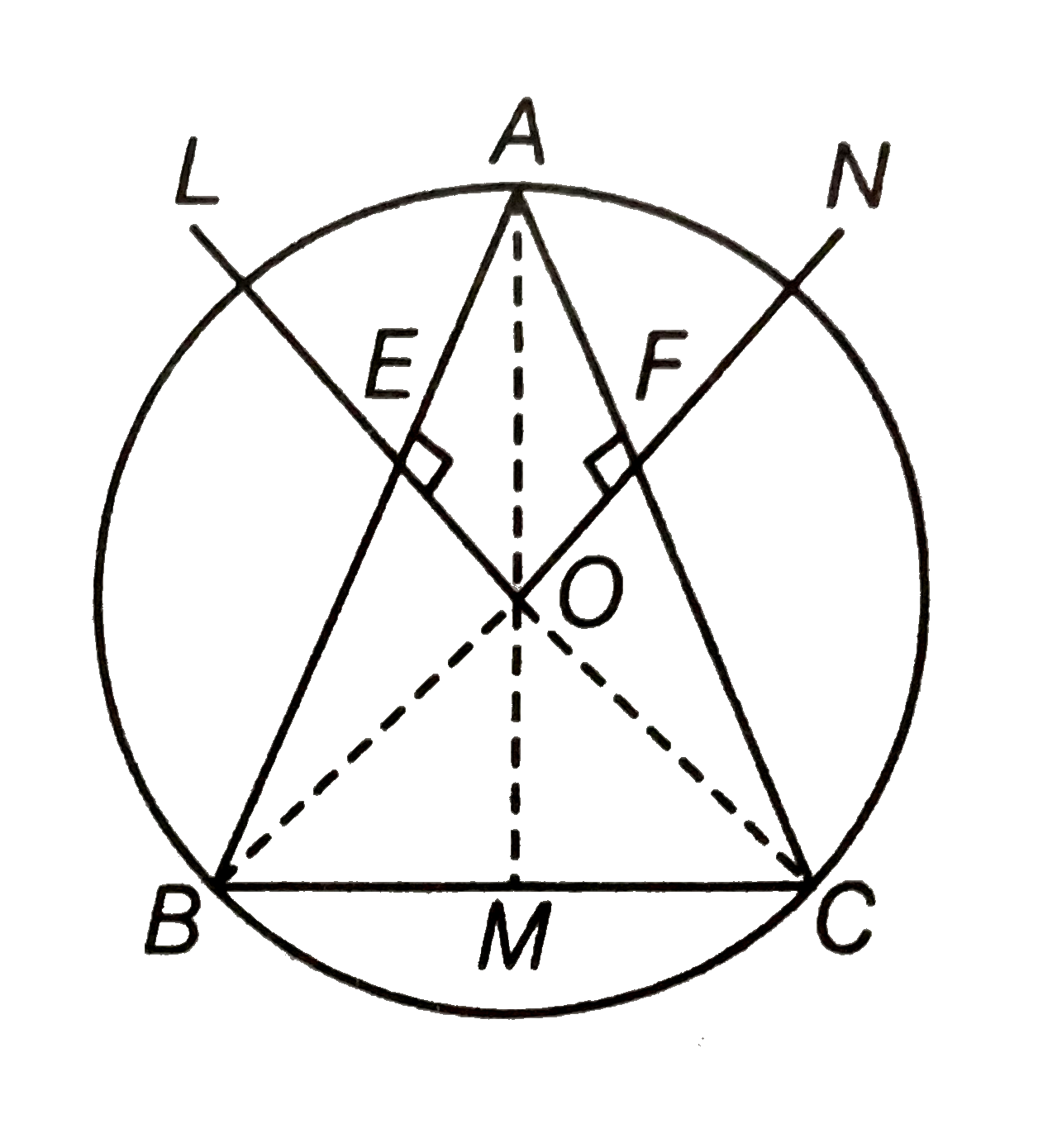 View Solution
View SolutionFrom a point O inside a triangle ABC, perpendiculars OD,OEandOf are drawn to rthe sides BC,CAandAB, respecrtively. Prove that the perpendiculars from A,B,andC to the sides EF,FDandDE are concurrent.
View Solution

