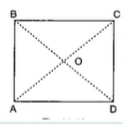
Show that if the diagonals of a quadrilateral are equal and bisect each other at right angles, then it is a square
Video Solution
Text Solution
From△AOBand△COD
AO=CO(Diagonals bisect each other)
∠AOB=∠COD(Vertically opposite)
OB=OD(Diagonals bisect each other)
△AOB≅△COD(SAS congruency)
Thus,AB=CD(CPCT) (i)
∠OAB=∠OCD(Alternate interior angles)
⇒AB∣∣CD
In △AODand△COD
AO=CO(Diagonals bisect each other)
∠AOD=∠COD(Vertically opposite)
OD=OD(Common)
△AOD≅△COD (SAS congruency)
AD=CD(CPCT)(ii)
AD=BCandAD=CD
⇒AD=BC=CD=AB(ii)
also,∠ADC=∠BCD(CPCT)
∠ADC+∠BCD180∘ (co-interior angles)
⇒2∠ADC=180∘
⇒∠ADC=90∘(iii)
One of the interior angles is right angle.
Thus, from (i), (ii) and (iii) given quadrilateral ABCD is a square.
|
Updated on:21/07/2023
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
If the diagonals of a quadrilateral are equal and bisect each other at right angles, then the quadrilateral is a
AreactangleBsquareCrhombusDtrapezium
Show that the diagonals of a square are equal and bisect each other at right angles.
View SolutionShow that the diagonals of a square are equal and bisect each other at right angles.
View SolutionThe diagonals of a rectangle are equal and bisect each other
View SolutionIf the diagonals of a quadrilateral are equal and bisect each other, then the quadrilateral is a
View SolutionThe diagonals of a rhombus are unequal and bisect each other at right angle
View SolutionShow that if the diagonals of a quadrilateral bisect each other at right angles, then it is a rhombus
View SolutionShow that if the diagonals of a quadrilateral bisect each other at right angles, then it is a rhombus.
View Solution