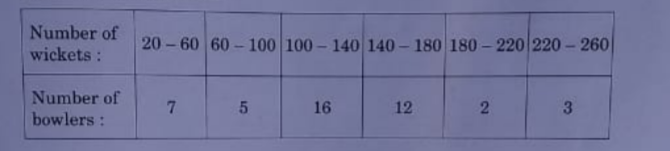
The distribution below shows the number of wickets taken by bowlers in one-day cricket matches. Find the mean number of wickets by choosing a suitable method. What does the mean signify?
To find the mean number of wickets taken by bowlers in one-day cricket matches using the step deviation method, we will follow these steps:
Step 1: Organize the Data
We need to organize the data into a frequency distribution table. The intervals and the corresponding frequencies (number of bowlers) are as follows:
| Wickets Interval | Frequency (f) |
|-------------------|---------------|
| 20 - 60 | 20 |
| 60 - 100 | 16 |
| 100 - 150 | 12 |
| 150 - 200 | 2 |
| 200 - 250 | 3 |
Step 2: Calculate the Midpoint (xi) for Each Interval
The midpoint (xi) for each interval is calculated as follows:
- For 20 - 60: x1=20+602=40
- For 60 - 100: x2=60+1002=80
- For 100 - 150: x3=100+1502=125
- For 150 - 200: x4=150+2002=175
- For 200 - 250: x5=200+2502=225
Step 3: Calculate the Assumed Mean (A)
The assumed mean (A) can be taken as the midpoint of the data. Here, we can take the midpoint of the midpoints calculated above. Since the data is not evenly distributed, we can choose a value around the middle of the intervals. Let's take A=125.
Step 4: Calculate the Deviation (di) for Each Midpoint
The deviation (di) is calculated as follows:
- d1=x1−A=40−125=−85
- d2=x2−A=80−125=−45
- d3=x3−A=125−125=0
- d4=x4−A=175−125=50
- d5=x5−A=225−125=100
Step 5: Calculate the Step Deviation (ui)
The step deviation (ui) is calculated by dividing the deviation (di) by the class width (h). Here, the class width (h) is 60−20=40.
- u1=d1h=−8540=−2.125
- u2=d2h=−4540=−1.125
- u3=d3h=040=0
- u4=d4h=5040=1.25
- u5=d5h=10040=2.5
Step 6: Calculate fi * ui for Each Interval
Now we calculate fi⋅ui:
| Frequency (fi) | ui | fi * ui |
|----------------|----------|-----------|
| 20 | -2.125 | -42.5 |
| 16 | -1.125 | -18 |
| 12 | 0 | 0 |
| 2 | 1.25 | 2.5 |
| 3 | 2.5 | 7.5 |
Step 7: Calculate the Summation of fi and fi * ui
Now we sum up the frequencies and the products:
- Total fi=20+16+12+2+3=53
- Total fi⋅ui=−42.5−18+0+2.5+7.5=−51.5
Step 8: Calculate the Mean
Using the formula for the mean in the step deviation method:
Mean=A+(∑fiui∑fi⋅h)
Substituting the values:
Mean=125+(−51.553⋅40)
Calculating:
Mean=125−38.8≈86.2
Conclusion
The mean number of wickets taken by bowlers in one-day cricket matches is approximately 86.2.
What Does the Mean Signify?
The mean signifies the average number of wickets taken by the bowlers in the given matches. It provides a central value that represents the overall performance of the bowlers in terms of wickets taken.
We need to organize the data into a frequency distribution table. The intervals and the corresponding frequencies (number of bowlers) are as follows:
|-------------------|---------------|
| 20 - 60 | 20 |
| 60 - 100 | 16 |
| 100 - 150 | 12 |
| 150 - 200 | 2 |
| 200 - 250 | 3 |
The midpoint (xi) for each interval is calculated as follows:
- For 60 - 100: x2=60+1002=80
- For 100 - 150: x3=100+1502=125
- For 150 - 200: x4=150+2002=175
- For 200 - 250: x5=200+2502=225
The assumed mean (A) can be taken as the midpoint of the data. Here, we can take the midpoint of the midpoints calculated above. Since the data is not evenly distributed, we can choose a value around the middle of the intervals. Let's take A=125.
The deviation (di) is calculated as follows:
- d2=x2−A=80−125=−45
- d3=x3−A=125−125=0
- d4=x4−A=175−125=50
- d5=x5−A=225−125=100
The step deviation (ui) is calculated by dividing the deviation (di) by the class width (h). Here, the class width (h) is 60−20=40.
- u2=d2h=−4540=−1.125
- u3=d3h=040=0
- u4=d4h=5040=1.25
- u5=d5h=10040=2.5
Now we calculate fi⋅ui:
|----------------|----------|-----------|
| 20 | -2.125 | -42.5 |
| 16 | -1.125 | -18 |
| 12 | 0 | 0 |
| 2 | 1.25 | 2.5 |
| 3 | 2.5 | 7.5 |
Now we sum up the frequencies and the products:
- Total fi⋅ui=−42.5−18+0+2.5+7.5=−51.5
Using the formula for the mean in the step deviation method:
The mean number of wickets taken by bowlers in one-day cricket matches is approximately 86.2.
The mean signifies the average number of wickets taken by the bowlers in the given matches. It provides a central value that represents the overall performance of the bowlers in terms of wickets taken.
Topper's Solved these Questions
- STATISTICSBOOK - NCERT ENGLISHCHAPTER - STATISTICSEXERCISE - EXERCISE 14.4
3 Videos
- STATISTICSBOOK - NCERT ENGLISHCHAPTER - STATISTICSEXERCISE - Exercise 14.3
7 Videos
- SOME APPLICATIONS OF TRIGONOMETRYBOOK - NCERT ENGLISHCHAPTER - SOME APPLICATIONS OF TRIGONOMETRYEXERCISE - SOLVED EXAMPLES
7 Videos
- SURFACE AREAS AND VOLUMESBOOK - NCERT ENGLISHCHAPTER - SURFACE AREAS AND VOLUMESEXERCISE - Exercise 13.3
9 Videos
Similar Questions
The distribution given below shows the number of wickets taken by bowlers in one-day cricket matches . Find the mean and the median of the number of wickets taken

View SolutionThe distribution given below shows the number of wickets taken by bowlers in one-day cricket matches. find the mean and the median of the number of wickets taken
View Solutionthe distribution given below shows the number of wickets taken by bowlers in one-day cricket matches. find the mean and the median of the number of wickets taken
View SolutionThe given distribution shows the number of runs scored by some top batsmen of the world in one-day international cricket matches.

Find the mode of the data.
View SolutionThe given distribution shows the number of runs scored by some top batsmen of the world in one-day international cricket matches.Find the mode of the data.
View SolutionThe wickets taken by a bowler in 10 cricket matches are as follows:2 6 4 5 0 2 1 3 2 3 Find the mode of the data.
View SolutionThe table below shows the daily expenditure on food of 25 households in a locality.Find the mean daily expenditure on food by a suitable method.
View SolutionThe following table gives the number of pages written by Saria for completing her own book for 30 days.
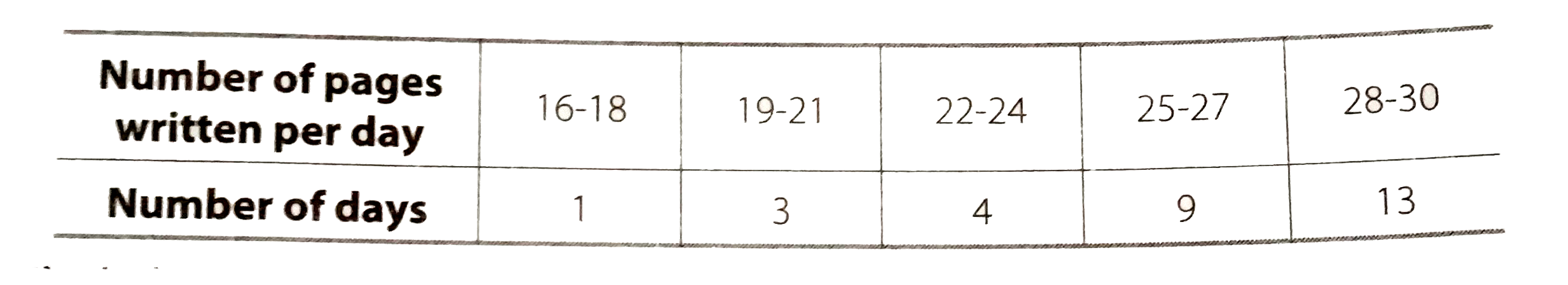
Find the mean number of pages written per day.
View SolutionIn a cricket match Anil took one wicket less than twice the number of wickets taken by Ravi. If the product of the number of wickets taken by them is 15, find the number of wickets taken by each of them.
View SolutionIn a retail market, fruit vendors were selling mangoes kept in packing boxes. These boxes contained varying number of mangoes. The following was the distribution of mangoes according to the number of boxes.Find the mean number of mangoes kept in a packing box. Which method of finding the mean did you choose?
View Solution
