TP and TQ are any two tangents to a parabola and the tangent at a third point R cuts them in P' and Q'. Prove that TP'TP+TQ'TQ=1Solution in Tamil
1
2
3
none of these
The correct Answer is:A
Let parabola be y2=4ax and co-ordinates of P and Q on this parabola be P≡(at21,2at1) and Q≡(at22,2at2),
T is the point of intersection of tangents at t1 and t2. Co-ordinates of
T≡{at1t2,a(t1+t2)}
P'≡{at1t3,a(t1+t3)}
Q'≡(at2t3,a(t2+t3)}
Let TP':TP=λ:1
∴λ=t3−t2t1−t2
⇒TP'TP=t3−t2t1−t2
Similarly, TQ'TQ=t1−t3t1−t2,TP'TP+TQ'TQ=1.
Topper's Solved these Questions
- PARABOLABOOK - CENGAGECHAPTER - PARABOLAEXERCISE - Multiple Correct Answers Type
10 Videos
- PARABOLABOOK - CENGAGECHAPTER - PARABOLAEXERCISE - Comprehension Type
1 Videos
- PARABOLABOOK - CENGAGECHAPTER - PARABOLAEXERCISE - JEE Advenced Single Answer Type
18 Videos
- PAIR OF STRAIGHT LINESBOOK - CENGAGECHAPTER - PAIR OF STRAIGHT LINESEXERCISE - Exercise (Numerical)
5 Videos
- PERMUTATION AND COMBINATIONBOOK - CENGAGECHAPTER - PERMUTATION AND COMBINATIONEXERCISE - Question Bank
4 Videos
Similar Questions
A family of curve S is given by S≡x2+2xy+y2−4x(1−λ)−4y(1+λ)+4, then S=0 represents
View SolutionA parabola is drawn through two given points A(1,0) and B(−1,0) such that its directrix always touches the circle x² + y^2 = 4. Then, The locus of focus of the parabola is=
View Solutionabout to only mathematics
View SolutionThe distance between the lines (x+7y)2+4√2(x+7y)−42=0 is_____________
View SolutionIf the number of ways in which a selection of 100 balls can be made out of 100 identical red balls, 100 identical blue balls and 100 identical white balls is
View SolutionTP&TQ are tangents to the parabola,y^(2)=4ax at P and Q. If the chord passes through the point (-a,b) then the locus of T is
View SolutionTwo tangents TP and TQ are drawn to a circle with centre O from an external point T . Prove that ∠PTQ=2∠OPQ .
View SolutionTP and TQ are any two tangents to a parabola and the tangent at a third point R cuts them in P' and Q'. Prove that (TP')/(TP)+(TQ')/(TQ)=1
View Solutionबिंदु T से वृत्त C(O,r) पर दो स्पर्श रेखाएँ TP और TQ खींची गई हैं । सिद्ध करो कि ∠PTQ=2∠OPQ
View SolutionTwo tangents TP and TQ are drawn to a circle with centre O from an external point T . Prove that ∠PTQ=2∠OPQ
View SolutionO केन्द्र के वृत्त में बाह्य बिन्दु T से दो स्पर्श रेखायें TP और TQ खींची गई है। सिद्ध कीजिए कि ∠PTQ=2∠OPQ
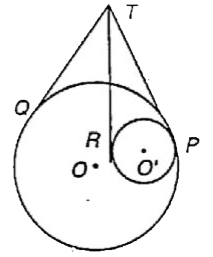
View SolutionIn the given figure, there are two circles with the centres O and O' touching each other internally at P. Q Tangents TQ and TP are drawn to the larger circle and tangents TP and TR are drawn to the smaller circle. Find TQ:TR :
View SolutionIf TP and TQ are the two tangents to a circle with centre O so that ∠POQ=110∘, then ∠PTQ is equal to
View SolutionTwo tangents TP and TQ are drawn to a circle with centre O from an external point T. Prove that ⌊PTQ=2⌊OPQ.
View SolutionTwo tangents TP and TQ are drawn to a circle with centre O from an external point T. Prove that ∠PTQ=2∠OPQ.
View Solution
