Two chords AB and CD of a circle are parallel and a line l is the perpendicular bisector of AB. Show that l bisects CD
To prove that the line l, which is the perpendicular bisector of chord AB, also bisects the parallel chord CD, we can follow these steps:
1. Understanding the Setup:
- Let O be the center of the circle.
- Chords AB and CD are parallel.
- Line l is the perpendicular bisector of chord AB.
2. Properties of Perpendicular Bisector:
- Since l is the perpendicular bisector of AB, it means that it intersects AB at its midpoint, say point E, and OE is perpendicular to AB.
3. Using the Property of Parallel Lines:
- Since AB is parallel to CD, the angles formed by the perpendicular line OE with the chords will be equal. Specifically, ∠OEA=∠OFC where F is the point where line l intersects chord CD.
4. Angle Relationships:
- We know that ∠OEA=90∘ (because l is perpendicular to AB).
- Since AB∥CD, by the Alternate Interior Angles Theorem, ∠OEA=∠OFC. Thus, ∠OFC=90∘ as well.
5. Conclusion from the Properties:
- Since OF is perpendicular to CD from the center O, it follows that OF bisects the chord CD.
- Therefore, we can conclude that CF=FD, which means line l bisects chord CD.
Final Statement
Thus, we have shown that the line l, which is the perpendicular bisector of chord AB, also bisects the parallel chord CD.
- Let O be the center of the circle.
- Chords AB and CD are parallel.
- Line l is the perpendicular bisector of chord AB.
- Since l is the perpendicular bisector of AB, it means that it intersects AB at its midpoint, say point E, and OE is perpendicular to AB.
- Since AB is parallel to CD, the angles formed by the perpendicular line OE with the chords will be equal. Specifically, ∠OEA=∠OFC where F is the point where line l intersects chord CD.
- We know that ∠OEA=90∘ (because l is perpendicular to AB).
- Since AB∥CD, by the Alternate Interior Angles Theorem, ∠OEA=∠OFC. Thus, ∠OFC=90∘ as well.
- Since OF is perpendicular to CD from the center O, it follows that OF bisects the chord CD.
- Therefore, we can conclude that CF=FD, which means line l bisects chord CD.
Thus, we have shown that the line l, which is the perpendicular bisector of chord AB, also bisects the parallel chord CD.
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Two particles each of mass 'm' are placed at A and C are such AB=BC=L. The gravitational force on the third particle placed at D at a distance L on the perpendicular bisector of the line AC is
AGm2L2 along BDBGm2√2L2 along DBCGm2L2 along ACDGm2√2L2 along BD - Question 2 - Select One
Observe the following facts for a parabola.
(i) Axis of the parabola is the only line which can be the perpendicular bisector of the two chords of the parabola.
(ii) If AB and CD are two parallel chords of the parabola and the normals at A and B intersect at P and the normals at C and D intersect at Q, then PQ is a normal to the parabola.
For the parabola y2=4x, AB and CD are any two parallel chords having slope 1. C1 is a circle passing through O, A and B and C2 is a circle passing through O, C and D. C1andC2 intersect atA(4, -4)B(-4, 4)C(4, 4)D(-4, -4) - Question 3 - Select One
Observe the following facts for a parabola.
(i) Axis of the parabola is the only line which can be the perpendicular bisector of the two chords of the parabola.
(ii) If AB and CD are two parallel chords of the parabola and the normals at A and B intersect at P and the normals at C and D intersect at Q, then PQ is a normal to the parabola.
The directrix of the parabola isAy−124=0By+124=0Cy+112=0Dy−112=0
AB is a line segment and line l is its perpendicular bisector. If a point P lies on l, show that P is equidistant from A and B.
View SolutionTheorem :-2 The perpendicular from centre of a circle to the chord bisects the chord and Perpendicular bisectors of two chords of a circle intersects at the centre.
View SolutionIn Figure, BM and DN are both perpendiculars to the segments AC and BM=DN . Prove that AC bisects BD
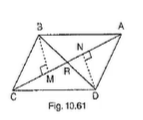 View Solution
View SolutionThe sides AB and CD of a parallelogram ABCD are bisected at E and F. Prove that EBFD is a parallelogram.
View SolutionStudy the diagram. The line l is perpendicular to line m (a) Is CE=EG (b) Does PE bisect CG = (c) Identify any two line segments for which PE is the perpendicular bisector. (d) Are these true? (i) AC>EG (ii) CD=GH (iii) BC<EH
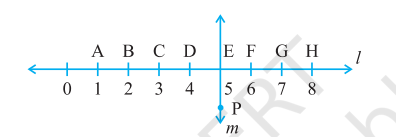 View Solution
View Solution

