Two circles of radii 4cm and 1cm touch each other externally and θ is the angle contained by their direct common tangents. Find sin(θ2)+cos(θ2).Solution in Bengali
The correct Answer is:7/5
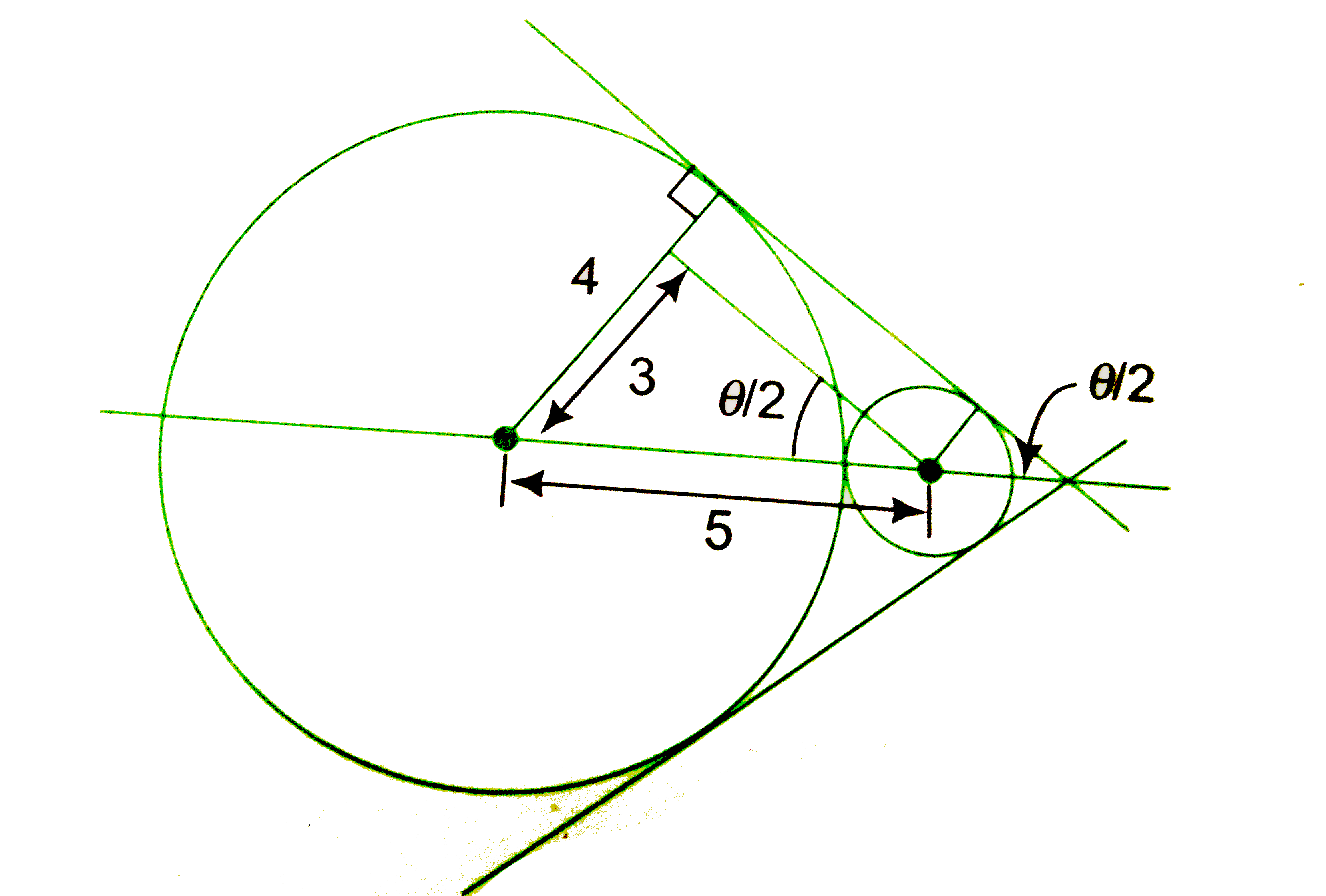
From figure, we have
sin(θ)2=35
cos(θ)2=45
∴Sin(θ)2+cos(θ)2=75
Topper's Solved these Questions
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Concept Application Exercises 2.2
8 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - Concept Application Exercises 2.3
4 Videos
- TRIGONOMETRIC FUNCTIONS BOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC FUNCTIONS EXERCISE - example
10 Videos
- TRIGONOMETRIC EQUATIONSBOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Numerical value type)
4 Videos
- TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASBOOK - CENGAGE PUBLICATIONCHAPTER - TRIGONOMETRIC RATIOS AND TRANSFORMATION FORMULASEXERCISE - Archives (Numerical Value Type)
2 Videos
Similar Questions
Prove that sinx−cosx+1sinx+cosx−1=secx+tanx
View SolutionA horse is tied to a post by a rope. If the horse moves along a circular path always keeping the rope tight, and describes 88 metres when it traces 72∘ at the centre, find the length of the rope.
View SolutionIf 2cosx+sinx=1, then find the valie of 7x+6sinx.
View SolutionThe number of all possible values of θ, where 0<θ<π, for which the system of equations (y+z)cos3θ=(xyz)sin3θ,xsin3θ=2cos3θy+2sin3θzand(xyz)sin3θ=(y+2z)cos3θ+ysin3θ have a solution (x0,y0,z0) wiith y0z0≠0 is
View SolutionThe maximum value of the expression 1sin2θ+3sinθcosθ+5cos2θ is………
View SolutionTwo circles with radii a and b touch each other externally such that theta is the angle between their direct common tangent (a>b>=2), then
View SolutionTwo circles of radii a and b touch each other externally and theta be the angle between their direct common tangents (a>b>=2) then
View SolutionTwo circles of radii 4cm and 1cm touch each other externally and θ is the angle contained by their direct common tangents. Find sinθ2+cosθ2.
View SolutionTwo circles with radii aandb touch each other externally such that θ is the angle between the direct common tangents, (a>b≥2) . Then prove that θ=2sin−1(a−ba+b) .
View SolutionTwo circles of radii 4cm and 1cm touch each other externally and θ is the angle contained by their direct common tangents. Then sinθ is equal to 2425 (b) 1225 34 (d) none of these
View SolutionIf two circles of radius 4cm and 1cm touch each other externally then length of the direct common tangents is
View SolutionTwo circles of radii 5cm and 2cm touch each other externally and θ is the angle contained by their direct common tangents.Then sinθ is equal to 12√10λ where λ equals
View SolutionTwo circles of radii 4cm and 1cm touch each other externally and θ is the angle contained by their direct common tangents. Find sin(θ2)+cos(θ2).
View SolutionTwo circles with radii aandb touch each other externally such that θ is the angle between the direct common tangents, (a>b≥2) . Then prove that θ=2sin−1(a−ba+b) .
View SolutionTwo circles with radii aandb touch each other externally such that θ is the angle between the direct common tangents, (a>b≥2) . Then prove that θ=2sin−1(a−ba+b) .
View SolutionTwo circles of radii 4cm and 1cm touch each other externally and θ is the angle contained by their direct common tangents. Then sinθ is equal to (a)2425 (b) 1225 (c)34 (d) none of these
View Solution