Two circles whose centres are O and O' intersect at P. Through P, a line l parallel to OO' intersecting the circles at C and D is drawn. Prove that CD=2 OO'
Draw perpendiculars O'Aand O'B'→CD \from\ Oand O',respectively.
Since OA⊥CD,OA bisects the chord CP. (Perpendicular from the centre of the chord bisects the chord)
AP=12CP,CP=2A- - - - - (i)
Similarly since O'B⊥PD, we must have BP=12PDorPD=2BP- - - - - (ii)
Now, CD=CP+DP
⇒CD=2AP+2BP=2(AP+BP)(from (i) and (ii))
⇒CD=2(AB)- - - - - (iii)
In quadrilateral ABO'O,OO'∥ABand ∠OAB=∠O'BA=900
Thus, ABO'O is a rectangle.
AB=OO',Hence CD=2AB=2OO'
Topper's Solved these Questions
Similar Questions
Knowledge Check
- Question 1 - Select One
Two circles of same radius r centred at O and O' touch each other at P as shown in the figure, if OO' is produced to meet the circle C(O'r) at A and AT is a tangent to the circle C(O,r) such that O'Q ⊥ AT . Then AO : AO'=
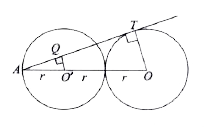 A32B2C3D14
A32B2C3D14
The diagonals of a parallelogram ABCD intersect at OA line through O intersects AB at XandDC at Y Prove that OX=OY
View SolutionThe diagonals of a parallelogram ABCD intersect at O.A line through O intersects AB at X and DC at Y. Prove that OX=OY.
View SolutionSeg AB is a diameter of a circle with centre P. Seg AC is a chord. A secant through P and parallel to seg AC intersects the tangent drawn at C in D. Prove that line DB is a tangent to the circle.
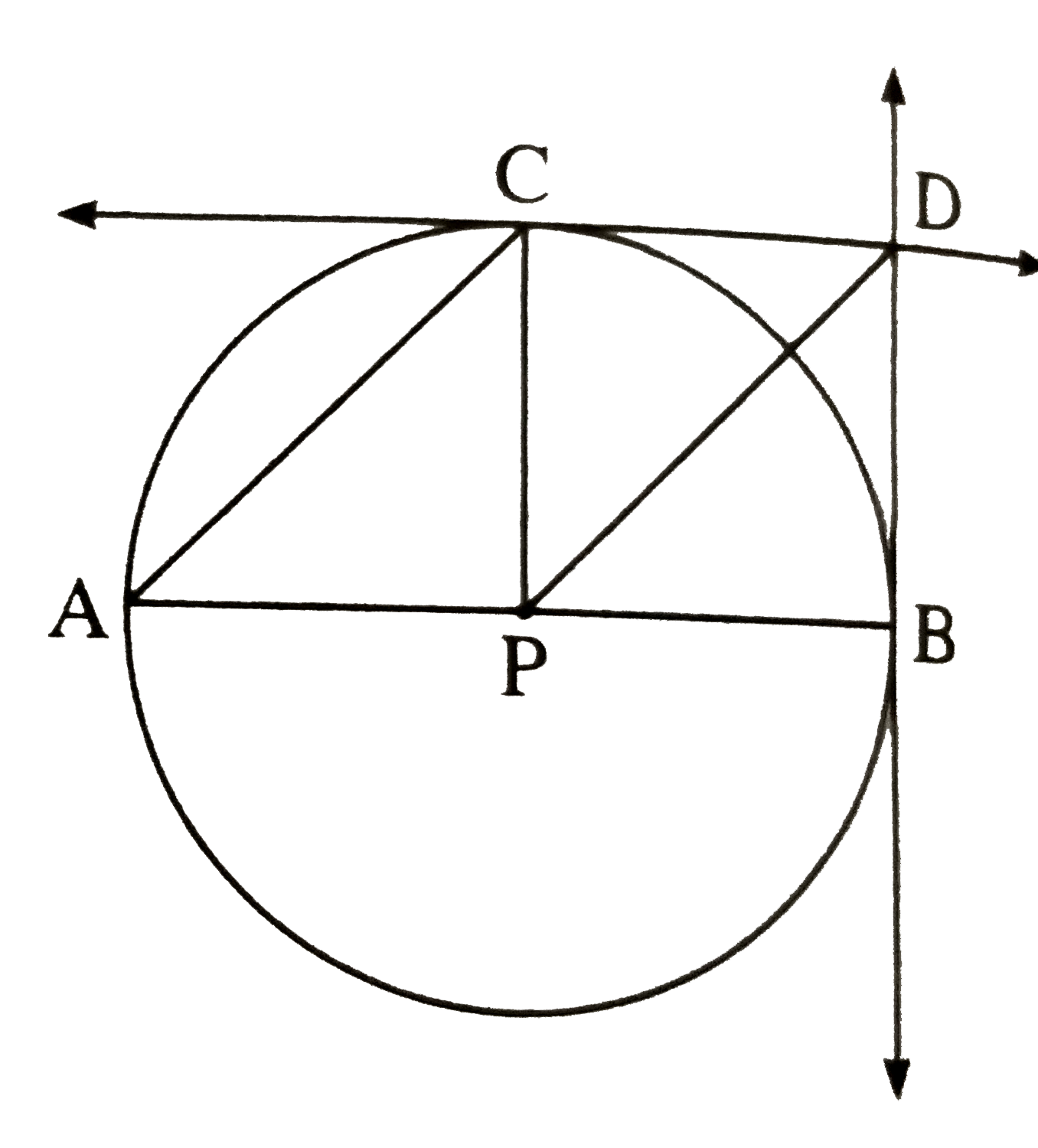 View Solution
View SolutionTwo circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D and P, Q respectively (see Fig. 10.40). Prove that ∠ACP =∠QCD.
View SolutionIn the figure, two circles with centers O and P intersect each other at points D and C. Line AB intersects the circle with centre O at points A and B and touches the circle with centre P at point E. Prove ∠ADE+∠BCE=180∘.
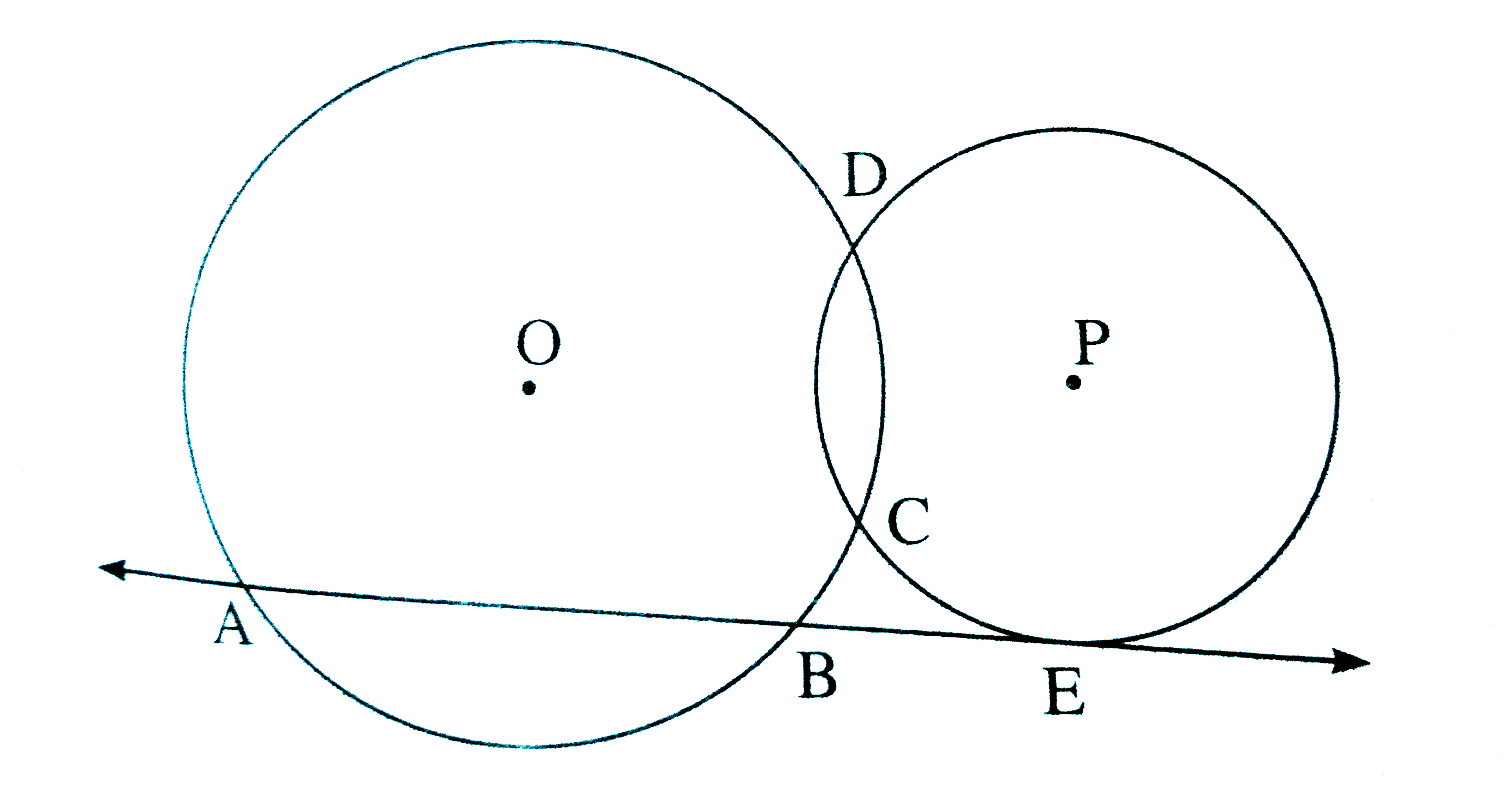 View Solution
View SolutionThe circumferences of three unequal circles whose centres are A, B and C pass througha common point, O, from which lines are drawn through A, B and C meeting the circumferences at A', B' and C". Show that the sides of ΔA'B'C' pass through the other points of intersection of the circles and are respectively parallel to the sides of the ΔABC
View SolutionABCD is a parallelogram whose AC and BD intersect at O. A line through O intersects AB at P and DC at Q. Prove that ar ( POA)= ar ( QOC).
View Solution

