Complete Solutions to EXERCISE 13 (A) of chapter SECTION AND MID-POINT FORMULA of Class 10 book with complete answers and questions
| Class 10 | MATHS |
| Chapter | SECTION AND MID-POINT FORMULA |
| SELINA PUBLICATION | EXERCISE 13 (A) |
| Number Of Questions | 33 |
| Academic Year | 2023-24 |
Calculate the co-ordinates of the point P which divides the line segment joining:
A (1, 3) and B (5,9) in the ratio 1 : 2
View SolutionCalculate the co-ordinates of the point P which divides the line segment joining:
A (-4, 6) and B (3, -5) in the ratio 3:2.
View SolutionThe ratio in which the line segment joining (2, -3) and (5,6) is divided by the x- axis is :
View SolutionIn what ratio is the line joining (2, -4) and (-3, 6) divided by the y-axis ?
View SolutionIn what ratio does the point (1, a) divide the join of (-1, 4) and (4, -1) ? Also, find the value of a.
View SolutionIn what ratio does the point (a, 6) divide the join of (-4, 3) and (2,8) ? Also, find the value of a
View SolutionIn what ratio is the join of (4, 3) and (2, -6) divided by the x-axis ? Also, find the co-ordinates of the point of intersection.
View SolutionFind the ratio in which the join of (-4, 7) and (3, 0) is divided by the y-axis. Also, find the co-ordinates of the point of intersection.
View SolutionPoints A, B, C and D divide the line segment joining the point (5, -10) and the origin in five equal parts. Find the co ordinates of B and D.
View SolutionThe line joining the points A (-3, -10) and B (-2, 6) is divided by the point P such that `(PB)/(AB) = (1)/(5).` Find the co-ordinates of P.
View SolutionP is a point on the line joining A (4, 3) and B (-2, 6) such that 5AP = 2BP. Find the coordinates of P.
View SolutionCalculate the ratio in which the line joining the points (-3, -1) and (5, 7) is divided by the line x = 2.
View SolutionCalculate the ratio in which the line segment A(6, 5) and B(4, -3) is divided by the line y = 2.
View SolutionThe point P (5,-4) divides the line segment AB, as shown in the figure, P (5, 4) in the ratio 2:5. Find the co-ordinates of points A and B. Given AP is smaller than BP.
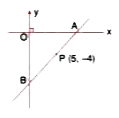
View SolutionFind the co-ordinates of the points of tri section of the line joining the points (-3, 0) and (6, 6).
View SolutionShow that the line segment joining the points (-5, 8) and (10, -4) is trisected by the co-ordinate axes.
View SolutionShow that A (3,-2) is a point of trisection of the line-segment joining the points (2, 1) and (5, -8).
View SolutionGiven a line segment AB joining the points A (-4, 6) and B (8, -3). Find :
(iii) the length of AB.
View SolutionIf A = (-4, 3) and B = (8,-6)
In what ratio is the line joining A and B, divided by the x-axis ?
View SolutionThe line segment joining the points M (5, 7) and N (-3, 2) is intersected by the y-axis at point L. Write down the abscissa of L. Hence, find the ratio in which L divides MN. Also, find the co-ordinates of L.
View SolutionA (2, 5), B (-1, 2) and C (5, 8) are the co-ordinates of the vertices of the triangle ABC. Points P and Q lie on AB and AC respectively, such that : AP: PB = AQ: QC = 1:2.
Calculate the co-ordinates of P and Q.
View SolutionA (2, 5), B (-1, 2) and C (5, 8) are the co-ordinates of the vertices of the triangle ABC. Points P and Q lie on AB and AC respectively, such that : AP: PB = AQ: QC = 1:2.
Show that : `PQ = (1)/(3) BC`.
View SolutionA (-3, 4), B (3, -1) and C (-2, 4) are the vertices of a triangle ABC. Find the length of line segment AP, where point P lies inside BC, such that BP: PC = 2 : 3.
View SolutionThe line segment joining A(2, 3) and B6, -5) is intercepted by x-axis at the point K. Write down the ordinate of the point K. Hence, find the ratio in which K divides AB. Also, find the co-ordinates of the point K.
View SolutionThe line segment joining A(4, 7) and B(-6, -2) is intercepted by the y-axis at the point K. Write down the abscissa of the point K. Hence, find the ratio in which K divides AB. Also, find the co-ordinates of the point K.
View SolutionThe line joining P(-4, 5) and Q(3, 2) intersects the y-axis at point R. PM and QN are perpendiculars from P and Q on the x-axis. Find:
the ratio PR : RQ
View SolutionThe line joining P(-4, 5) and Q(3, 2) intersects the y-axis at point R. PM and QN are perpendiculars from P and Q on the x-axis. Find:
the co-ordinates of R.
View SolutionThe line joining P(-4, 5) and Q(3, 2) intersects the y-axis at point R. PM and QN are perpendiculars from P and Q on the x-axis. Find:
the area of the quadrilateral PMNQ.
View SolutionIn the given figure, line APB meets the x-axis at point A and y-axis at point B. P is the point (-4, 2) and AP : PB = 1 : 2. Find the co-ordinates A and B .
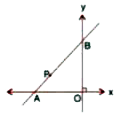
View SolutionGiven a line segment AB joining the points A (-4, 6) and B (8, -3). Find :
(i) the ratio in which AB is dividend by the Y-axis.
View SolutionGiven a line segment AB joining the points A (-4, 6) and B (8, -3). Find :
(ii) find the coordinates of the point of intersection. Divided by y-axis.
View SolutionGiven a line segment AB joining the points A (-4, 6) and B (8, -3). Find :
(iii) the length of AB.
View SolutionIf P (9a-2,-b) divides line segment joining A (3a+1,-3) and B(8a,5) in the ratio `3 : 1`, then find the values of a and b.
View Solution
EXERCISE 13 (A) questions and complete solutions for chapter SECTION AND MID-POINT FORMULA of SELINA MATHS (ENGLISH) of Class 10