Complete Solutions to (C )Medical entrances gallery of chapter MOTION IN A PLANE of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | MOTION IN A PLANE |
| DC PANDEY NEET | (C )Medical entrances gallery |
| Number Of Questions | 32 |
| Academic Year | 2023-24 |
If the velocity of a particle is `v = At + Bt^2`, where `A` and `B` are constant, then the distance travelled by it between `1 s` and `2 s` is :
View SolutionA particle of unit mass undergoes one-dimensional motion such that its velocity varies according to
`v(x) = beta x^(-2 n)`
where `beta` and `n` are constant and `x` is the position of the particle. The acceleration of the particle as a function of `x` is given by.
View SolutionThe ball is dropped from a bridge ` 122.5 m` above a river, After the ball has been falling for 2 s, a second ball is thrown straight down after it. What must its initial velocity be so that both hit the water at the same time ?
View SolutionA ball is thrown vertically upwards from the ground with a speed of `25.2 ms^(-1)`. How long does it take to reach its highest point and how high does it rise ? (Take `g=9.8 ms^(-2)`)
View SolutionA particle moves in an XY-plane in such a way that its x and y-coordinates vary with time according to
`x(t)=t^(3)-32t, y(t)=5t^(2)+12`
Find the acceleration of the particle, if t = 3 s.
View SolutionA point moving with constant acceleration from A to B in the straight line AB has velocities u and v at A and B respectively. Find its velocity at C, the mid point of AB. Also show that if the time from A to C is twice that from C to B, then `v =7 u`.
View SolutionThe displacement-time graph of a particle is as shown below. It indicates that
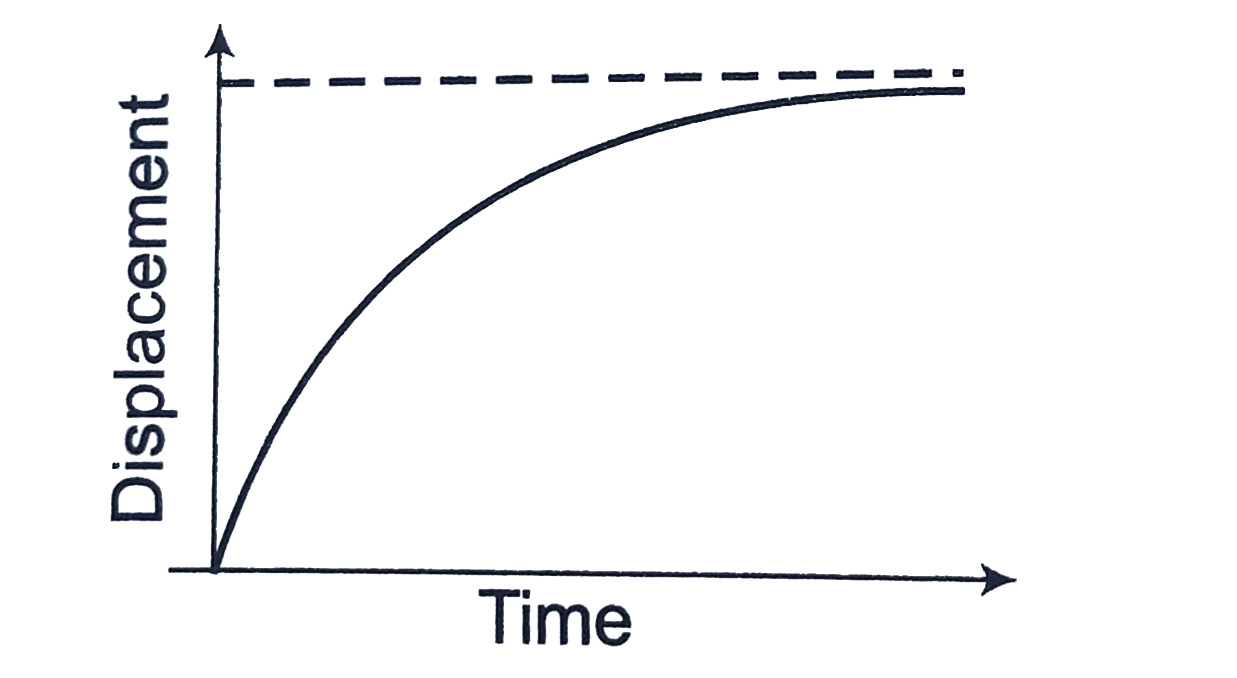
View SolutionA car starts from rest and accelerates uniformly to a speed of `180 kmh^(-1)` in 10 s. The distance covered by the car in the time interval is
View SolutionThe velocity - time graph for two bodies A and B are shown in figure. Then, the acceleration of A and B are in the ratio
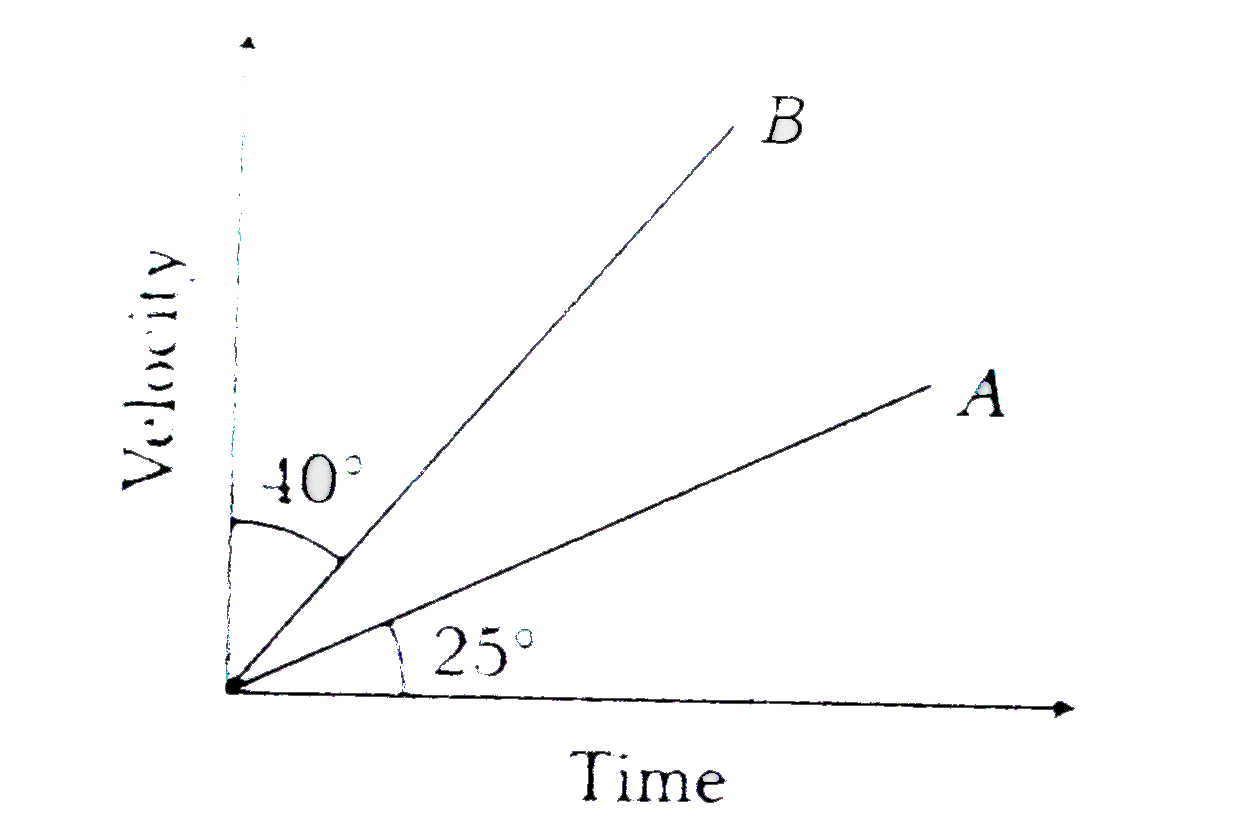
View SolutionA particle is moving such that its position coordinates `(x, y)` are `(2m, 3m)` at time `t=0, (6m, 7m)` at time `t=2 s`, and `(13 m, 14m)` at time `t=5 s`.
Average velocity vector`(vec(V)_(av))` from `t=0` to `t=5 s` is
View SolutionA ball thrown vertically upwards after reaching a maximum height h returns to the starting point after a time of 10 s. Its displacement after 5 s is
View SolutionA police jeep is chasing with, velocity of `45 km//h` a thief in another jeep moving with velocity `153 km//h`. Police fires a bullet with muzzle velocity of `180 m//s`. The velocity it will strike the car of the thief is.
View SolutionA particle moves with constant acceleration along a straight line streaming from rest. The percentage increase in its displacement during the 4th second compared to that in the 3rd second is
View SolutionA car covers the first half of the distance between two places at a speed of `40 kmh^(-1)` and second half at `60 kmh^(-1)` Calculate the average speed of the car.
View SolutionA particle starts moving from rest under uniform acceleration it travels a distance x in the first two seconds and a distance y in the next two seconds. If `y=nx`, then `n=`
View SolutionAt time t = 0, two bodies A and B at the same point. A moves with constant velocity `upsilon` and B starts from rest and moves with constant acceleration. Relative velocity of B w.r.t. A when the bodies meet each other is
View SolutionA motorclist drives from A to B with a uniform speed of `30 kmh^(-1)` and returns back with a speed of `20kmh^(-1)` . Find its average speed.
View SolutionA body starts from rest and moves with constant acceleration for t s. It travels a distance `x_1` in first half of time and `x_2` in next half of time, then
View SolutionThe acceleration of a moving body can be found from
View SolutionA stone falls freely under gravity. It covered distances `h_1, h_2` and `h_3` in the first `5` seconds. The next `5` seconds and the next `5` seconds respectively. The relation between `h_1, h_2` and `h_3` is :
View SolutionThe motion of a particle in straight line is an example of
View SolutionThe velocity-time graph of particle comes out to be a non-linear curve. The motion is
View SolutionA person reaches on a point directly opposite on the other bank of a river.The velocity of the water in the river is `4 m//s`and the velocity of the person in still water is `5 m//s`.If the width of the river is `84.6m`, time taken to cross the river in seconds is
View SolutionA body is thrown vertically upward from a point `A` 125 m above the ground. It goes up to a maximum height of 250 m above the ground and passes through `A` on its downward journey. The velocity of the body when it is at a height of 70 m above the ground is `(g=10 m//s^(2))`
View SolutionA particle is moving eastwards with velocity of `5 m//s`. In `10 sec` the velocity changes to `5 m//s` northwards. The average acceleration in this time is.
View SolutionThe velocity-time graph of robber's car and a chasing police car are shown in the following graph. Police car crosses the robber's car in time
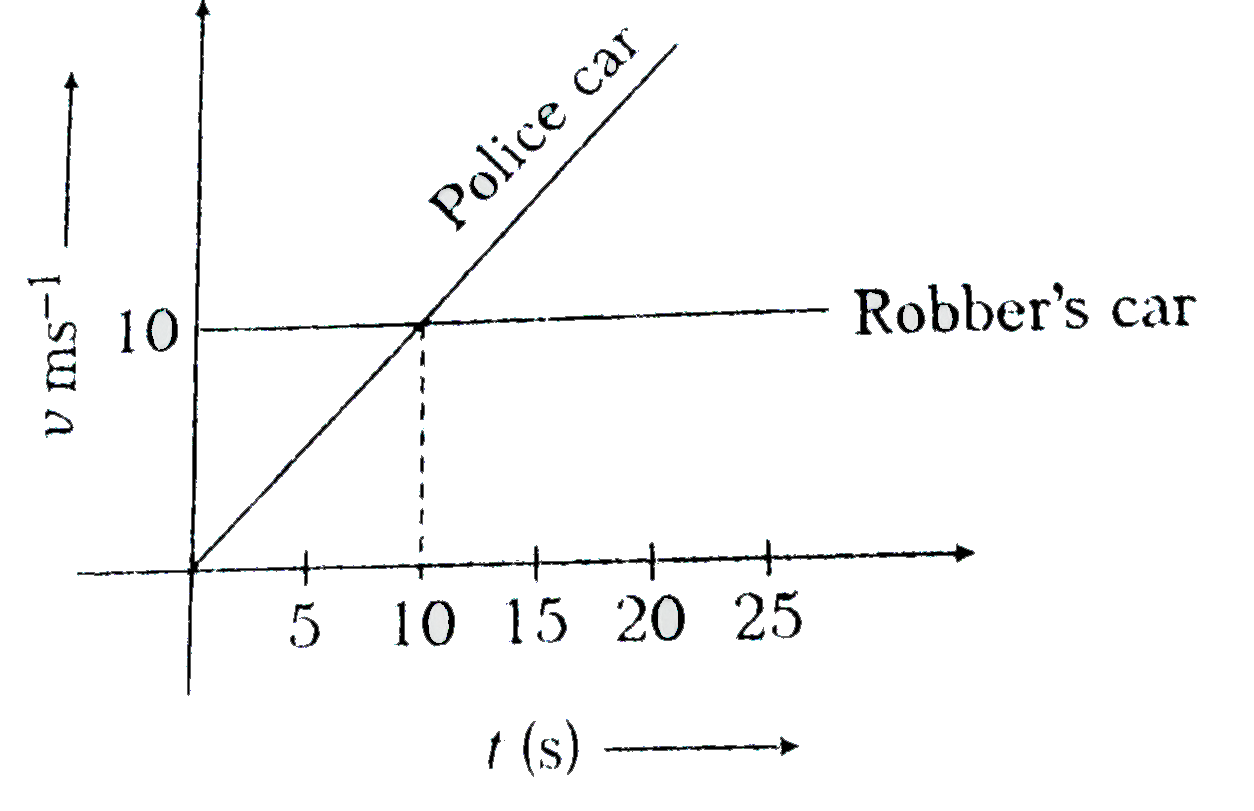
View SolutionInitial speed of an `alpha` particle inside a tube of length 4m is `1 kms^(-1)`, if it is accelerated in the tube and comes out with a speed of `9 kms^(-1)` , then the time for which the particle remains inside the tube is
View SolutionA body X is projected upwards with a velocity of `98 ms^(-1)`, after 4s, a second body Y is also projected upwards with the same initial velocity . Two bodies will meet after
View SolutionLet `r_(1)(t)=3t hat(i)+4t^(2)hat(j)`
and `r_(2)(t)=4t^(2) hat(i)+3t^()hat(j)`
represent the positions of particles 1 and 2, respectively, as function of time t, `r_(1)(t)` and `r_(2)(t)` are in meter and t in second. The relative speed of the two particle at the instant t = 1s, will be
View SolutionThe motion of a particle along a straight line is described by equation : `x = 8 + 12 t - t^3` where `x` is in meter and `t` in second. The retardation of the particle when its velocity becomes zero is.
View SolutionA scooter starts from rest have an acceleration of `1 ms^(-2)` while a car 150 m behind it starts from rest with an acceleration of `2ms^(-2)`. After how much time the car catches up with the scooter ?
View SolutionThe displacement x of a particle along a straight line at time t is given by `x = a_(0) +a_(1)t + a_(2)t ^(2)`. The acceleration of the particle is
View Solution
(C )Medical entrances gallery questions and complete solutions for chapter MOTION IN A PLANE of DC PANDEY ENGLISH of Class 11