Complete Solutions to Level 2 Subjective of chapter CENTRE OF MASS, LINEAR MOMENTUM AND COLLISION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | CENTRE OF MASS, LINEAR MOMENTUM AND COLLISION |
| DC PANDEY | Level 2 Subjective |
| Number Of Questions | 21 |
| Academic Year | 2023-24 |
A ladder AP of length `5m` inclined to a vertical wall is slipping over a horizontal surface with velocity of `2m//s`, when A is at distance `3m` from ground. What is the velocity of COM at this moment?

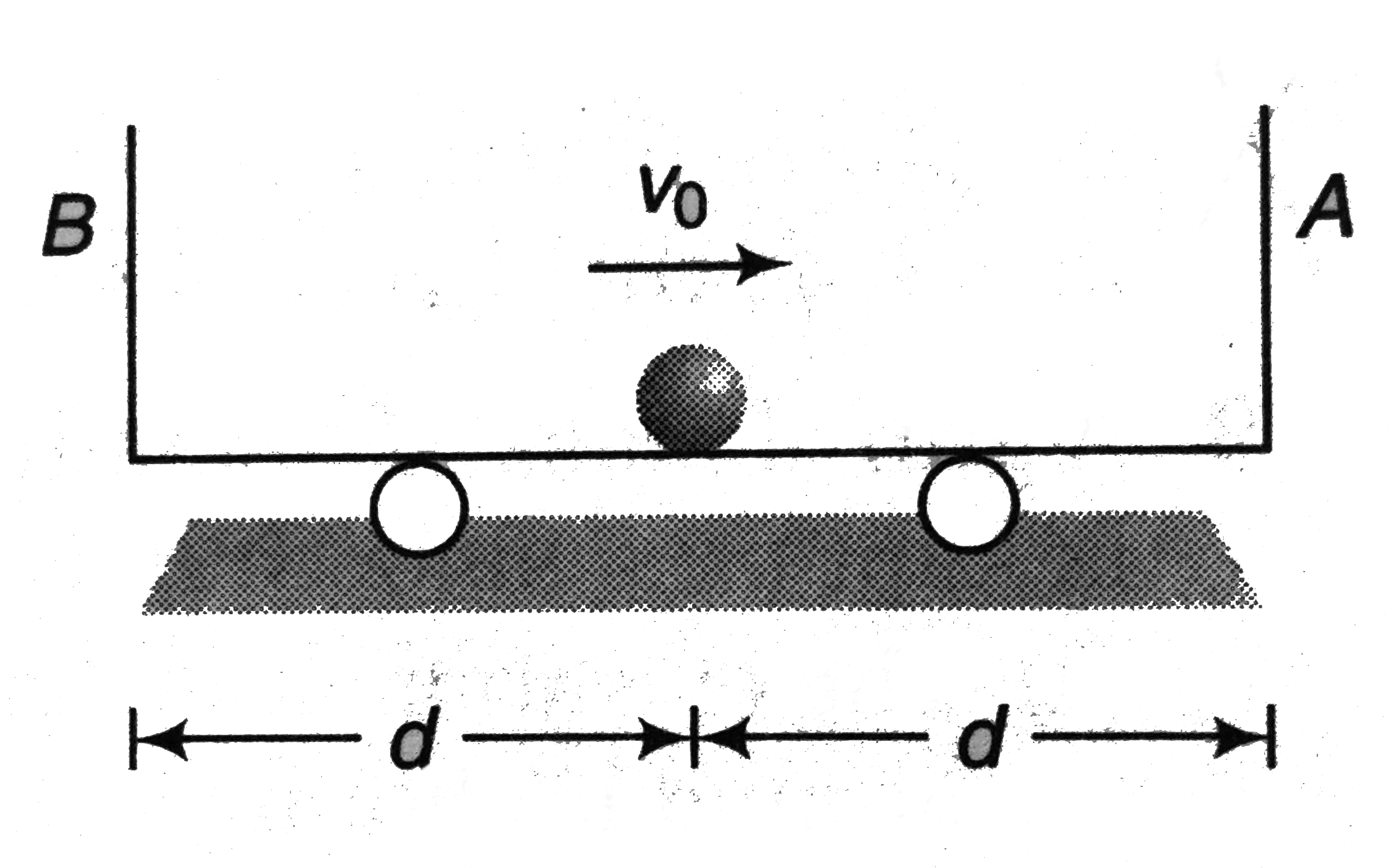
View SolutionA ball of negligible size and mass `m` is given a velocity `v_0` on the centre of the cart which has a mass M and is originally at rest. If the coefficient of restitution between the ball and walls A and B is e. Determine the velocity of the ball and the cart just after the ball strikes A. Also, determine the total time needed for the ball to strike A, rebound, then strike B, and rebound and then return to the centre of the cart. Neglect friction.
View SolutionTwo point masses `m_1` and `m_2` are connected by a spring of natural length `l_0`. The spring is compressed such that the two point masses touch each other and then they are fastened by a string. Then the system is moved with a velocity `v_0` along positive x-axis. When the system reached the origin, the string breaks `(t=0)`. The position of the point mass `m_1` is given by `x_1=v_0t-A(1-cos omegat)` where A and `omega` are constants. Find the position of the second block as a function of time. Also, find the relation between A and `l_0`.
View SolutionA small sphere of radius R is held against the inner surface of larger sphere of radius `6R` (as shown in figure). The masses of large and small spheres are `4M` and M respectively. This arrangement is placed on a horizontal table. There is no friction between any surfaces of contact. The small sphere is now released. Find the coordinates of the centre of the large spheres, when the smaller sphere reaches the other extreme position.

View SolutionA chain of length `l` and mass `m` lies in a pile on the floor. If its end A is raised vertically at a constant speed `v_0`, express in terms of the length y of chain which is off the floor at any given instant.
(a) The magnitude of the force P applied to end A.
(b) Energy lost during the lifting of the chain.

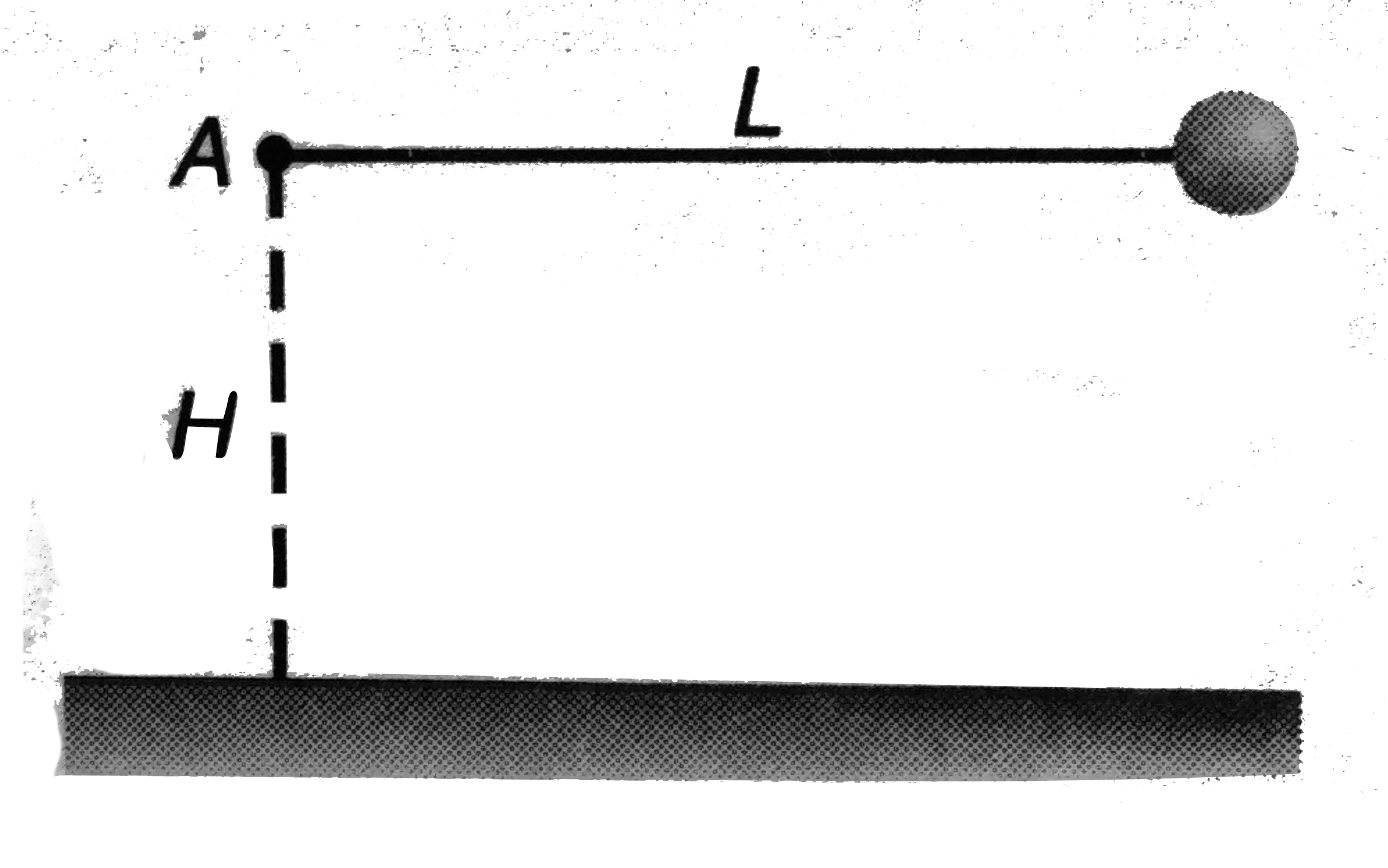
View SolutionA is a fixed point at height H above a perfectly inelastic smooth horizontal plane. A light inextesnsible string of length `L(gtH)` has one end attached to A and other to a heavy particle. The particle is held at the level of A with string just taut and released from rest. Find the height of the particle above the plane when it is next instaneously at rest .
View SolutionA particle of mass `2m` is projected at an angle of `45^@` with horizontal with a velocity of `20sqrt2m//s`. After `1s` explosion takes place and the particle is broken into two equal pieces. As a result of explosion one part comes to rest. Find the maximum height attained by the other part. Take `g=10m//s^2`.
View SolutionA sphere of mass `m`, impinges obliquely on a sphere, of mass M, which is at rest. Show that, if `m=eM`, the directions of motion of the sphere after impact are at right angles.
View SolutionA gun of mass M(including the carriage) fires a shot of mass `m`. The gun along with the carriage is kept on a smooth horizontal surface. The muzzle speed of the bullet `v_r` is constant. Find
(a) The elevation of the gun with horizontal at which maximum range of bullet with respect to the ground is obtained.
(b) The maximum range of the bullet.
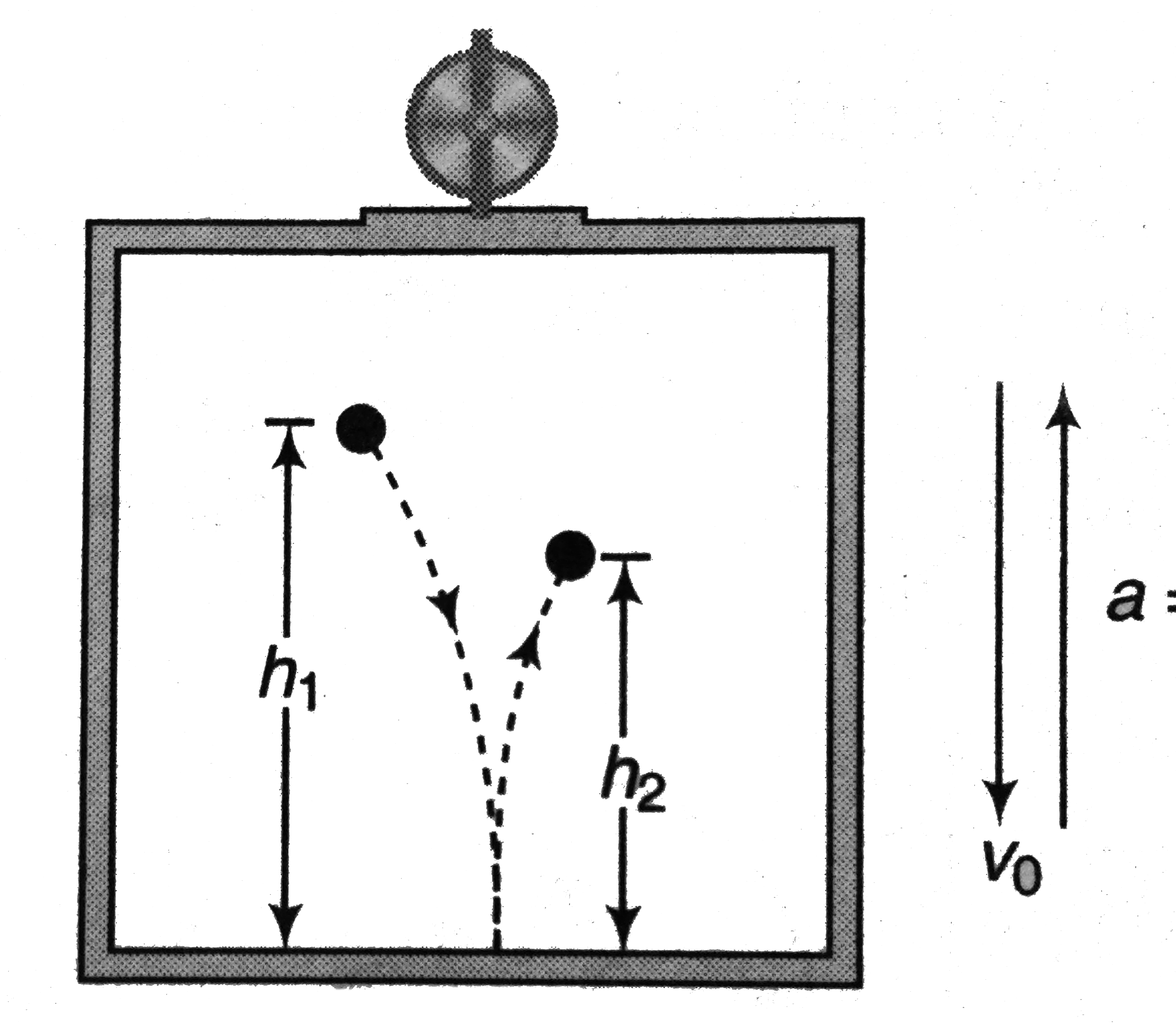
View SolutionA ball is released from rest relative to the elevator at a distance `h_1` above the floor. The speed of the elevator at the time of ball release is `v_0`. Determine the bounce height `h_2` relative to elevator of the ball (a) if `v_0` is constant and (b) if an upward elevator accleration `a=g/4` begins at the instant the ball is released. The coefficient of restitution for the impact is `e`.
View SolutionA plank of mass `5kg` is placed on a frictionless horizontal plane. Further a block of mass `1kg` is placed over the plank. A massless spring of natural length `2m` is fixed to the plank by its one end. The other end of spring is compressed by the block by half of spring's natural length. They system is now released from the rest. What is the velocity of the plank when block leaves the plank? (The stiffness constant of spring is `100N//m`)

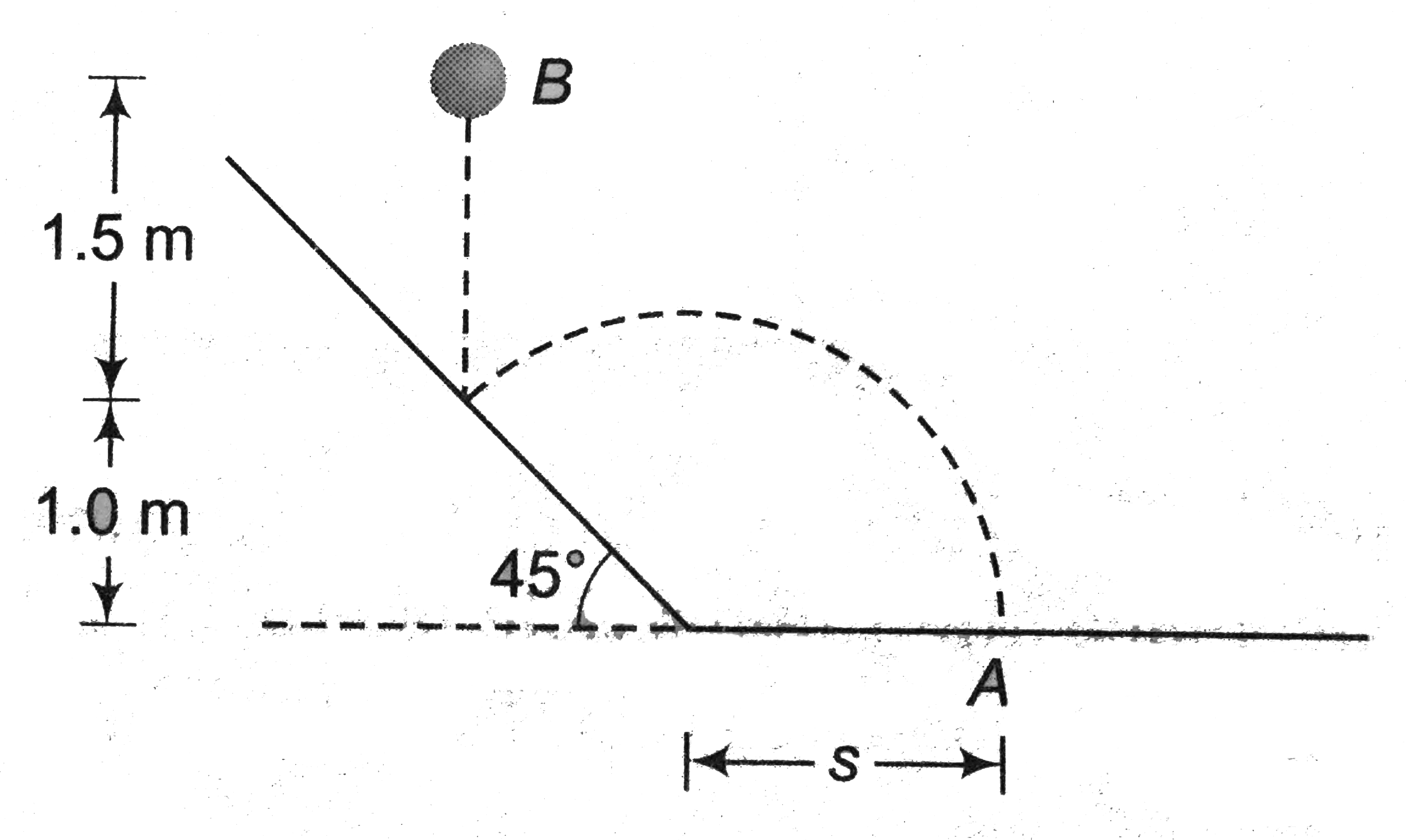
View SolutionTo test the manufactured properties of `10N` steel balls, each ball is released from rest as shown and strikes a `45^@` inclined surface. If the coefficient of restitution is to be `e=0.8`, determine the distance s, where the ball must strike the horizontal plane at A. At what speed does the ball stike at A? (`g=9.8m//s^2`)
View SolutionTwo particles A and B of equal masses lie close together on a horizontal table and are connected by a light inextensible string of length `l`. A is projected vertically upwards with a velocity `sqrt(10gl)`. Find the velocity with which it reaches the table again.
View SolutionA small cube of mass `m` slides down a circular path of radius R cut into a large block of mass M, as shown in figure. M rests on a table, and both blocks move without friction. The blocks are initially at rest, and m starts from the top of the path. Find the horizontal distance from the bottom of block where cube hits the cable
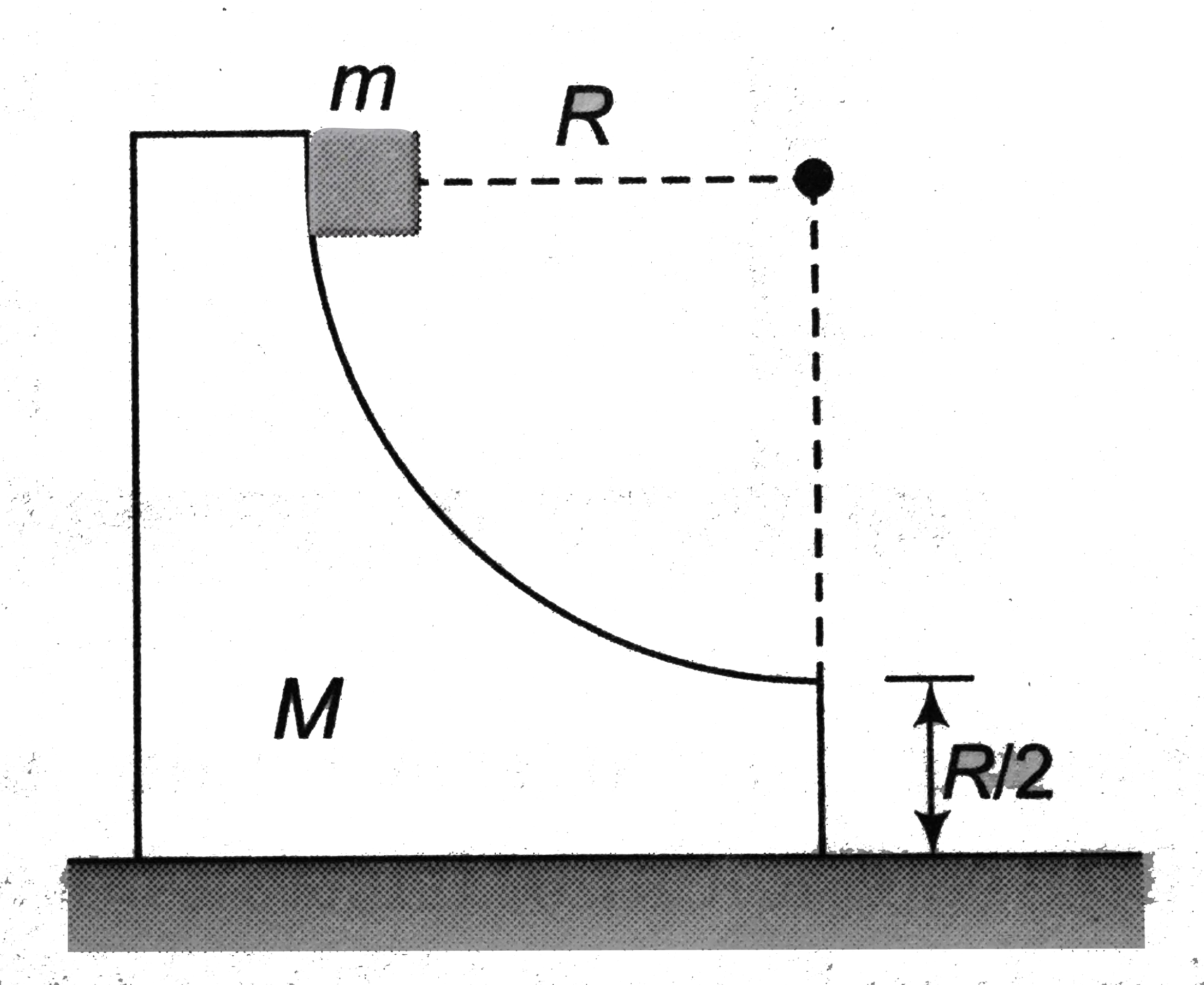
View SolutionA thin hoop of mass M and radius r is placed on a horizontal plane. At the initial instant, the hoop is at rest. A small washer of mass m with zero initial velocity slides from the upper point of the hoop along a smooth groove in the inner surfaces of the hoop. Determine the velocity u of the centre of the hoop at the moment when the washer is at a certain point A of the hoop, whose radius vector forms an angle `phi` with the vertical (figure). The friction between the hoop and the plane should be neglected

View SolutionA shell of mass `1kg` is projected with velocity `20m//s` at an angle `60^@` with horizontal. It collides inelastically with a ball of mass `1kg` which is suspended through a thread of length `1m`. The other end of the thread is attached to the ceiling of a trolley of mass `4/3kg` as shown in figure. Initially the trolley is stationary and it is free to move along horizontal rails wihtout any friction. What is the maximum deflection of the thread with vertical? String does not slack. Take `g=10m//s^2`.

View SolutionA small ball is projected at an angle `alpha` with an initial velocity u between two vertical walls such that in the absence of the wall its range would have been `5d`. Given that all the collisions are perfectly elastic and distance between the walls be `d/2`, find.
(a) maximum height atained by the ball.
(b) total number of collisions with the walls before the ball comes back to the ground, and
(c) point at which the ball finally falls. The walls are supposed to be very tall.
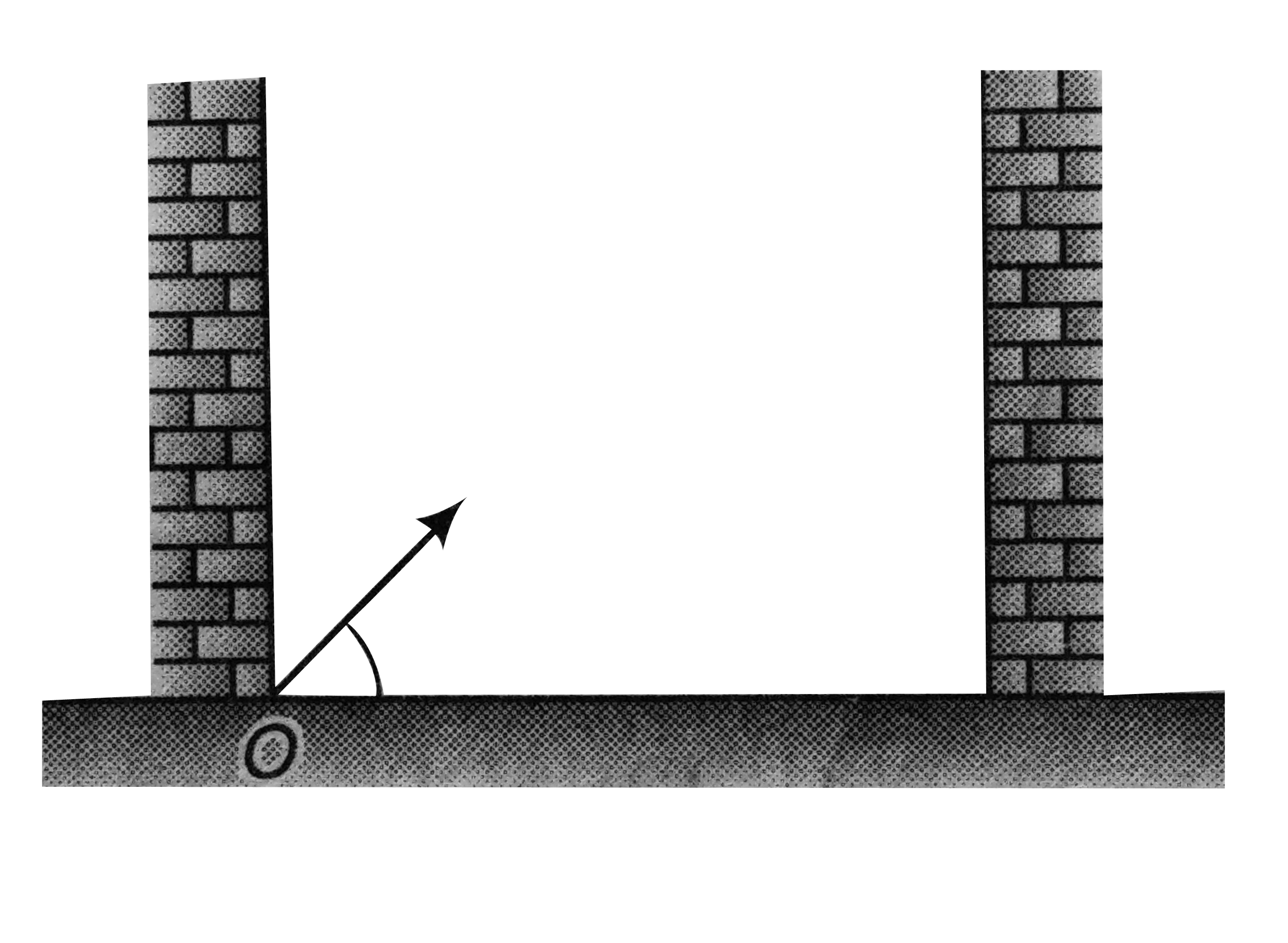
View SolutionTwo large rigid vertical walls A and B are parallel to each other and separated by `10m`. A particle of mass `10g` is projected with an initial velocity of `20m//s` at `45^@` to the horizontal from point P on the ground, such that `AP=5m`. The plane of motion of the particle is vertical and perpendicular to the walls. Assuming that all the collisions are perfectly elastic, find the maximum height attained by the particle and the total number of collisions suffered by the particle with the walls before it hits ground. Take `g=10m//s^2`.

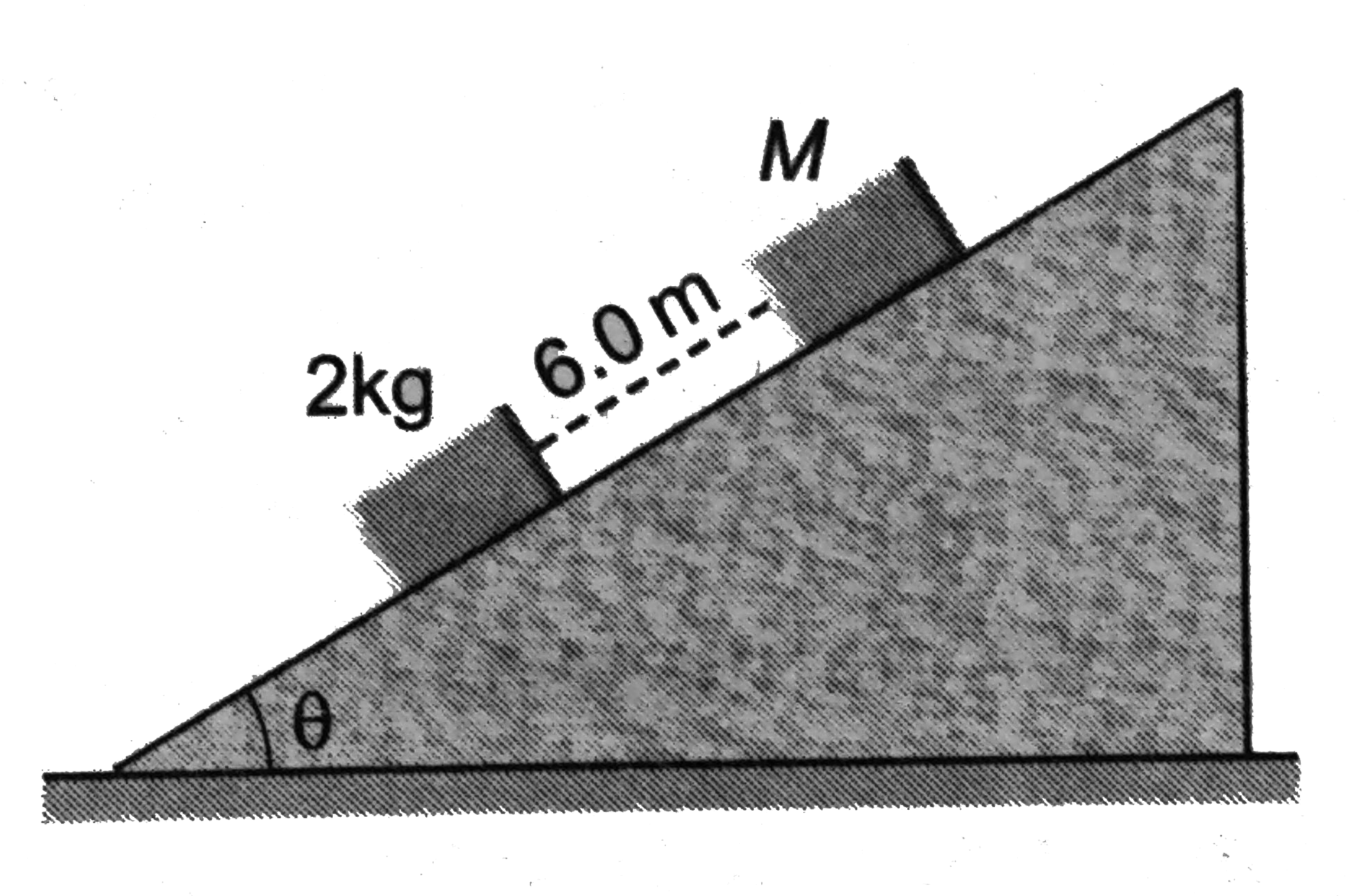
View SolutionTwo blocks of masses `2kg` and M are at rest on an inclined plane and are separated by a distance of `6.0m` as shown. The coefficient of friction between each block and the inclined plane is `0.25`. The `2kg` block is given a velocity of `10.0m//s` up the inclined plane. It collides with M, comes back and has a velocity of `1.0m//s` when it reaches its initial position. The other block M after the collision moves `0.5m` up and comes to rest. Calculate the coefficient of restitution between the blocks and the mass of the block M.
[Take `sintheta=tantheta=0.05` and `g=10m//s^2`]
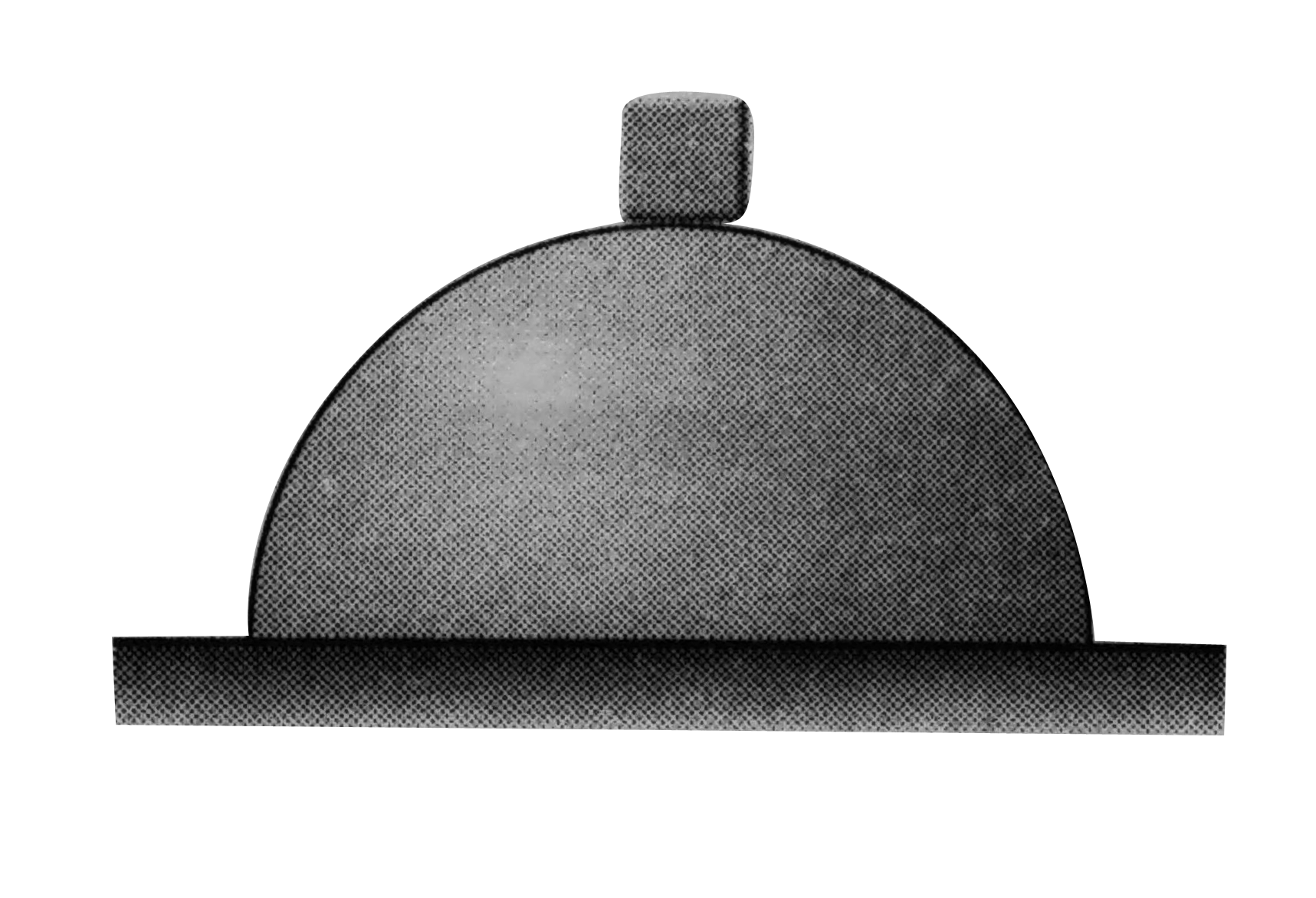
View SolutionA small block of mass `m` is placed on top of a smooth hemisphere also of mass `m` which is placed on a smooth horizontal surface. If the block begins to slide down due to a negligible small impulse, show that it will loose contact with the hemisphere when the radial line through vertical makes an angle `theta` given by the equaition `cos^3theta-6costheta+4=0`.
View SolutionA ball is projected from a given point with velocity u at some angle with the horizontal and after hitting a vertical wall returns to the same point. Show that the distance of the point from the wall must be less than `(eu^2)/((1+e)g)`, where e is the coefficient of restitution.
View Solution
Level 2 Subjective questions and complete solutions for chapter CENTRE OF MASS, LINEAR MOMENTUM AND COLLISION of DC PANDEY ENGLISH of Class 11
All Exercises from DC PANDEY Class 11 PHYSICS CENTRE OF MASS, LINEAR MOMENTUM AND COLLISION
- Example
- Exercise 11.1
- Exercise 11.2
- Exercise 11.3
- Exercise 11.4
- Exercise 11.5
- Exercise 11.6
- Level 1 Assertion And Reason
- Level 1 Objective
- Level 1 Subjective
- Level 1 Subjective Questions
- Level 2 Comprehension Based
- Level 2 Comprehension Based Questions
- Level 2 More Than One Correct
- Level 2 Single Correct
- Level 2 Single Correct Option
- Level 2 Subjective
- MiscellaneousExamples
- Solved Examples
- Type 1