Complete Solutions to Miscellaneous Example of chapter KINEMATICS of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | KINEMATICS |
| DC PANDEY | Miscellaneous Example |
| Number Of Questions | 12 |
| Academic Year | 2023-24 |
A rocket is fired vertically upwards with a net acceleration of `4 m//s^2` and initial velocity zero. After `5s` its fuel is finished and it decelerates with g. At the highest point its velocity becomes zero. Then, it accelerates downwards with acceleration g and return back to ground. Plot velocity-time and displacement -time graphs for the complete journey. Take `g=10m//s^2.`
View SolutionAn open lift is moving upward with velocity `10 m//s.` It has an upward acceleration of `2 m//s^2.` A ball is projected upwards with velocity `20 m//s` relative to ground. Find
(a) time when ball again meets the lift
(b) displacement of lift and ball at that instant.
(c) distance travelled by the ball upto that instant.
Take `g=10 m//s^2`
View SolutionA particle starts with an initial velocity and passes successively over the two halves of a given distance with constant accelerations `a_1 and a_2` respectively. Show that the final velocity is the same as if the whole distance is covered with a uniform acceleration `(a_1 + a_2)/2 .`
View SolutionIn a car race, car A takes a time t less than car B at the finish and passes the finishing point with speed v more than that of the car B. Assuming that both the cars start from rest and travel with constant acceleration `a_1 and a_2` respectively. Show that `v=sqrt (a_1 a_2) t.`
View SolutionAn open elevator is ascending with constant speed `v=10m//s.` A ball is thrown vertically up by a boy on the lift when he is at a height `h=10m` from the ground. The velocity of projection is `v=30 m//s` with respect to elevator. Find
(a) the maximum height attained by the ball.
(b) the time taken by the ball to meet the elevator again.
(c) time taken by the ball to reach the ground after crossing the elevator.
View SolutionFrom an elevated point A, a stone is projected vertically upwards. When the stone reaches a distance h below A, its velocity is doubleof what it was at a height h above A. Show that the greatest height attained by the stone is `5/3 h.`
View SolutionVelocity of a particle moving in a straight line varies with its displacement as `v=(sqrt(4 +4s))m//s.` Displacement of particle at time `t =0` is `s = 0`. Find displacement of particle at time `t=2 s`.
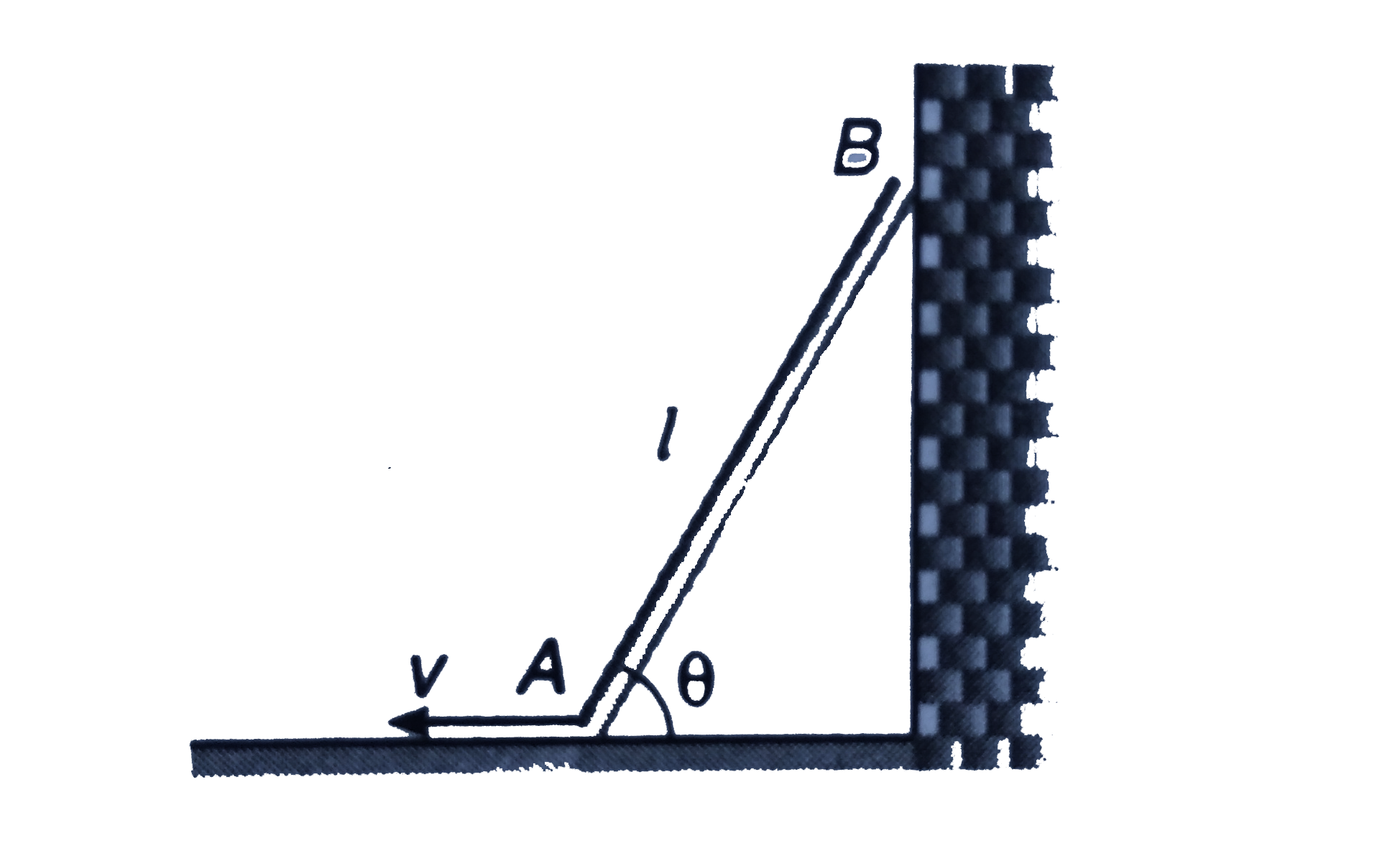
View SolutionFigure shows a rod of length l resting on a wall and the floor. Its lower end A is pulled towards left with a constant velocity v. Find the velocity of the other end B downward when the rod makes an angle `theta` with the horizontal.
View SolutionA particle is moving in a straight line with constant acceleration. If x,y and z be the distances described by a particle during the pth, qth and rth second respectively, prove that (q-r)x+(r-p)y+(p-q)z=0
View SolutionThree particles A, B and C are situated at the vertices of an equilateral triangle ABC of side d at time `t=0.` Each of the particles moves with constant speed v. A always has its velocity along AB, B along BC and C along CA. At what time will the particles meet each other?
View SolutionAn elevator car whose floor to ceiling distance is equal to `2.7m` starts ascending with constant acceleration `1.2 m//s^2.` 2 s after the start, a bolt begins falling from the ceiling of the car. Find
(a)the time after which bolt hits the floor of the elevator.
(b)the net displacement and distance travelled by the bolt, with respect to earth. (Take `g=9.8 m//s^2)`
View SolutionA man wants to reach point B on the opposite bank of a river flowing at a speed as shown in figure. What minimum speed relative to water should the man have so that he can reach point B? In which direction should he swim?
View Solution
Miscellaneous Example questions and complete solutions for chapter KINEMATICS of DC PANDEY ENGLISH of Class 11
All Exercises from DC PANDEY Class 11 PHYSICS KINEMATICS
- Assertion And Reason
- Comprehension
- COMPREHENSION_TYPE
- Example
- Example Type 1
- Example Type 2
- Example Type 3
- Example Type 4
- Example Type 5
- Example Type 6
- Example Type 7
- Exercise 6.1
- Exercise 6.2
- Exercise 6.3
- Exercise 6.4
- Exercise 6.5
- Exercise 6.6
- Exercise 6.7
- Exercise 6.8
- Exercise 6.9
- INTEGER_TYPE
- MATCH THE COLUMN
- MCQ_TYPE
- Miscellaneous Example
- More Than One Correct
- Objective
- SCQ_TYPE
- Subjective
- Subjective Questions