Complete Solutions to LEVEL - (C.W) of chapter TRANSMISSION OF HEAT of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | TRANSMISSION OF HEAT |
| NARAYANA | LEVEL - (C.W) |
| Number Of Questions | 27 |
| Academic Year | 2023-24 |
Three rods `A,B` and `C` have the same dimensions Their conductivities are `K_(A)` K and `K_(C)` respectively `A` and `B` are placed end to end with their free ends kept at certain temperature difference `C` is placed separately with its ends kept at same temperature difference The two arrangements conduct heat at the same rate `K_(c)` must be equal to .
View SolutionTwo identical slabs are welded end to end and `20cal` of heat flows through if for `4min` If the two slabs are now welded by placing them one through two ends under the same difference of temperatures the time taken is .
View SolutionA slab consists of two parallel layers of copper and brass of the same thichness and having thermal conductivities in the ratio `1:4` If the free face of brass is at `100^(@)C` anf that of copper at `0^(@)C` the temperature of interface is .
View SolutionTwo metal plates of same area and thickness `l_(1)` and `l_(2)` are arranged in series If the thermal conductivities of the materials of the two plates are `K_(1)` and `K_(2)` The thermal conductivity of the combination is .
View SolutionTwo hollow suphers of same material one with double the radius of the other and double the thickness of the other filled with ice, the ratio of time in which ice gets melted in the two spheres is .
View SolutionA wall has two layers A and B, each made of different material. Both the layers have the same thickness. The thermal conductivity of the material of A is twice that of B . Under thermal equilibrium, the temperature difference across the wall is `36^@C.` The temperature difference across the layer A is
View SolutionTwo rods of length l and `2l` thermal conductivities `2K` and `K` are connected end to end. If cross sectional areas of two rods are eual, then equivalent thermal conductivity of the system is .
View SolutionThree rods of identical cross-sectional area and made from the same metal form the sides of an insosceles triangle `ABC` right angled at `B` The pints `A` and `B` are maintained at temperatures `T` and `sqrtT` respectively in the steady state Assuming that only heat conduction takes place, temperature of point `C` is .
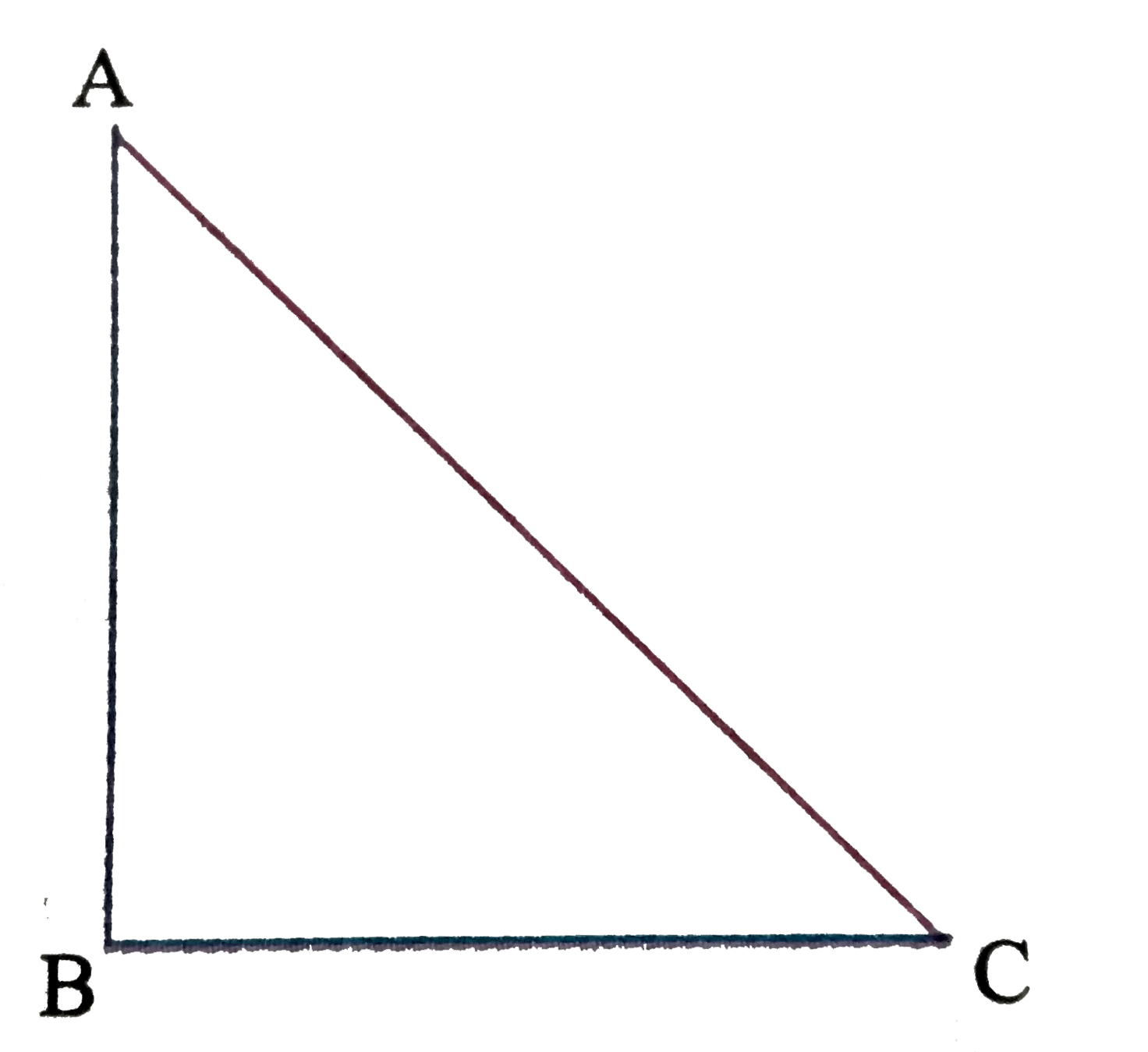 .
.
View SolutionA cube of side `10cm` is filled with ice of density `0.9//c.c` Thickness of the walls of the cube is `1mm` and thermal conductivity of the material of the cube is steam bath maintained at a placed in steam bath maintained at a temperature of `100^(@)C` the time in which ice completely melts is `(L_(ice)=80cal//gm)` .
View SolutionA slab of stone area `3600 cm^(2)` and thickness `10cm` is exposed on the lower surface to steam at `100^(@)C` A block of ice at `0^(@)C` rest on upper surface of the slab. In one hour `4.8kg` of ice is melted. The thermal conductivity of the stone in `Js^(-1)m^(-1)k^(-1)` is (latent heat of ice `=3.36 xx 10^(5) J//Kg` .
View SolutionA black body is at a temperature of `2800K` The energy of radiation emitted by this object with wavelength between `499nm` and 500 nm is `U_(1)` between 999nm and 1000nm is `U_(2)` and between 1499 nm and 1500 nm is `U_(3)` The Wien's constant `b =2.80 xx 10^(6)` nm `K` Then .
View SolutionWhen the temperature of a black body increases, it is observed that the wavelength corresponding to maximum energy changes from `0.26mum` to `0.13mum`. The ratio of the emissive powers of the body at the respective temperatures is
View SolutionFor an enclosure maintained at 2000K, the maximum radiation occurs at wavelength `lambda_m`. If the temperature is raised to 3000K, the peak will shift to
View SolutionThe power radiated by a black is `P` and it radiates maximum energy around the wavelength `lambda_(0)` If the temperature of the black body is now changed so that it radiates maximum energy around a wavelength `3lambda_(0)//4` the power radiated by it will increase by a factor of .
View SolutionThe rates of heat radiation from two patches of skin each of area A on a patient's chest differ by `2%` If the patch of the lower temp is at `300K` and emissivity of both the patches is assumed to be unity, the temp of other patch would be .
View SolutionA spherical black body with a radius of `12cm` radiates `450W `power at `500K`. If the radius were halved and the temperature doubled, the power radiated in watt would be
View SolutionIf the temperature of the sun were to increase form T to 2T and its radius from R to 2R, then the ratio of the radiant energy received on earth to what it was previously will be
View SolutionThe radiation emitted by a star `A` is `1000` times that of the sun. If the surface temperature of the sun and star `A` are `6000 K` and `2000 K` respectively. The ratio of the radii of the star `A` and the sun is:
View SolutionTwo electric bulbs have filaments of lengths `L` and `2L` diameters `2d` and d and emissivities `3e` and 4e If their temperatures are in the ratio 2:3 their powers will be in the ratio of .
View SolutionIf the absolute temperature of a black body is doubled the percentage increase in the rate of loss of heat by radiation is .
View SolutionA sphere and a cube of same material and same volume are heated up to same temperature and allowed to cool in the same sorroundings. The radio of the amounts of radiations emitted in equal time intervals will be
View SolutionA black metal foil is warmed by radiation from a small sphere at temperature `T` and at a distance d it is found that the power received by the foil is `P` If both the temperature and the distance are doubled the power received by the foil will be .
View SolutionA very small hole in an electric furnace is used for heating metals. The hole nearly acts as black body. The area of the hole is `200mm^(2)` To keep a metal at `727^(@)C` heat energy flowing throungh this hole per sec in joules is `(sigam =5.67 xx 10^(-8)Wm^(-2)K^(-4))` .
View SolutionThe surface of a black body is at a tempera ture `727^(@)C` and its cross section is `1m^(2)` Heat radi ated from this surface in one minute in Joules is (Stefan's constant `=5.7 xx 10^(-8) W//m^(2)//k^(4))` .
View SolutionA body cools down from `50^(@)C` to `45^(@)C` in `5` minutes and to `40^(@)C` in another `8` minutes. Find the temperature of the surrounding.
View SolutionA hot body is placed in cold surroundings It's rate of cooling is `3^(@)C` per minute when its temperature is `70^(@)C` and `1.5^(@)C` per minute when its temperature `50^(@)C` it s rate of cooling when its temperature is `40^(@)C` .
View SolutionA calorimeter of water equivalent `5g` has water of mass `55g` upto a certain level Another identical calorimeter has a liquid of mass '38g' upto same level As both of them cool in the same surrounding from `50^(@)C` to `46^(@)C` water takes `80s` where as the liquid takes `32s` to cool. If the specific heat of water is `1cal//g-.^(@)C` the specific heat of the liquid in `cal//g-.^(@)C` is .
View Solution
LEVEL - (C.W) questions and complete solutions for chapter TRANSMISSION OF HEAT of NARAYANA PHYSICS (HINGLISH) of Class 11