Complete Solutions to LEVEL-II(C.W) of chapter TRANSMISSION OF HEAT of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | TRANSMISSION OF HEAT |
| NARAYANA | LEVEL-II(C.W) |
| Number Of Questions | 27 |
| Academic Year | 2023-24 |
Three metal rods of coefficient of thermal conductivities `K,2K,3K` conducts heats of `3Q, 2Q,Q` per seconds through unit area then the ratio of temperature gradients .
View SolutionTwo rods one is semi circular of thermal conductivity `K_(1)` and otheir is straight of thermal conductivity `K_(2)` and of same cross sectional area are joined as shown in the The points `A` and `B` are maintained at same temperature difference. If rate of flow of heat is same in two rods then `K_(1)//K_(2)` is
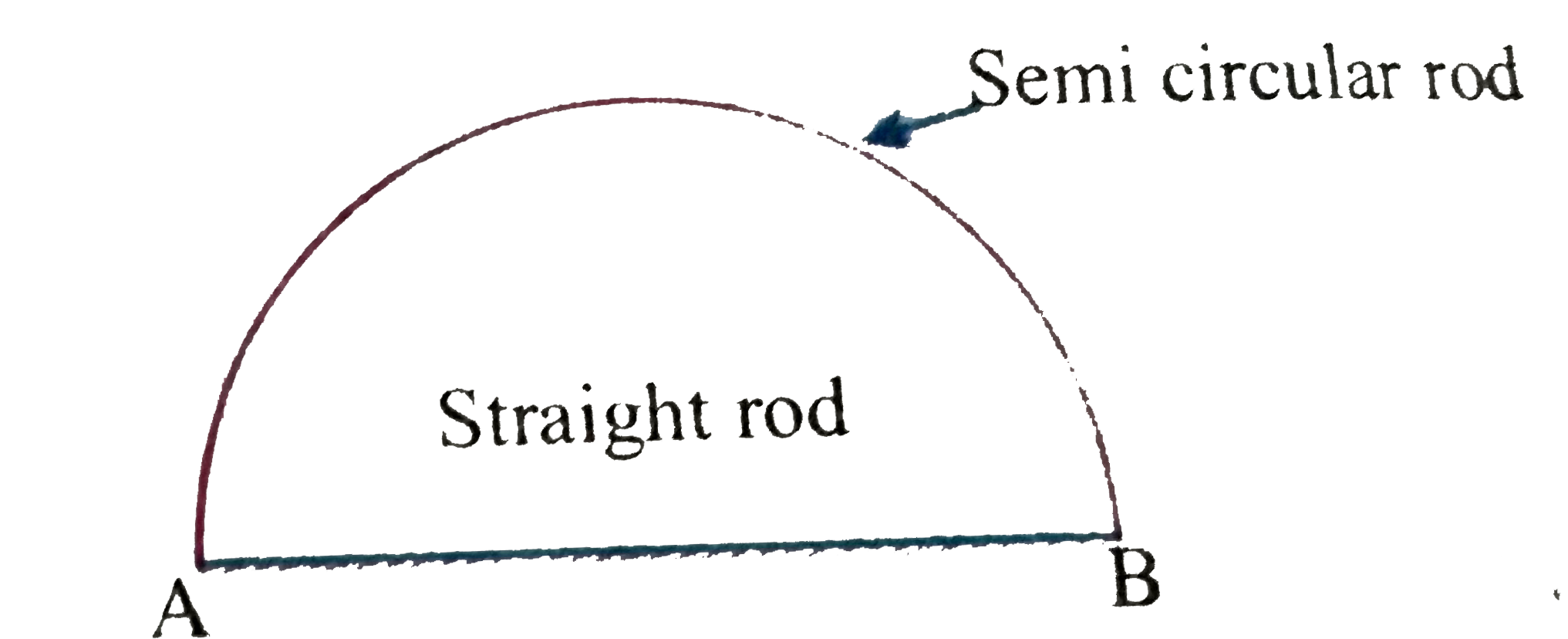 .
.
View SolutionTwo identical rods of same metal are first waleded in series and then in parallel are maintained at same temperature difference then the ratio of heats conducted in same time is .
View SolutionTwo slabs `A` and `B` of equal surface area are placed one over the other such that their surfaces are completely in contact The thickness and coefficient of thermal conductivities of slab `A` is twice that `B` The first surface of slab `A` is maintained at `100^(@) C` while the second surface of slab `B` is maintained at `25^(@)C` The temperature at the contact of their surfaces is .
View SolutionThree metal rods of same lenghs and same area of cross sections having conductivities `1,2,3,` units are connected in series Then their effective conductivity will be .
View SolutionTwo hollow supheres of thickness are filled with ice The ratio of their diameter is `1:2` and the materials is `2 :3` The ratio of times in which the ice gets melted in the two spheres is .
View SolutionThree rods of same dimensions have thermal condutivity `3K,2K` and `K` They are arranged as shown in Then the temperature of the junction in steady state is
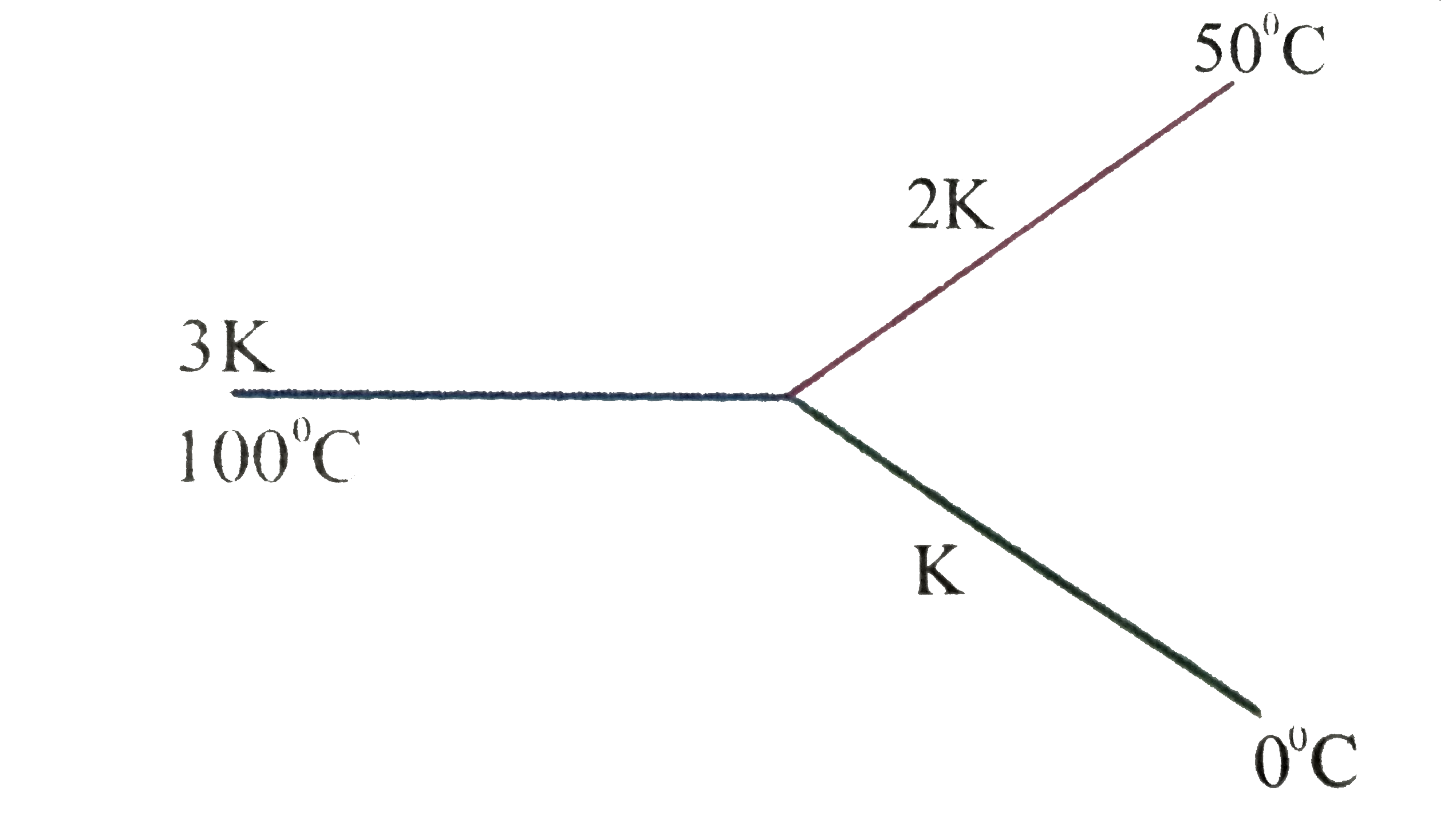 .
.
View SolutionThree rods of lengths `L,21` and `3L` having thermal conductivities `3K,2K` and `K` are connected end to end If cross sectional areas of three rods are equal If cross sectional areas of three rods are equal then equivalent thermal conductivity of the system is .
View SolutionThree rods of identical cross-sectional area and made from the same metal form the sides of an equilateral triangle `ABC` The points `A` and `B` are maintained at temperature `sqrt3 T` and `T` respectively In the steady state, the temperature of the point `C` is `T_(C)` Assuming that only heat conduction takes place, the value of `T_(C)//T` is equal to .
View Solutionhollow metal with side `0.5m` and wall thickess `5 xx 10^(-3)m` is filled with ice It is immersed in water tank maintained at `100^(@)C` Calculate the amount of ice melted in `335sex` (Conductivity of metal)
`= 0.5 W m^(-1) K^(-1)` Letent heat of fusion of ice `=335 xx 10^(3) Jkg^(-1))` .
View SolutionOne end of thermally insulated rod is kept at a temperature `T_(1)` and the other at `T_(2)`. The rod is composed of two section of length `l_(1)` and `l_(2)` thermal conductivities `k_(1)` and `k_(2)` respectively. The temerature at the interface of two section is
View SolutionThe temperature of a furnace is `2227^(@)C` and the intensity is maximum in its spectrum nearly at `12000 A^(@)` If the intensity in the spectrum of star is maximum nearly at `48000 A^(@)` then the surface temperature of the star is .
View SolutionBlack body at a temperature of `1640K` has the wavelength corresponding to maximum emission equal to `1.75mu m` Assuming the moon to be a perfectly black body the temperature of the moon if the wavelength corresponding to maximum emission is `14.35mum` is .
View SolutionA particular star (assuming it as a black body) has a surface temperature of about `5 xx 10^(4)K` The wave length in nano-meters at which its radiation becomes maximum is `(b = 0.0029mk)` .
View SolutionThe power radiated by a black body is 'P' and it radiates maximum energy around the wavelength `lmbda_(0)` If the temperature of the black body is now changed so that it raddiates maximum energy around a wavelength
`lambda_(0)//2` the power radiated becomes .
View SolutionThere is a temperature difference of `1K` between two black patches of skin on patient's chest and each patch having area A The radiant heat emitted from them is differ by `2%` then temperature of two patches may be .
View SolutionA black body radiates energy at the rate of `E "watt"//m^(2)` at a high temperature `TK` when the temperature is reduced to `[(T)/(2)]K` Then radiant energy is .
View SolutionThe radiant energy from the Sun incident normally at the surface of earth is `20kcal//m^(2)` min What would have been the radiant energy incident normally on the earth if the sun had a temperature twice of the present one ? .
View SolutionA star behaves like perfeact Black body emitting radiant energy The ratio of radiant energy emitted per sec by this star to that emitted by an another star having having `8times the radius of the former but having Kelvin temperature one fourth of the former is .
View SolutionTwo spherical bodies have radii `R,2R` and emissivities e,2e If the temperature ratio is `2:1` then the powers will be in the ratio .
View SolutionThe temperature of a body is increased by `50%`. The amount of radiation emitted by it would be nearly
View SolutionA solid sphere is at a temperature `T.K` The sphere is cut into two halves. The fraction of energy emitted per second by the half sphere th that by complete sphere is .
View SolutionA black metal foil is warmed by radiation from a small sphere at temperature `T` and at a distance d it is found that the power received by the foil is `P` If both the temperature and the distance are doubled the power received by the foil will be .
View SolutionPower radiated by a black body is `P_0` and the wavelength corresponding to maximum energy is around `lamda_0`, On changing the temperature of the black body, it was observed that the power radiated becames `(256)/(81)P_0`. The shift in wavelength corresponding to the maximum energy will be
View SolutionA body cools from `70^(@)C` to `50^(@)C` in 5minutes Temperature of surroundings is `20^(@)C` Its temperature after next `10 minutes is .
View SolutionThe bodies have thermal capacities in the ratio `3:4` and the rates of loss of heat in the ratio `3:5` Their rates of cooling will be in the ratio of .
View SolutionA calorimeter of water equivalent `6g` has water of mass `64g` up to a certain volume An other identical calorimeter has liquid of mass 5g and specific heat `0.6 calg^(@)C` upto same level If both pf them cool in the same surroundings through same range of temperature and the time taken for the water to cool is `140s` the time taken for the liquid to cool is .
View Solution
LEVEL-II(C.W) questions and complete solutions for chapter TRANSMISSION OF HEAT of NARAYANA PHYSICS (HINGLISH) of Class 11