Complete Solutions to Eexercies of chapter SIMPLE HARMONIC MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | SIMPLE HARMONIC MOTION |
| NN GHOSH | Eexercies |
| Number Of Questions | 39 |
| Academic Year | 2023-24 |
A particle moves with a simple harmonic motion. If the velocities at distance of 4cm and 5cm from the equilibrium position are 13 cm per second and 5 cm per second respectively, find the period and amplitude.
[Hint: Use the formula v=`omegasqrt(a^(2)-x^(2)).`]
View SolutionA point describes simle harmonic motion in a line 4 cm long. The velocity of the point while passing through the cente of the line is 12cm per second. Find the period.
[Hint: use formula v = `omegasqrt(a^(2)-a^(1))`]
View SolutionA particle moves with simple harmonic motion in a straight line. When the distances of the particle from the equilibrium position are `x_(1)` and `x_(2)`, the corresponding velocities are `u_(1)` and `u_(2)`. Find the period of the moton.
View SolutionThe maximum velocity of a body undergoing SHM is 0.004 m`s^(-1)` and its acceleration at 0.002 m from the mean position is 0.06 m`s^(-2)`. Find its amplitude and period of vibration.
[Hint: Use `v_(m) = aomega^(2)x`numerically.[
View SolutionA test tube of weight 5.6 g and external diameter 2.5 cm is allowed to float vertically in water by placing 10.4g of mercury at the bottom of the tube. The tube is depressed by a small amount and then released. Find the period of oscillation.
View SolutionFind the phase difference between two particles executing simple harmonic motion with the same frequency if they are found inn the states shown in the figures at four different points of time.
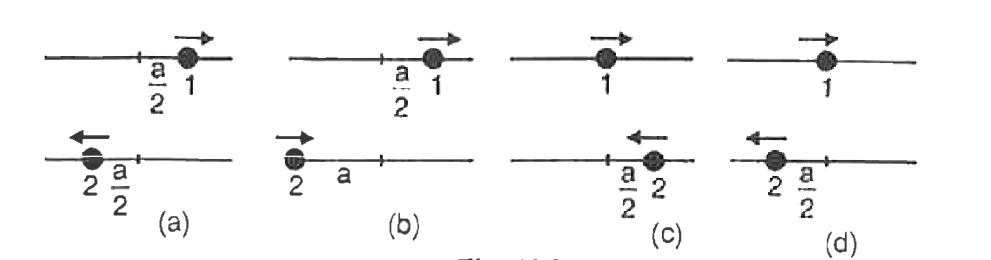
View SolutionA body of mass 10g executes SHM with amplitude `2 xx 10^(-2)` m and time period 2 s
Calculate the energy of the particle.
View SolutionA spring balance reading from 0 to 15kg is 10cm long. A body suspended from the spring is found to oscillate vertically with a frequency of 2 oscillations per second. How much does the body weigh?
View SolutionWhen the displacement is one-half the amplitude, what fraction of the total energy is kinetic and what fraction is potential? At what displacement is the energy half kinetic and half potential?
View SolutionA particle moves according to the equation f + 4x = 0, where x is its instaneous displacement and f its instaneous acceleration. The maximum value of x is `20 xx 10^(-2)` m. How much time will the particle take to move from x = 0.02 m to x = 0.08 m?
View SolutionAssuming that the atoms of a solid of atomic weight 24 behave like harmonic oscillators of frequency ` 3 xx 10^(15)hz`. Calculate their amplitude of vibration if the energy of a kg mole of the solid is `1.7 xx 10^(4)`joule.
View SolutionThe balance wheel of a watch vibrates with an angualr amplitude `pi` radians and a period of 0.5s. Find a) the maximum angular speed of the wheel, b) the angular speed of the wheel when its displacement is `pi/2` radians and c) the angular acceleration of the wheel when its displacement is `pi/4` radians.
[hint: use formulae of linear SHM for the angular SHM of the wheel]
View SolutionA block is on a piston, which executes simple harmonic motion in the vertical plane with a period of 1s. At what amplitude will the block and the piston separate? If the piston has an amplitude of 0.05m, what is the maximum frequency at which the black and piston will remain in contact continously?
[hint: the block and piston will separate when acceleration of piston = acceleration due to gravity.]
View SolutionA body of mass `m=0.50 kg `is suspended from a rubber cord with elasticity coefficient `k=50N//M`. Find the maximum distance over which the body can be pulled down for the body's oscillations to remain harmonic. What is the energy of oscillation in this case ?
View SolutionTwo springs of force constants `k_(1)` and `k_(2)` are joined together and the combination is attached to a mass m resting on a frictionless table at one end and clamped to a fixed support at the other. Show the frequency of oscillation of the mass is `f = 1/(2pi)sqrt((k_(1)k_(2))/((k_(1) + k-(2)))m`
[Hint: Let the mass be displaced by x and the clamped end of the second spring by `x^(')`. Then mf = `-k_(1)(x-x^('))`. Considering the first spring and the mass as a single system we have mf =`-k_(2)x^(')`]
View SolutionTwo springs of force constants `k_(1)` and `k_(2)` are attached to two opposite sides of a cube of mass m resting on a frictionless table and clamped to two supports at their other ends. Show that if the cube is displaced a little it will execute simple harmonic motion of frequency `f = 1/2pi sqrt((k_(1)+k_(2))/m`
View SolutionA point of mass m is suspended at the end of a massless wire of length l and cross-section A. If Y is the Young's modulus for the wire, obtain the frequency of oscillation for the simple harmonic motion along the vertical line.
[Hint: If x is the displacement of mass m from the equilibrium position then `Y = (stress)/(strain)= (F/A)/(x/l)` or `F = (YAx)/(l) and this force acts opposite to x. Now proceed as in example 3.
View SolutionTwo mass `m_(1)` and `m_(2)` are suspended from a massless spring of force constant k. When the masses are in equilibrium, `m_(1)` is removed without disturbing the system. Find the angular frequency and amplitude of oscillations.
View SolutionAn ideal gas enclosed in a vertical cylindrical container supports a freely moving piston of mass `M`. The piston and the cylinder have equal cross - section area `A`. When the piston is in equilibrium, the volume of the gas is `(V_0)` and the its pressure is `(P_0)`. The piston is slightly displaced from the equilibrium position and released. Assuming that the system is completely isolated from its surrounding, Show that the piston executes simple harmonic motion and find the frequency of oscillations.
View SolutionIf the mass of a spring, m, is not negligible but small compared to the mass M suspended from it, show that the period of oscillations is given by `T= 2pisqrt((M+m/3)//k)`, where k is the force constant of the spring.
[Hint: If x and v be the instaneous displacement and velocity of the lower end, then the displacement of an element at a distance z from the fixed end is xz/l and velocity zv/l. The kinetic energy of the spring = `(int_(0)^(t))1/2(mdz/l)z^(2)v^(2)/l^(2) = 1/6 mv^(2)`
Total energy of the system = `1/6mv^(2)+1/2Mv6(2) + 1/2kx^(2)` = a constant. Differentiate anmd find T.]
View SolutionA solid cylinder of mass `m` is attached to a horizontal spring with force constant `k`. The cylinder can roll without slipping along the horizontal plane. (See the accompanying figure.) Show that the center of mass of the cylinder executes simple harmonic motion with a period `T = 2pisqrt((3m)/(2k))`, if displaced from mean position.
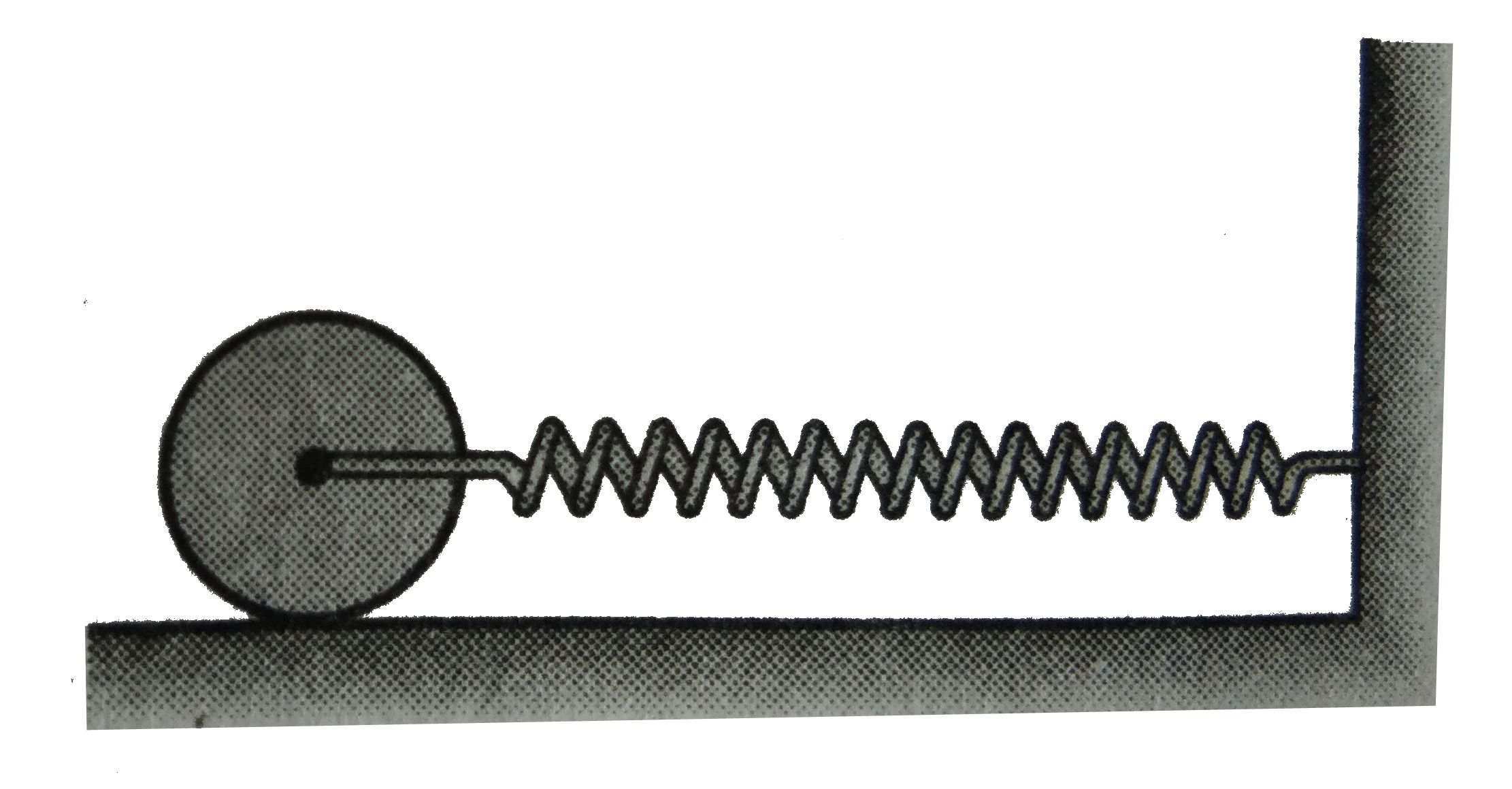
View SolutionA point executes SHM about a fixed point O. Its distance from O at a certain time is 1cm and 1second later its distance from O is 5cm. After yet another second is distance is again 5cm. Find the time taken for a complete oscillations.
View SolutionA plank rests symmetrically on two cylinders which are separated by a distance of 2l and rotate with uniform speed in opposite direction. If the plank is displaced a little and then released, show that the motion of the plank is simple harmonic. Calculate the period of oscillations of the peak. The co-efficient of friction between the plank and either cylinder is `mu`.
View SolutionA horizontal disc is oscillating in its own plane harmonically with amplitude a and period T. A body placed on the disc is about to slip on it. What is the coefficient of friction between the disc and the body?
View SolutionA body of mass m falls from a height h on to the pan of a spring balance, figure, The masses of the pan and spring are negligible. The spring constant of the spring is k. The body gets attached to the pan and starts executing S.H.M. in the vertical direction. Find the amplitude and energy of oscillation.
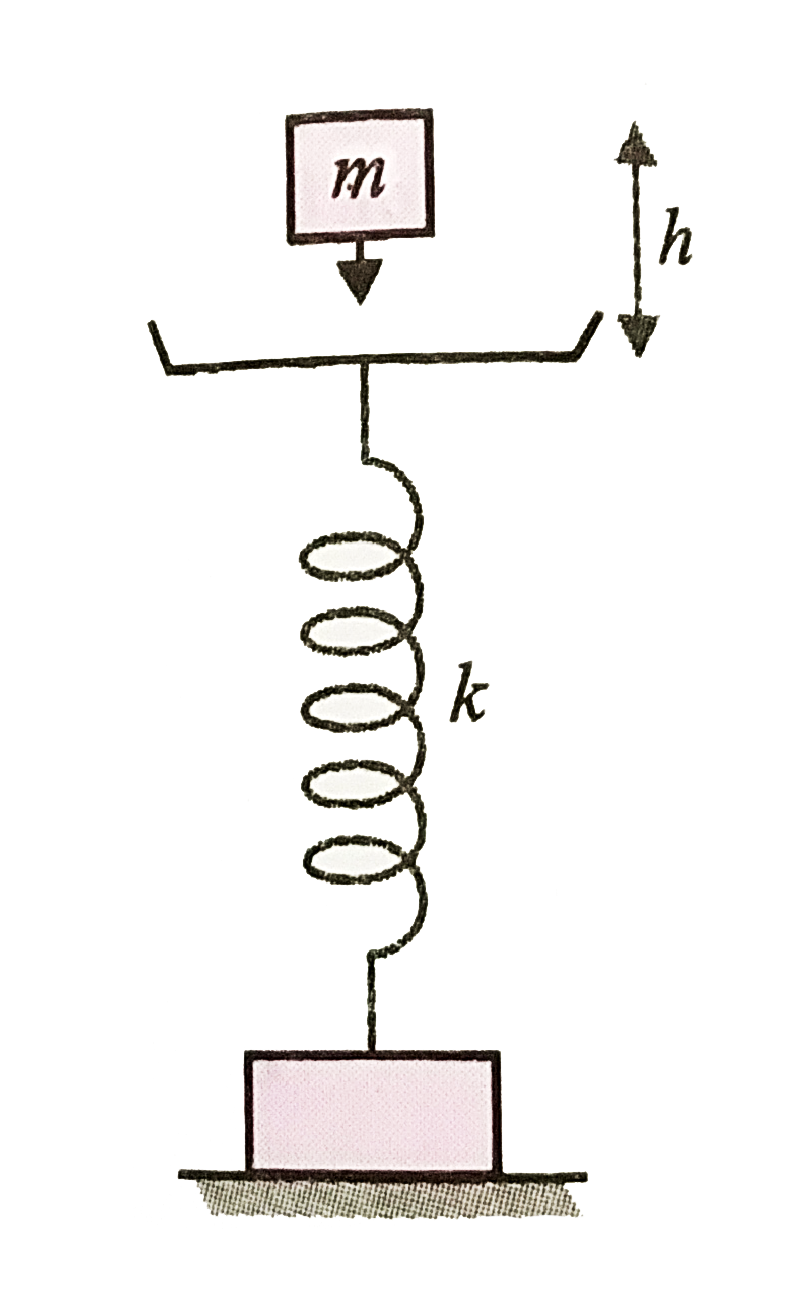
View SolutionA particle executes SHM with period T about a point O. It passes through a point P with velocity V along OP. Show that the time that elapses when it again comes to P is given by `t = (T/pi)tan^(-)(TV)/(2pi.OP)`
View SolutionTwo particles are in SHM with the same amplitude and frequency along the same line and about the same point. If the maximum separation between them is `sqrt(3)` times their amplitude, what is the phase difference between them?
View SolutionA small block of mass m resting at the bottom of a hemispherical cup of radius R is displaced a little and released. Determine the period of oscillations of the block, assuming the cup to be smooth and fixed rigidly to the table on which it rests. How will period change if the cup is free to move on the table, it is smooth and its mass is M?
View SolutionIf in the above problem the block is replaced by a small spherical ball of radius r and the cup is fixed, what will be the period of oscillations of the ball?
View SolutionA vertical U-tube has one of the its arms bent at `theta` from the vertical and then filled with a liquid of density `rho` up to a height h in the vertical arm. Calculate the period of oscillations of the liquid when disturbed a little and released.
View SolutionA block of mass M executes SHM with amplitude a and time period T. When it passes through the mean position, a lump of putty of mass m is dropped on it. Find the new amplitude and time period.
View SolutionA plank with a body of mass `m` places on it starts moving straight up according to the law `y=a(1-cos omegat t )`, whee `y` is the displacement from the initial position, `omega =11 s^(-1)`. Find :
(a) the time dependence of the force that the body exerts on the plank if `a=4.0cm,` plot this dependence,
`(b)` the minimum amplitude of oscillation of the plank at which the body starts falling behind the plank,
(c) the amplitude of oscillation of the plank at which the body springs up to a height `h=50cm` relative to the initial position (at the moment `t=0)`.
View SolutionA small sleeve of mass `m=1//10kg` can move along the diameter of a horizontal disc, which slides without friction along a guide rode. The sleeve is tied to the end of the rod with the aid of a massless spring whose force constant `k=10 N//m`. When the spring is not under tension, the sleeve is at the oscillations of the sleeve when the disc rotates about its axis at the angular speed `omega` equal to a) 6 rad/s, b) 15 rad/s.
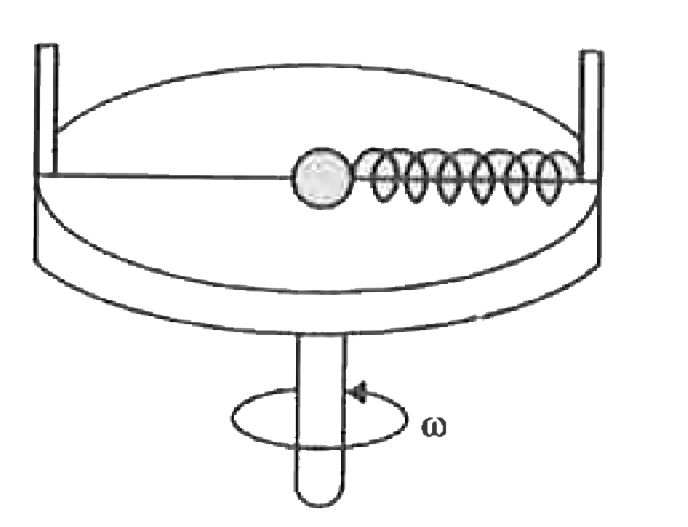
View SolutionA string of length l=1m and tensioned by a weight M = 4kg has two masses, each of mass = 10g, attached to it at distance `l/4` and `3/4` from one of the ends. Find the frequency of oscillations of the masses in the two cases.
a) When the masses are displaced equally in the same direction b) when the masses are displaced equally in opposite directions.
View SolutionA block of mass `m_(1)=1kg` is attached to a spring of force constant `k=24N//cm` at one end and attached to a string tensioned by mass `m_(2)=5kg` . Dedcue the frequency of oscillaitons of the system. If `m_(2)` is initially supported in hand and then suddenly released, find

`(a)` instantaneous tension just after `m_(2)` is released . ltbr. `(b)` the maximum displacement of `m_(1)`.
`(c)` the maximum and minimum tensions in the string during oscillations.
View SolutionA rubber cord of force constant k = 100 N/m and l=1m is attached to a particle of mass m = 1kg at one end and fixed to a vertical wall at the other. The body is displaced by `x_(0)` = 25cm so as to stretch the cord and then released. Calculate the time particle takes to reach the wall.
View SolutionFind the frequency of oscillations of the system in the figure. The bar is rigid and light.
[Hint: A spring of force constant `k_(1)` at a distance a is equivalent to a spring of force constant `k_(1)(a/b)o^(2)` at a distance b)
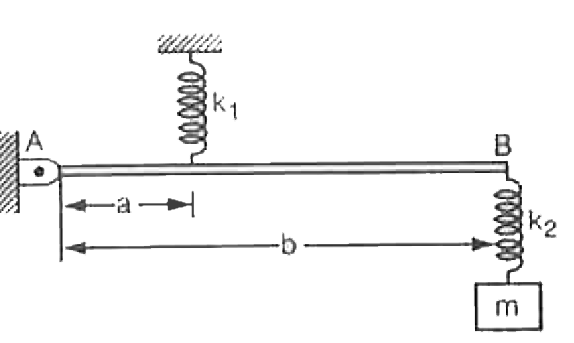
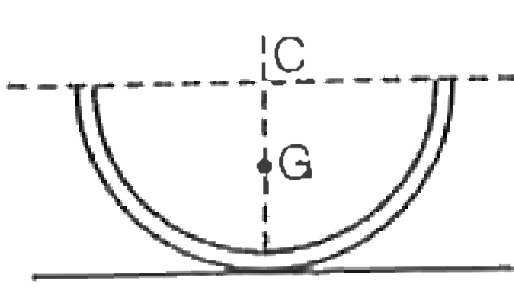
View SolutionA pipe in the form of a half ring is placed on a horizontal surface. If it is rotated through a small angle, and then released, assuming that it rolls without sliding determine the period of oscillations. The center of gravity of such a body is at distance `(2r)/pi)` from the center where r is the radius of the ring.
Hint: `(vec_(c) = alpha rhat(i)` and
`vec(a_(c)) = vec(a_(c)) +(veca_(G//c))_("tangent") +(veca_(G//c))_("normal")`
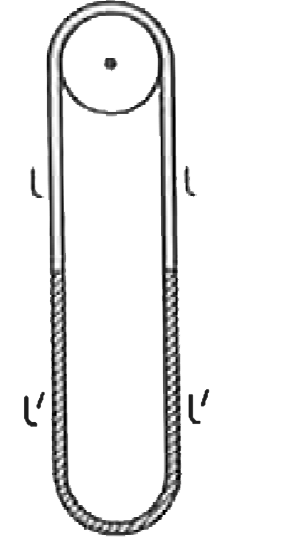
View SolutionAn endless cord consists of lengths 2l and `2l^(')` and their masses per unit length are m and `m^(')`. It is placed in stable equilibrium over a smooth peg as shown in the figure. It is slightly displaced and left to itself. Show that motion is simple harmonic. Find the period of oscilattions.
View Solution
Eexercies questions and complete solutions for chapter SIMPLE HARMONIC MOTION of BHARATI BHAWAN PHYSICS (HINGLISH) of Class 11