Complete Solutions to Unsolved Numerical of chapter RIGID BODIES AND ROTATIONAL MOTION of Class 11 book with complete answers and questions
| Class 11 | PHYSICS |
| Chapter | RIGID BODIES AND ROTATIONAL MOTION |
| PHYSICS GALAXY | Unsolved Numerical |
| Number Of Questions | 63 |
| Academic Year | 2023-24 |
Two masses M and m are connect by a light string gong over a pulley of radis r. The pulley is free to rotate about its axis which is kept horizontal. The moment of inertia of the pulley about the axis is I. The system is releaed from rest. Find the angular momentum fo teh system when teh mass Mhas descended through a height h. The string does not slip over the pulley.
View SolutionA ball of radius `R=10.0cm` rolls without slipping down an inclined plane so that its centre moves with constant acceleration
`w=2.50cm//s^2`, `t=2.00s` after the beginning of motion its position corresponds to that shown in figure. Find:
(a) the velocities of the points A, B, and O,
(b) the accelerations of these points.

View SolutionA body of radius `R` and mass `m` is rolling smoothly with speed `v` on a horizontal surface. It then rolls up a hill to a maximum height `h`. If `h = 3 v^2//4g`. What might the body be ?
View SolutionA conical pendulum, a thin uniform rod of length `l` and mass `m`, rotates uniformly about a vertical axis with angular velocity `omega`(the upper end of the rod is hinged). Find the angle `theta` between the rod and the vertical.
View SolutionA point A is located on the rim of a wheel of radius R which rolls witout slipping along a horizontal surface witih velocity V. Find the total distance traversed by the point A between successive moments at which it touches the surface.
View SolutionA spool (consider it as a double disc system joined by a short tube at their centre) is placed on a horizontal surface as shown Fig. A light string wound several times over the short connecting tube leaves it tangentially and passes over a light pulley. A weight of mass `m` is attached to the end of the string. The radius of the connecting tube is r and mass of the spool is `M` and radius is `R`. Find the acceleration of the falling mass `m`. Neglect the mass of the connecting tube and slipping of the spool.

View SolutionA midpoint of a thin uniform rod AB of mass `m` and length `l` is rigidly fixed to a rotation axle `OO^'` as shown in figure. The rod is set into rotation with a constant angular velocity `omega`. Find the resultant moment of the centrifugal forces of inertia relative to the point C in the reference frame fixed to the axle `OO^'` and to the end.
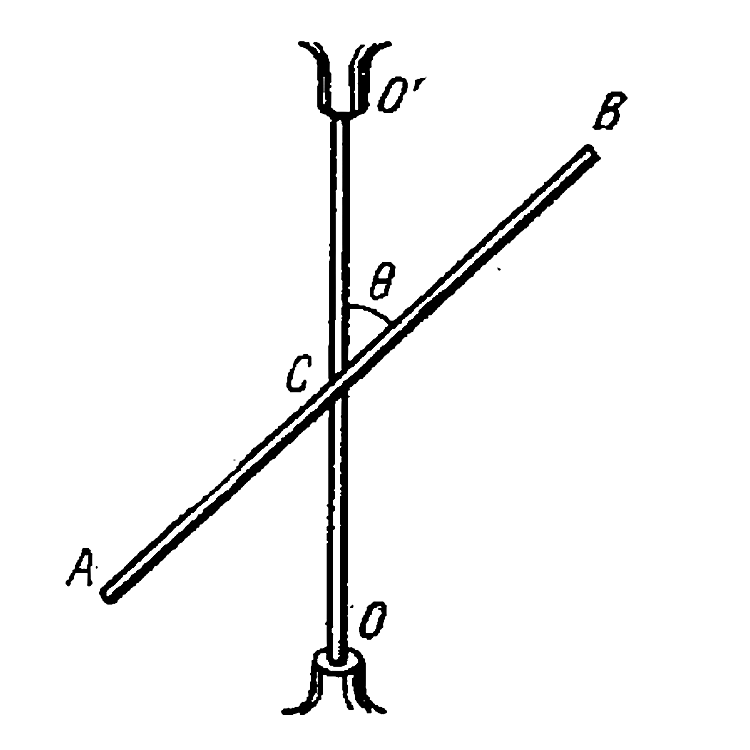
View SolutionA particle is projected at time t=0 from a point P on the ground with a speed `v_0,` at an angle of `45^@` to the horizontal. Find the magnitude and direction of the angular momentum of the particle about P at tiem `t= v_0//g`
View SolutionWhat is the angular momentum of the seconds hand on a clock about an axis through the centre ofthe clock face if the clock hand has a length of 25 cm and amass of 15gm ?
View SolutionA solid uniform sphere, with radius R = 0.2 m and mass M=50kg,is at rest in an inertial reference frame in deep space. A bullet with mass w = 20 gm and a velocity v= 400 m/s strikes the sphere along the line shownin figufe-5.137, and rapidly comes to rest within the sphere at point P. Determine the subsequent motion of the sphere and the embedded bullet.
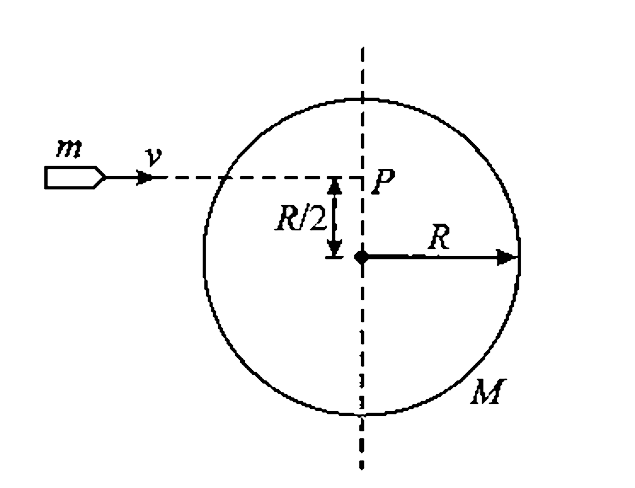
View SolutionIn a spring gun, a spring of force constant 200N/mis compressed 0.15 m. When fired, 80% of the elastic potential energy stored in the spring is eventually converted into kinetic energy of a 0.1 kg uniform ball that is rolling without slipping at the base of a ramp. The ball continues to roll without slipping up the ramp with 90% of the kinetic energy at the bottom converted into an increase in gravitational potential energy at the instant it stops.
(a) What is the speed of the ball's centre ofmass at the base of the ramp
(b) At this position, what is the speed of a point at the top of the ball?
(c ) What maximum vertical height up the ramp does the ball move?
View SolutionA diver makes `2.5` revolutions on the way from a `10-m-high` platform to the water. Assuming zero initial vertical velocity, the average angular velocity during the dive is.
View Solution(a) Compute the torque developed by an automotive engine whose output is 180 kW at an angular velocity of 4000rev/min. (b)A drum of negligible mass, 0.min diameter, is attached to the motorshaft,and the powerout put of the motor is used to raise a weight hanging from a rope wrapped around the drum. How large a weight can be liftd? Assume contant speed(c) With with what speed will the weigh rise?
View Solution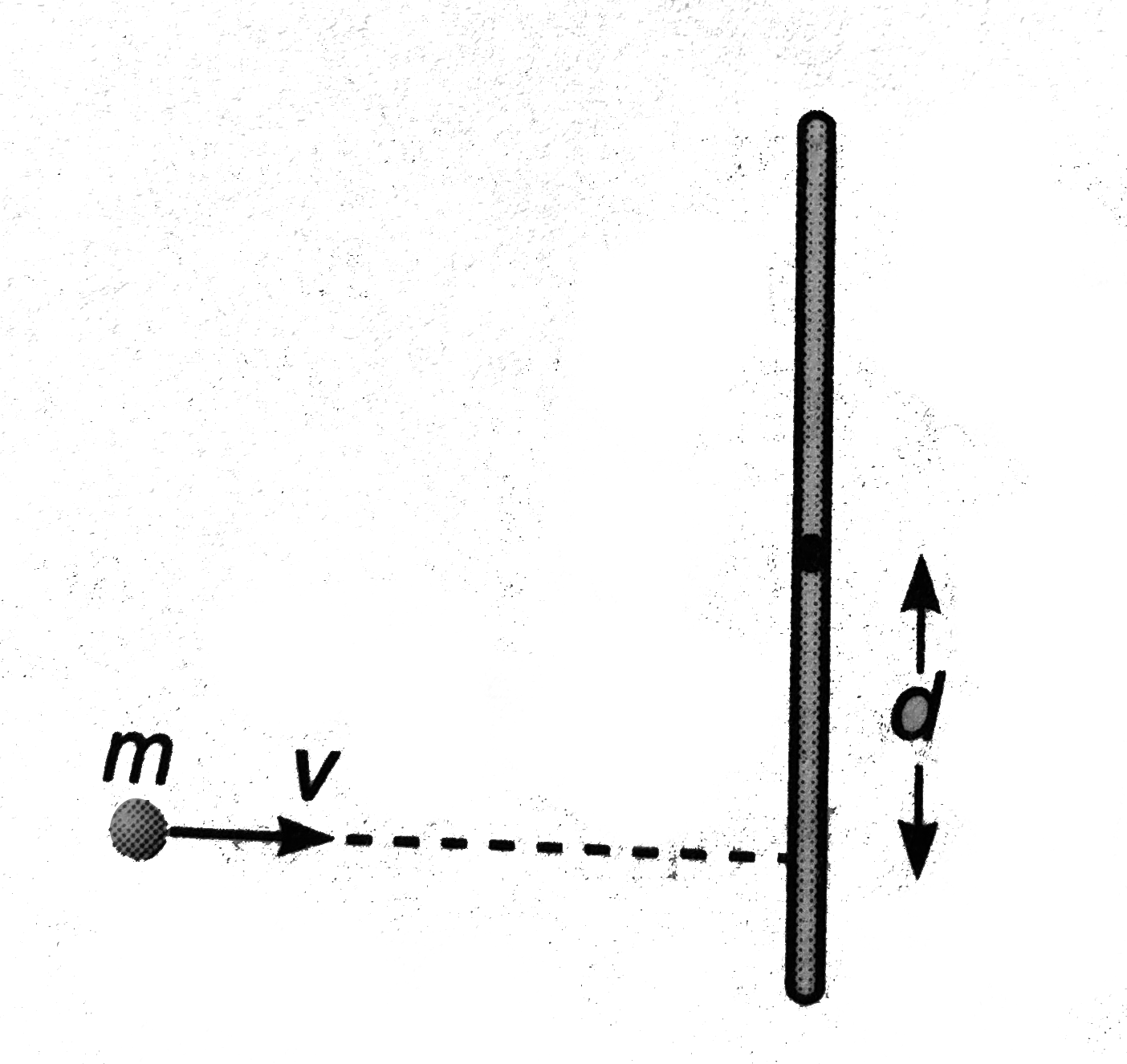
A stick of length `l` lies on horizontal table. It has a mass `M` and is free to move in any way on the table. A ball of mass `m` moving perpendicularly to the stick at a distance d from its centre with speed `v` collides elastically with it as shown in figure. What quantities are conserved in the collision ? what must be the mass of the ball, so that it remains at rest immediately after collision?
View SolutionA small steel sphere of mass m and radius r rolls without slipping on the fiictionless surface of a large hemisphere of radius R{R gt gt r) whose axis of symmetry is vertical. It starts at the top from the rest, (a) What is the kinetic energy at the bottom ? (b) What fraction is the rotational kinetic energy of the total kinetic energy at the bottom? (b) What fraction is the rotational kinetic energy of the total kinetic energy? (c) What fraction is the translational kinetic energy of the total kinetic energy? (d) Calculate the normal force that the small sphere will exerton.the hemisphere at its bottom. Howthe results will be affected if r is not very sjnall as compared to R.
View SolutionThe carbide tips of the cutting teeth of a circulars aware 9.2 cm from the axis ofrotation, (a) The no-load speed of the saw,whenit is not cutting anything, is 5000 rev/min.Why is its no-load power output negligible ? (b) While cutting lumber, the angular speed of the saw slows to 2500 rev/min, and the power output is 2.1 hp. What is the tangential force that the wood exerts on the carbide tips.
View SolutionA uniform rod ofmassm and length / rests on a smooth horizontal surface. One of the ends ofthe rod is struck in'a horizontal direction at right angles to the rod. As a result the rod obtains velocity Vq. Findtheforcewithwhich onehalfof the rod will act on the other in the process ofmotion.
View SolutionA smooth uniform rod AB of mass M and length l rotates freely with an angular velocity `omega_0` in a horizontal plane about a stationary vertical axis passing through its end A. A small sleeve of mass m starts sliding along the rod from the point A. Find the velocity `v^'` of the sleeve relative to the rod at the moment it reaches its other and B.
View SolutionThe fly wheel of a large motorin a factory has mass 30kg and moment of inertia `67.5 kg-m^(2)` about its rotation axis. The motor develops a constanttorque of 600N-m, and the fly wheel starts from rest, (a) What is the angular acceleration of the fly wheel ? (b) What is its angular velocity after making 4 revolutions ? (c) How much work is done by the motor during the first 4 revolutions ? (d) What is the average power output ofthe motor during the first 4 revolutions ?
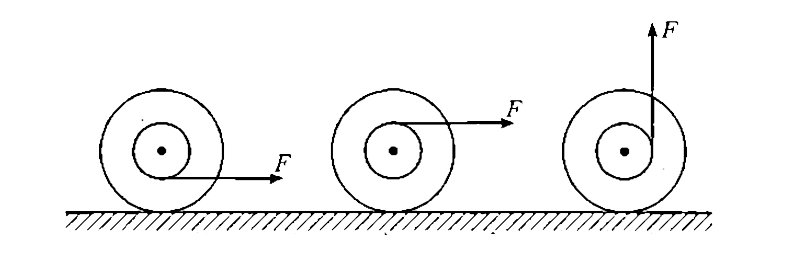
View SolutionFigure-5.139 shows three identical kiting spools at rest on a rough horizontal groundinitially.In each case the string is pulled in the direction shown in figure. In each case it is given that spool rolls without slipping. In what direction will each spoolwill move and with what acceleration. Moment ofinertia ofeach spool is 7and radius of innertube is r and that ofouter disc is R.
View SolutionA closed system consists of two particles of masses `m_1` and `m_2` which move at right angles to each other with velocities `v_1` and `v_2`. Find:
(a) the momentum of each particle and
(b) the total kinetic energy of the two particles in the reference frame fixed to their centre of inertia.
View SolutionTwo uniform thin rods A and B of length 0.6 m each and of masses 0.01 kg and 0.02kg respectively are rigidly joined end to end. The combination is pivoted at the lighter end, P as shown in fig. Such that it can freely rotate about point P in a vertical plane. A small object of mass 0.05kg, moving horizontally, hits the lower end of the combination and sticks to it what should be the velocity of the object so that the system could just be reised to the horizontal position.
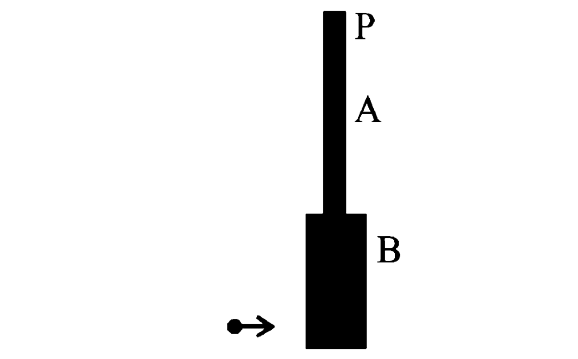
View SolutionA insect of mass m stands on a horizontal disc platform of moment of inertia 1 which is at rest. What is the angular velocity of the disc platform when the insect goes along a circle of radius r, concentric with the disc, with velocity v relative to the disc.
View SolutionA uniform rod AB of mass M is placed in contact. with a second rod BC of mass m = M/2 on a horizontal smooth table at right angles to each other as shown in figure-5.141. Find the initial velocity of BC and kinetic energy generated in it ifan impulse 7 is imparted to
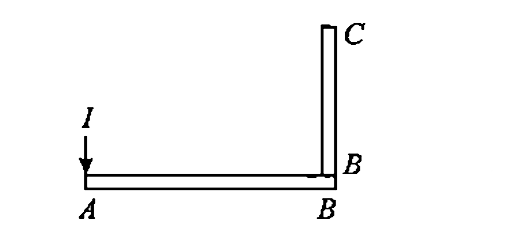
View SolutionA uniform thin rod of mass m and length L is standing vertically along the y-axis on a smooth horizontal surface, with its lower end at the origin (0,0). A slight disturbane at t = 0 causes the lower end t slip on the smooth surface along the positive x-axis, and the rod starts falling.
(i) What is the path followed by the centr of mass of the rod during its fall?
(ii) Find the equaiton to the trajectory of a point on the rod shape of the path of this point?
View SolutionA carpet of mass `M` is rolled along its length so as to from a cylinder of radius `R` and is kept on a rough floor. When a negligibly small push is given to the cylindrical carpet, it stars unrolling itself without sliding on the floor. Calculate horizontal velocity of cylindrical part of the carpet when its radius reduces to `R//2`.
View SolutionA small sphere of radius R is held against the inner surface of larger sphere of radius `6R` (as shown in figure). The masses of large and small spheres are `4M` and M respectivley. This arrangement is placed on a horizontal table. There is no friction between any surfaces of contact. The small sphere is now released. Find the coordinates of the centre of the large spheres, when the smaller sphere reaches the other extreme position.

View SolutionA vertically oriented uniform rod of mass M and length l can rotate about its upper end. A horizontally flying bullet of mass m strikes the lower end of the rod and gets stuck in it, as a result, the rod swings through an angle `alpha`. Assuming that `m lt lt M`, find:
(a) the velocity of the flying bullet,
(b) the momentum increment in the system "bullet-rod" during the impact, what causes the change of that momentum,
(c) at what distance x from the upper end of the rod the bullet must strike for the momentum of the system "bullet-rod" to remain constant during the impact.
View SolutionA Stick of length L and mass M lies on a fnctionless horizontal surface on which it is free to move in any way. A ball of mass m moving with speed v collides elastically with the stick as shownin figure-5.115. If after the collision ball comes to rest, then what should be the mass of the ball ?

View SolutionA uniform solid cylinder of radius `R=15cm` rolls over a horizontal plane passing into an inclined plane forming an angle `alpha=30^@` with the horizontal(figure). Find the maximum value of the velocity `v_0` which still permits the cylinder to roll onto the inclined plane section without a jump. The sliding is assumed to be absent.

View SolutionA small spherical marble of mass m and radius r is rolling without slipping on a rough track with speed v.The track further is in the shape of a vertical circle of radius R as shown in figure-5.144. With what minimum linear speed the marble is rolling so that it completely goes round the circle on the circular part of track.
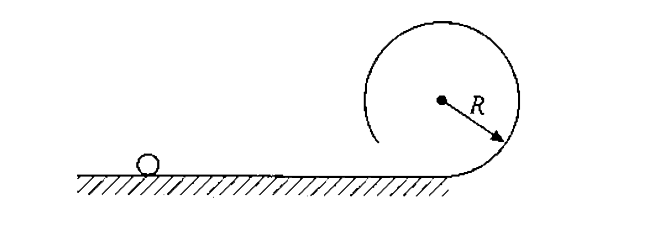
View SolutionA horizontal rotating disc placed on arough surface has an angular speed of 8 rad`//`s when it is lowered on the surface. After 3 seconds, it is observed to have an angular speed of 2.6 rad`//`s. How many revolutions it made from the time of lowering`on the surface until it stops ? Assume the pressure on the surface due to disc is uniform on its area.
View SolutionA thin horizontal uniform rod `AB` of mass `m` and length `l` can rotate freely about a vertical axis passing through its end `A`. At a certain moment, the end `B` starts experiencing constant force `F` which is always perpendicular to the original position of the stationary rod and directed in a horizontal plane. Find the angular velocity of the rod as a function of its rotation angle `phi` counted relative to the initial position.
View SolutionA disc of circumference S stands vertically on a horizontal surface as shown in figure-5.145, A horizontal force P acts on the centre of the disc. Half of the circumference {ABC) is rough and the friction is sufficient to prevent slipping when the disc rolls along X¥.The other half of the circumference (ADC) is smooth. The disc starts from rest when P begins to act and the point C is at the bottom. Find the distance moved by the disc along xy when it completes one rotation.
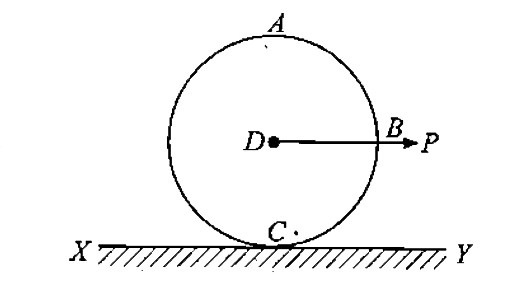
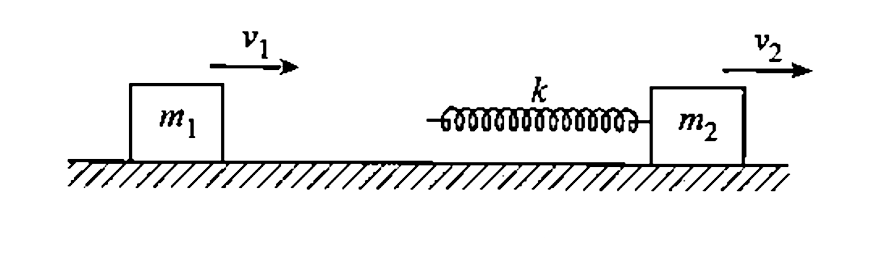
View SolutionA block of mass `m_(1)` =2 kg slides along a firictionless table with a speed of 10m`//`s. Directly infront of it,and moving in the same direction is a block of mass `m_(2)` 5 kg moving at 3 m`//`s. A mass less spring with a spring constant 11.2 N`//`cm is attached to as shown in figure-5.146. When the block collide,what is the maximum compression of the spring ? Assume that the spring does not bend.
View SolutionA uniform rod of length 2a is held with one end resting on a smooth horizontal table making an angle a with the vertical. Show that when the rod is released, its angular velocity when it makes an angle `theta` with the vertical is given by
`omega=[(6g(cosalpha-costheta))/((1+3sin^(2)alpha))]`
View SolutionA hollow sphere of radius r is rotating about a horizontal axis at some angular speed `omega_(0)`. It is gently lowered to ground and the coefficient of friction between sphere and the ground is `mu`.How far does the sphere move before it starts pure rolling?
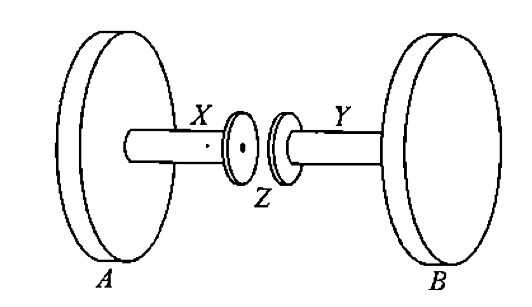
View SolutionDiscs A and B are mounted on a shaft XY and may be connected or disconnected by a coupling Z as shown in figure-5.147. The moment of inertia of disc A about the shaft is half that of disc B. The moments of inertia of the shaft and the coupling are negligible. Initially A is disconnected and rotated at an angular velocity `omega`. It is now coupled to disc B using couplings.It is found that 5000 J of thermal energy is developed in the coupling when the connection is made. What was the original kinetic energy of the disc A.
View SolutionA spool with thread wound on it, of mass m, rests on a rough horizontal surface. Its moment of inertia relative to its own axis is equal to `I=gammamR^2`, where `gamma` is a numerical factor, and R is the outside radius of the spool. The radius of the wound thread layer is equal to r. The spool is pulled without sliding by the thread with a constant force F directed at an angle `alpha` to the horizontal (figure). Find:
(a) the projection of the acceleration vector of the spool axis on the x-axis,
(b) the work performed by the force F during the first t seconds after the beginning of motion.

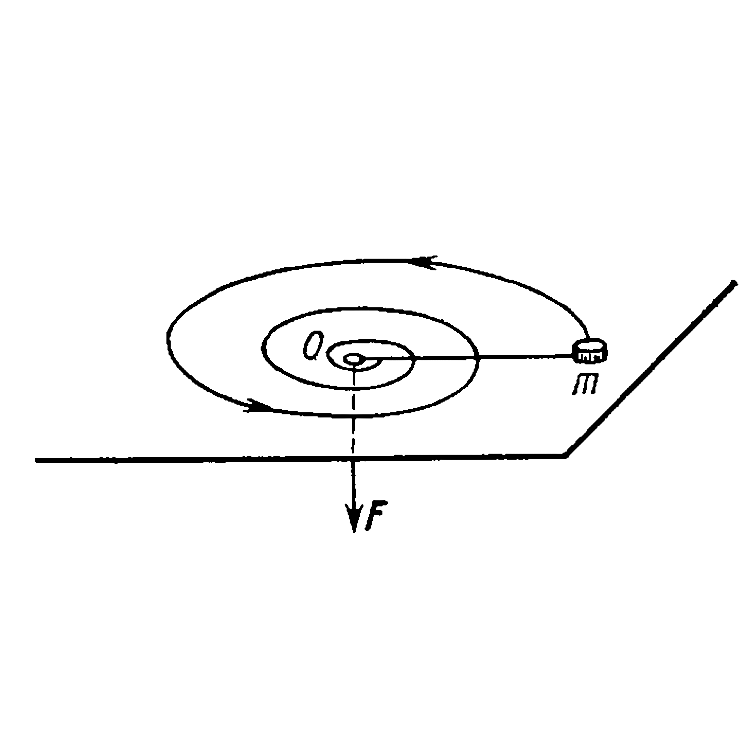
View SolutionA small body of mass m tied to a non-stretchable thread moves over a smooth horizontal plane. The other end of the thread is being drawn into a hole O(figure) with a constant velocity. Find the thread tension as a function of the distance r between the body and the hole if at `r=r_0` the angular velocity of the thread is equal to `omega_0`.
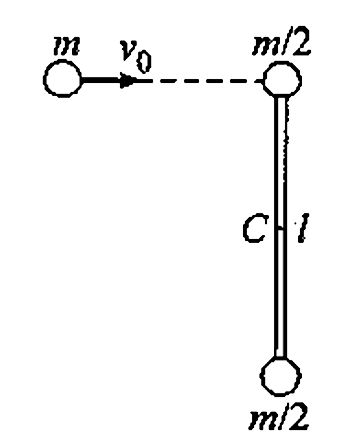
View SolutionA ball ofmass m moving with velocity experiences a head on elastic collision with one of the spheres of as tationary rigid dumbbell as shown in figure-5.149. The mass of each sphere equals m`//`2, and the distance between them is l.Disregarding the size of the spheres, find the angular momentum of the dumbbell in the reference frame moving translationally and fixed to the centre of mass of the dumbbell.
View SolutionA spool has a mass of 2 kg,an inner radius `R_(1)` =3cm,and an outer radius `R_(2)= "5cm, the radius of gyration about the axis of the spool is if" =4cm`.A constant horizontal force of 5N is applied to the free end of a massless thread that is wrapped around the inner cylinder of the spool as shownin figure-5.150. If the spool rolls without slipping, calculate the linear acceleration along the horizontal surface. What is the minimum coefficient of static friction required to prevent slipping ?
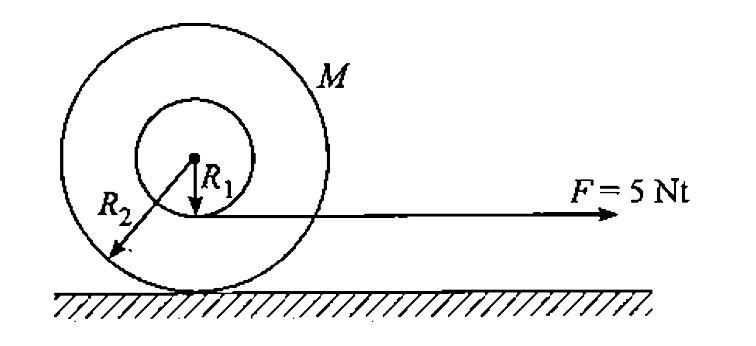
View SolutionA homogeneous disc of weight W and radius R rotates about the vertical axis OZ, the initial angular velocity being `omega_(0)`. During the motion, the brake block A is pressed to the disc,with a radial force N for `t_(0)` seconds and the disc comes to rest because of friction. Find the coefficient of friction.
View SolutionA uniform disc of mass m and radius R is projected horizontally with velocity `v_(0)` on a rough horizontal floor so that it starts with a purely sliding motion at t= 0. After `t_(0)` seconds it acquires a purely rolling motion.
(a) Calculate the velocity of the centre of mass of the disc at `t_(0)`
(b) Assuming the coefficient of friction to be `mu`, calculate to. Also calculate the work done by the frictional force as a function of time and the total work done by it over a time t muchlonger than `t_(0)`.
View SolutionA uniform rod `AB` of mass `m = 2 kg` and length `l = 1.0 m` is placed on a sharp support `P` such that `a = 0.4 m` and `b = 0.6 m`. A. spring of force constant `k= 600 N//m` is attached to end `B` as shown in Fig. To keep the rod horizontal, its end `A` is tied with a thread such that the spring is `B` elongated by `1 CM`. Calculate reaction of support `P` when the thread is burnt.
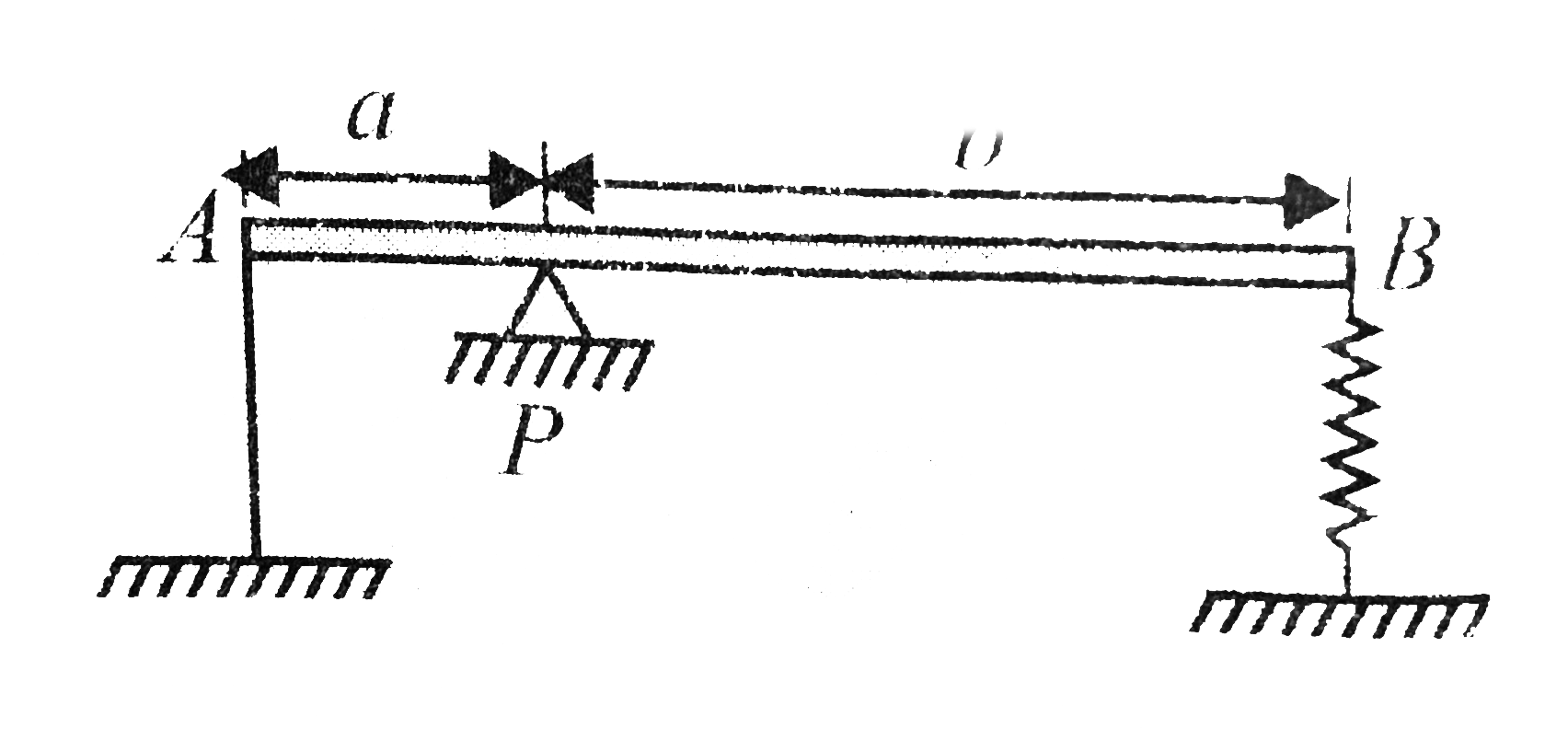
View SolutionA small ball is suspended from a point O by a light thread of length l. Then the ball is drawn aside so that thread deviates through an angle `theta` from the vertical and set in motion in a horizontal direction at right angles to the vertical plane in which the thread is located. What is the initial velocity that has to be imparted to the ball so that it could deviate through the maximum angle `pi//2` in the process of motion?
View SolutionAn object rotates about a fixed axis, such that a reference line on the object makes an angle `theta=ae^(bt)` with its starting position at time t. Find for a particle on object at a distance r from the axis of rotation, the tangential, the radial and the total acceleration of the point.
View SolutionA rectangular rigid fixed block has a long horizontal edge. A solid homogeneous cylinder of radus R is placed horizontally at rest its length parallel to the edge such that the exis of the cylinder and the endg of the block are in the same vertical plane as shown in the figure below. Ther is sufficinet friction present at the edge s that a very small displacement causes the cylinder to roll off the edge without slipping. Determine:
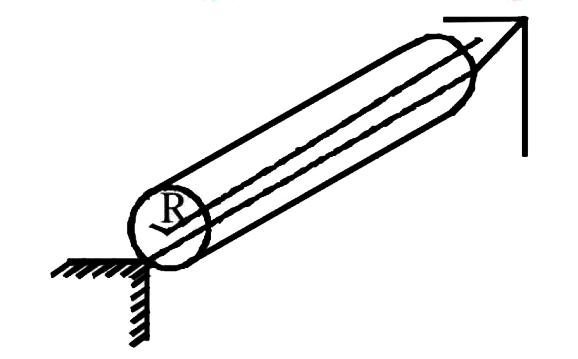
(a) the angle `theta_c` through which the cylinder rotates before it leaves contact with the edge,
(b) the speed of the centre of mass of the cylinder before leaving contact with the edge, and
(c) the ratio of the translational to rotational kinetic energy of the cylinder when its centre of mass is in horizontal line with the edge.
View SolutionA 392 N wheel comes off a moving truck and rolls without slipping along a highway. At the bottom of a hill it is rotating at 50 rad`//`s. The radius ofthewheel is 0.6m, and its moment of inertia about its rotation axis is `0.8MR^(2)`. Friction does 3000 J of work on the wheel as it rolls up the hill to a stop a height h above the bottom of the hill. Calculate h.
View SolutionA grindstone in the form of a solid cylinder has a radius of 0.2m and a mass of 30 kg. (a)What constant torque will bring it from rest to an angular velocity of 250 rev`//`min in 10 s ?
(b) Through what angle has it turned during that time ?
(c) Calculate the work done by the torque.
View SolutionA constant net torque equal to 20 N-m is exerted on a pivoted wheel for 8 sec, during which time the angular velocity of the wheel increases from zero to 100 re`//`min. The external torque is then removed and the wheel is brought to rest by friction in its bearings in 70 sec. Compute (a) the moment of inertia of the wheel about the rotation axis, (b) the friction torque(c)thetotal no. of revolutions made by the wheel in the70 sec time interval
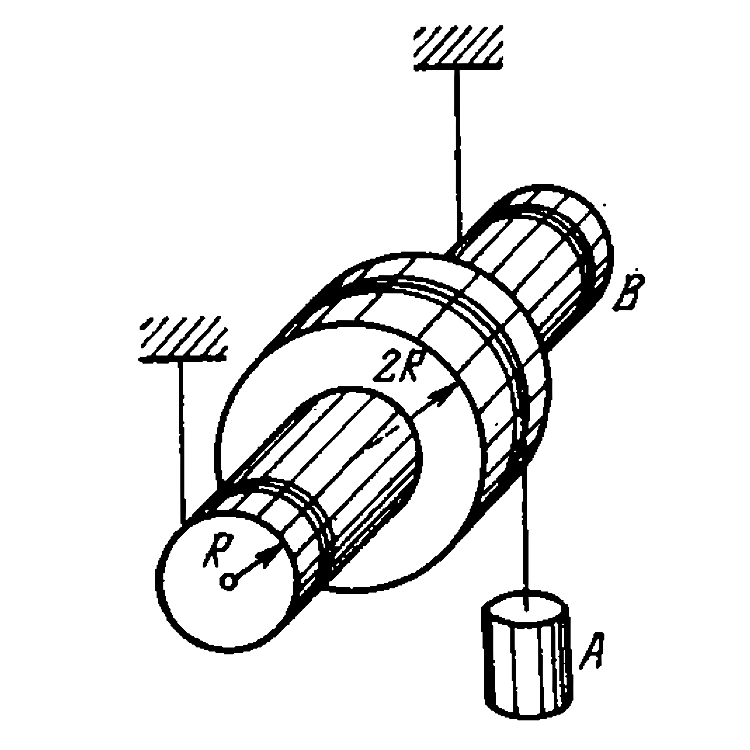
View SolutionIn the arrangement shown in figure a weight A possesses mass m, a pulley B possesses mass M. Also known are the moment of inertia I of the pulley relative to its axis and the radii of the pulley R and `2R`. The mass of the threads is negligible. Find the acceleration of the weight A after the system is set free.
View SolutionA solid spherical ball of radius 30 cm and mass M is connected to a point A on wall with a thread and freely rotate about its central axis with angular velocity 60 rad`//`sec. If the ball is resting onthe vertical face of a wall, what time will elapse before it comes to rest ? The coefficient of friction between wall and ball is 0.25 and inclination of thread to vertical is `15^(@)`.
View SolutionA thin uniform rod of mass m and length l rotates with the constant angular velocity `omega` about the vertical axis passing through the rod's suspension point O. In doing so, the rod describes a conical surface with a half separated angle `theta`.Find the angle `theta` as well as the magnitude and direction of the reaction force at the point O.
View SolutionA rod of mass m and length l is held vertically on a smooth horizontal floor. Now it is released from this position, find the speed of its centre of mass when it makes an angle `theta` with the vertical.
View Solution
Consider a cylinder of mass `M` and radius `R` lying on a rough horizontal plane. It has a plank lying on its top as shown in figure. A force F is applied on the plank such that the plank moves and causes the cylinder to roll the plank always remains horizontal. there is no slipping at any point of contact. Calculate the acceleration of the cylinder and the frictional forces at the two contact.
View SolutionA uniform rod of length `1 m` and mass `2 kg` is suspended. Calculate tension `T` (in `N`) in the string at the instant when the right string snaps `(g = 10 m//s^(2))`.

View SolutionThe mechanism shown in figure-5.156 is used to raise a wooden box of mass 50kg .A string is wrapped around a cylinder that turns on an axle.The cylinder has radius 0.25 m and moment of inertia 0.92 `kg-m^(2)` about the axle. What magnitude of the force F applied tangentially to the rotating crank handle is required to raise the box with an acceleration of 0.80m`//`s.Here we can neglect the moment of inertia of the axle and the crank
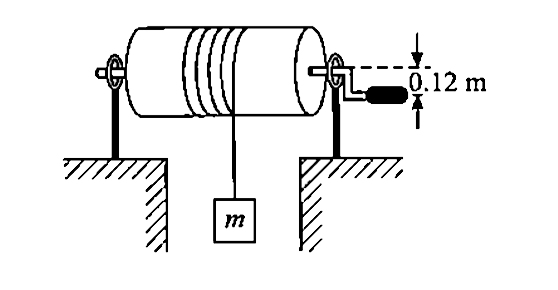
View SolutionA uniform spherical shell of mass `M` and radius `R` rotates, about a vertical axis on frictionless bearing. A massless cord passes around the equator of the shell., over a pulley of rotational inertia `I` and radius `r` and is attached to small object of mass `m` that is otherwise free to fall under the influence of gravity. There is no friction of pulley's axle, the cord does not slip on the pulley. What is the speed of the object after it has fallen a distance `h`. from rest? Use work-energy considerations.

View SolutionShow that if a rod held at angle `theta` to the horizontal and released, its lower end will not slip if the friction coefficient between rod and ground is greater than `(3sinthetacostheta)/(1+3sin^(2)theta)`
View SolutionTwo wooden disc , one with radius 2 cm and mass 1 kg and the other with radius 4 cm and mass 2 kg , are welded together coaxially and mounted on a frictionless axis through their common centre. Alight string is wrapped around the edge of the smaller disc, and a 3 kg block is suspended from the free end of the string.What is the acceleration of the block after it is released? Repeat the above process if the stringi swrapped around the edge of the larger disc.
View SolutionA horizontal wooden disc of mass 8 kg and diameter 1m is pivoted on frictionless bearing about a vertical axis through its centre.We put a toy train track model in the disc.The track has a negligible mass and average diameter 0.95 ra.The mass ofmodel train is 1.2 kg which can run with a battery.When we switch on the engine the train moves anticlockwise, soon attaining a constant speed of 0.6 m`//`s with respect to the track. Find the magnitude and direction of the angular velocity of the disc relative to the earth.
View SolutionA 50 kg runner runs around the edge of a turn table mounted on frictionless bearings. With respect to earth the velocity of runner is 2m`//`s.The turntable is rotating in opposite direction with an angular velocity of magnitude 0.2 rad`//`s with respect to earth.The radius of turntable is 4m, and its moment of inertia is 1000 `kg-m^(2)`. Find the final angular velocity of the system if runner comes to rest relative to turntable.
View Solution
Unsolved Numerical questions and complete solutions for chapter RIGID BODIES AND ROTATIONAL MOTION of GK PUBLICATIONS PHYSICS (HINGLISH) of Class 11