Complete Solutions to Archives Subjective of chapter CHEMICAL KINETICS of Class 12 book with complete answers and questions
| Class 12 | CHEMISTRY |
| Chapter | CHEMICAL KINETICS |
| CENGAGE CHEMISTRY | Archives Subjective |
| Number Of Questions | 23 |
| Academic Year | 2023-24 |
Rate of a reaction `A + B rarr` Product, is given as a function of different initial concentration of `A` and `B`.
`|{:([A] (mol L^(-1)),(B) (mol L^(-1)),"Initial rate" (mol L^(-1) min^(-1)),),(0.01,0.01,0.005,),(0.02,0.01,0.010,),(0.01,0.02,0.005,):}|`
Determine the order of the reaction with respect to `A` and with respect to `B`. What is the half life of `A` in the reaction ?05:45View SolutionA first order reaction is `20%` complete in `10 min`. Calculate (a) the specific rate constant of the reaction and (b) the time taken for the reaction to reach `75%` completion.
01:59View SolutionWhile studying the decompoistion of gaseous `N_(2)O_(5)`, it is observed that a plot of logarithm of its partial pressure versus time is linear. What kinetic parameters can be obtained form this observation?
05:04View SolutionA first order gas reaction has `k = 1.5 xx 10^(-6) s^(-1)` at `200^(@)C`. If the reaction is allowed to run for `10 h`, what have changed in the Product? What is the half-life of this reaction?
03:17View SolutionA first order reaction is `50%` completed in `30 min` at `27^(@)C` and in `10 min` at `47^(@)C`. Calculate the reaction rate constants at `27^(@)C` and the energy of activation of the reaction in `kJ mol^(-1)`.
03:34View SolutionIn a Arrhenius equation for a certain reaction, the values of `A and E_(a)` ( energy of activation)are `4xx10^(13)s^(-1)` and `98.6KJ mol^(-1)`, respectively. If the reaction of first order at, what temperature will its life periof be `10 min`.
03:52View SolutionThe decomposition of `N_(2)O_(5)` according to the equation: `2N_(2)O_(5)(g) to 4NO_(2)(g) + O_(2)(g)` is a first order reaction. After 30 min. from the start of the decomposition in a closed vessel, the total pressure developed is found to be 284.5 mm of Hg and on complete decomposition, the total pressure is 584.5 mm of Hg. Calculate the rate constant for the reaction.
10:57View SolutionTwo reaction, `(I)A rarr` Products and `(II) B rarr` Products, follow first order kinetics. The rate of reaction `(I)` is doubled when the temperature is raised form `300 K` to `310K`. The half life for this reaction at `310K` is `30 min`. At the same temperature `B` decomposes twice as fast as `A`. If the energy of activation for reaction `(II)` is twice that of reaction `(I)`, (a) calculate the rate of constant of reaction `(II)` at `300 K`.
13:32View SolutionThe gas phase decomposition of dimethyl ether follows first order kinetics.
`CH_(3)-O-CH_(3)(g)rarrCH_(4)(g)+CO(g)`
The reaction is carried out in a constant volume container at `500^(@)C` and has a half life of `14.5 min`. Initially, only dimethyl ether is present at a pressure `0.40 atm`. What is the total pressure of the system after `12min`? (Assume ideal gas behaviour)04:25View SolutionA first order reaction `A rarr B` requires activation energy of `70 kJ mol^(-1)`. When a `20%` solution of `A` was kept at `25^(@)C` for `20 min`, `25%` decomposition took place. What will be the percentage decomposition in the same time in a `30%` solution maintained at `40^(@)C` ? (Assume that activation energy remains constant in this range of temperature)
09:11View Solutionform the following data for the reaction between `A` and `B`,

(a) Calculate the order of the reaction with respect to `A` and with respect to `B`.
(b) Calculate the rate constant at `300 K`.
( c) Calculate the pre-expontential factor.15:35View SolutionAt `380^(@)C` , the half-life periof for the first order decompoistion of `H_(2)O_(2)` is `360 min`. The energy of activation of the reaction is `200 kJ mol^(-1)`. Calculate the time required for `75%` decompoistion at `450^(@)C`.
05:34View SolutionThe ionization constant of `overset(o+)(NH_(4))` ion in water is `5.6 xx 10^(-10)` at `25^(@)C`. The rate constant the reaction of `overset(o+)NH_(4)` and `overset(ɵ)(OH)` ion to form `NH_(3)` and `H_(2)O` at `25^(@)C` is `3.4 xx 10^(10)L mol^(-1) s^(-1)`. Calculate the rate constant for proton transfer form water to `NH_(3)`.
04:14View SolutionThe time required for `10%` completion of a first order reaction at `298 K` is equal to that required for its `25%` completion at `308 K`. If the pre-exponential factor for the reaction is `3.56 xx 10^(9) s^(-1)`, calculate its rate constant at `318 K` and also the energy of activation.
07:54View SolutionThe rate constant for the first order decompoistion of a certain reaction is described by the equation
`log k (s^(-1)) = 14.34 - (1.25 xx 10^(4)K)/(T)`
(a) What is the energy of activation for the reaction?
(b) At what temperature will its half-life periof be `256 min`?04:26View SolutionFor the equation
`N_(2)O_(5)(g)=2NO_(2)(g)+(1//2)O_(2)(g)`, calculate the mole fraction of `N_(2)O_(5)(g)` decomposed at a constant volume and temperature, if the initial pressure is `600 mm Hg` and the pressure at any time is `960 mm Hg`. Assume ideal gas behaviour.03:38View SolutionThe rate constant of a reaction is `1.5 xx 10^(7)s^(-1)` at `50^(@)C` and `4.5 xx 10^(7) s^(-1)` at `100^(@)C`. Evaluate the Arrhenius parameters `A` and `E_(a)`.
04:11View SolutionThe rate constant for an isomerization reaction, `A rarr B` is `4.5 xx 10^(-3) min^(-1)`. If the initial concentration of `A` is `1 M`, calculate the rate of the reaction after `1 h`.
02:40View SolutionA hydrogenation reaction is carried out at `500` K. If the same reaction is carried out in the presence of a catalyst at the same rate, the temperature required is `400` K. Calculate the activation energy of the reaction if the catalyst lowers the activation barrier by `20` kJ `mol^(-1)`.
06:27View SolutionThe vapour pressure of two miscible liquids `(A)` and `(B)` are `300` and `500 mm` of `Hg` respectively. In a flask `10` mole of `(A)` is mixed with `12` mole of (B). However, as soon as `(B)` is added, `(A)` starts polymerising into a completely insoluble solid. The polymerisation follows first-order kinetics. After `100` minute, `0.525` mole of a solute is dissolved whivh arrests the polymerisation completely. The final vapour pressure of the solution is `400 mm` of `Hg`. Estimate the rate constant of the polymerisation reaction. Assume negligible volume change on mixing and polymerisation and ideal behaviour for the final solution.
03:41View SolutionThe rate of first order reaction is `0.04 mol L^(-1) s^(-1)` at `10 min` and `0.03 mol L^(-1) s^(-1)` at `20 min` after initiation. Find the half life of the reaction.
04:31View SolutionFor the given reaction
`A+B rarr` Products,
the following data are given.
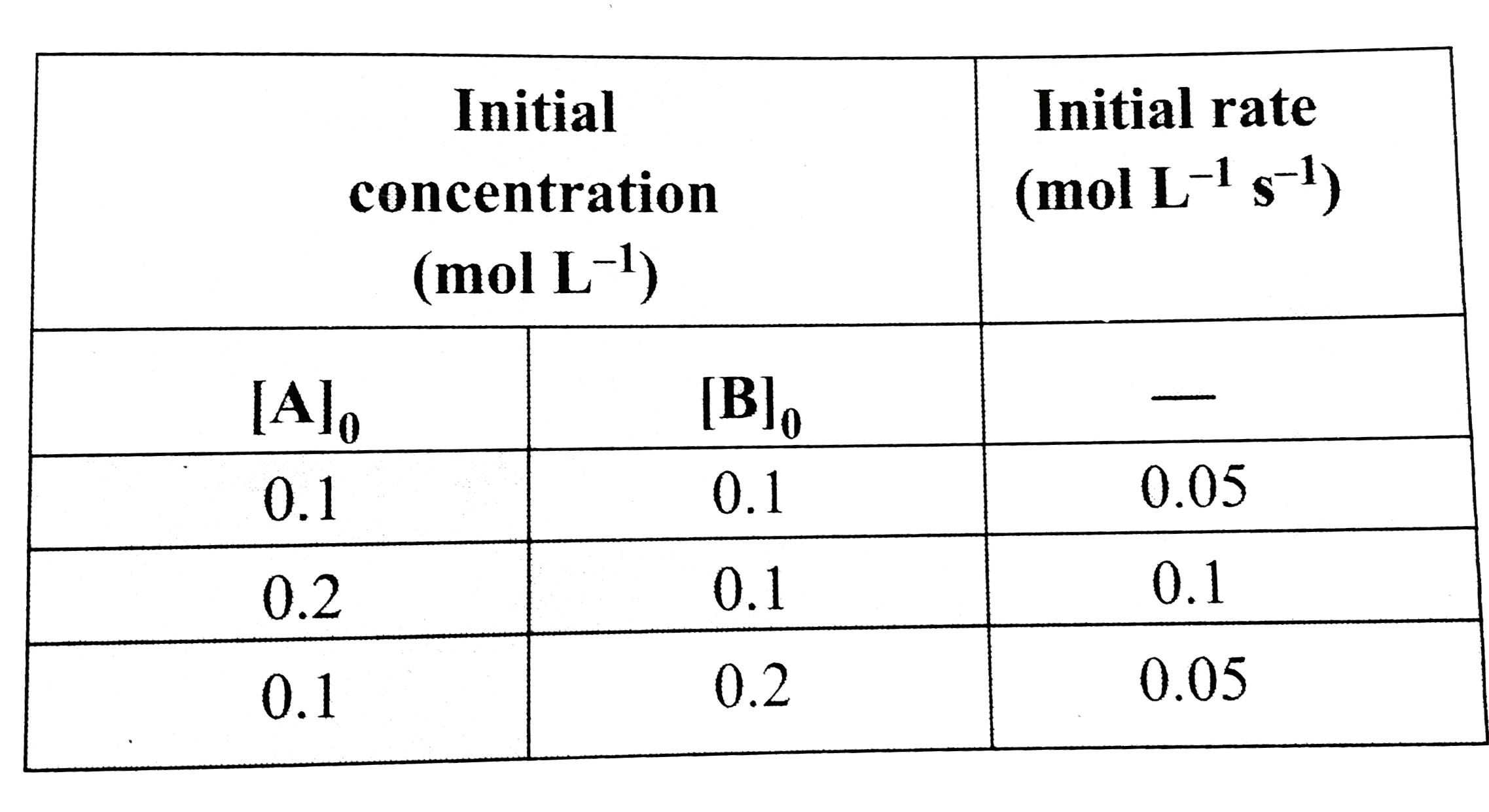
(a) Write the rate equation.
(b) Calculate the rate constant.05:07View Solution`2X(g) rarr 3Y(g)+2Z(g)`
`{:("Time (in min)",0,100,200,):}`
`|{:("Partial pressure of X (mm Hg)",800,400,200,),("Assuming ideal gas condition, calculate",,,,):}|`
(a) Order of reaction
(b) Rate constant
( c) Time taken for `75%` completion of reaction
(d) Total pressure when `p_(x) = 700 mm`05:23View Solution
Archives Subjective questions and complete solutions for chapter CHEMICAL KINETICS of CENGAGE CHEMISTRY (HINGLISH) of Class 12
All Exercises from CENGAGE CHEMISTRY Class 12 CHEMISTRY CHEMICAL KINETICS
- Archives Fill In The Blanks
- Archives Integer
- Archives Multiple Correct
- Archives Single Correct
- Archives Subjective
- Archives True/False
- Ex 4.1 (Objective)
- Ex 4.2 (Objective)
- Ex 4.3 (Objective)
- Ex 4.3 More Than One Correct
- Ex4.4 Objective
- Exercises Assertion-Reasoning
- Exercises Fill In The Blanks
- Exercises Integer
- Exercises Linked Comprehension
- Exercises Multiple Correct
- Exercises Single Correct
- Exercises True/False
- Illustration
- Solved Example
