Complete Solutions to Linked comprehension type of chapter MONOTONICITY AND MAXIMA MINIMA OF FUNCTIONS of Class 12 book with complete answers and questions

`f(x) = sin^(-1)x+x^(2)-3x + (x^(3))/(3),x in[0,1]`

which of the following is true for `x in [0,1]`?

Let`f'(sin x)lt0 and f''(sin x) gt0 forall x in (0,(pi)/(2))` and g(x) =f(sinx)+f(cosx)
which of the following is true?

Let`f'(sin x)lt0 and f''(sin x) gt0 forall x in (0,(pi)/(2))` and g(x) =f(sinx)+f(cosx)
which of the following is true?

Let`f'(sin x)lt0 and f''(sin x) gt0 forall x in (0,(pi)/(2))` and g(x) =f(sinx)+f(cosx)
which of the following is true?

Let`f'(sin x)lt0 and f''(sin x) gt0 forall x in (0,(pi)/(2))` and g(x) =f(sinx)+f(cosx)
If x = 3 is the only point of minima in its neighborhood and x=4 is neither a point of maxima nor a point minima, then which of the following can be true?

Let`f'(sin x)lt0 and f''(sin x) gt0 forall x in (0,(pi)/(2))` and g(x) =f(sinx)+f(cosx)
If x = 3 is the only point of minima in its neighborhood and x=4 is neither a point of maxima nor a point minima, then which of the following can be true?

Let`f'(sin x)lt0 and f''(sin x) gt0 forall x in (0,(pi)/(2))` and g(x) =f(sinx)+f(cosx)
If x = 3 is the only point of minima in its neighborhood and x=4 is neither a point of maxima nor a point minima, then which of the following can be true?

If `phi` (x) is a differentiable real valued function satisfying `phi (x) +2phi le 1`, then it can be adjucted as `e^(2x)phi(x)+2e^(2x)phi(x)lee^(2x) or (d)/(dx)(e^(2)phi(x)-(e^(2x))/(2))le or (d)/(dx)e^(2x)(phi(x)-(1)/(2))le0`
Here `e^(2x)` is called integrating factor which helps in creating single differential coefficeint as shown above. Answer the following question:
If p(1)=0 and `dP(x)/(dx)ltP(x)` for all `xge1` then

If H `(x_(0))=0` for some `x=x_(0)and (d)/(dx)H(x)gt2cxH(x)` for all `xgex_(0),` where `cgt0,` then prove that H (x) cannot be zero for any `xgtx_(0).`

Let `h(x) =f(x)-a(f(x))^(3)` for every real number x
h(x) increase as f(x) increses for all real values of x if

Let `h(x) =f(x)-a(f(x))^(3)` for every real number x
h(x) increase as f(x) increses for all real values of x if

If f(x) is strictly increasing function then h(x) is non monotonic function given

Let `f(x)=x^(3)-9x^(2)+24x+c=0` have three real and distinct roots `alpha, beta` and `lambda`.
(i) Find the possible values of c.
(ii) If `[alpha]+[beta]+[lambda]=8`, then find the values of c, where `[*]` represents the greatest integer function.
(ii) If `[alpha]+[beta]+[lambda]=7`, then find the values of c, where `[*]` represents the greatest integer function.s

Let `f(x)=x^(3)-9x^(2)+24x+c=0` have three real and distinct roots `alpha, beta` and `lambda`.
(i) Find the possible values of c.
(ii) If `[alpha]+[beta]+[lambda]=8`, then find the values of c, where `[*]` represents the greatest integer function.
(ii) If `[alpha]+[beta]+[lambda]=7`, then find the values of c, where `[*]` represents the greatest integer function.s

Let `f(x)=x^(3)-9x^(2)+24x+c=0` have three real and distinct roots `alpha, beta` and `lambda`.
(i) Find the possible values of c.
(ii) If `[alpha]+[beta]+[lambda]=8`, then find the values of c, where `[*]` represents the greatest integer function.
(ii) If `[alpha]+[beta]+[lambda]=7`, then find the values of c, where `[*]` represents the greatest integer function.s

consider the graph of y=g(x)=f'(x) given that f(c ) =0, where y=f(x) is a polynomial funtion

The graph of y=f(x) will intersect the x axis

Consider the graph of `y=g(x)=f'(x)` given that `f(c) =0,` where `y=f(x)` is a polynomial function

The equation `f(x)=0,alexleb`,has

consider the graph of y=g(x)=f'(x) given that f(c ) =0, where y=f(x) is a polynomial funtion

The graph of y =`f(x),alexleb` has

consider the graph of y=g(x)=f'(x) given that f(c ) =0, where y=f(x) is a polynomial funtion

The function y = `f(x),alexleb`,has

consider the graph of y=g(x)=f'(x) given that f(c ) =0, where y=f(x) is a polynomial funtion

The equation f''(x)=0

Let f(x) = `4x^(2)-4ax+a^(2)-2a+2` and the golbal minimum value of f(x) for x in [0,2] is equal to 3
The number of values of a for which the global minimum value equal to 3 for x in [0,2] occurs at the endpoint of interval[0,2] is

Let f(x) = `4x^(2)-4ax+a^(2)-2a+2` and the golbal minimum value of f(x) for x in [0,2] is equal to 3
The number of values of a for which the global minimum value equal to 3 for x in [0,2] occurs at the endpoint of interval[0,2] is

Let f(x) = `4x^(2)-4ax+a^(2)-2a+2` and the golbal minimum value of f(x) for x in [0,2] is equal to 3
The number of values of a for which the global minimum value equal to 3 for x in [0,2] occurs at the endpoint of interval[0,2] is

Let f(x) =`x^(3)-3(7-a)X^(2)-3(9-a^(2))x+2`
The values of parameter a if f(x) has a negative point of local minimum are

Let f(x) =`x^(3)-3(7-a)X^(2)-3(9-a^(2))x+2`
The values of parameter a if f(x) has a positive point of local maxima are

Let f(x) =`x^(3)-3(7-a)x^(2)-3(9-a^(2))x+2`
The values of parameter a if f(x) has points of extrema which are opposite in sign are

consider the function f(x) =`1(1+(1)/(x))^(x)`
The domain of f(x) is

consider the function f(x) =`1(1+(1)/(x))^(x)`
The domain of f(x) is

consider the function f(x) =`(1+(1/x))^(x)`
The range of the function f(x) is

consider the function f(X) =x+cosx
which of the following is not true about y =f(x)?

consider the function f(X) =x+cosx -a
values of a which f(X) =0 has exactly one positive root are

consider the function f(X) =x+cosx -a
values of a for which f(X) =0 has exactly one negative root are

consider the function f(X) =`3x^(4)+4x^(3)-12x^(2)`
Y= f(X) increase in the inerval

consider the function f(X) =`3x^(4)+4x^(3)-12x^(2)`
The range of the function y=f(x) is

consider the function f(X) =`3x^(4)+4x^(3)-12x^(2)`
The range of values of a for which f(x) = a has no real

consider the function `f:R rarr R,f(x)=(x^(2)-6x+4)/(x^(2)+2x+4)`
f(x) is

consider the function `f:R rarr R,f(x)=(x^(2)-6x+4)/(x^(2)+2x+4)`
which of the following is not true about f(x)?

consider the function `f:R rarr R,f(x)=(x^(2)-6x+4)/(x^(2)+2x+4)`
Range of fX() is

Consider a polynomial y = P(x) of the least degree passing through A(-1,1) and whose graph has two points of inflection B(1,2) and C with abscissa 0 at which the curve is inclined to the positive axis of abscissa at an angle of `sec^(-1)sqrt(2)`
The value of P(2) ius

Consider a polynomial y = P(x) of the least degree passing through A(-1,1) and whose graph has two points of inflection B(1,2) and C with abscissa 0 at which the curve is inclined to the positive axis of abscissa at an angle of `sec^(-1)sqrt(2)`
The value of P(0) is

Consider a polynomial y = P(x) of the least degree passing through A(-1,1) and whose graph has two points of inflection B(1,2) and C with abscissa 0 at which the curve is inclined to the positive axis of abscissa at an angle of `sec^(-1)sqrt(2)`
The equation P(x) =0 has

Let f(X) be real valued continous funcion on R defined as f(X) =`x^(2)e^(-|x|)`
The values of k for which the equation `x^(2)e^(-|x|)`=k has four real roots are

Let `f(x) `be real valued continous funcion on R defined as `f(x)` =`x^(2)e^(-|x|)`
Number of points of inflection for `y = f(x)` is (a) 1 (b) 2 (c) 3 (d) 4

Let `f(x) `be real valued continous funcion on R defined as `f(x)` =`x^(2)e^(-|x|)`
Number of points of inflection for `y = f(x)` is (a) 1 (b) 2 (c) 3 (d) 4

P(x) be a polynomial of degree 3 satisfying P(-1) =10 , P(1) =-6 and p(x) has maxima at x = -1 and p(x) has minima at x=1 then The value of P(2) is (a) -15 (b) -16 (c) -17 (d) -22 (c) -17 (d) -22

P(x) be a polynomial of degree 3 satisfying P(-1) =10 , P(1) =-6 and p(x) has maxima at x = -1 and p(x) has minima at x=1 then The value of P(1) is

The graph of y =g(x) =f(X) is as shown in the following figure analyse this graph and answer the following question

The graph of y=f(x) for `altxltb`has

The graph of y =g(x) =f(X) is as shown in the following figure analyse this graph and answer the following question

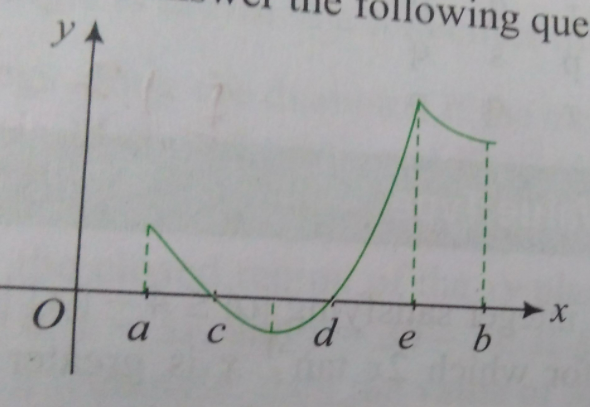
Number of points of inflectionn the graph of y = f(x) for `alt xlt b` has

The graph of y =g(x) =f(X) is as shown in the following figure analyse this graph and answer the following question

Which of the following is not true about the graph of y = f(x) ,`a lt x lt b`