Complete Solutions to Exercise 2.2 of chapter ELECTRIC FLUX AND GAUSS LAW of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ELECTRIC FLUX AND GAUSS LAW |
| CENGAGE PHYSICS | Exercise 2.2 |
| Number Of Questions | 14 |
| Academic Year | 2023-24 |
A ball of charge q is placed in a hollow conducting uncharged sphere. After this, the sphere is connected with earth for a short time and the ball is then removed from the sphere. The ball has not been brought into contact with the sphere.
a. What charge will the sphere have after these operations? Where and how will this charge be distributed?
b. What will be the electric field inside as well as outside the sphere?
View Solutionfigure shows the field produced by two point charges +q and -q of equal magnitude but opposite signs (an electric dipole). Find the electric flux through each of the closed surfaces A,B,C, and D.

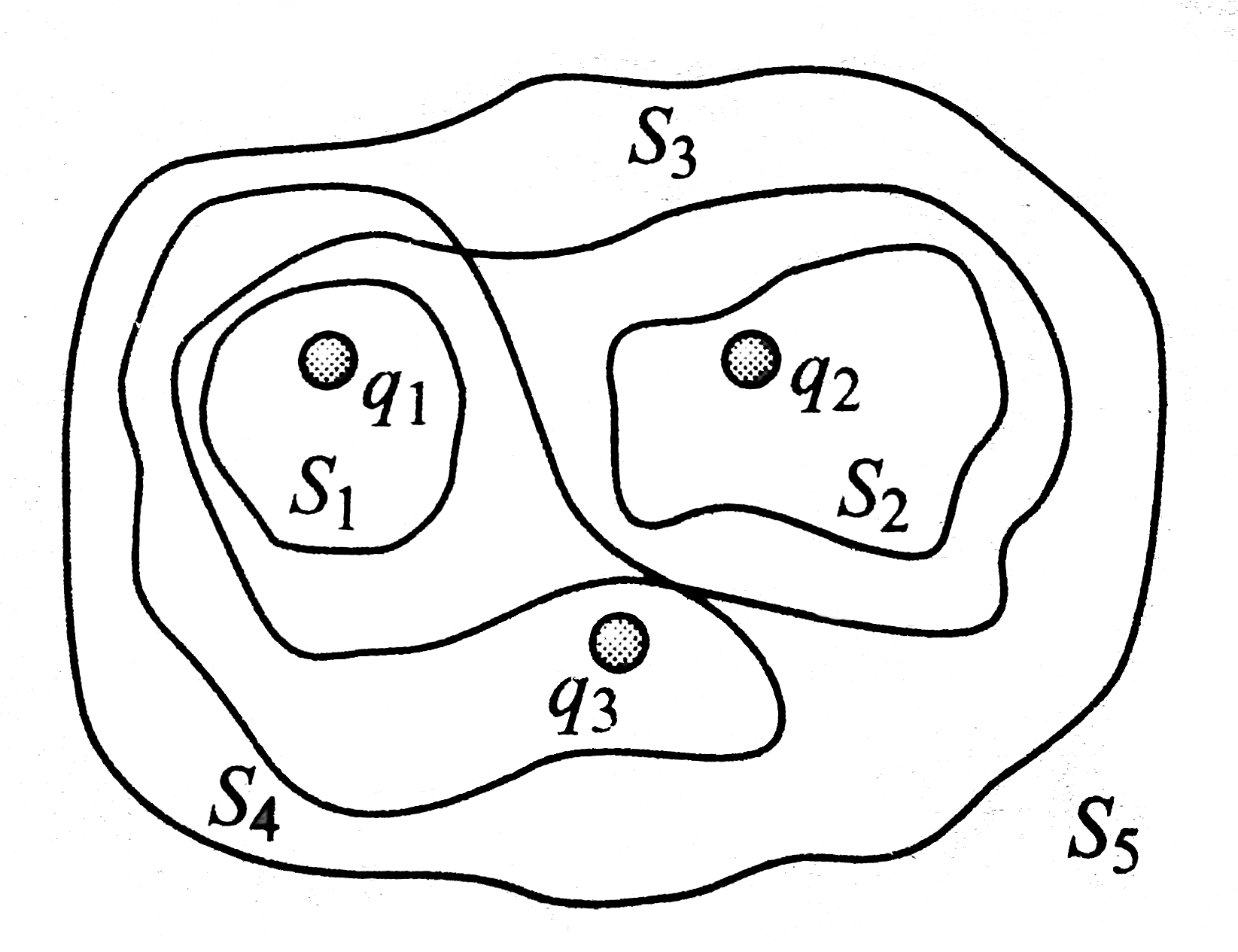
View SolutionThe three small spheres as shown in figure carry charges `q_1 = 4nC, q_2 = -7.8nC and q_3 = 2.4nC`. Find the net electic flux through each of the following closed surfaces shown in cross section in the figure.
a. `S_1` b.`S_2`
c. `S_3` d. `S_4`
e. `S_5`.
Do your answers to parts from (a) to (e) depend on how the charge is distributed over each small sphere? Why or why not?
 .
.
View SolutionA conducting sphere carrying charge Q is surrounded by a spherical conducting shell.
a. What is the net charge on the inner surface of the shell?
b. Another charge q is placed outside the shell. Now, what is the net charge on the inner surface of the shell?
c. If q is moved to a position between the shell and the sphere, what is the net charge on the inner surface of the shell?
d. Are your answer valid if the sphere and shell are not concentric?
View SolutionA solid insulating sphere of radius a carries a net positive charge `3Q`, uniformly distributed throughout its volume. Concentric with this sphere is a conducting spherical shell with inner radius b and outer radius c and having a net charge -Q, as shown in figure

a. Consider a spherical Gaussian surface of radius rgtc, the net charge enclosed by this surface is .............
b. The direction of the electric field rgtc is .............
c. The electric field at rgtc is ............... .
d. The electric field in the region with radius r, which cgtrgtb, is ...............
e. Consider a spherical Gaussian surface of radius r, where `cgtrgtb`, the net charge enclosed by this surface is ................ .
f. Consider a spherical Gaussian surface of radius r, where`bgtrgta`, the net charge enclosed by this surface is ................. .
g. The electric field in the region `bgtrgta` is ................ .
h. Consider a spherical Gaussian surface of radius `rlta`. Find an expression for the net charge Q(r) enclosed by this surface as a function of r. Note that the charge inside the surface is less than 3Q.
i. The electric field in the region `rlta` is ................. .
j. The charge on the inner surface of the conducting shell is .......... .
k. The charge on the outer surface of the conducting shell is .............. .
l. Make a plot of the magnitude of the electric field versus r.
View SolutionA small conducting spherical shell with inner radius a and outer radius b is concentric with a larger conducting sphereical shell with inner radius c and outer radius d. The inner shell has total charge `+2q` and the outer shell has charge `+4q`.
a. Make a plot of the magnitude of the electric field versus r.
b. Calculate the electric field `("magnitude and direction in terms of q")` and the distance r from the common centre of the two shell for `(i) rlta, (ii)altrltb, (iii) `bltrltc,` (iv) `cltrltd`, (v) `rgtd`. Show uour result in a graph with radial component of `vecE` as function of `r`.
c. What is the total charge on the
i. inner surface of the small shell,
ii. outer surface of the small shell,
iii. inner surface of the large shell,
iv. outer surface of the large shell?
View SolutionWhich of the following statements `is//are` correct?
a. Electric field calculated by Gauss law is the field due to only those charges which are enclosed inside the Gaussian surface.
b. Gauss law is applicable only when there is a symmetrical distribution of charge.
c. Electric flux through a closed surface is equal to total flux due to all the charges enclosed within that surface only.
View SolutionWhich of the following statement is correct ? If E = 0 , at all points of a closed surface.
a. the electirc flux through the surface is zero.
b. the total charge enclosed by the surface is zero.
View SolutionA hollow dielectric sphere, as shown in figure, has inner and outer radii of `R_1 and R_2`, respectively. The total charge carried by the sphere is +Q, this charge is uniformly distributed `R_1 and R_2`.
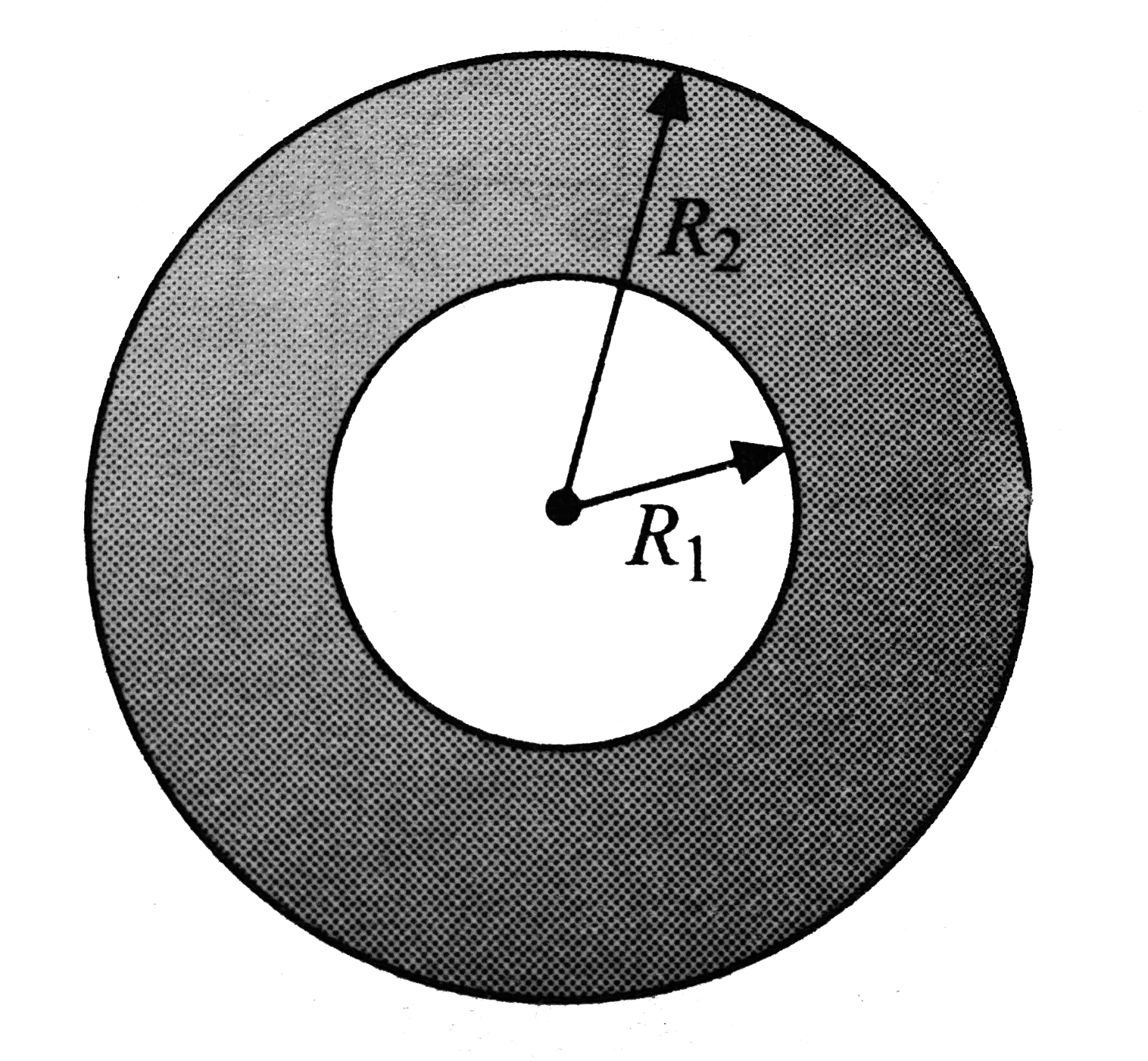
a. the electric field for `rltR_1` is zero. `(Yes//No)`
b. the electric field for `R_1ltrltR_2` is given by ........... .
c. the electric field for `rgtR_2` is given by .......... .
View SolutionA ring of diameter d is rotated in a uniform electric field until the position of maximum electric flux is found. The flux is found to be `phi`. What is the electric field strength?
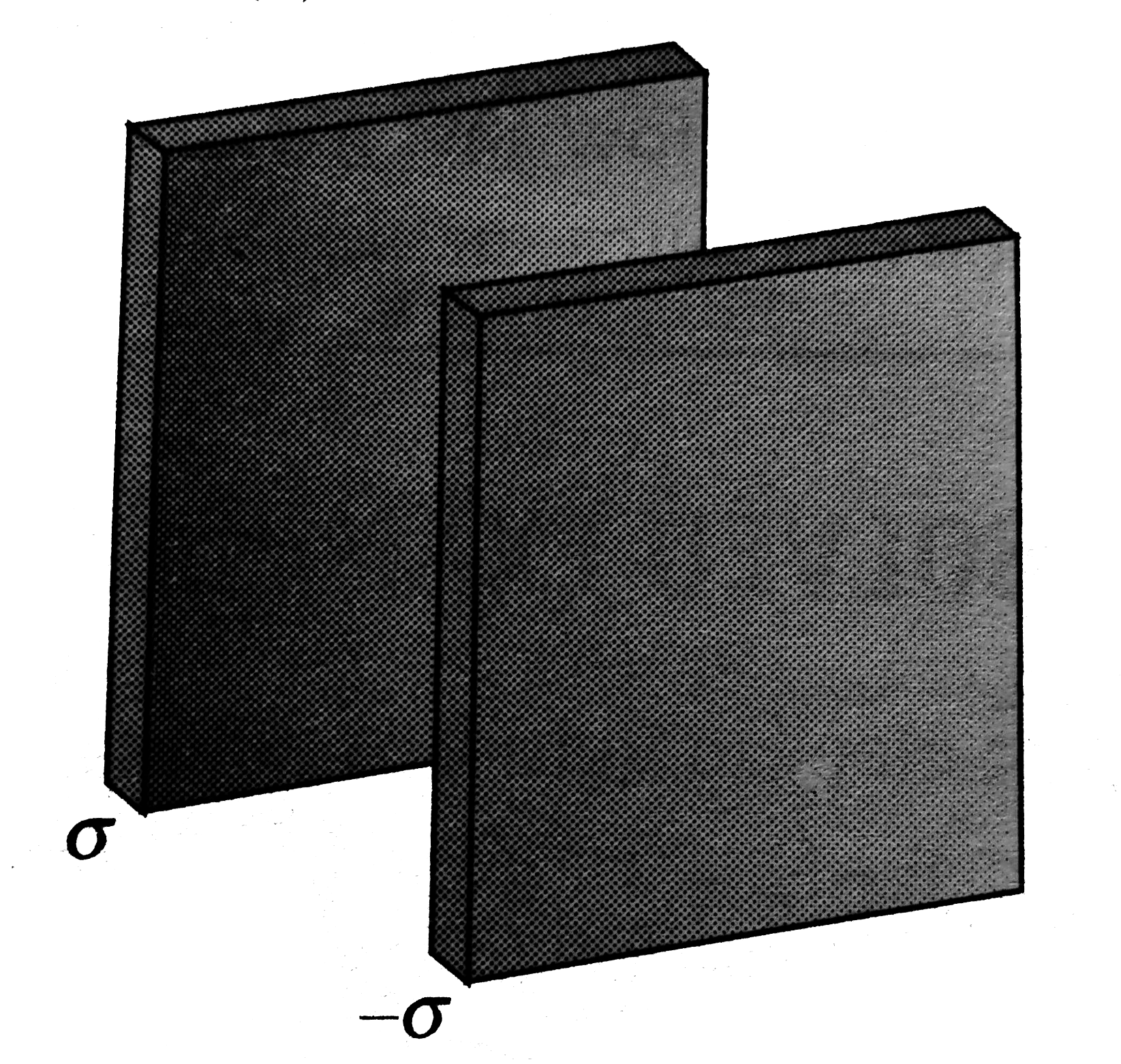
View SolutionTwo infinite, non-conducting sheets of charge are parallel to each other, as shown in figure. The sheet on the left has a uniform surface charge density `sigma`, and the one on the right has a uniform charge density `-sigma`. Calculate the electric field at points (a) to the left of, (b) in between, and (c) to the right of the two sheets.
View Solution`S_1 and S_2` are two hollow concentric spheres enclosing charge Q and 2Q, respectively, as shown in figure. What is the ratio of the electric flux leaving through the surface of `S_1 and S_2`?

View SolutionA hollow half cylinder surface of radius R and length l is placed in a uniform electric field `vecE`. Electric field is acting perpendicular on the plane ABCD. Find the flux through the curved surface of the hollow cylindrical surface

View SolutionConsider two concentric conducting spheres. The outer sphere is hollow and initially has a charge `-7Q` on it. The inner sphere is solid and has a charge `+2Q` on it.
a. How much charge is on the outer surface and inner surface of the outer sphere.
b. If a wire is connected between the inner and outer sphere, after electrostatic equilibrium is established how much total charge is on the outer sphere? How much charge is on the outer surface and inner surface of the outer sphere? Does the electric field at the surface of the inside sphere change when the wire is connected?
c. We return to original condition in (a). We now connect the outer sphere to ground with a wire and then disconnect it. How much total charge will be on the outer sphere? How much charge will be on the inner surface and outer surface of the outer sphere?
View Solution
Exercise 2.2 questions and complete solutions for chapter ELECTRIC FLUX AND GAUSS LAW of CENGAGE PHYSICS (HINGLISH) of Class 12