Complete Solutions to Subjective of chapter ELECTRIC FLUX AND GAUSS LAW of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | ELECTRIC FLUX AND GAUSS LAW |
| CENGAGE PHYSICS | Subjective |
| Number Of Questions | 12 |
| Academic Year | 2023-24 |
A solid spherical region, having a spherical cavity whose diameter R is equal to the radius of the spherical region, has a total charge Q. Find the electric field at a point P as shown in figure
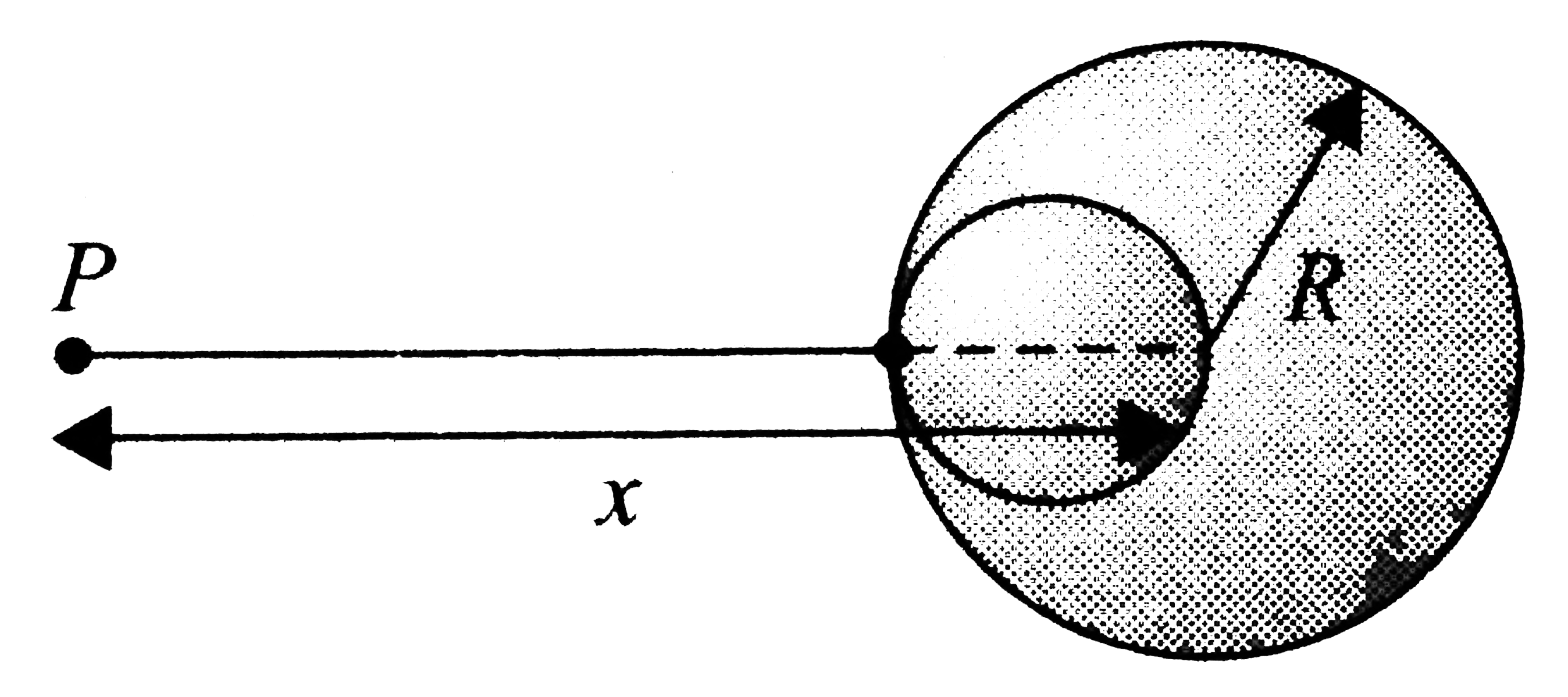 .
.
View SolutionA very long, solid insulating cylinder with radius R has a cylindrical hole with radius a bored along its entire length. The axis of the hole is at a distance b from the axis of the cylinder, where `altbltR` (as shown in figure). The solid material of the cylinder has a uniform volume charge density `rho`. Find the magnitude and direction of the electric field inside the hole, and show that this is uniform over the entire hole.
 .
.
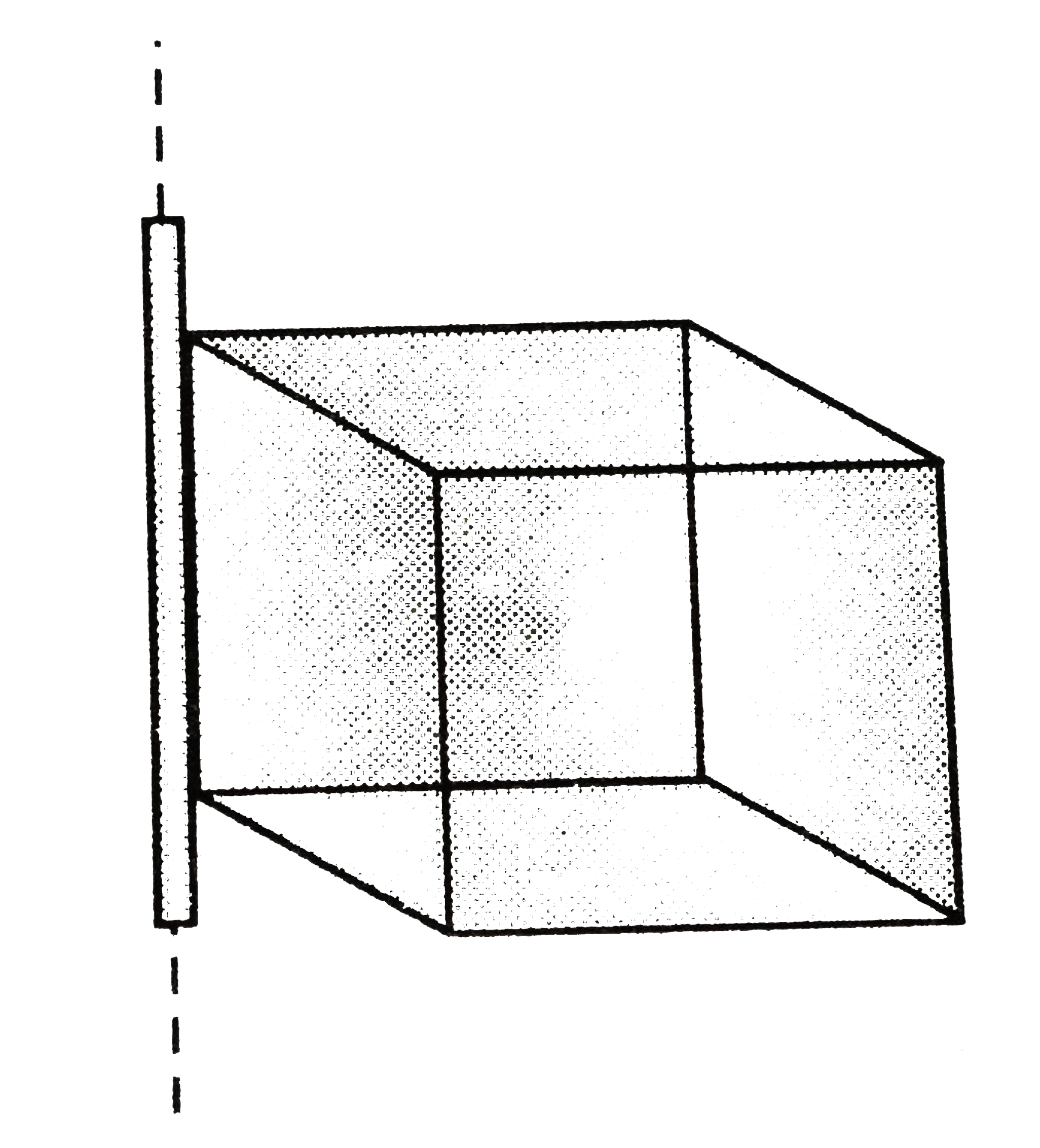
View SolutionAn infinite wire having charge density `lambda` passes through one of the edges of a cube having edge length l.
a. Find the total flux passing through the cube.
b. Find the flux passing through the surfaces in contact with the wire.
c. Find the flux passing through the surfaces not in contact with the wire.
View SolutionIt has been experimentally observed that the electric field in a large region of earth's atmosphere is directed vertically down. At an altitude of 300 m, the electric field `60 Vm^(-1)`. At an altitude of 200 m, the field is 100 V m^(-1), the field is `100 Vm^(-1)`. Calculate the net amount of charge contained in the cube of 100 m edge, located between 200 and 300 m altitude.

View SolutionA point charge Q is located on the axis of a disk of radius R at a distance b from the plane of the disk. Show that if one-fourth of the electric flux from the charge passes through the disk, then `R = sqrt(3b)`.

View SolutionA very long uniformly charged wire oriented along the axis of a circular ring of radius R rests on its centre with one of the ends (as shown in figure). The linear charge density on the wire is `lambda`. Evalute the flux of the vector `vecE` across the circle area.
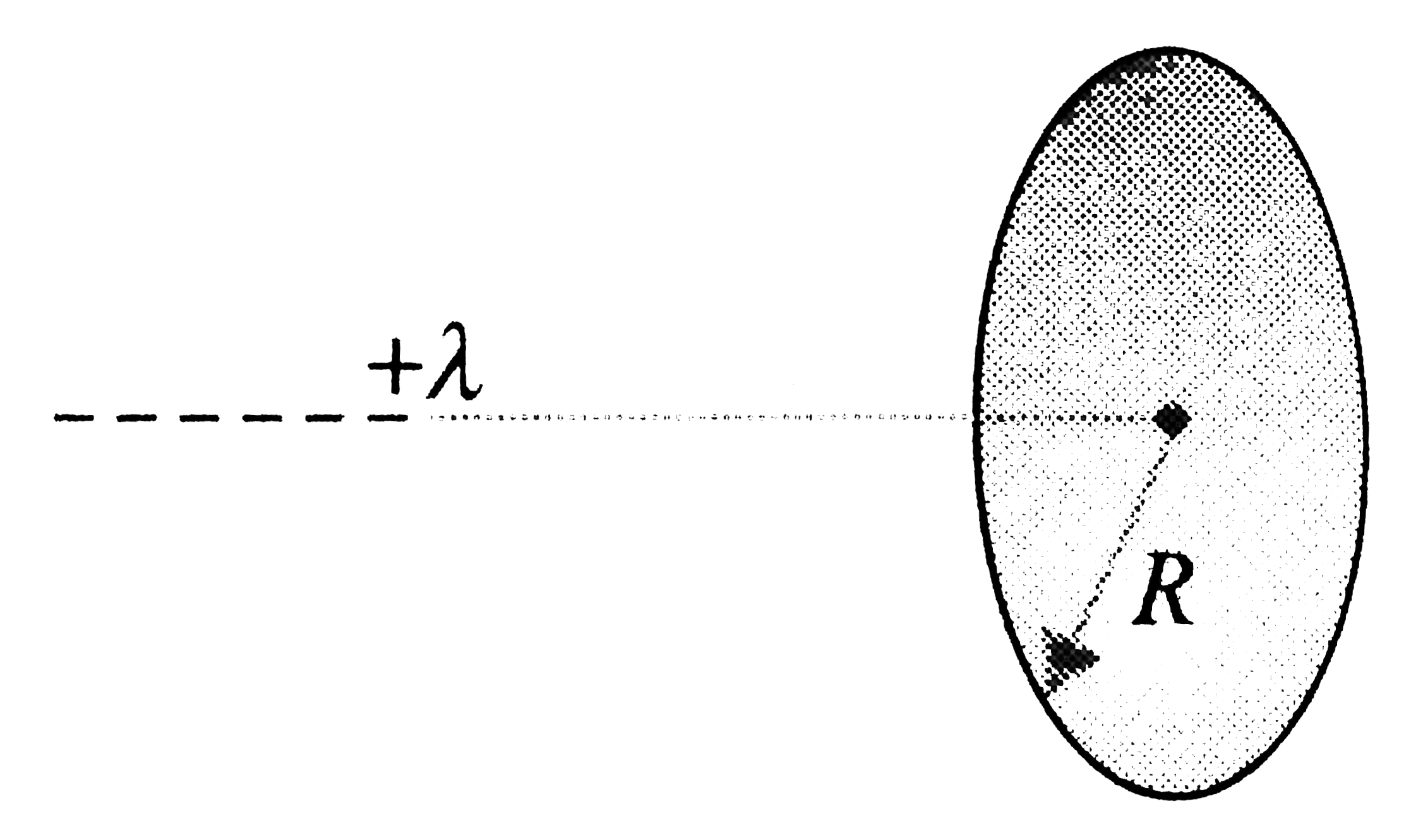
View SolutionTwo point charges q and -q are separated by a distance 2a. Evaluate the flux of the electric field strength vector across a circle of radius R.

View SolutionAn infinitely long line charge having a uniform charge per unit length `lambda` lies at a distance d from a point O as shown in figure. Determine the total electric flux through through the surface of a sphere of radius R centered at O resulting from this line charge. Consider both cases where Rltd and Rgtd`.
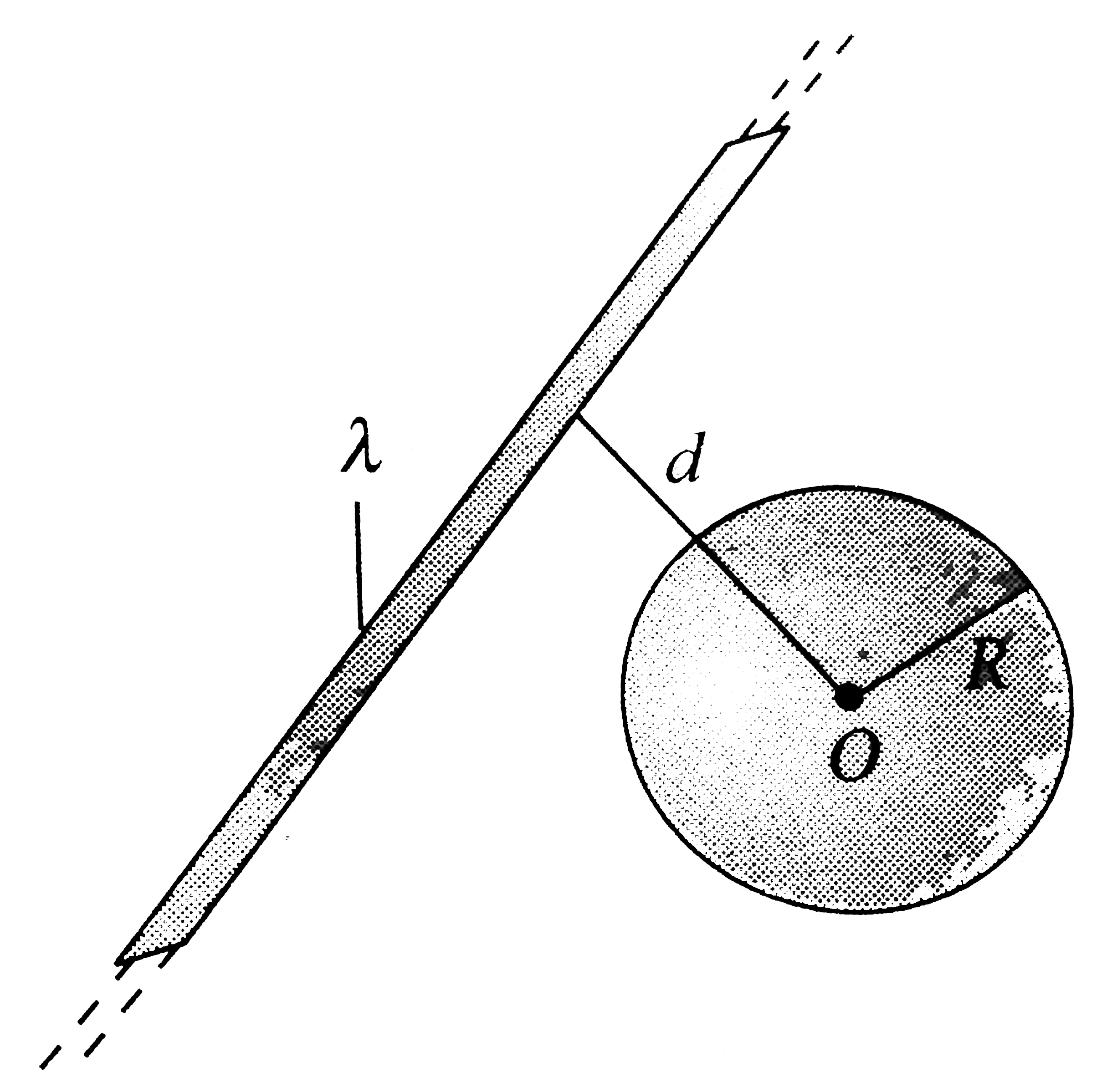
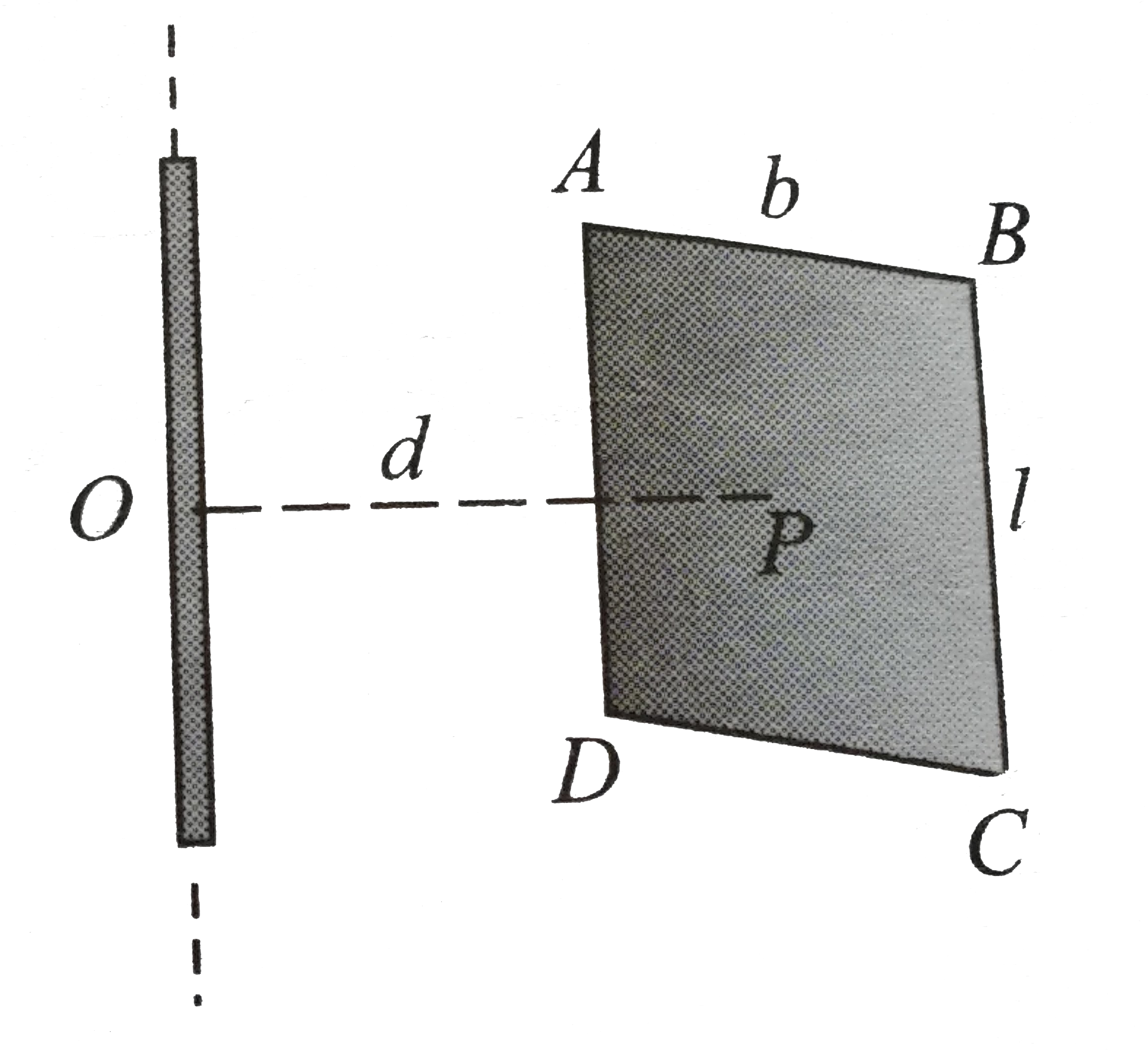
View SolutionFind the electric flux crossing the wire frame ABCD of length l, width b, and center at a distance OP = d from an infinite line of charge with linear charge density `lambda`. Consider that the plane of the frame is perpendicular to the line OP
View SolutionA solid insulating sphere of radius R has a nonuniform charge density that varies with r according to the expression `rho = Ar^2`, where A is a constant and rltR is measured from the centre of the sphere. Show that (a) the magnitude of the electric field outside (rgtR) the sphere is `E = AR^5//5epsilon_0r^2` and (b) the magnitude of the electric field inside (rltR) the sphere is `E = AR^3//5epsilon_0`.
View SolutionThe electric field in a region is radially outward with magnitude `E=Ar`. Find the charge contained in a sphere of radius a centred at the origin. Take `A=100 V m^(-2)` and `a=20.0 cm`.
View SolutionA system consists of a ball of radius R carrying a uniformly distributed charge q and the surrounding space filled with a charge of volume density `rho = alpha//r`, where `alpha` is a constant and r is the distance from the centre of the ball. Find the charge on the ball for which the magnitude of electric field strength outside the ball is constant? The dielectric constant of the ball and the surrounding may be taken equal to unity.
View Solution
Subjective questions and complete solutions for chapter ELECTRIC FLUX AND GAUSS LAW of CENGAGE PHYSICS (HINGLISH) of Class 12