Complete Solutions to EXERCISE 1 of chapter CURRENT ELECTRICITY of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | CURRENT ELECTRICITY |
| MHTCET PREP GUIDE | EXERCISE 1 |
| Number Of Questions | 75 |
| Academic Year | 2023-24 |
A wire of resistance `4 Omega` is stretched to twice its original length. The resistance of stretched wire would be
View SolutionA current 4.0 A exist in a wire of cross-sectional area `2.0 mm^(2)`. If each cubic metre of the wire contains `12.0xx10^(28)` free electrons, then the drift spped is
View SolutionWith the rise of temperature the resistivity of a semiconductor
View SolutionA carbon film resistor has colour green, black, violet and gold . The value of the resistor is
View SolutionTwo wires of the same material but of different diameters carry the same current `i`. If the ratio of their diameters is `2:1` , then the corresponding ratio of their mean drift velocities will be
View SolutionFor a metallic wire, the ratio `V/i` ( `V=` applied potential difference and `i=` current flowing ) is
View SolutionTwo conductors are made of the same material and have the same length. Conductor `A` is a solid wire of diameter `1 mm`. Conductor `B` is a hollow tube of outer diameter `2 mm` and inner diameter `1mm`. Find the ratio of resistance `R_(A)` to `R_(B)`.
View SolutionTwo wires of the same dimensions but resistivities `rho_(1)` and `rho_(2)` are connected in series. The equivalent resistivity of the combination is
View SolutionA material 'B' has twice the specific resistance of 'A'. A circular wire made of 'B' has twice the diameter of a wire made of 'A'. Then for the two wires to have the same resistance, the ratio `l_(B)//l_(A)` of their respective lengths must be
View SolutionThe resistance of a 10 m long wire is 10 `Omega`. Its length is increased by 25% by stretching the wire uniformly . The resistance of wire will change to
View SolutionCalculate the average drift speed of conduction electrons in a copper wire of cross-sectional area `1.0xx10^(-7)m^(2)`, carrying a current of 1.5 A. Assume that each copper atom contributes roughly one conduction electron. The density of copper is `9.0xx10^(3) kg m^(-3)` and its atomic mass is 63.5. Take Avogadro's number `=6.0xx10^(23)`.
View SolutionThe resistance of a bulb filmanet is `100Omega` at a temperature of `100^@C`. If its temperature coefficient of resistance be 0.005 per `""^@C`, its resistance will become `200 Omega` at a temperature of
View SolutionAll the edges of a block with parallel faces are unequal. Its longest edge is twice its shortest edge. The ratio of the maximum to minimum resistance between parallel faces is.
View SolutionTwo copper wire of length l and 2l have radii, r and 2r respectively. What si the ratio of their specific resistance.?
View SolutionWhich of the following materials is the best conductor of electricity ?
View SolutionElectric field (E) and current density `(J)` have relation
View SolutionThe temperature (T) dependence of resistivity (rho) of a semiconductor is represented by :
View SolutionA current 4.0 A exist in a wire of cross-sectional area `2.0 mm^(2)`. If each cubic metre of the wire contains `12.0xx10^(28)` free electrons, then the drift spped is
View SolutionA wire of resistance `R` is elongated `n-fold` to make a new uniform wire. The resistance of new wire.
View SolutionThe four colours on a resistor are: brown, yellow, green and gold as read from left to right. What is resistance corresponding to these colours.
View SolutionThe resistance will be least in a wire with length, cross-section area respectively,
View SolutionA wire has resistance of `10Omega`. If it is stretched by 1/10th of its length, then its resistance is nearly
View SolutionIf resistivity of copper conductor is `1.7xx10^(-8) Omega -m` and electric field is `100Vm^(-1)`, then current density will be
View SolutionA wire is stretched so as to change its diameter by `0.25%` . The percentage change in resistance is
View SolutionA potential difference of 100 V is applied to the ends of a copper wire one metre long . What is the average drift velocity of electrons ?
(Given, `sigma=5.81xx10^(7)Omega` or `n_(Cu)=8.5xx10^(28)m^(-3)`)
View SolutionIf power dissipated in the `9 Omega` resistor in the resistor shown is `36 W`, the potential difference across the `2 Omega` resistor is
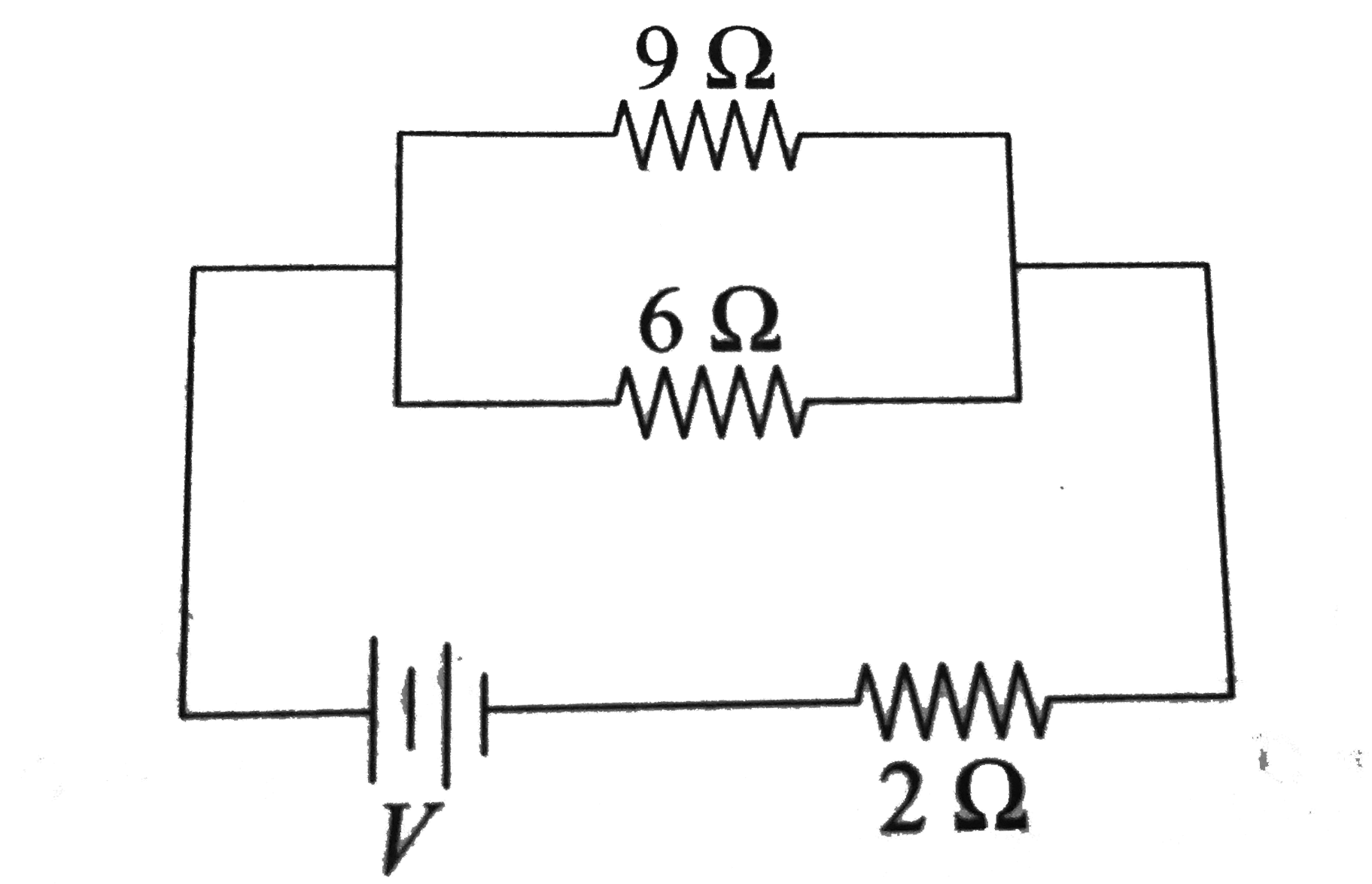
View SolutionThe effective resistance between points A and C for the network shown figure is
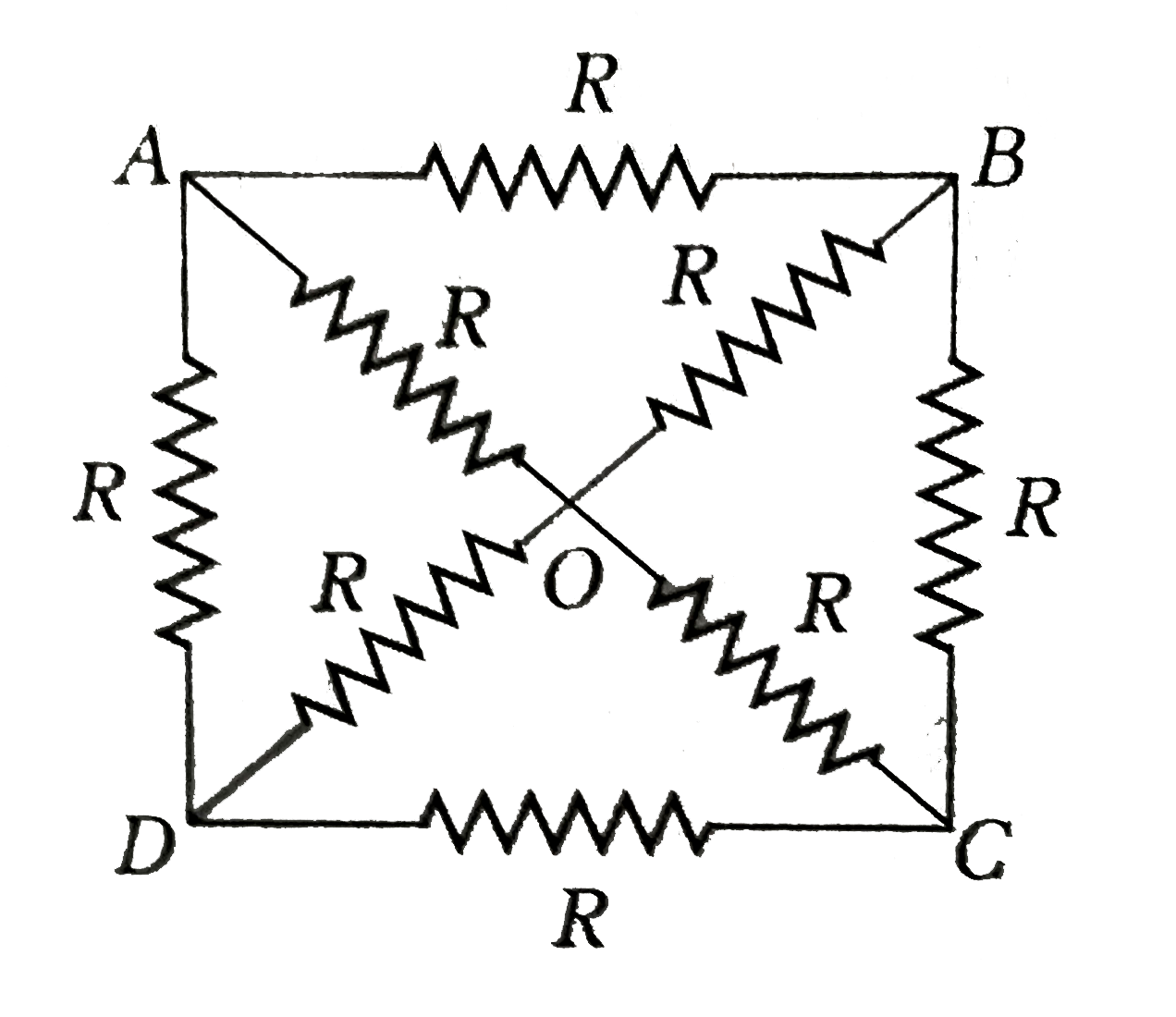
View SolutionA resistor of 6k `Omega` with tolerance 10% and another resistance of `4kOmega` with tolerance `10%` are connected in series. The tolerance of the combination is about
View SolutionIn the circuit given E = 6.0 volt, `R_(1) = 100Omega, R_(2) = R_(3)=50Omega` and `R_(4)=75Omega`. The equivalent resistance of the circuit, in ohms is
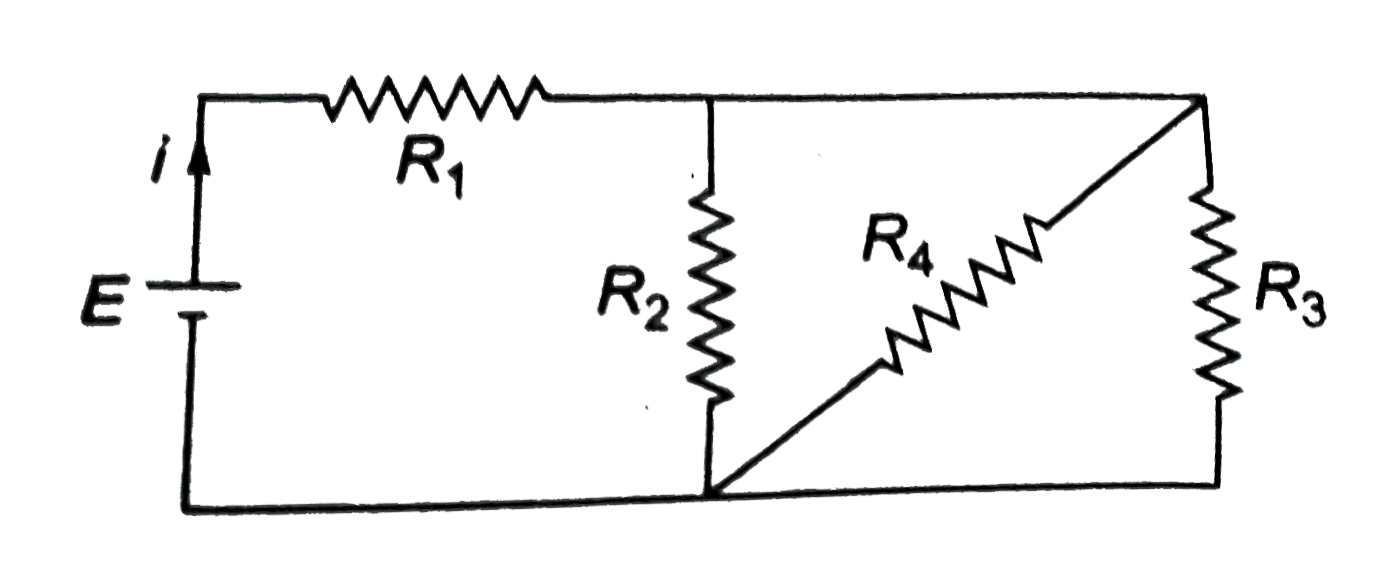
View SolutionThe circuit in figure, where there are three resistors `R_(1),R_(2)` and `R_(3)` . If the voltage between A and C is V, the current / is given by
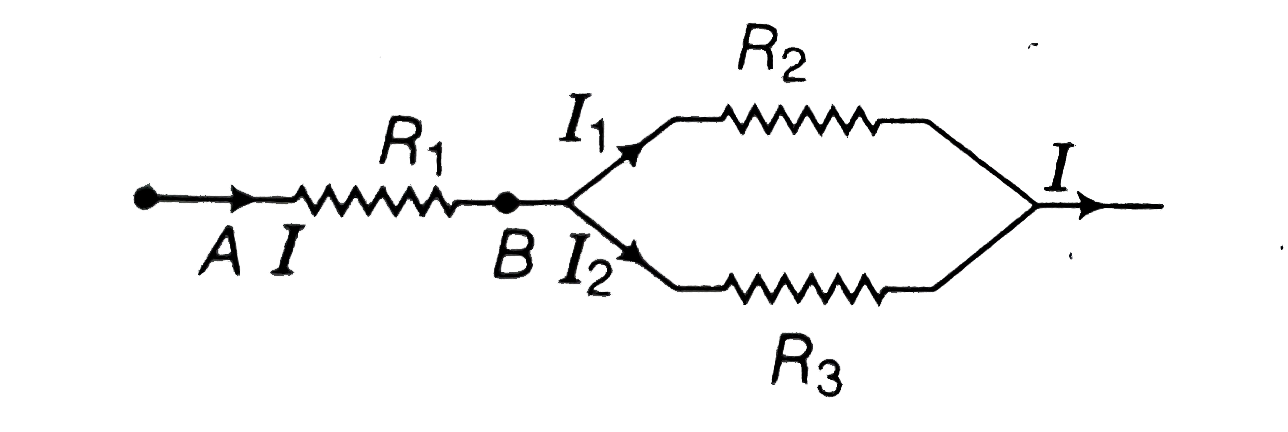
View SolutionThe resistance across R and Q in the figure is
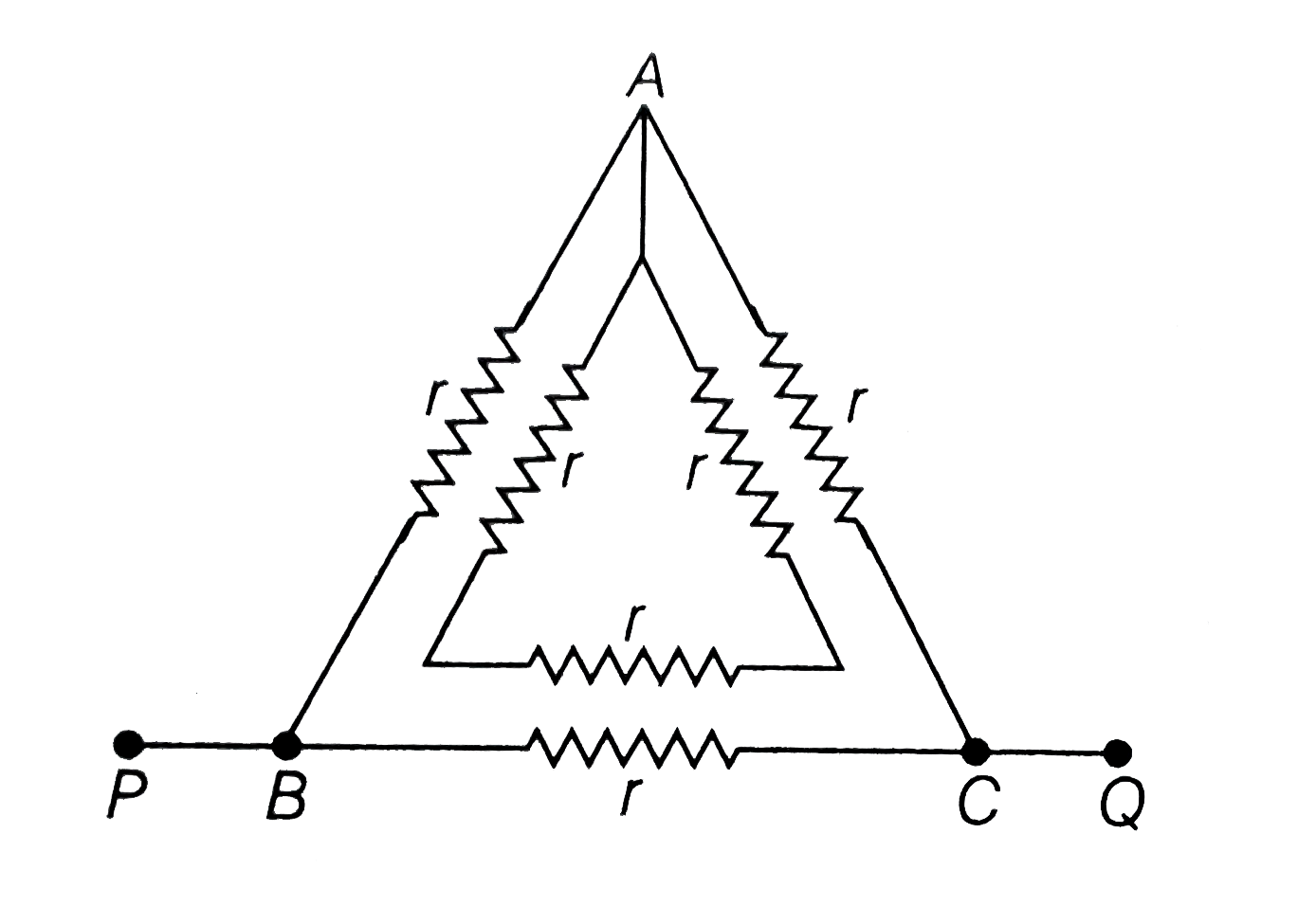
View SolutionTwo resistances are joined in parallel whose equivolent resistance is `3/5Omega`. One of the resistance wire is broken and the effective resistance becomes `3Omega`. The resistance (in ohms) of the wire that got broken was
View SolutionThe equivalent resistance of two resistors connected in series `6Omega` and their parallel equivalent resistance is `4/3Omega`. What are the value of resistance?
View SolutionThree resistances `2 Omega, 3 Omega` and `4 Omega` are connected in parallel. The ratio of currents passing through them when a potential difference is applied across its ends will be
View SolutionFour resistances are connected in a circuit in the given figure. The electric cirrent flowing through `4 ohm` and `6 ohm` resistance is respectively
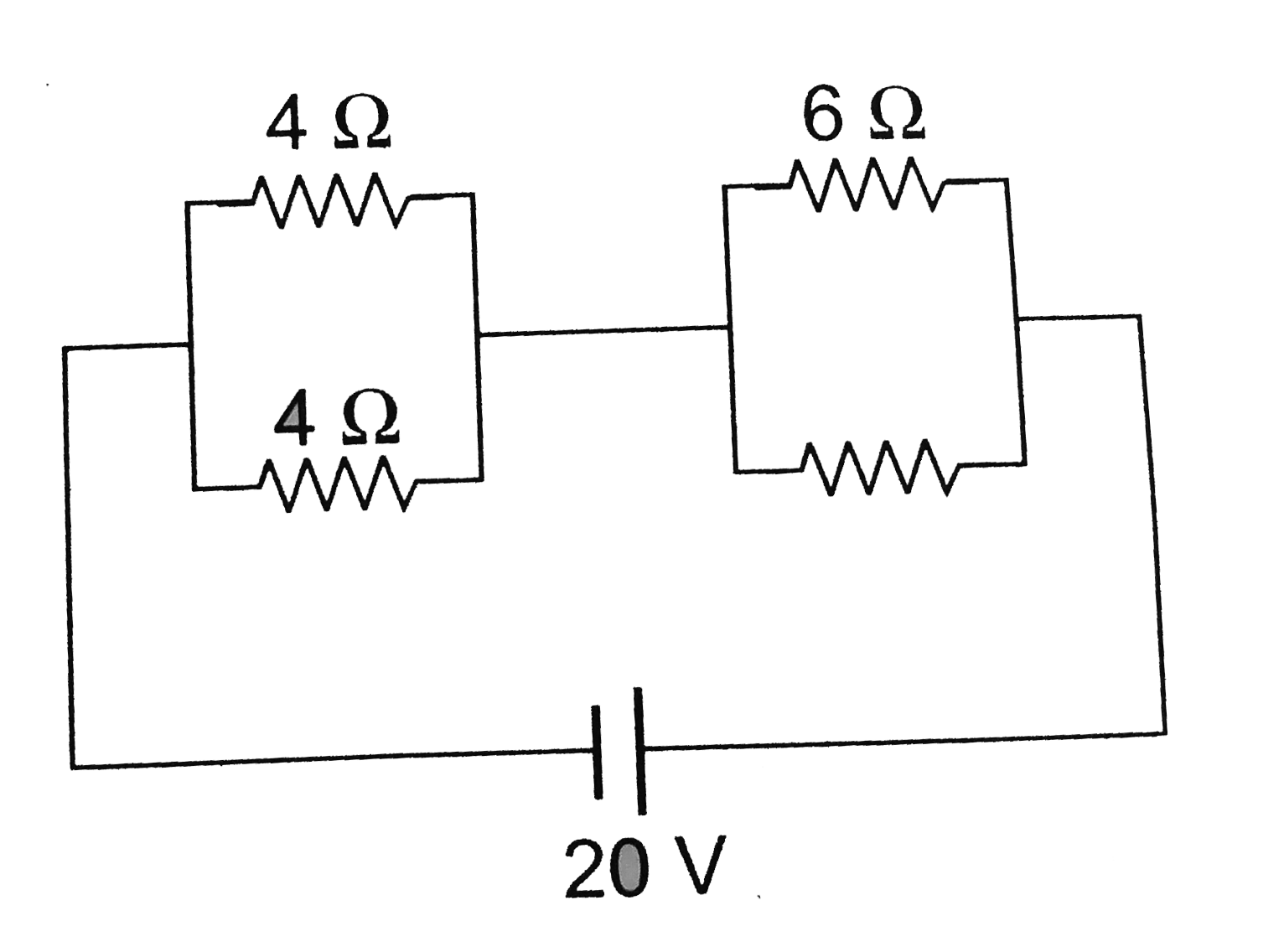
View SolutionFive resistors are connected as shown in figure. Find the equivalent resistance between the points B and C.
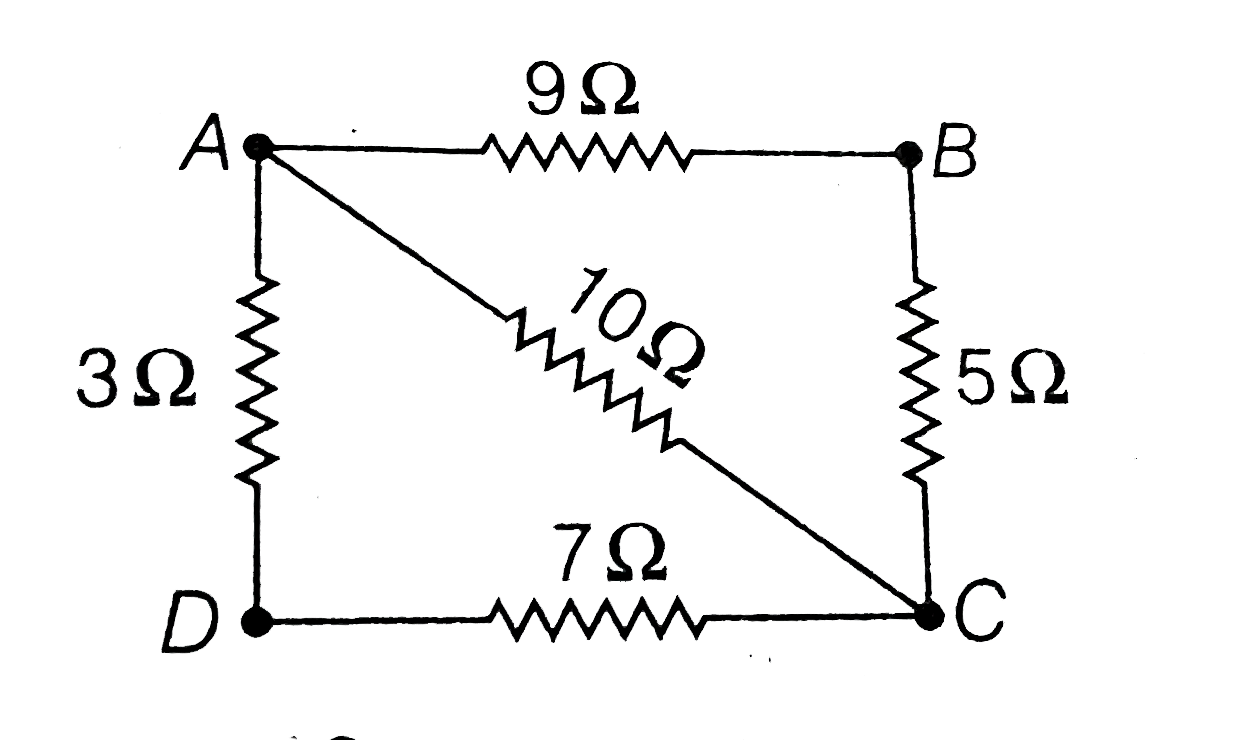
View SolutionIf `400 Omega` of resistance is made by adding four `100 Omega` resistance of tolerance `5%`, then the tolerance of the combinations
View SolutionIn the circuit shown below, the ammeter and the voltmeter readings are 3 A and 6 V respectively . Then, the value of the resistance R is
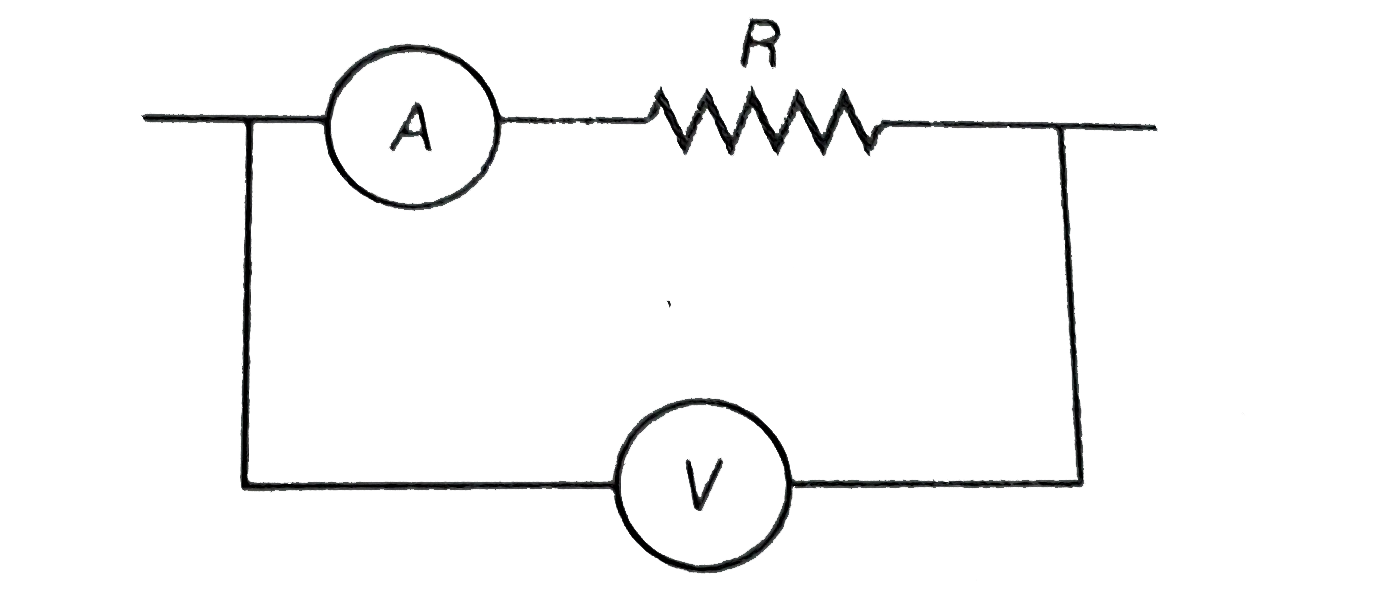
View SolutionIn the circuit show below total resistance between A and B is

View SolutionHow many minimum number of `2Omega` resistance can be connected to have an effective resistance of `1.5Omega`
View SolutionTwo resistances R and 2R are connected in parallel in an electric circuit. The thermal energy developed in R and 2R are in the ratio
View SolutionA wire has a resistance of `6 Omega`. It is cut into two parts and both half values are connected in parallel. The new resistance is ....
View SolutionFour cells, each of emf E and internal resistance r, are connected in series across an external resistance reverse. Then, the current in the external circuit is
View SolutionTwo resistors of resistances `2Omega` and `6Omega` are connected in parallel. This combination is then connected to a battery of emf 2 V and internal resistance `0.5 Omega`. What is the current flowing through the battery ?
View SolutionTow cells with the same emf E and different internal resistances `r_(1)` and `r_(2)` are connected in series to an external resistances R . The value of R so that the potential difference across the first cell be zero is
View SolutionConsider the following two statement.
`(A) Kirchhoff's junction law follows from the conservation of charge.
(B) Krichhoff's loop law follows from the conservation of energy.
Which of the following is correct ?
View SolutionFor the circuit (figure) the currents is to be measured. The ammeter shown is a galvanometer with a resistance `R_(G)=60.0 0 Omega` converted to an ammeter by a shunt resistance `r_(S)=0.02 Omega`. The value of the current is

View SolutionFor driving a current of 2 A for 6 minutes in a circuit, 1000 J of work is to be done. The e.m.f. of the source in the circuit is
View SolutionA 10 m long wire ofresistance `20 Omega` is connected in series with battery of EMF `3V` and negligible internal resistance and a resistance of `10 Omega`. The potential gradient along the wire is :
View SolutionA battery consists of a variable number `n` of identical cells having internal resistance connected in series. The terminals of the battery are short circuited and the current `I` measured. Which one of the graph below shows the correct relationship between `I` and `n`?
View SolutionWhat will be the value of current I in the circuit shown ?
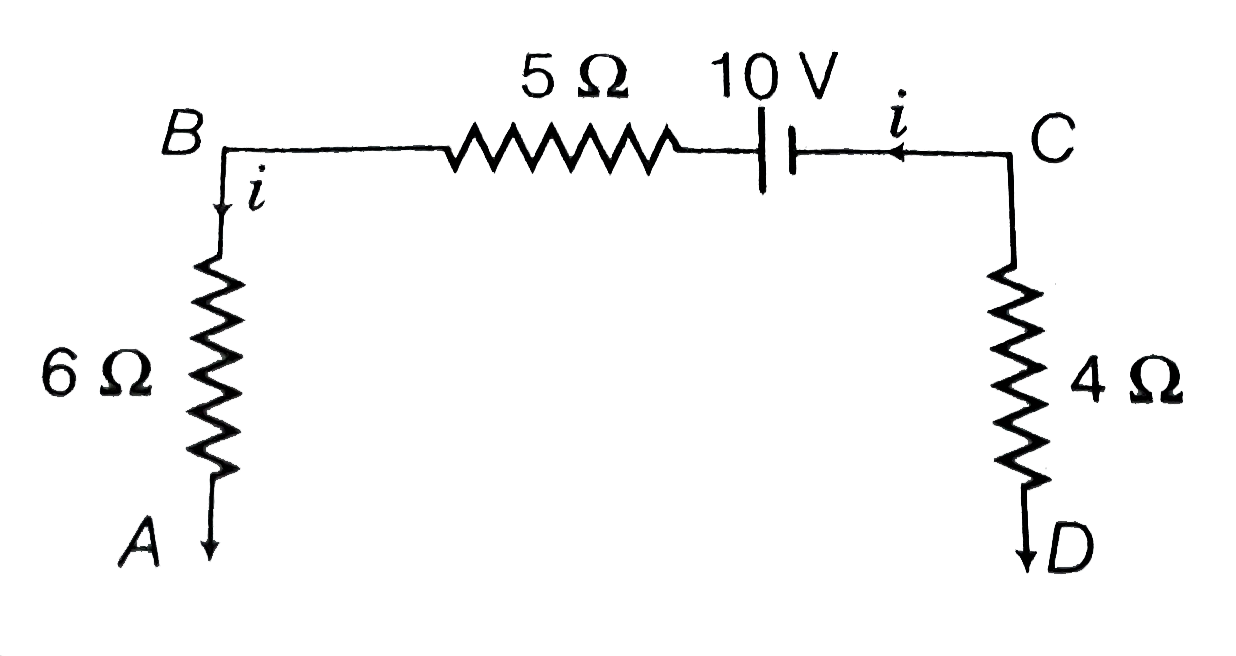
View SolutionIn the circuit given here, the points A,B and C are 70 V, zero, 10 V respectively . Then,

View SolutionIn the following circuit `E_(1)=E_(2)=E_(3) 2V` and `R_(1)=R_(2)=AOmega`. The current passing through battery `E_(2)` between points A and B is
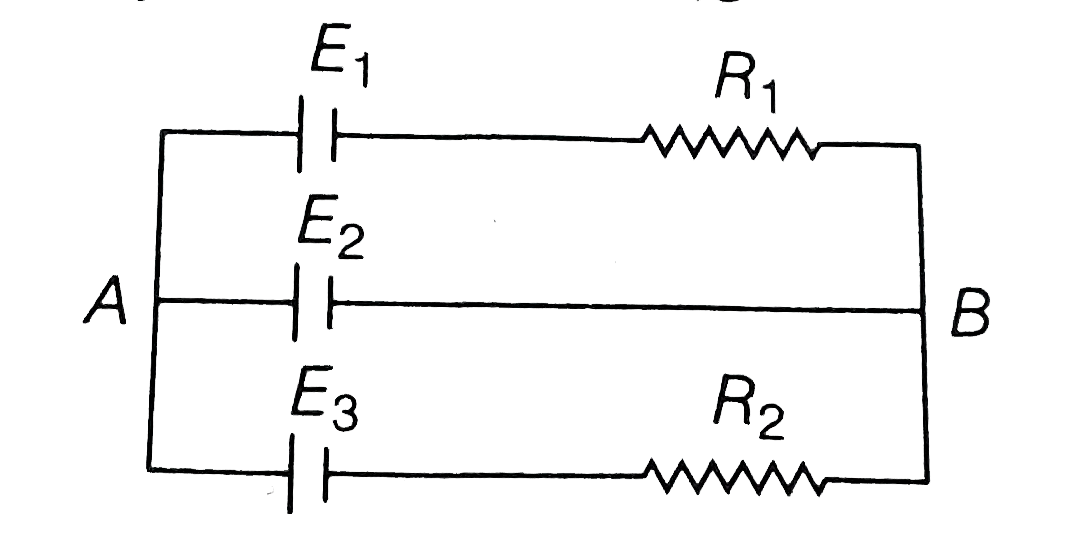
View SolutionFour resistence of `10 Omega, 60Omega,100Omega`and `200Omega`, respectively taken in order are used to form a Wheatstone's bridge . A 15 V battery is connected to the ends of a `200Omega` resistance, the current through it will be
View SolutionTo get a maximum current through a resistance of `2.5Omega` one can use m rows of cells each row having n cells . The internal resistance of each cell is `0.5Omega`. What are the values of m and n, if the total number of cells are 20?
View SolutionFigure shows a circuit with known resistances `R_(1)` and `R_(2)` . Neglect the internal resistance of the sources of current and resistance of the connecting wire . The magnitude of electromotive force E, such that the current through the resistance R is zero will be
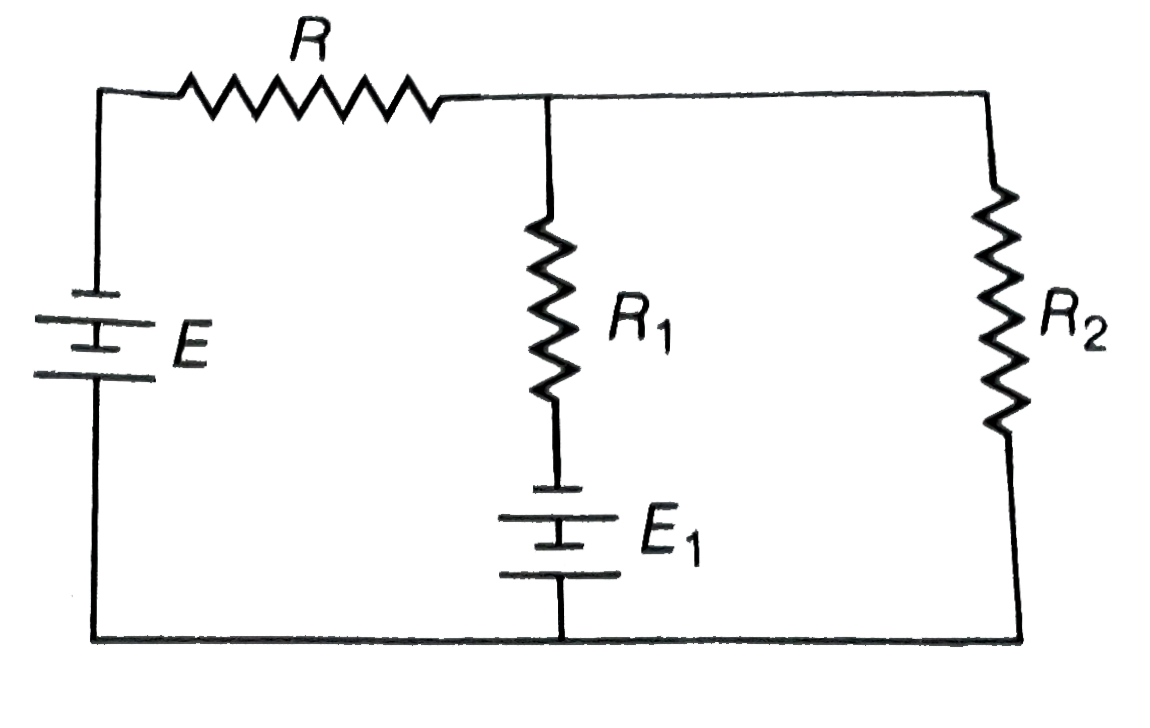
View SolutionThe potential difference across the terminals of a battery is 50 V when 11 A current is drawn and 60 V, when 1A current is drawn . The emf the battery is
View SolutionIn the circuit shown, the currents `i_(1)` and `i_(2)` are
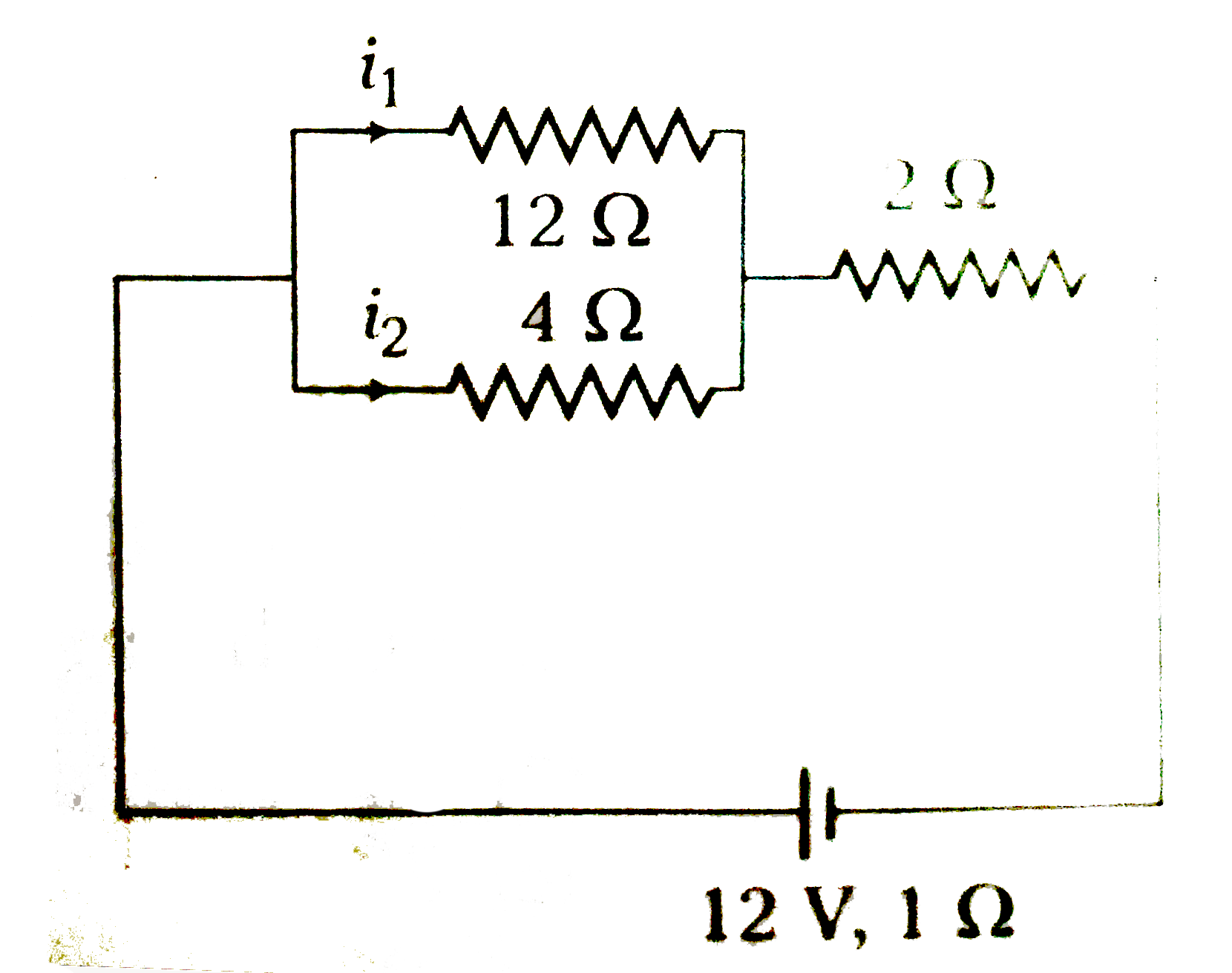
View SolutionA battery of emf 10 V and internal resistance `3Omega`is connected to a resistor. The current in the circuit is 0.5 A . The terminal voltage of the battery when the circuit is closed is
View SolutionIn the following circuit reading of voltmeter V is
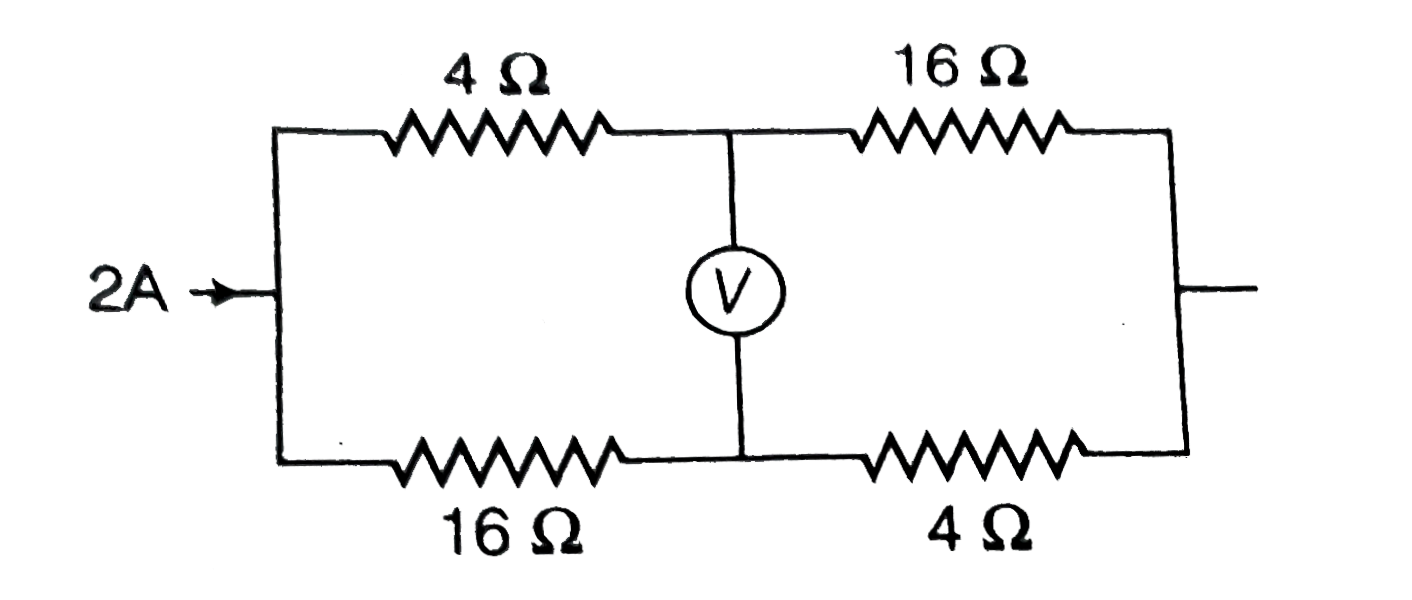
View SolutionIn the electric circuit shown each cell has an emf of 2 V and internal resistance of `1Omega`. The external resistance is `2Omega` . The value of the current is (in A)
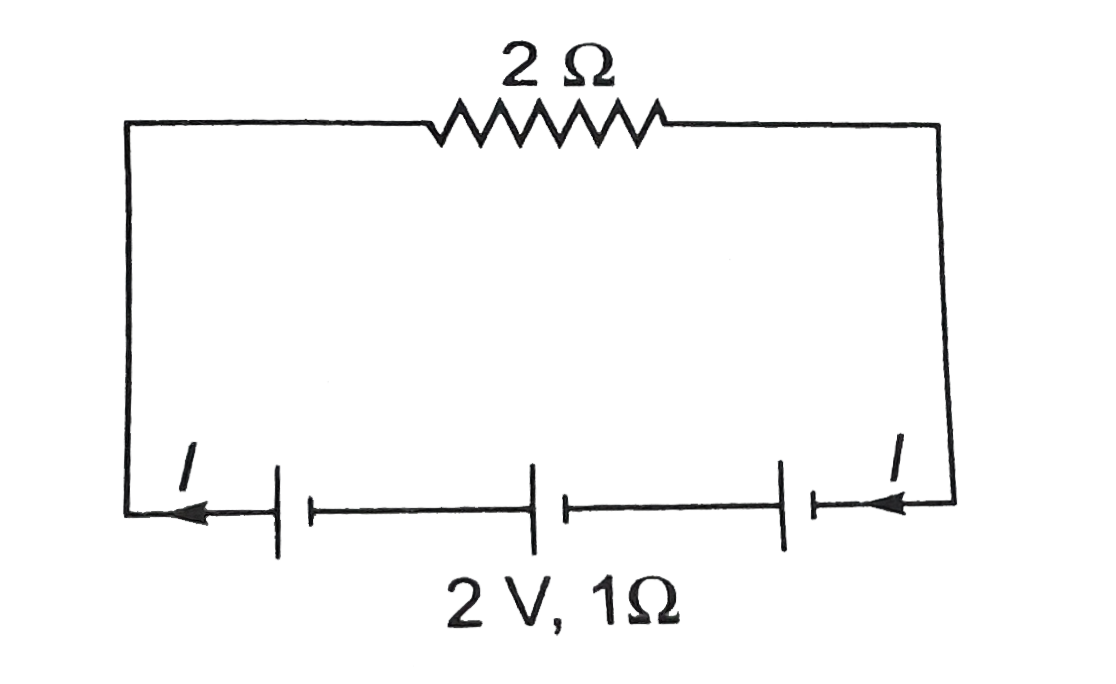
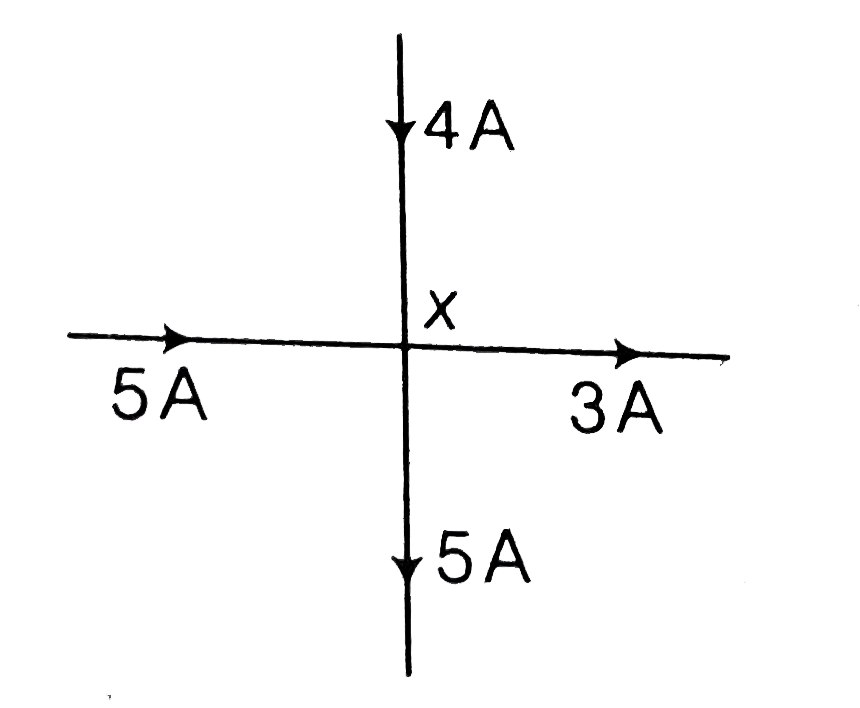
View SolutionFive conductors are meeting a point x as shown in the figures . What is the value of current in fifth conductor ?
View SolutionTwo resistors of resistances `2Omega` and `6Omega` are connected in parallel. This combination is then connected to a battery of emf 2 V and internal resistance `0.5 Omega`. What is the current flowing through the battery ?
View SolutionFour identical cells of emf `epsilon` and internal resistance r are to be connected in series. Suppose, if one of the cell is connected wrongly, then the equivalent emf and effective internal resistance of the combination is
View SolutionTwo cells having an internal resistance of `0.2 Omega` and `0.4 Omega` are connected in parallel, the voltage across the battery is 1.5 V. If the emf of one cell is 1.2 V, then the emf of second cell is
View SolutionTwo cells of emf `E_(1)` and `E_(2)` are joined in opposition (such that `E_(1) gt E_(2)`) . If `r_(1)` be the internal resistances and R be the external resistance, then the terminal potential difference is
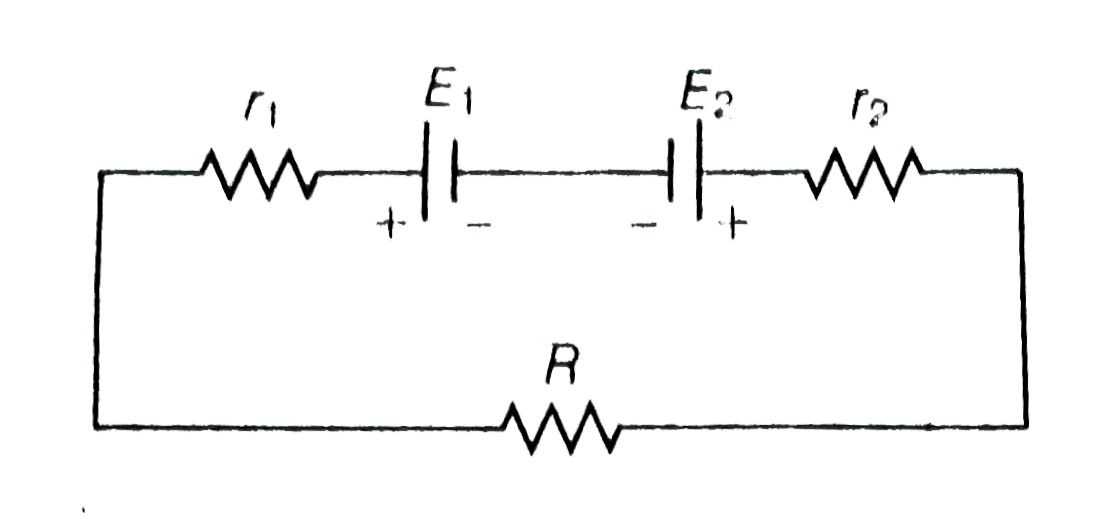
View SolutionA circuit consists of three bateries of emf `E_(1) = 1V, E_(2)=2V` and `E_(3)=3V` and internal resistance `1Omega, 2Omega` and `1Omega` respectively which are connected in parallel as shown in figure. The potential difference between points P and Q is
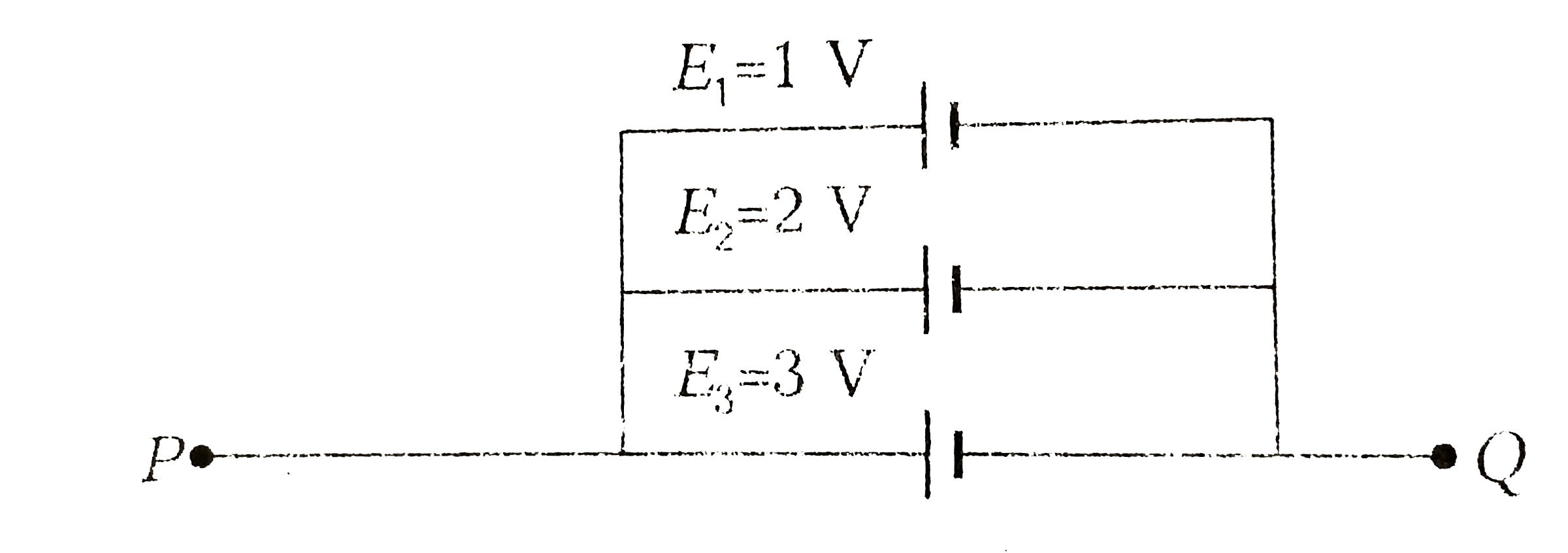
View SolutionTwo identical cells whether connected in parallel or in series gives the same current, when connected to an external resistance `1.5Omega`. Find the value of internal resistance of each cell.
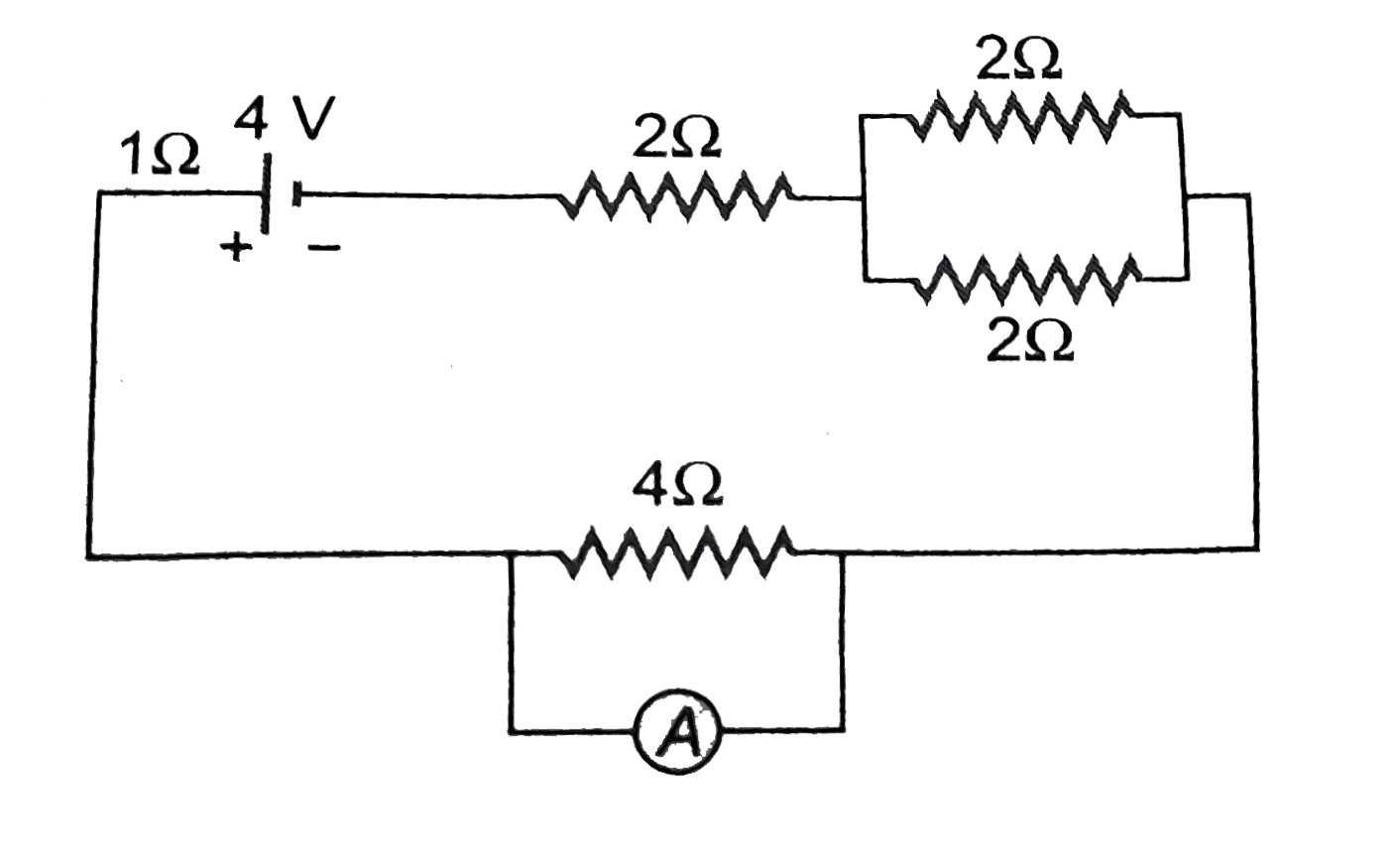
View SolutionThe current pasing through the ideal ammeter in the circuit given below is
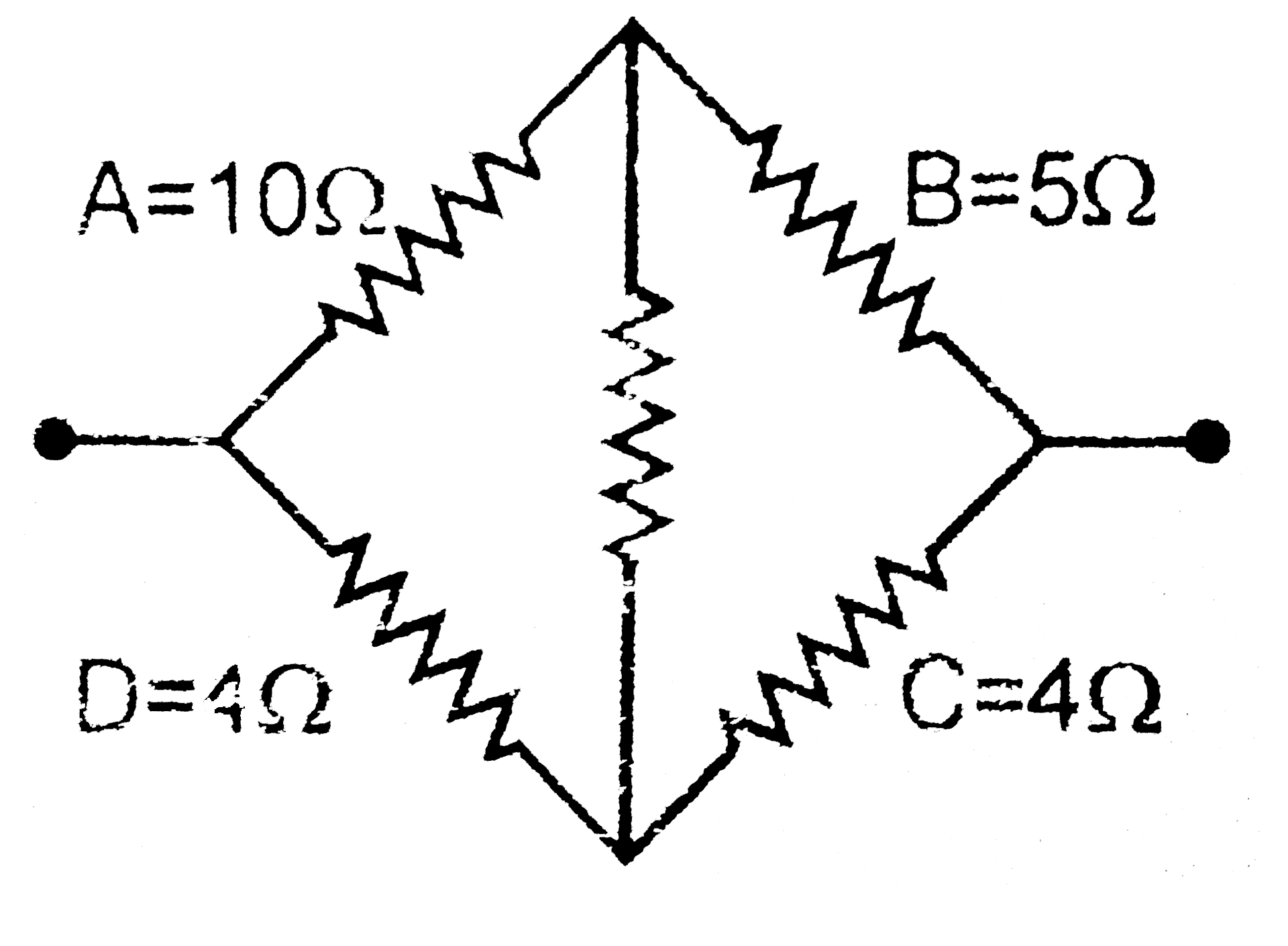
View SolutionIn a typical wheatstone network the resistance in cyclic order are A=`10Omega,B=5O,ega,C=4Omega` and `D=4Omega` for the bridge to be balanced.
View SolutionIn a potentiometer experiment for measuring the emf of cell, the null point is at 480 cm when we have a `400Omega` resistor in series with the cell and galvanometer . If the series resistances is reduced to half, the null point will be at
View SolutionIn a Wheatstone's network, `P=2Omega, Q=2Omega, R=2Omega and S=3Omega`. The resistance with which S is to be shunted in order that the bridge may be balanced is
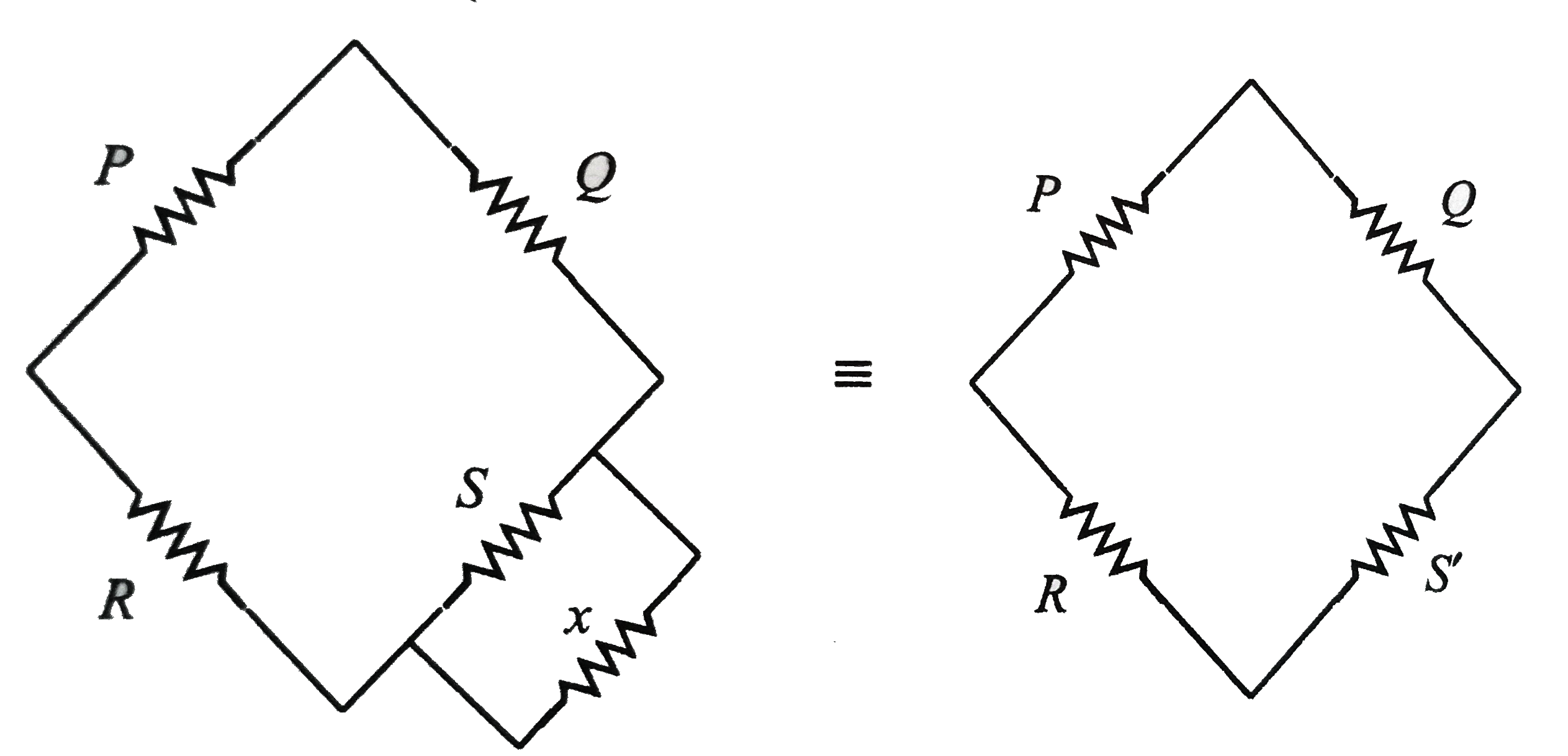
View SolutionIn a potentiometer experiment the balancing with a cell is at length 240 cm. On shunting the cell with a resistance of `2Omega`, the balancing length becomes 120 cm.The internal resistance of the cell is
View SolutionFor measurement of potential difference, potentiometer is perferred in comparison to voltmeter because
View SolutionFive resistance are connected as shown in the figure . The equivalent resistance between P and Q will be
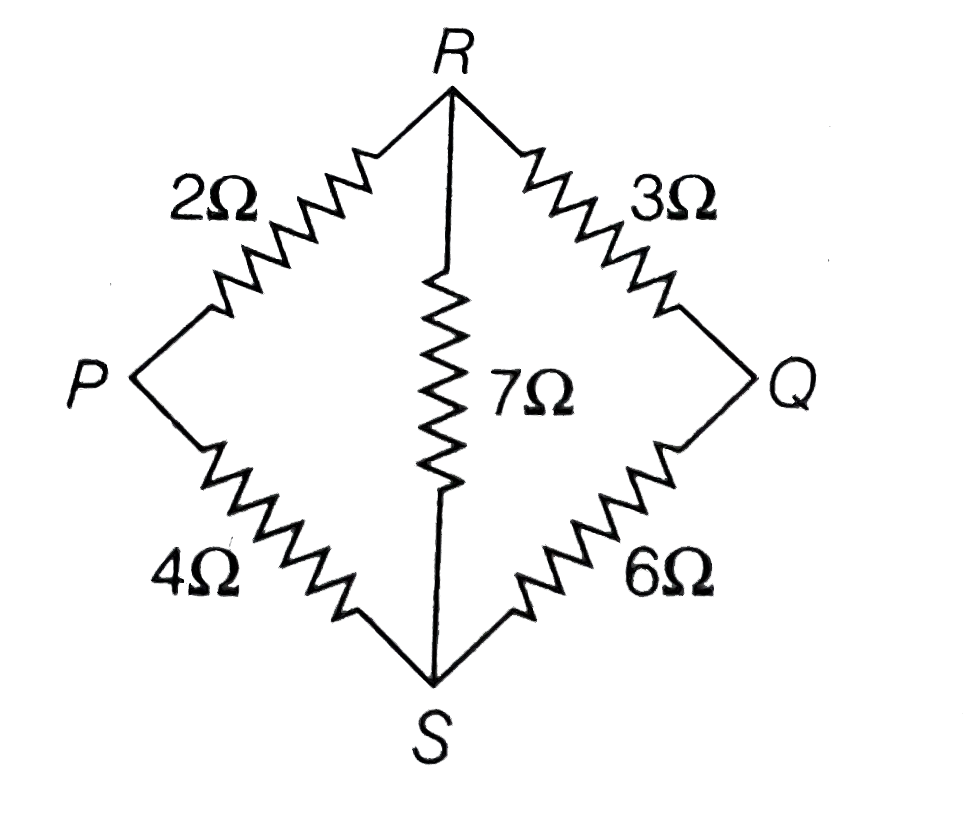
View Solution
EXERCISE 1 questions and complete solutions for chapter CURRENT ELECTRICITY of MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS of Class 12