Complete Solutions to Multiple Choice Questions of chapter UNIFORM CIRCULAR MOTION of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | UNIFORM CIRCULAR MOTION |
| MODERN PUBLICATION | Multiple Choice Questions |
| Number Of Questions | 56 |
| Academic Year | 2023-24 |
A particle moves along a circle of radius`((20)/(pi))` m with constant tangential acceleration. If the velocity of the particle is 80 m/s at the end of the second revolution after motion has begun, the tangential acceleration is:
View SolutionThe maximum and minimum tension in the string whirling in a circle of radius 2.5 m are in the ratio 4: 3 then its velocity is :
View SolutionA particle is kept at rest at the top of a sphere of diameter 42 m. When disturbed slightly, it slides down. At what heighth from the bottom, the particle will leave the sphere :
View SolutionA bucket full of water is revolved in vertical circle of 2 m. What should be the maximum time period of revolution so that the water doesn't fall off the bucket?
View SolutionA tube of length L is filled completely with an incompressible liquid of mass M and closed at both the ends. The tube is then rotated in a horizontal plane about one its ends with a uniform angular velocity 0. The force exerted by the liquid at the other end is:
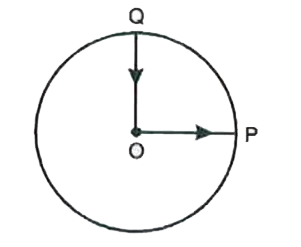
View SolutionA cyclist starts from the centre o of a circular park of radius one kilometre, reaches the edge P of the park, then cycles along the circumference and returns to the centre along QO as shown in the figure. If the round trip takes ten minutes, the net, displacement and average speed of the cyclist (in metre and kilometer per hour) is :
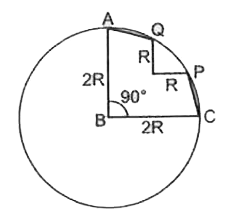
View SolutionA racing path is divided into following parts as shown in the fig ABC is a circular arc of radius 2R.CP and QA are two straight paths each of length R and third part PO is a circular are of radius R = 100 m. A racing car is to go on this track with a maximum uniform speed of 50 ms. The coefficient of friction between the tyres of the car and the circular track is i = 0.1. What is the minimum time in which the car would complete one round of the track?
View SolutionA particle of mass 'me' has a displacement given by the vector `vecr(t)=hatiAcosomegat+hatiBsinomegat`. What is the force acting on it?
View SolutionIn the above question what type of trajectory the body will follow?
View SolutionA cyclist starts from the centre o of a circular or park of radius 1 km and moves along the path OPRQO as shown in the Fig. If he maintains a constant speed of 10 ms', what is his acceleration at the point R both in magnitude and direction?
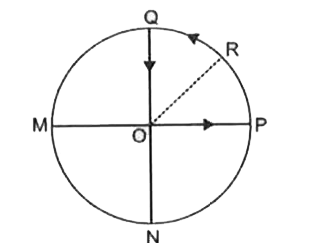
View SolutionIn the question no. 11 how much time is taken by the cyclist to complete one round of the path and what is net displacement ?
View SolutionA car runs at a constant speed on a circular track of radius 100 m, taking 62.8 seconds for every circular lap. The average velocity and average speed for each circular lap respectively is:
View SolutionA cyclist moves at an angle with the vertical along the road. He moves with speed v describing a certain angle, the angle being small. If the speed changes to 2u, then the angle in this case bears a ratio with the former angle:
View SolutionA small object placed on a rotating horizontal turn table just slips, when it is placed at a distance of 4 cm from the axis of rotation. If the angular velocity of the turn is doubled the object slips, when its distance from the axis of rotation is:
View SolutionA car weighing 500 kg can take a turn of radius 50 m with velocity of 36 km/hr. The centripetal force is :
View SolutionIn a bicycle, the radius of rear wheel is twice the radius of front wheel. If `r_(F)` and `r_(r)` are the radil,`v_(F)`and `v_(r)` are the speeds of top most points of wheel. Then:
View SolutionAn athlete completes one round of a circular track of radius R in 40 sec. His displacement at the end of 2 min. 20 sec, will be
View SolutionIn case of uniform circular motion which of the following physical quantity does not remain constant?
View SolutionTwo bodies of equal masses revolve in circular orbits of radii `R_(1)` and `R_(2)` with the same period. Their centripetal forces are in the ratio :
View SolutionA mass of 2 kg is whirled in a horizontal circle at a speed of 5 revolutions/min. keeping the radius constant, the tension in the string is doubled. The new speed nearly
View SolutionThe coefficient of friction between the tyres and the road is 0.25. The maximum speed with which car can be driven round a curve of radius 40 m without skidding is (assume g = 10`ms^(-2)`).
View SolutionThe earth of mass = `6xx10^(24)` kg revolves around th sun with an angular velocity at `2xx10^(-7)`radius in the circular orbit of radius `1.5xx10^(8)` km. The force exerted by the sun on earth is
View SolutionA wheel has a radius 10 cm and it is coupled by a belt to another wheel of radius 30 cm. The smaller wheel increases its speed from rest at a uniform rate of rad/ sec. After how much time will the speed of larger wheel become 100 c.p.m. ?
View SolutionA body is suspended from a smooth horizontal nail by a string of length 0.25 metre. What minimum horizontal velocity should be given to it in the lowest position so that it may move in a complete vertical circle with the nail at the centre ?
View SolutionA stone tied to one end of spring 80 cm long is whirled in a horizontal circle with a constant speed. If stone makes 25 revolutions in 14 sec, the magnitude of acceleration of stone is
View SolutionThe acceleration of a motor car is `8 m/s^(2)`. If the diameter of its wheel be 2 meter, its angular acceleration will be
View SolutionA body is allowed to slide down a frictionless track freely under gravity. The track ends in a semicircular shaped part of diameter D. What should be the height (minimum) from which the body must fall so that completes the circle ?
View SolutionA stone is tied to one end of a spring 50 cm long whirled in a horizontal circle with a constant speed. If the stone makes 10 revolutions in 20 second. The magnitude of acceleration of stone will be:
View SolutionA 2 kg mass is rotating on a circular path of radius 0.8 m with angular velocity of 44 rad/sec. If radius of path suddenly becomes 1 m. Then the value of angular velocity will be:
View SolutionA car is moving at a speed of 72 km/hr. The diameter of its wheels is 0.5 m. If the wheels are stopped in 20 rotations applying brakes. Then angular retardation produced by the brake is :
View SolutionThe string of a pendulum of mass m and length is displaced through `90^(@)`. Minimum strength of the string to withstand the tension will be:
View SolutionA car is moving along a circular arc at a speed of 20 ms! The radius of the arc is 10 m. If the speed is increased at the rate of 30`ms^(-2)`, what is the resultant acceleration ?
View SolutionA planet takes three earth years to complete the orbit round the sun. The orbit of the planet is coplanar with the earth. What will be the time taken by the planet to complete the orbit round the sun with respect to an observer on earth?
View SolutionTwo wheels are connected by a belt. The radius of larger wheel is three times that of the smaller one. What is the ratio of the angular velocity of the two wheels ie.
View SolutionA fighter plane is moving in a vertical circle of radius r. Its minimum velocity at the highest point of the circle will be :
View SolutionA cyclist turns around the curve at 15 km hl. If he turns at double the speed the tendency to over tum is :
View SolutionA cyclist turns around the curve at 15 km hl. If he turns at double the speed the tendency to over tum is :
View SolutionA stone of mass 1 kg tied to a light inextensible of length`L=(10)/(3)`m is whirling in a circular radius L in vertical plane. If the ratio of the maximum tension in the string to the minimum tension in string is 4 and if g is taken to be 10 m 52, the speed of the stone at the highest point of the circle is:
View SolutionA small disc is placed at the top of a hemisphere of radius R What is the smallest horizontal velocity that should be given to the disc for it to leave the hemisphere without sliding down the sphere?
View SolutionA boy is sitting on ajoy wheel at a distance of 5 m from the centre. When the joy wheel is rotated, the boy just slips. If the coefficient of friction is 0.56 between the boy and the platform of the wheel, what is the speed of rotation ?
View SolutionWhen the angular velocity of a uniformly rotating body has increased thrice the resultant of forces applied to it increases by 60 N. Find the acceleration of the body in two cases, if the mass of the body is 3 kg:
View SolutionA body crosses the topmost point with the critical speed, then the ratio of the speeds at point A, B and C is
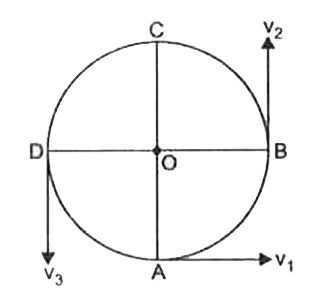
View SolutionA satellite is moving in a circular orbit round the earth and has a period of 24 hours. How many times its velocity should be made so that the periodic time becomes half?
View SolutionA particle goes round a circular path with uniform velocity v. After describing half the circle, the average acceleration of the particle (if its radius is r) is:
View SolutionA sphere of mass 100 g is attached to a string of length 1.3 m whose upper end is fixed to ceilings. The sphere is made to describe a horizontal circle of radius 50 cm. The time period of the revolution is :
View SolutionThe diameter of a solid disc is 0.5 m and its mass is 16kg. What torque is required to increase its angular velocity from zero to 120 revolutions per minute in 8 seconds?
View SolutionIn the above problem at what rate is the work done by the torque at the end of eight second?
View SolutionAn electron revolves round a proton in a hydrogen atom in a circle of radius `5.28 xx 10^(-11)` m with a velocity of `2.18 xx 10^(6)` ms. The acceleration of the 3 electron in ms? will be
View SolutionA simple pendulum of mass mand length L is oscillating on a circular arc with angular amplitude 8. Another ball of mass m is lying stationary on the extreme end B of the are. The momentum imparted by the moving ball to the stationary ball will be.
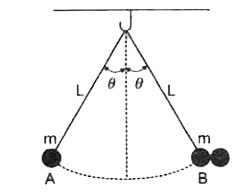
View SolutionA particle performing uniform circular motion has angular momentum L. If its angular frequency is doubled and its kinetic energy halved, then the new angular momentum is :
View SolutionA car is moving in a circular path is radius 500 m with a speed of 30 m/s. If the speed is increased at the rate of 2 m/s, the resultant acceleration will be:
View SolutionA particle undergoes uniform circular motion. About which point on the plane of the circle, will the angular momentum of the particle remain conserved ?
View SolutionConsider a body, shown in figure, consisting of two identical balls, each of mass M connected by a light rod. If an impulse J = MV is imparted to the body at one of its ends, what would be its angular velocity :

View SolutionAn annular ring with inner and outer radii Rand R is rolling without slipping with uniform angular speed 00. The ratio of the forces experienced by the two particles situated at the inner and outer parts of the ring i.e. is :`(F_(1))/(F_(2)`
View SolutionFor a particle in uniform circular motion, the acceleration at a point P `(R.theta)` on the circle of radius Ris (Here`theta` is measured from the x-axis :
View SolutionA point P moves in counter-clockwise direction on a circular path as shown in the Fig
The movement of 'p' is such that it sweeps out a length `s=t^(3)+5` where s is in metres and is in seconds. The radius of the path is 20 m. The acceleration of 'P' when t = 2 s is nearly .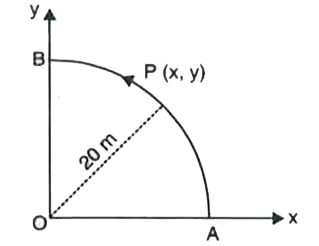
View Solution
Multiple Choice Questions questions and complete solutions for chapter UNIFORM CIRCULAR MOTION of MODERN PUBLICATION PHYSICS (KANNADA ENGLISH) of Class 12