Complete Solutions to Multiple Choice Questions (Level - II) of chapter TRANSFERENCE OF HEAT of Class 12 book with complete answers and questions
| Class 12 | PHYSICS |
| Chapter | TRANSFERENCE OF HEAT |
| MODERN PUBLICATION | Multiple Choice Questions (Level - II) |
| Number Of Questions | 49 |
| Academic Year | 2023-24 |
Two vessels of different materials are similar in size and other respects. The same quantity of ice filled in them gets melted in 40 and 70 minutes respectively. The ration of thermal conductivities for the materials of boxes is :
View SolutionTwo slabs A and B, each though similar, in all other respects, have different materials. They are placed one above the other in perfect contact and a steady difference of temperature of `36^(@)C` is maintained across the combination. If the thermal conductivity of A is twice that of B, what is the temperature of interface ?
View SolutionThere are two spherical balls A and B of the same material with same surface finish but the radius of A is half than of B. If A and B are heated to the same temperature and allowed to cool then :
View SolutionLuminosity of sun is `3.9xx10^(26)` watts. Mean distance of earth from the sun is `1.496xx10^(11)m`. What is the value of solar constant ?
View SolutionIf the tempearture of black body is raised by `5%`, the heat energy radiated would increases by :
View SolutionTwo identical slabs are welded end to end 20 cals of heat flows through it in 4 min. If the two slabs are now welded by placing them one above the other, and same heat is flowing through two ends under the same difference of temperature, the time taken is :
View SolutionThermal resistance of two metallic plates of same material and cross -section joined in series are 3 and 4 respectively. What is thermal resistance of the combination ?
View SolutionRatio of thermal conductivities of two different materials is `4:3` To have same thermal resistance of the two rods of having equal thickness, what should be the ratio of their lengths ?
View SolutionThe ratio of thermal conductivities of two rods is `5:3`. If the thermal resistances of the two rods of same, lengths of the rod is :
View SolutionA wall has two layers A and B, each made of defferent material. A has thickness 10 cm, while B has thickness 20 cm, their coefficients of conductivities are in the ratio of `3:1`. A constant temperature difference of `35^(@)C` exists across the wall. The difference of temperature across the layer A is :
View SolutionDue to fall in main voltage, the temperature of filament of a bulb falls from 3000 K to 2000 K. What is the percentage fall in power consumption ?
View SolutionTwo metal rods of same length have same temperature difference between their ends. Their thermal conductivities are `K_(1)` and `K_(2)` and cross-sections `A_(1)` and `A_(2)` respectively. What is the condition for the same rates of flow of heat ?
View SolutionThe ends of the two rods of conductivities , radii and lengths in the ratio of `1:2` are maintained at the same temperature difference. If the rate of flow through the bigger rod is `12" cal s"^(-1)`, in shorter it will be (in `"cal s"^(-1)`) :
View SolutionA cylindrical rod with one end in steam and other end in ice results in melting of `0.1` g of ice per second. If the rod is replaced by another with half the length and double the radius and `1//4th` the conductivity than that of the first, the rate at which the ice melts in g/sec will be :
View SolutionIn the steady state of conduction of rod AB of length 100 cm, the temperature at the ends A is `80^(@)C` and at B the temperature is `0^(@)C`. The temperature of the rod at the distance 60 cm from A is :
View SolutionTwo spheres of different materials, one with double the radius but `1//4` th wall thickness than other are filled with ice. If the time taken for complete melting of ice in the bigger sohere is 25 minutes and that in smaller is 16 minutes, the ratio of the thermal conductivities of larger to the smaller is :
View SolutionA compound slab is composed of two parallel layers of different materials with thickness 3 cm and 2 cm. The temp of outer face of the slab are maintained at `100^(@)C` and `0^(@)C` If the conductivities are `0.36` and `0.16` cgs units, then the temp. of junction is :
View SolutionThe coefficient of the thermal conductivity of copper is 9 times that of steel . In the composite sylindrical bar shown in the figure , what will be the temperature at the junction of copper and steel ?

View SolutionA solid cube and a solid sphere have equal surface areas. Both are at the same temperature of `120^(@)C`. Then :
View SolutionA cylindrical rod is having temperature `T_(1)` and `T_(2)` at its ends. The rate of flow of heat is `Q_(1)` cal/sec. If all the linear dimensions are doubled keeping temperatures constant, then rate of flow of heat `Q_(2)` will be :
View SolutionThe rate of cooling at 600 K, if surrounding tempearture is 300 K is R. The rate of cooling at 900 K is :
View SolutionA cup of tea cools from `65.5^@C " to " 62.5^@C` in 1 minute in a room at `22.5^@C` How long will it take to cool from `46.5^@C " to " 40.5^@C` C in the same
View SolutionStatement - I : Water kept in an open vessel will evaporate quickly on the surface of moon.
Statement - II : The temperature at the surface of moon is much higher than boiling point of water.
View SolutionStatement - I : A body which is good radiator is is good absorber of radiation at a given wavelength.
Statement - II : According to Kirchhoff's law, the absorptivity of a body is equal to its emissivity at a given wavelength.
View SolutionStatement - I : It is hotter above the top of a fire than at some distance on the sides.
Statement - II : Airsorrounding the fire conducts more heat upwards.
View SolutionStatement - I : A hallow metallic closed containes maintained at a uniform temperature can act as a source of black body radiation.
Statement - II All metals act as a black body
View SolutionStatement - I : The absorbtance of a perfact black body is unity.
Statement - II : A perfect black body when heated emits radiations of all possible wavelength at that temperature.
View SolutionRaed the following passage and and answer the following questions.
Stefan's law states that the ernergy emitted per second per unit area of the perfect black body is directly proportional to fourth power of its absolute temperature i.e. `EpropT^(4)` or `E=sigmaT^(4)` where `sigma` is called Stefan's constant. If the body is placed in enclosure maintained at temperature `T_(0)` then `E=sigma(T^(4)-T_(0)^(4))`. If temperature difference between the body and the surroundings is very small then energy emitted per second per unit area of the body and surrounding i.e `Eprop(T-T_(0))`. This is known as Newton's Law of cooling and is a special case of Stefan's Law.
Two bodies P and Q are having equal surface areas and are maintained at temperature `17^(@)C` and `27^(@)C` respectively. Then radiant energy emitted per second are in the ratio of :
View SolutionA body cools from `50^(@)C` to `40^(@)C` in 5 minutes and from `40^(@)C` to `30^(@)C` in 8 minutes. Then the temperature of the surrounding is
View SolutionTime taken by the body to cool from `45^(@)C` to `35^(@)C` is
View SolutionThe plots of intensity versus wavelength for three black bodies at temperatures `T_(1),T_(2)` and `T_(3)` respecttively are shown in fig. Their temperatures are such that :
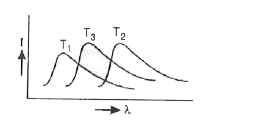
View SolutionTwo spheres of the same material have radii 1 m and 4 m and temperatures 4000 K and 2000 K respectively. The ratio of the energy rediated per second by the first sphere to that by the second is :
View SolutionThe graph shown in the adjacent diagram, represents the variation of temperature T of two bodies x and y having same surface area, with time (t) due to emission of radiation. Find the correct relation between the emissive and adsoptive powers of the two bodies :

View SolutionThree discs A, B and C having radii 2m, 4 m and 6 m respectively are coated with carbon black on their outer surfaces. The wavelengths corresponding to maximum intensity are 300 nm, 400 nm and 500 nm respectively. The power radiated by them are `Q_(A),Q_(B)` and `Q_(C)` respectively :
View SolutionTwo identical conducting rods are first connected independently to two vessels, one containing water at `100^(@)C`, and the other containing ice at `0^(@)C`. In the second case, the rods are joined end to end and connected to the same vessels. Let `q_(1),q_(2)` gram/sec be the rate of melting of ice in the two cases respectively. The ratio `q_(1)//q_(2)` is :
View SolutionA radiation of energy E falls normally on a perfectly reflecting surface. The momentum transferred to the surface is
View SolutionA body with area A at maintained temperature T and emissivity `e=0.6` is kept inside a spherical black body. What will be the maximum energy radiated per second ?
View SolutionIce starts forming in a lake water at `0^(@)C` when the atmospheric temperature is `10^(@)C`. If the time taken for 1 cm of ice to be formed is 7 hour, the time taken for the thickness of the ice to change from 1 cm to 2 cm is :
View SolutionA body is placed in an insulating container at temperature `227^(@)C`. In order to keep its temperature `727^(@)C`, power of 60 W is required, the power required to keep its temperature `1227^(@)C` will be :
View SolutionA cylindrical rod is having temperature `T_(1)` and `T_(2)` at its ends. The rate of flow of heat is `Q_(1)` cal/s. If all the linear dimensions are doubled keeping temperatures constant, the rate of flow of heat `Q_(2)` will be :
View SolutionThe rate of cooling at 600 K, if surrounding tempearture is 300 K is R. The rate of cooling at 900 K is :
View SolutionOne end of a copper rod of length `1.0m` and area of cross-section `10^(-3)m^(2)` is immersed in boiling water and the other ens in ice. If the coefficient of thermal conductivity of copper is 92 cal/m-s- `""^(@)C` and the latent heat of ice is `8xx10^(4)` cal/kg, then the amount of ice which will melt in one munite is :
View SolutionConsider a compound slab consisting of two different materials having equal thickness and thermal conductivities K and 2 K respectively. The equivalent thermal conductivity of the slab is
View SolutionThe dimensions of thermal resistance are :
View SolutionThere is formation of layer of snow x cm thick on water, when the temperature of air is `-theta^(@)C` (less than freezing point). The thickness of layer increased from x to in the time t, then the value of t is given by :
View SolutionSuppose the sun expands, so that its radius becomes 100 times its present radius and its surface temperature becomes half its present value. The total energy emitted by it will increases by a factor of :
View SolutionWhich of the following circular rods, (given radius r and length l) each made of the same material and whose ends are maintaind at the same temperature diff. will conduct most heat ?
View SolutionA hot liquid kept in a breaker cools from `80^(@)C` to `70^(@)C` in two minutes. If the surrounding temperature is `30^(@)C`, then the time of cooling of the same liquid from `60^(@)C` to `50^(@)C` is :
View SolutionThe two ends of a rod of length L and a uniform cross-sectional area A are kept at two temperatures `T _(1)` and `T_(2)` rod in a steady state is given by :
View Solution
Multiple Choice Questions (Level - II) questions and complete solutions for chapter TRANSFERENCE OF HEAT of MODERN PUBLICATION PHYSICS (KANNADA ENGLISH) of Class 12